jzxx2860引水入城
题目描述
在一个遥远的国度,一侧是风景秀美的湖泊,另一侧则是漫无边际的沙漠。该国的行政区划十分特殊,刚好构成一个 N行 M 列的矩形,如上图所示,其中每个格子都代表一座城市,每座城市都有一个海拔高度。
为了使居民们都尽可能饮用到清澈的湖水,现在要在某些城市建造水利设施。水利设施有两种,分别为蓄水厂和输水站。蓄水厂的功能是利用水泵将湖泊中的水抽取到所在城市的蓄水池中。因此,只有与湖泊毗邻的第 1 行的城市可以建造蓄水厂。而输水站的功能则是通过输水管线利用高度落差,将湖水从高处向低处输送。故一座城市能建造输水站的前提,是存在比它海拔更高且拥有公共边的相邻城市,已经建有水利设施。
由于第 N 行的城市靠近沙漠,是该国的干旱区,所以要求其中的每座城市都建有水利设施。那么,这个要求能否满足呢?如果能,请计算最少建造几个蓄水厂;如果不能,求干旱区中不可能建有水利设施的城市数目。
输入
输入文件的每行中两个数之间用一个空格隔开。
输入的第一行是两个正整数 N和 M,表示矩形的规模。
接下来 N行,每行 M 个正整数,依次代表每座城市的海拔高度。
输出
输出有两行。如果能满足要求,输出的第一行是整数 1,第二行是一个整数,代表最少建造几个蓄水厂;如果不能满足要求,输出的第一行是整数 0,第二行是一个整数,代表有几座干旱区中的城市不可能建有水利设施。
样例
输入1
2 5
9 1 5 4 3 1
8 7 6 1 2
输出1
1
输入2
3 6
8 4 5 6 4 4
7 3 4 3 3 3
3 2 2 1 1 2
输出2
1
3
提示
【样例 1 说明】
只需要在海拔为 9 的那座城市中建造蓄水厂,即可满足要求。
【样例 2 说明】
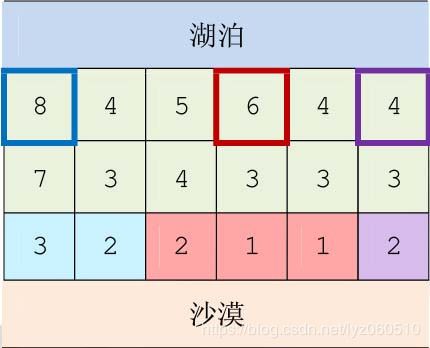
上图中,在 3 个粗线框出的城市中建造蓄水厂,可以满足要求。以这 3个蓄水厂为源头在干旱区中建造的输水站分别用3种颜色标出。当然,建造方法可能不唯一。
传送门
满分代码:
const h:array[1..4,1..2] of longint=((1,0),(-1,0),(0,-1),(0,1));
var col,a:array[0..501,0..501] of longint;
f:array[0..500] of longint;
c:array[0..500,1..2] of longint;
d:array[0..500000] of record x,y:longint;end;
n,m,i,j,color:longint;
function min(a,b:longint):longint;
begin if a<b then exit(a) else exit(b); end;
procedure Judge;
var i,j:longint;
procedure bfs(x,y:integer);
var i,t,f:longint;
begin
t:=1;f:=1;
d[t].x:=x;d[t].y:=y;
col[x,y]:=color;
repeat
for i:=1 to 4 do
if col[d[f].x+h[i,1],d[f].y+h[i,2]]=0 then
if a[d[f].x+h[i,1],d[f].y+h[i,2]]<a[d[f].x,d[f].y] then begin
inc(t);
d[t].x:=d[f].x+h[i,1]; d[t].y:=d[f].y+h[i,2];
col[d[t].x,d[t].y]:=color;
end;
inc(f);
until f>t;
end; {end bfs}
begin {judge}
for i:=0 to n+1 do begin
a[i,0]:=maxlongint;
a[i,m+1]:=maxlongint;
end;
for i:=0 to m+1 do begin
a[0,i]:=maxlongint;
a[n+1,i]:=maxlongint;
end;
for color:=1 to m do bfs(1,color);
j:=0;
for i:=1 to m do
if col[n,i]=0 then inc(j);
if j>0 then begin
writeln(0);
writeln(j);
halt;
end;
end;
procedure floodfill;
procedure bfs(x,y:integer);
var i,t,f:longint;
begin
t:=1;f:=1;
d[t].x:=x;d[t].y:=y;
col[x,y]:=color;
repeat
for i:=1 to 4 do
if col[d[f].x+h[i,1],d[f].y+h[i,2]]=0 then
if a[d[f].x+h[i,1],d[f].y+h[i,2]]>a[d[f].x,d[f].y] then begin
inc(t);
d[t].x:=d[f].x+h[i,1]; d[t].y:=d[f].y+h[i,2];
col[d[t].x,d[t].y]:=color;
end;
inc(f);
until f>t;
end;
var i,j:longint;
begin
for i:=0 to n+1 do begin
a[i,0]:=0; a[i,m+1]:=0;
end;
for i:=0 to m+1 do begin
a[0,i]:=0; a[n+1,i]:=0;
end;
fillchar(col,sizeof(col),0);
for color:=1 to m do
if col[n,color]=0 then
bfs(n,color);
for i:=1 to m do
c[i,1]:=col[1,i];
fillchar(col,sizeof(col),0);
for color:=m downto 1 do
if col[n,color]=0 then bfs(n,color);
for i:=1 to m do c[i,2]:=col[1,i];
end;
procedure DP;
var i,j:longint;
begin
f[0]:=0;
for i:=1 to m do
begin
f[i]:=maxint;
for j:=1 to m do
if (c[j,2]>=i)and(c[j,1]<=i) then
f[i]:=min(f[i],f[c[j,1]-1]+1);
end;
writeln(1);
writeln(f[m]);
end;
BEGIN {main}
read(n,m);
for i:=1 to n do
for j:=1 to m do
read(a[i,j]);
judge;
floodfill;
DP;
END.