- 231转序和321转序的姿态角与四元数的变换关系(文末附VC++代码和Matlab验证代码)
小亨GNC颐园
matlabVC++运载火箭321转序231转序导弹导航初始化
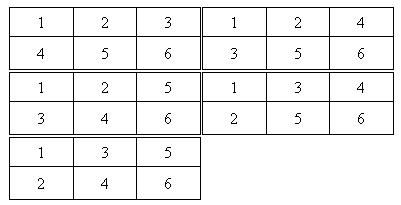
近程战术导弹的转序一般采用231的顺序,先偏航、后俯仰、再滚转。远程导弹、运载火箭、某些垂直发射拦截导弹的初制导段会采用321的转序,先俯仰、后偏航、再滚转。这两种转下的姿态角与四元数的转换关系如下:321转序//--------惯性坐标系到箭体系的四元数--------------------//doublesic_T=sin(Theta_T_rad/2.0);余下的VC++代码和Matlab代
- Python常考面试题汇总(附答案)
TT图图
面试职场和发展
写在前面本文面向中高级Python开发,太基本的题目不收录。本文只涉及Python相关的面试题,关于网络、MySQL、算法等其他面试必考题会另外开专题整理。不是单纯的提供答案,抵制八股文!!更希望通过代码演示,原理探究等来深入讲解某一知识点,做到融会贯通。部分演示代码也放在了我的github的该目录下。语言基础篇Python的基本数据类型Python3中有六个标准的数据类型:Number(数字)(
- spring中@Transactional注解和事务的实战理解附代码
GJCTYU
springoracle数据库springbootmybatis后端
文章目录前言一、事务是什么?二、事务的特性2.1隔离性2.2事务的隔离级别三、@Transactional注解@Transactional注解简介基本用法常用属性配置事务传播行为事务隔离级别异常处理与回滚性能优化建议四、事务不生效的可能原因方法访问权限非public自调用问题异常被捕获未抛出数据库引擎不支持事务未启用事务管理特殊场景:final/static方法五、分布式事务考虑总结前言在开发过程
- 2024年11月架构设计师论文真题回顾,附参考解答、解析及所涉知识点(一)
一几文
架构系统架构系统架构设计师软考高级IT考证
软考高级系统架构设计师考试包含三个科目:信息系统综合知识、案例分析和系统架构设计论文。考试形式为机考。本文主要回顾2024年下半年(2024-11-10)系统架构设计师考试下午论文的题目,同时附带参考解答、解析和所涉知识点。综合知识2024年11月架构设计师综合知识真题回顾,附参考答案、解析及所涉知识点(一)2024年11月架构设计师综合知识真题回顾,附参考答案、解析及所涉知识点(二)2024年1
- python实现多元线性回归算法 (附完整源码)
源代码大师
python算法完整教程算法python线性回归
python实现多元线性回归算法1.使用正规方程实现多元线性回归代码说明运行结果示例2.使用梯度下降法实现多元线性回归代码说明运行结果示例进一步优化与注意事项下面是使用Python从头实现多元线性回归算法的完整源码。这个实现利用了numpy进行矩阵运算,并展示了如何训练模型、进行预测以及评估模型性能。为了更全面,代码中还包含了一个使用梯度下降法(GradientDescent)优化参数的实现。多元
- Neo4j Windows 3.5 详细安装教程
赵允静Joy
Neo4jWindows3.5详细安装教程neo4jwindows3.5详细安装教程附百度云链接项目地址:https://gitcode.com/Resource-Bundle-Collection/1af38本资源文件提供了Neo4jWindows3.5版本的详细安装教程,帮助用户快速完成Neo4j的安装和配置。教程内容包括从下载、解压、配置环境变量到启动Neo4j服务的全过程。安装步骤访问官网
- Transformer已死?2025年十大替代架构实战评测
前言前些天发现了一个巨牛的人工智能免费学习网站,通俗易懂,风趣幽默,忍不住分享一下给大家。点击跳转到网站封面图建议:十大架构3D渲染图环绕碎裂的Transformer图标,背景为动态性能雷达图副标题:实测推理速度/显存占用/长文本能力,附迁移成本决策树一、争议源起:Transformer的时代性局限(2025版)graphLRA[Transformer痛点]-->B[显存黑洞:千亿模型推理需1.6
- 生物启发AI新突破:神经形态芯片+脉冲神经网络落地指南
HeartException
人工智能
前言前些天发现了一个巨牛的人工智能免费学习网站,通俗易懂,风趣幽默,忍不住分享一下给大家。点击跳转到网站《生物启发AI新突破:神经形态芯片+脉冲神经网络落地指南》副标题:基于2025年英特尔Loihi3芯片的工业级部署实战(附能耗对比&代码库)封面建议:脉冲神经网络动态脉冲传导图覆盖在神经形态芯片显微结构上,标注「能效比:传统GPU的1/800」一、2025生物启发AI的临界点突破生物神经特性事件
- 《卷积神经网络到Vision Transformer:计算机视觉的十年架构革命》
HeartException
人工智能学习
前言前些天发现了一个巨牛的人工智能免费学习网站,通俗易懂,风趣幽默,忍不住分享一下给大家。点击跳转到网站题目《卷积神经网络到VisionTransformer:计算机视觉的十年架构革命》展开深度解析,全文采用技术演进史+架构对比+产业影响的三段式结构,附关键数据与趋势预测:卷积神经网络到VisionTransformer:计算机视觉的十年架构革命副标题:从局部感知到全局建模,一场改变AI视觉基石的
- 阿里P7面试实录:靠这份“收割机指南”,他当场拿下60k+ offer!
“上周面了个前阿里P7,Java八股文和分布式架构原理背得炉火纯青,秒杀系统设计讲得比我们架构组还细!”一位蚂蚁金服面试官在技术群感慨道。细问才知,这位求职者刚用一份阿里内部流出的《后端offer收割机养成指南》突击了2周,直接通过6轮面试斩下60k+offer。2025年Java后端面试的3大新趋势(附高频考点)据近期阿里、字节、美团等大厂面试反馈,技术考察正发生显著变化:八股文升级场景化基础题
- 《ARM64 迁移深度实战:在飞腾 D2000+ 麒麟 V10 构建高可用全栈环境》
2301_82150492
pythonc++c语言c#
从源码编译优化到容器跨架构迁移|附自研文档转换工具开发全记录目录(带锚点)环境深度适配:飞腾芯片+KylinOS安全内核特性基础组件迁移(源码级优化)2.1JDK17GraalVMARM编译指南(性能提升40%)2.2MySQL8.0深度适配(解决麒麟安全模块冲突)2.3Redis7.0内存池优化(ARMNUMA架构调优)容器化迁移企业级实践3.1Docker离线安装+麒麟内核模块编译3.2构建多
- 【网络通信安全】OSPF 邻居建立全过程解析:从状态机到实战排错(附 eNSP 动态验证)
不羁。。
网络通信安全开发语言网络运维安全
目录一、引言:OSPF如何构建“网络对话”?二、OSPF邻居状态机:8阶段状态转换图三、逐状态深度解析:每个阶段在“干什么”?1.Down状态:对话的起点2.Attempt状态:NBMA网络的“主动连接”3.Init状态:“我知道你存在”4.2-Way状态:双向通信达成5.Exstart状态:主从关系确立6.Exchange状态:LSDB摘要交换7.Loading状态:补全缺失的LSA8.Full
- 【网络通信安全】深入解析 OSPF 协议:从概念到 eNSP 实战配置(附完整代码与排错指南)
不羁。。
网络通信安全智能路由器网络
目录一、OSPF协议核心概念:为什么它是企业网络的“神经网络”?1.协议本质与设计目标2.核心组件与工作原理(1)链路状态数据库(LSDB)(2)区域划分原则(3)路由器角色二、实验环境搭建:3台路由器构建跨区域OSPF网络1.网络拓扑图2.设备与IP规划表三、逐设备配置详解:从接口到OSPF进程的全流程操作1.基础配置:接口IP与设备命名(以R1为例)2.OSPF进程配置:区域划分与网络宣告(1
- Dockerfile 设定镜像时区
半吊子游戏开发者
我整理的一些关于【Docker】的项目学习资料(附讲解~~)和大家一起分享、学习一下:https://d.51cto.com/f2PFnNDockerfile设定镜像时区指南在Docker中,镜像的时区配置常常被忽视,但这对于确保应用在运行时的时间正确性至关重要。在这篇文章中,我将指导你如何通过Dockerfile设定镜像的时区,并提供一个清晰的操作流程。一、步骤流程我们将整个过程分为几个步骤,以
- 2025年Java后端岗互联网大厂技术场景题的总结(附100w字面试题)
小凡敲代码
javajava后端java面试Java面试题互联网大厂求职Java场景题
一、高并发与分布式系统设计1.百万级QPS秒杀系统问题:如何设计支持瞬时高并发的秒杀系统?解决方案:Redis预减库存:使用Lua脚本保证原子性操作,防止超卖。异步下单:通过MQ(如Kafka/RocketMQ)削峰,降低数据库压力。限流降级:Sentinel/Nginx限流,防止恶意请求。热点数据隔离:独立Redis集群存储秒杀商品数据。2.分布式事务一致性问题:跨服务下单如何保证数据一致性?方
- Eureka、Nacos、LoadBalance、OpenFeign之间的区别联系和协作 (附代码讲解)
这篇文章聊聊微服务里的这几个老伙计:Eureka、Nacos、LoadBalance、OpenFeign。咱们做微服务开发,总会跟这几个组件打交道:Eureka、Nacos、SpringCloudLoadBalancer、OpenFeign。它们各司其职又互相配合,今天就把它们的关系、用法捋清楚,再上点代码示例,保证看完就明白。不然很久不用就忘了。先从注册中心说起,这俩货是服务的"通讯录"不管是E
- 25年最新Java后端社招场景项目题总结!(附100w字面试题)
小凡敲代码
javajava面试Java面试题Java场景题程序员互联网大厂计算机
一、高并发与分布式系统设计百万级QPS秒杀系统核心问题:如何解决超卖、库存一致性、高并发请求?技术方案:Redis预减库存+异步扣减(Kafka/RocketMQ)分布式锁(Redisson)或乐观锁(CAS)限流策略(Nginx/Sentinel)扩展:热点数据隔离(独立Redis集群)、风控防刷(IP限流、验证码)。分布式文件存储系统(类似GFS)需求:支持海量文件存储、高可用、快速检索。关键
- 全素山药开发指南:从防痒处理到高可用食谱架构
109702008
杂谈人工智能
摘要:本文系统性解析山药的化学特性(黏液蛋白/皂苷致痒机制)及全素场景下的烹饪解决方案,提供6种高内聚低耦合的食谱实现,附完整防氧化与黏液控制技术方案。一、核心问题分析:山药处理中的“痛点”致痒物质皂角素+植物碱→刺激皮肤神经末梢解决方案(5种设计模式):graphLRA[防痒方案]-->B[物理隔离-手套]A-->C[化学中和-醋/油涂层]A-->D[热力破坏-蒸煮预处理]氧化发黑酚类物质+氧气
- Rust+Blender:打造高性能游戏引擎
KENYCHEN奉孝
Rustrust开发工具
基于Rust和Blender的游戏引擎以下是基于Rust和Blender的游戏引擎开发实例,涵盖不同应用场景和技术方向的实际案例。案例分为工具链整合、渲染技术、物理模拟等类别,每个案例附核心代码片段或实现逻辑。工具链整合案例案例1:Blender模型导出到Bevy引擎使用blender-bevy-io插件将Blender模型导出为Bevy支持的.gltf格式。关键步骤包括在Blender中设置自定
- SpringBoot电商项目实战:从零搭建百万级架构
博主介绍:Java、Python、js全栈开发“多面手”,精通多种编程语言和技术,痴迷于人工智能领域。秉持着对技术的热爱与执着,持续探索创新,愿在此分享交流和学习,与大家共进步。DeepSeek-行业融合之万象视界(附实战案例详解100+)全栈开发环境搭建运行攻略:多语言一站式指南(环境搭建+运行+调试+发布+保姆级详解)感兴趣的可以先收藏起来,希望帮助更多的人SpringBoot电商项目实战:从
- 【机器学习|学习笔记】类别特征(Categorical Features)处理方法,附代码。
努力毕业的小土博^_^
机器学习学习笔记机器学习学习笔记神经网络人工智能深度学习
【机器学习|学习笔记】类别特征(CategoricalFeatures)处理方法,附代码。【机器学习|学习笔记】类别特征(CategoricalFeatures)处理方法,附代码。文章目录【机器学习|学习笔记】类别特征(CategoricalFeatures)处理方法,附代码。前言✅为什么要处理类别特征?原因1:大多数模型不能处理字符串原因2:避免“错误的顺序假设”原因3:方便模型泛化与特征交互✅
- 【信号去噪】基于NLM时间序列心电信号去噪附matlab代码
天天Matlab科研工作室
信号处理Matlab各类代码matlab开发语言fpga开发
1简介作为一种信号预处理手段,信号去噪在众多信号处理应用中发挥着重要的作用.到目前为止,信号去噪问题被大量研究,并取得了许多重要成果,涌现出了包括非局部均值(NLM)去噪算法在内的一批优秀的去噪方法.值得一提的是,相比于传统的局部去噪算法,非局部均值去噪算法有着更好的去噪性能和更好的信号细节保留能力.2部分代码function[denoisedSig,debug]=NLM_1dDarbon(sig
- 关于信号降噪的一些方法
我不是哆啦A梦
故障诊断人工智能信号处理机器学习算法
在通信系统中传输信号或在接收信号的同时,一些不需要的信号被引入到通信中,使接收机信号变差,从而影响了通信质量,一般称这些干扰称为噪声。从而,可以理解噪声是一些没有模式的信号,其特点是没有恒定的频率或振幅,且随机性强,不能完全消除。常用的信号降噪方法有以下这些:(1)滑动平均法(movingaverage)也叫做移动平均法、移动平均值滤波法等等,是一种时间域思想上的信号光滑方法。算法思路为:将该点附
- MySQL Buffer Pool 深度解析:从架构设计到性能优化(附详细结构图解)
小W求学之旅
mysql性能优化数据库springjavaBufferPool
在MySQL数据库的世界里,有一个决定性能上限的"神秘仓库"——BufferPool。它就像超市的货架,把最常用的商品(数据)放在最方便拿取的地方,避免每次都要去仓库(磁盘)取货。今天我们就来深入了解BufferPool内部结构,以及它如何在高并发场景下高效工作。一、BufferPool:数据库的"高速缓存"1.1为什么需要BufferPool?想象一下:磁盘读写速度约为100次/秒,而内存读写速
- 【ICLR 2022】时序精选论文08|Pyraformer: 基于金字塔注意力机制与多尺度辨识卷积的时间序列预测模型(代码解读附源码)
OverOnEarth
时间序列预测项目实战人工智能机器学习深度学习python算法
ICLR2022PYRAFORMER:LOW-COMPLEXITYPYRAMIDALAT-TENTIONFORLONG-RANGETIMESERIESMODELINGANDFORECASTINGPyraformer要解决的问题基于时间序列数据面临的挑战:建立一个灵活但简约的模型,能够捕获不同范围的时间依赖性。时间序列通常表现为短期和长期的重复模式,将他们考虑在内是准确预测的关键。即能够获得一个同时
- Cursor黑科技实战:AI编程革命,效率提升300%的秘籍
IT莫染
科技AI编程pythonCursorProDeepSeek-CoderAI重构
目录⚡程序员痛点诊断Cursor核心黑科技揭秘实战演示(附操作截图)场景1:AI秒杀样板代码(效率提升10倍)场景2:智能Debug(精准定位问题)场景3:跨语言迁移(Java→Python)生产力暴涨秘籍黑科技1:AI结对编程(Ctrl+L)黑科技2:代码气味检测(Ctrl+Shift+K)黑科技3:SQL优化(自然语言转SQL)避坑指南:Cursor三大死亡陷阱陷阱1:过度生成幻觉代码陷阱2:
- 国内知名的语音芯片厂家介绍(附主要产品线和型号)
唯创知音
语音识别人工智能
这个厂商成1999年成立于广州,随后2000年进军语音芯片行业,2003年研发出叉车超速报警器,2004年研发出MP3音频芯片,并提供MP3方案,随着业务快速扩张2014年搬迁至深圳并更名深圳唯创知音电子有限公司。唯创知音旗下主要产品线介绍1.OTP语音芯片型号:WTN6020(20秒)、WTN6040(40秒)、WTN6080(80秒)、WTN6170(170秒)特点:低功耗(待机电流≤2μA)
- 分享我自己的导航网站bituplink导航 (附GitHub开源代码项目OneHtmlNav)
bituplink
分享导航GitHub开源bituplink分享
话不多说,先上资源biuplink导航:http://www.bituplink.com/goodwebOneHtmlNav项目:https://github.com/bituplink/OneHtmlNavOneHtmlNav下载:本地下载或者github直接打包下载小呆导航源项目:https://github.com/xiaodai945/WEBJIKE然后上图再然后说说这个项目的初衷最近新建
- 奇偶校验位学习理解
幸运学者
verilog编码学
一个字节一般有8bit,若是需要进行奇偶校验需要添加一个码元,所以发送接收时是8位数据位,一位校验位。奇偶校验就是指每次发送接收的每一帧数据加上校验码之后1的个数是奇数还是偶数奇偶校验码是奇校验码和偶校验码的统称,是一种最基本的检错码。它是由n-1位信息元和1位校验元组成,可以表示成为(n,n-1)。如果是奇校验码,在附加上一个校验元以后,码长为n的码字中“1”的个数为奇数个;如果是偶校验码,在附
- Python 3.7 安装包及详细安装指南:助你轻松搭建开发环境
金琴莺
Python3.7安装包及详细安装指南:助你轻松搭建开发环境python3.7安装包附安装教程.zip项目地址:https://gitcode.com/open-source-toolkit/a278c项目介绍在编程的世界里,Python以其简洁易读的语法和强大的功能,成为了众多开发者的首选语言。然而,对于初学者或需要在Windows系统上搭建Python开发环境的用户来说,安装Python可能是
- java线程的无限循环和退出
3213213333332132
java
最近想写一个游戏,然后碰到有关线程的问题,网上查了好多资料都没满足。
突然想起了前段时间看的有关线程的视频,于是信手拈来写了一个线程的代码片段。
希望帮助刚学java线程的童鞋
package thread;
import java.text.SimpleDateFormat;
import java.util.Calendar;
import java.util.Date
- tomcat 容器
BlueSkator
tomcatWebservlet
Tomcat的组成部分 1、server
A Server element represents the entire Catalina servlet container. (Singleton) 2、service
service包括多个connector以及一个engine,其职责为处理由connector获得的客户请求。
3、connector
一个connector
- php递归,静态变量,匿名函数使用
dcj3sjt126com
PHP递归函数匿名函数静态变量引用传参
<!doctype html>
<html lang="en">
<head>
<meta charset="utf-8">
<title>Current To-Do List</title>
</head>
<body>
- 属性颜色字体变化
周华华
JavaScript
function changSize(className){
var diva=byId("fot")
diva.className=className;
}
</script>
<style type="text/css">
.max{
background: #900;
color:#039;
- 将properties内容放置到map中
g21121
properties
代码比较简单:
private static Map<Object, Object> map;
private static Properties p;
static {
//读取properties文件
InputStream is = XXX.class.getClassLoader().getResourceAsStream("xxx.properti
- [简单]拼接字符串
53873039oycg
字符串
工作中遇到需要从Map里面取值拼接字符串的情况,自己写了个,不是很好,欢迎提出更优雅的写法,代码如下:
import java.util.HashMap;
import java.uti
- Struts2学习
云端月影
最近开始关注struts2的新特性,从这个版本开始,Struts开始使用convention-plugin代替codebehind-plugin来实现struts的零配置。
配置文件精简了,的确是简便了开发过程,但是,我们熟悉的配置突然disappear了,真是一下很不适应。跟着潮流走吧,看看该怎样来搞定convention-plugin。
使用Convention插件,你需要将其JAR文件放
- Java新手入门的30个基本概念二
aijuans
java新手java 入门
基本概念: 1.OOP中唯一关系的是对象的接口是什么,就像计算机的销售商她不管电源内部结构是怎样的,他只关系能否给你提供电就行了,也就是只要知道can or not而不是how and why.所有的程序是由一定的属性和行为对象组成的,不同的对象的访问通过函数调用来完成,对象间所有的交流都是通过方法调用,通过对封装对象数据,很大限度上提高复用率。 2.OOP中最重要的思想是类,类是模板是蓝图,
- jedis 简单使用
antlove
javarediscachecommandjedis
jedis.RedisOperationCollection.java
package jedis;
import org.apache.log4j.Logger;
import redis.clients.jedis.Jedis;
import java.util.List;
import java.util.Map;
import java.util.Set;
pub
- PL/SQL的函数和包体的基础
百合不是茶
PL/SQL编程函数包体显示包的具体数据包
由于明天举要上课,所以刚刚将代码敲了一遍PL/SQL的函数和包体的实现(单例模式过几天好好的总结下再发出来);以便明天能更好的学习PL/SQL的循环,今天太累了,所以早点睡觉,明天继续PL/SQL总有一天我会将你永远的记载在心里,,,
函数;
函数:PL/SQL中的函数相当于java中的方法;函数有返回值
定义函数的
--输入姓名找到该姓名的年薪
create or re
- Mockito(二)--实例篇
bijian1013
持续集成mockito单元测试
学习了基本知识后,就可以实战了,Mockito的实际使用还是比较麻烦的。因为在实际使用中,最常遇到的就是需要模拟第三方类库的行为。
比如现在有一个类FTPFileTransfer,实现了向FTP传输文件的功能。这个类中使用了a
- 精通Oracle10编程SQL(7)编写控制结构
bijian1013
oracle数据库plsql
/*
*编写控制结构
*/
--条件分支语句
--简单条件判断
DECLARE
v_sal NUMBER(6,2);
BEGIN
select sal into v_sal from emp
where lower(ename)=lower('&name');
if v_sal<2000 then
update emp set
- 【Log4j二】Log4j属性文件配置详解
bit1129
log4j
如下是一个log4j.properties的配置
log4j.rootCategory=INFO, stdout , R
log4j.appender.stdout=org.apache.log4j.ConsoleAppender
log4j.appender.stdout.layout=org.apache.log4j.PatternLayout
log4j.appe
- java集合排序笔记
白糖_
java
public class CollectionDemo implements Serializable,Comparable<CollectionDemo>{
private static final long serialVersionUID = -2958090810811192128L;
private int id;
private String nam
- java导致linux负载过高的定位方法
ronin47
定位java进程ID
可以使用top或ps -ef |grep java
![图片描述][1]
根据进程ID找到最消耗资源的java pid
比如第一步找到的进程ID为5431
执行
top -p 5431 -H
![图片描述][2]
打印java栈信息
$ jstack -l 5431 > 5431.log
在栈信息中定位具体问题
将消耗资源的Java PID转
- 给定能随机生成整数1到5的函数,写出能随机生成整数1到7的函数
bylijinnan
函数
import java.util.ArrayList;
import java.util.List;
import java.util.Random;
public class RandNFromRand5 {
/**
题目:给定能随机生成整数1到5的函数,写出能随机生成整数1到7的函数。
解法1:
f(k) = (x0-1)*5^0+(x1-
- PL/SQL Developer保存布局
Kai_Ge
近日由于项目需要,数据库从DB2迁移到ORCAL,因此数据库连接客户端选择了PL/SQL Developer。由于软件运用不熟悉,造成了很多麻烦,最主要的就是进入后,左边列表有很多选项,自己删除了一些选项卡,布局很满意了,下次进入后又恢复了以前的布局,很是苦恼。在众多PL/SQL Developer使用技巧中找到如下这段:
&n
- [未来战士计划]超能查派[剧透,慎入]
comsci
计划
非常好看,超能查派,这部电影......为我们这些热爱人工智能的工程技术人员提供一些参考意见和思想........
虽然电影里面的人物形象不是非常的可爱....但是非常的贴近现实生活....
&nbs
- Google Map API V2
dai_lm
google map
以后如果要开发包含google map的程序就更麻烦咯
http://www.cnblogs.com/mengdd/archive/2013/01/01/2841390.html
找到篇不错的文章,大家可以参考一下
http://blog.sina.com.cn/s/blog_c2839d410101jahv.html
1. 创建Android工程
由于v2的key需要G
- java数据计算层的几种解决方法2
datamachine
javasql集算器
2、SQL
SQL/SP/JDBC在这里属于一类,这是老牌的数据计算层,性能和灵活性是它的优势。但随着新情况的不断出现,单纯用SQL已经难以满足需求,比如: JAVA开发规模的扩大,数据量的剧增,复杂计算问题的涌现。虽然SQL得高分的指标不多,但都是权重最高的。
成熟度:5星。最成熟的。
- Linux下Telnet的安装与运行
dcj3sjt126com
linuxtelnet
Linux下Telnet的安装与运行 linux默认是使用SSH服务的 而不安装telnet服务 如果要使用telnet 就必须先安装相应的软件包 即使安装了软件包 默认的设置telnet 服务也是不运行的 需要手工进行设置 如果是redhat9,则在第三张光盘中找到 telnet-server-0.17-25.i386.rpm
- PHP中钩子函数的实现与认识
dcj3sjt126com
PHP
假如有这么一段程序:
function fun(){
fun1();
fun2();
}
首先程序执行完fun1()之后执行fun2()然后fun()结束。
但是,假如我们想对函数做一些变化。比如说,fun是一个解析函数,我们希望后期可以提供丰富的解析函数,而究竟用哪个函数解析,我们希望在配置文件中配置。这个时候就可以发挥钩子的力量了。
我们可以在fu
- EOS中的WorkSpace密码修改
蕃薯耀
修改WorkSpace密码
EOS中BPS的WorkSpace密码修改
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
蕃薯耀 201
- SpringMVC4零配置--SpringSecurity相关配置【SpringSecurityConfig】
hanqunfeng
SpringSecurity
SpringSecurity的配置相对来说有些复杂,如果是完整的bean配置,则需要配置大量的bean,所以xml配置时使用了命名空间来简化配置,同样,spring为我们提供了一个抽象类WebSecurityConfigurerAdapter和一个注解@EnableWebMvcSecurity,达到同样减少bean配置的目的,如下:
applicationContex
- ie 9 kendo ui中ajax跨域的问题
jackyrong
AJAX跨域
这两天遇到个问题,kendo ui的datagrid,根据json去读取数据,然后前端通过kendo ui的datagrid去渲染,但很奇怪的是,在ie 10,ie 11,chrome,firefox等浏览器中,同样的程序,
浏览起来是没问题的,但把应用放到公网上的一台服务器,
却发现如下情况:
1) ie 9下,不能出现任何数据,但用IE 9浏览器浏览本机的应用,却没任何问题
- 不要让别人笑你不能成为程序员
lampcy
编程程序员
在经历六个月的编程集训之后,我刚刚完成了我的第一次一对一的编码评估。但是事情并没有如我所想的那般顺利。
说实话,我感觉我的脑细胞像被轰炸过一样。
手慢慢地离开键盘,心里很压抑。不禁默默祈祷:一切都会进展顺利的,对吧?至少有些地方我的回答应该是没有遗漏的,是不是?
难道我选择编程真的是一个巨大的错误吗——我真的永远也成不了程序员吗?
我需要一点点安慰。在自我怀疑,不安全感和脆弱等等像龙卷风一
- 马皇后的贤德
nannan408
马皇后不怕朱元璋的坏脾气,并敢理直气壮地吹耳边风。众所周知,朱元璋不喜欢女人干政,他认为“后妃虽母仪天下,然不可使干政事”,因为“宠之太过,则骄恣犯分,上下失序”,因此还特地命人纂述《女诫》,以示警诫。但马皇后是个例外。
有一次,马皇后问朱元璋道:“如今天下老百姓安居乐业了吗?”朱元璋不高兴地回答:“这不是你应该问的。”马皇后振振有词地回敬道:“陛下是天下之父,
- 选择某个属性值最大的那条记录(不仅仅包含指定属性,而是想要什么属性都可以)
Rainbow702
sqlgroup by最大值max最大的那条记录
好久好久不写SQL了,技能退化严重啊!!!
直入主题:
比如我有一张表,file_info,
它有两个属性(但实际不只,我这里只是作说明用):
file_code, file_version
同一个code可能对应多个version
现在,我想针对每一个code,取得它相关的记录中,version 值 最大的那条记录,
SQL如下:
select
*
- VBScript脚本语言
tntxia
VBScript
VBScript 是基于VB的脚本语言。主要用于Asp和Excel的编程。
VB家族语言简介
Visual Basic 6.0
源于BASIC语言。
由微软公司开发的包含协助开发环境的事
- java中枚举类型的使用
xiao1zhao2
javaenum枚举1.5新特性
枚举类型是j2se在1.5引入的新的类型,通过关键字enum来定义,常用来存储一些常量.
1.定义一个简单的枚举类型
public enum Sex {
MAN,
WOMAN
}
枚举类型本质是类,编译此段代码会生成.class文件.通过Sex.MAN来访问Sex中的成员,其返回值是Sex类型.
2.常用方法
静态的values()方