- C++11堆操作深度解析:std::is_heap与std::is_heap_until原理解析与实践
文章目录堆结构基础与函数接口堆的核心性质函数签名与核心接口std::is_heapstd::is_heap_until实现原理深度剖析std::is_heap的验证逻辑std::is_heap_until的定位策略算法优化细节代码实践与案例分析基础用法演示自定义比较器实现最小堆检查边缘情况处理性能分析与实际应用时间复杂度对比典型应用场景与手动实现的对比注意事项与最佳实践迭代器要求比较器设计C++标
- 上位机知识篇---SD卡&U盘镜像
常用的镜像烧录软件balenaEtcherbalenaEtcher是一个开源的、跨平台的工具,用于将操作系统镜像文件(如ISO和IMG文件)烧录到SD卡和USB驱动器中。以下是其使用方法、使用场景和使用注意事项的介绍:使用方法下载安装:根据自己的操作系统,从官方网站下载对应的安装包。Windows系统下载.exe文件后双击安装;Linux系统若下载的是.deb文件,可在终端执行“sudodpkg-
- JavaScript 树形菜单总结
Auscy
microsoft
树形菜单是前端开发中常见的交互组件,用于展示具有层级关系的数据(如文件目录、分类列表、组织架构等)。以下从核心概念、实现方式、常见功能及优化方向等方面进行总结。一、核心概念层级结构:数据以父子嵌套形式存在,如{id:1,children:[{id:2}]}。节点:树形结构的基本单元,包含自身信息及子节点(若有)。展开/折叠:子节点的显示与隐藏切换,是树形菜单的核心交互。递归渲染:因数据层级不固定,
- 全面触摸屏输入法设计与实现
长野君
本文还有配套的精品资源,点击获取简介:触摸屏输入法是针对触摸设备优化的文字输入方案,包括虚拟键盘、手写、语音识别和手势等多种输入方式。本方案通过提供主程序文件、用户手册、界面截图、示例图、说明文本和音效文件,旨在为用户提供一个完整的、多样的文字输入体验。开发者通过持续优化算法和用户界面,使用户在无物理键盘环境下也能高效准确地进行文字输入。1.触摸屏输入法概述简介在现代信息技术飞速发展的今天,触摸屏
- 嵌入式系统LCD显示模块编程实践
本文还有配套的精品资源,点击获取简介:本文档提供了一个具有800x480分辨率的3.5英寸液晶显示模块LW350AC9001的驱动程序代码,以及嵌入式系统中使用C/C++语言进行硬件编程的实践指南。该模块的2mm厚度使其适用于空间受限的便携式设备。内容包括驱动程序源代码、硬件控制接口使用方法,以及如何在嵌入式系统中进行图形处理、电源管理与性能优化。1.嵌入式系统原理1.1嵌入式系统概念嵌入式系统是
- 算法学习笔记:15.二分查找 ——从原理到实战,涵盖 LeetCode 与考研 408 例题
呆呆企鹅仔
算法学习算法学习笔记考研二分查找
在计算机科学的查找算法中,二分查找以其高效性占据着重要地位。它利用数据的有序性,通过不断缩小查找范围,将原本需要线性时间的查找过程优化为对数时间,成为处理大规模有序数据查找问题的首选算法。二分查找的基本概念二分查找(BinarySearch),又称折半查找,是一种在有序数据集合中查找特定元素的高效算法。其核心原理是:通过不断将查找范围减半,快速定位目标元素。与线性查找逐个遍历元素不同,二分查找依赖
- MySQL Explain 详解:从入门到精通,让你的 SQL 飞起来
引言:为什么Explain是SQL优化的“照妖镜”?在Java开发中,我们常常会遇到数据库性能瓶颈的问题。一条看似简单的SQL语句,在数据量增长到一定规模后,可能会从毫秒级响应变成秒级甚至分钟级响应,直接拖慢整个应用的性能。此时,你是否曾困惑于:为什么这条SQL突然变慢了?索引明明建了,为什么没生效?到底是哪里出了问题?答案就藏在MySQL的EXPLAIN命令里。EXPLAIN就像一面“照妖镜”,
- kube-scheduler 抢占机制
分享放大价值
kubernetes源码分析kuberneteskube-scheduler抢占
当pod调度失败后,会在PostFilter扩展点执行抢占流程,下面分析相关的代码实现抢占接口//PodNominatorabstractsoperationstomaintainnominatedPods.typePodNominatorinterface{//将pod加入抢占成功的node中AddNominatedPod(pod*PodInfo,nodeNamestring)//将pod从no
- 前端 NPM 包的依赖可视化分析工具推荐
前端视界
前端艺匠馆前端npmarcgisai
前端NPM包的依赖可视化分析工具推荐关键词:NPM、依赖管理、可视化分析、前端工程、包管理、依赖冲突、性能优化摘要:本文将深入探讨前端开发中NPM包依赖可视化分析的重要性,介绍5款主流工具的使用方法和特点,并通过实际案例展示如何利用这些工具优化项目依赖结构、解决版本冲突问题以及提升构建性能。文章将帮助开发者更好地理解和掌控项目依赖关系,提高开发效率和项目可维护性。背景介绍目的和范围本文旨在为前端开
- 蓝牙MTU含义 ,协商修改的过程案例分析
悟空胆好小
嵌入式硬件网络人工智能
蓝牙MTU含义,协商修改的过程案例分析文章目录**蓝牙MTU含义,协商修改的过程案例分析****一、MTU含义解析****二、MTU协商过程详解****步骤流程****三、修改MTU的实践案例分析****案例1:中心设备主动设置(主控端)****案例2:外设端响应优化(从设备)****案例3:调试工具强制修改****四、关键限制与注意事项**蓝牙MTU(MaximumTransmissionUni
- Kimi Chat 1.5 与 2.0 架构升级对比
charles666666
人工智能transformer深度学习产品经理chatgpt
1.5版的MoE架构优化KimiChat1.5采用了优化后的MoE架构,其核心在于“专家网络动态路由”。这一机制类似于快递系统智能选择最优路径,能够根据输入数据的特性动态分配计算资源。这种优化显著提升了模型的计算效率,同时降低了硬件资源的浪费。在实际应用中,这意味着开发者可以在相同的硬件配置下处理更复杂的任务,或者在有限的资源下实现更高的性能。2.0的混合专家系统创新点与1.5版相比,KimiCh
- 无面试无offer? 你需要AI 求职co-pilot的帮助!
大家好啊,我写的开源免费求职AIco-pilot工具发布了v3.0.0,欢迎大家参与、使用!https://github.com/weicanie/prisma-ai一、项目介绍开源免费的求职co-pilot,自动化简历准备至offer到手的整个流程。优化您的项目、定制您的简历、为您匹配工作,并帮助您做好面试准备。二、核心价值prisma-ai旨在解决求职者在准备简历和寻找工作时最头疼的3个问题:
- 反光衣识别漏检率 30%?陌讯多尺度模型实测优化
在建筑工地、交通指挥等场景中,反光衣是保障作业人员安全的重要装备,对其进行精准识别是智能监控系统的核心功能之一。但传统视觉算法在实际应用中却屡屡碰壁:强光下反光衣易与背景混淆、远距离小目标漏检率高达30%、复杂场景下模型泛化能力不足[实测数据来源:某智慧工地项目2024年Q1日志]。这些问题直接导致安全监控系统预警滞后,给安全生产埋下隐患。一、技术解析:反光衣识别的核心难点与陌讯算法创新反光衣识别
- 【AI大模型学习路线】第三阶段之RAG与LangChain——第十六章(Advanced RAG[1])其他Query优化相关策略?
985小水博一枚呀
AI大模型学习路线人工智能学习langchain
【AI大模型学习路线】第三阶段之RAG与LangChain——第十六章(AdvancedRAG[1])其他Query优化相关策略?【AI大模型学习路线】第三阶段之RAG与LangChain——第十六章(AdvancedRAG[1])其他Query优化相关策略?文章目录【AI大模型学习路线】第三阶段之RAG与LangChain——第十六章(AdvancedRAG[1])其他Query优化相关策略?一
- 系统迁移从CentOS7.9到Rocky8.9
我有两台阿里云上的服务器是CentOS7.9,由于CentOS7已经停止支持,后续使用的话会有安全漏洞,所以需要尽快迁移,个人使用的话目前兼容性好的还是RockyLinux8,很多脚本改改就能用了。一、盘点系统和迁移应用查看当前系统发行版版本cat/etc/os-release盘点迁移清单服务器应用部署方式docker镜像来源v1wordpressdockerdockerhubv1zdirdock
- 【unity编辑器开发与拓展EditorGUILayoyt和GUILayoyt】
死也不注释
Unity编辑器开发与拓展笔记unity编辑器游戏引擎
EditorGUILayout与GUILayout的核心区别及使用场景详解一、对比表特性GUILayoutEditorGUILayout命名空间UnityEngineUnityEditor使用场景运行时UI+编辑器扩展仅限编辑器扩展控件风格基础游戏风格(无编辑器优化)原生Unity编辑器风格布局复杂度基础流式布局高级自动布局(带标签对齐/间距优化)序列化支持❌不支持✅直接支持SerializedP
- 【华为机试】HJ61 放苹果
不爱熬夜的Coder
算法华为机试golang华为golang算法面试
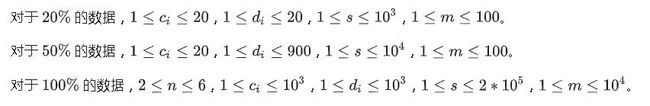
文章目录HJ61放苹果描述输入描述输出描述示例1示例2解题思路算法分析问题本质分析状态定义与转移递推关系详解动态规划表构建算法流程图示例推导过程代码实现思路时间复杂度分析关键优化点边界情况处理递归解法对比实际应用场景测试用例分析算法特点数学原理完整题解代码HJ61放苹果描述我们需要将m个相同的苹果放入n个相同的盘子中,允许有的盘子空着不放。求解有多少种不同的分法。输入描述输入两个整数m,n(0B[
- Python 爬虫实战:视频平台播放量实时监控(含反爬对抗与数据趋势预测)
西攻城狮北
python爬虫音视频
一、引言在数字内容蓬勃发展的当下,视频平台的播放量数据已成为内容创作者、营销人员以及行业分析师手中极为关键的情报资源。它不仅能够实时反映内容的受欢迎程度,更能在竞争分析、营销策略制定以及内容优化等方面发挥不可估量的作用。然而,视频平台为了保护自身数据和用户隐私,往往会设置一系列反爬虫机制,对数据爬取行为进行限制。这就向我们发起了挑战:如何巧妙地突破这些限制,同时精准地捕捉并预测播放量的动态变化趋势
- Java中的Tomcat,开启Web应用腾飞【基础版】
目录一、Tomcat初登场:揭开神秘面纱(一)啥是Tomcat(二)为啥要有Tomcat二、Tomcat的安装与启动:开启第一步(一)下载Tomcat(二)启动Tomcat三、Tomcat的目录结构:探秘内部布局(一)核心目录介绍(二)目录间的协同工作四、部署JavaWeb应用到Tomcat:让应用上线(一)打包Web应用为WAR文件(二)部署WAR文件到Tomcat五、Tomcat的配置优化:让
- 提升企业级数据处理效率!TDengine 四个集群优化点详解
TDengine (老段)
TDengine运维大数据数据库物联网时序数据库服务器运维tdengine
为了帮助企业更好地进行大数据处理,我们在此前TDengine3.x系列版本中进行了几项与集群相关的优化和新功能开发,以提升集群的稳定性和在异常情况下的恢复能力。这些优化包括clusterID隔离、leaderrebalance、raftlearner和restorednode。本文将对这几项重要优化进行详细阐述,以解答企业在此领域的疑问,并帮助大家更好地应对相关挑战。clusterID隔离问题fi
- c++ 编译链接时 报错找不到 某个函数,如何排查?
sun007700
c++chrome开发语言
在C++开发中,链接时出现“undefinedreferenceto”错误是常见问题,以下是系统化的排查流程和解决方案:1.确认基础问题(30秒检查)#检查函数声明是否存在grep"function_name"include/*.hsrc/*.cpp#检查是否包含实现文件ls-lsrc/#确认包含实现的.cpp文件在编译列表中2.签名匹配检查(最常见问题)//头文件声明-voidprocess_d
- ZooKeeper架构及应用场景详解
走过冬季
学习笔记zookeeper架构分布式
ZooKeeper是一个开源的分布式协调服务,由Apache软件基金会维护。它旨在为分布式应用提供高性能、高可用、强一致性的基础服务,解决分布式系统中常见的协调难题(如配置管理、命名服务、分布式锁、服务发现、领导者选举等)。核心软件架构ZooKeeper的架构设计围绕其核心目标(协调)而优化,主要包含以下关键组件:集群模式(Ensemble):ZooKeeper通常部署为集群(称为ensemble
- zookeeper etcd区别
sun007700
zookeeperetcd分布式
ZooKeeper与etcd的核心区别体现在设计理念、数据模型、一致性协议及适用场景等方面。ZooKeeper基于ZAB协议实现分布式协调,采用树形数据结构和临时节点特性,适合传统分布式系统;而etcd基于Raft协议,以高性能键值对存储为核心,专为云原生场景优化,是Kubernetes等容器编排系统的默认存储组件。12架构与设计目标差异ZooKeeper。设计定位:专注于分
- 赛亚超频:蚂蚁、阿瓦隆、神马矿工超频解除低温限制,高温保护
Punkhash算力租赁
超频虚拟货币矿机
www.punkhash.com赛亚超频在比特币挖矿行业日益激烈的今天,矿工们越来越重视矿机的效率与稳定性。随着电价的波动、币价的不确定以及矿机成本的攀升,单纯依靠“买新设备”提升产出,已经不再是最优选择。越来越多有经验的矿工开始转向对现有设备进行超频优化,以提高算力、降低单位能耗,从而获得更高的收益回报。而在众多第三方超频固件中,赛亚超频(SaiyanFirmware)凭借稳定性强、兼容机型广、
- 具身语义导航算法总揽
Shilong Wang
具身导航算法算法
端到端方法小脑大脑GNMNavDPNaVILAViNTNomadNavidStreamVLNMapNavNavGPTUni-NavidOctoNavNavGPT2模仿学习行为克隆BCDAgger模块化方法GOATVLFMSayPlanLM-NavETPNavVoroNavEmbodiedRAGVL-NavStairwaytoSuccess业内大佬北大王鹤NavidUni-NavidOctoNav吴
- 7. TCP 和 UDP 的区别
yqcoder
前端面试-服务协议网络网络协议http
总结TCP面向连接,需要三次握手建立连接,UDP无连接,不需要握手,直接发送数据。UDP有较好的实时性,效率比TCP高。TCP面向字节流,实际上是TCP把数据看成一连串无结构的字节流,UDP是面向报文的,一次交付一个完整的报文,报文不可分割,报文是UDP数据报处理的最小单位。每一条TCP连接时一对一的,UDP可以一对多,多对一,多对多。UDP分组首部开销小,八个字节,TCP首部开销大约20字节。U
- 《C++性能优化指南》 linux版代码及原理解读 第一章
v俊逸
C++性能优化指南性能优化C++性能优化性能优化
概述:目录概述:性能优化的必要性:C++代码优化策略总结用好的编译器并用好编译器使用更好的算法使用更好的库减少内存分配和复制移除计算使用更好的数据结构提高并发性优化内存管理性能优化的必要性:按照当今的CPU运行速度来说,执行一条指令所需要的时间是10的-9次方的时间单位,如此快速的执行速度是否就没有性能优化的必要了呢?其实不然,性能优化与CPU的执行速度并无非常大的关系,试想一下,一段代码,如果用
- 《C++性能优化指南》 linux版代码及原理解读 第四章
v俊逸
C++性能优化指南性能优化C++性能优化指南性能优化
目录概述为什么字符串很麻烦字符串是动态分配的字符串赋值背后的操作如何面对字符串会进行大量复制写时复制COW(copyonwrite)尝试优化字符串避免临时字符串通过预留存储空间减少内存分配通过传递引用减少实参复制使用迭代器操作减少循环中的比较操作减少返回值的复制还没有结束,使用字符数组代替字符串再次优化字符串尝试其他的算法叠加以前的优化方式使用其他的编译器使用其他字符串的库功能丰富的字符串库使用s
- 2.4 基于dpdk的用户态协议栈的实现
百亿苍狗
高性能网络设计专栏开发语言网络
操作系统PosixAPI所提供的网络接口,数据收发是基于用户态与内核态的频繁切换实现。而dpdk实现了绕过内核监管,直接在用户态访问网络硬件,避免频繁状态切换。DPDK安装与配置虚拟机环境配置检查是否支持多队列网卡cat/proc/interrupts|grepens33(获取整个机器的终端),结果19:4202120IO-APIC19-fasteoiens33,不支持多队列网卡。虚拟机关机,修改
- 法律科技领域人工智能代理构建的十个经验教训,一位人工智能工程师通过构建、部署和维护智能代理的经验教训来优化法律工作流程的历程。
知识大胖
NVIDIAGPU和大语言模型开发教程人工智能ai
目录介绍什么是代理人?为什么它对法律如此重要?法律技术中代理用例示例-合同审查代理-法律研究代理在LegalTech中使用代理的十个教训-教训1:即使代理很酷,它们也不能解决所有问题-教训2:选择最适合您用例的框架-教训3:能够快速迭代不同的模型-教训4:从简单开始,必要时扩展-教训5:使用跟踪解决方案;您将需要它-教训6:确保跟踪成本,代理循环可能很昂贵-教训7:将控制权交给最终用户(人在环路中
- java的(PO,VO,TO,BO,DAO,POJO)
Cb123456
VOTOBOPOJODAO
转:
http://www.cnblogs.com/yxnchinahlj/archive/2012/02/24/2366110.html
-------------------------------------------------------------------
O/R Mapping 是 Object Relational Mapping(对象关系映
- spring ioc原理(看完后大家可以自己写一个spring)
aijuans
spring
最近,买了本Spring入门书:spring In Action 。大致浏览了下感觉还不错。就是入门了点。Manning的书还是不错的,我虽然不像哪些只看Manning书的人那样专注于Manning,但怀着崇敬 的心情和激情通览了一遍。又一次接受了IOC 、DI、AOP等Spring核心概念。 先就IOC和DI谈一点我的看法。IO
- MyEclipse 2014中Customize Persperctive设置无效的解决方法
Kai_Ge
MyEclipse2014
高高兴兴下载个MyEclipse2014,发现工具条上多了个手机开发的按钮,心生不爽就想弄掉他!
结果发现Customize Persperctive失效!!
有说更新下就好了,可是国内Myeclipse访问不了,何谈更新...
so~这里提供了更新后的一下jar包,给大家使用!
1、将9个jar复制到myeclipse安装目录\plugins中
2、删除和这9个jar同包名但是版本号较
- SpringMvc上传
120153216
springMVC
@RequestMapping(value = WebUrlConstant.UPLOADFILE)
@ResponseBody
public Map<String, Object> uploadFile(HttpServletRequest request,HttpServletResponse httpresponse) {
try {
//
- Javascript----HTML DOM 事件
何必如此
JavaScripthtmlWeb
HTML DOM 事件允许Javascript在HTML文档元素中注册不同事件处理程序。
事件通常与函数结合使用,函数不会在事件发生前被执行!
注:DOM: 指明使用的 DOM 属性级别。
1.鼠标事件
属性
- 动态绑定和删除onclick事件
357029540
JavaScriptjquery
因为对JQUERY和JS的动态绑定事件的不熟悉,今天花了好久的时间才把动态绑定和删除onclick事件搞定!现在分享下我的过程。
在我的查询页面,我将我的onclick事件绑定到了tr标签上同时传入当前行(this值)参数,这样可以在点击行上的任意地方时可以选中checkbox,但是在我的某一列上也有一个onclick事件是用于下载附件的,当
- HttpClient|HttpClient请求详解
7454103
apache应用服务器网络协议网络应用Security
HttpClient 是 Apache Jakarta Common 下的子项目,可以用来提供高效的、最新的、功能丰富的支持 HTTP 协议的客户端编程工具包,并且它支持 HTTP 协议最新的版本和建议。本文首先介绍 HTTPClient,然后根据作者实际工作经验给出了一些常见问题的解决方法。HTTP 协议可能是现在 Internet 上使用得最多、最重要的协议了,越来越多的 Java 应用程序需
- 递归 逐层统计树形结构数据
darkranger
数据结构
将集合递归获取树形结构:
/**
*
* 递归获取数据
* @param alist:所有分类
* @param subjname:对应统计的项目名称
* @param pk:对应项目主键
* @param reportList: 最后统计的结果集
* @param count:项目级别
*/
public void getReportVO(Arr
- 访问WEB-INF下使用frameset标签页面出错的原因
aijuans
struts2
<frameset rows="61,*,24" cols="*" framespacing="0" frameborder="no" border="0">
- MAVEN常用命令
avords
Maven库:
http://repo2.maven.org/maven2/
Maven依赖查询:
http://mvnrepository.com/
Maven常用命令: 1. 创建Maven的普通java项目: mvn archetype:create -DgroupId=packageName
- PHP如果自带一个小型的web服务器就好了
houxinyou
apache应用服务器WebPHP脚本
最近单位用PHP做网站,感觉PHP挺好的,不过有一些地方不太习惯,比如,环境搭建。PHP本身就是一个网站后台脚本,但用PHP做程序时还要下载apache,配置起来也不太很方便,虽然有好多配置好的apache+php+mysq的环境,但用起来总是心里不太舒服,因为我要的只是一个开发环境,如果是真实的运行环境,下个apahe也无所谓,但只是一个开发环境,总有一种杀鸡用牛刀的感觉。如果php自己的程序中
- NoSQL数据库之Redis数据库管理(list类型)
bijian1013
redis数据库NoSQL
3.list类型及操作
List是一个链表结构,主要功能是push、pop、获取一个范围的所有值等等,操作key理解为链表的名字。Redis的list类型其实就是一个每个子元素都是string类型的双向链表。我们可以通过push、pop操作从链表的头部或者尾部添加删除元素,这样list既可以作为栈,又可以作为队列。
&nbs
- 谁在用Hadoop?
bingyingao
hadoop数据挖掘公司应用场景
Hadoop技术的应用已经十分广泛了,而我是最近才开始对它有所了解,它在大数据领域的出色表现也让我产生了兴趣。浏览了他的官网,其中有一个页面专门介绍目前世界上有哪些公司在用Hadoop,这些公司涵盖各行各业,不乏一些大公司如alibaba,ebay,amazon,google,facebook,adobe等,主要用于日志分析、数据挖掘、机器学习、构建索引、业务报表等场景,这更加激发了学习它的热情。
- 【Spark七十六】Spark计算结果存到MySQL
bit1129
mysql
package spark.examples.db
import java.sql.{PreparedStatement, Connection, DriverManager}
import com.mysql.jdbc.Driver
import org.apache.spark.{SparkContext, SparkConf}
object SparkMySQLInteg
- Scala: JVM上的函数编程
bookjovi
scalaerlanghaskell
说Scala是JVM上的函数编程一点也不为过,Scala把面向对象和函数型编程这两种主流编程范式结合了起来,对于熟悉各种编程范式的人而言Scala并没有带来太多革新的编程思想,scala主要的有点在于Java庞大的package优势,这样也就弥补了JVM平台上函数型编程的缺失,MS家.net上已经有了F#,JVM怎么能不跟上呢?
对本人而言
- jar打成exe
bro_feng
java jar exe
今天要把jar包打成exe,jsmooth和exe4j都用了。
遇见几个问题。记录一下。
两个软件都很好使,网上都有图片教程,都挺不错。
首先肯定是要用自己的jre的,不然不能通用,其次别忘了把需要的lib放到classPath中。
困扰我很久的一个问题是,我自己打包成功后,在一个同事的没有装jdk的电脑上运行,就是不行,报错jvm.dll为无效的windows映像,如截图
最后发现
- 读《研磨设计模式》-代码笔记-策略模式-Strategy
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
/*
策略模式定义了一系列的算法,并将每一个算法封装起来,而且使它们还可以相互替换。策略模式让算法独立于使用它的客户而独立变化
简单理解:
1、将不同的策略提炼出一个共同接口。这是容易的,因为不同的策略,只是算法不同,需要传递的参数
- cmd命令值cvfM命令
chenyu19891124
cmd
cmd命令还真是强大啊。今天发现jar -cvfM aa.rar @aaalist 就这行命令可以根据aaalist取出相应的文件
例如:
在d:\workspace\prpall\test.java 有这样一个文件,现在想要将这个文件打成一个包。运行如下命令即可比如在d:\wor
- OpenJWeb(1.8) Java Web应用快速开发平台
comsci
java框架Web项目管理企业应用
OpenJWeb(1.8) Java Web应用快速开发平台的作者是我们技术联盟的成员,他最近推出了新版本的快速应用开发平台 OpenJWeb(1.8),我帮他做做宣传
OpenJWeb快速开发平台以快速开发为核心,整合先进的java 开源框架,本着自主开发+应用集成相结合的原则,旨在为政府、企事业单位、软件公司等平台用户提供一个架构透
- Python 报错:IndentationError: unexpected indent
daizj
pythontab空格缩进
IndentationError: unexpected indent 是缩进的问题,也有可能是tab和空格混用啦
Python开发者有意让违反了缩进规则的程序不能通过编译,以此来强制程序员养成良好的编程习惯。并且在Python语言里,缩进而非花括号或者某种关键字,被用于表示语句块的开始和退出。增加缩进表示语句块的开
- HttpClient 超时设置
dongwei_6688
httpclient
HttpClient中的超时设置包含两个部分:
1. 建立连接超时,是指在httpclient客户端和服务器端建立连接过程中允许的最大等待时间
2. 读取数据超时,是指在建立连接后,等待读取服务器端的响应数据时允许的最大等待时间
在HttpClient 4.x中如下设置:
HttpClient httpclient = new DefaultHttpC
- 小鱼与波浪
dcj3sjt126com
一条小鱼游出水面看蓝天,偶然间遇到了波浪。 小鱼便与波浪在海面上游戏,随着波浪上下起伏、汹涌前进。 小鱼在波浪里兴奋得大叫:“你每天都过着这么刺激的生活吗?简直太棒了。” 波浪说:“岂只每天过这样的生活,几乎每一刻都这么刺激!还有更刺激的,要有潮汐变化,或者狂风暴雨,那才是兴奋得心脏都会跳出来。” 小鱼说:“真希望我也能变成一个波浪,每天随着风雨、潮汐流动,不知道有多么好!” 很快,小鱼
- Error Code: 1175 You are using safe update mode and you tried to update a table
dcj3sjt126com
mysql
快速高效用:SET SQL_SAFE_UPDATES = 0;下面的就不要看了!
今日用MySQL Workbench进行数据库的管理更新时,执行一个更新的语句碰到以下错误提示:
Error Code: 1175
You are using safe update mode and you tried to update a table without a WHERE that
- 枚举类型详细介绍及方法定义
gaomysion
enumjavaee
转发
http://developer.51cto.com/art/201107/275031.htm
枚举其实就是一种类型,跟int, char 这种差不多,就是定义变量时限制输入的,你只能够赋enum里面规定的值。建议大家可以看看,这两篇文章,《java枚举类型入门》和《C++的中的结构体和枚举》,供大家参考。
枚举类型是JDK5.0的新特征。Sun引进了一个全新的关键字enum
- Merge Sorted Array
hcx2013
array
Given two sorted integer arrays nums1 and nums2, merge nums2 into nums1 as one sorted array.
Note:You may assume that nums1 has enough space (size that is
- Expression Language 3.0新特性
jinnianshilongnian
el 3.0
Expression Language 3.0表达式语言规范最终版从2013-4-29发布到现在已经非常久的时间了;目前如Tomcat 8、Jetty 9、GlasshFish 4已经支持EL 3.0。新特性包括:如字符串拼接操作符、赋值、分号操作符、对象方法调用、Lambda表达式、静态字段/方法调用、构造器调用、Java8集合操作。目前Glassfish 4/Jetty实现最好,对大多数新特性
- 超越算法来看待个性化推荐
liyonghui160com
超越算法来看待个性化推荐
一提到个性化推荐,大家一般会想到协同过滤、文本相似等推荐算法,或是更高阶的模型推荐算法,百度的张栋说过,推荐40%取决于UI、30%取决于数据、20%取决于背景知识,虽然本人不是很认同这种比例,但推荐系统中,推荐算法起的作用起的作用是非常有限的。
就像任何
- 写给Javascript初学者的小小建议
pda158
JavaScript
一般初学JavaScript的时候最头痛的就是浏览器兼容问题。在Firefox下面好好的代码放到IE就不能显示了,又或者是在IE能正常显示的代码在firefox又报错了。 如果你正初学JavaScript并有着一样的处境的话建议你:初学JavaScript的时候无视DOM和BOM的兼容性,将更多的时间花在 了解语言本身(ECMAScript)。只在特定浏览器编写代码(Chrome/Fi
- Java 枚举
ShihLei
javaenum枚举
注:文章内容大量借鉴使用网上的资料,可惜没有记录参考地址,只能再传对作者说声抱歉并表示感谢!
一 基础 1)语法
枚举类型只能有私有构造器(这样做可以保证客户代码没有办法新建一个enum的实例)
枚举实例必须最先定义
2)特性
&nb
- Java SE 6 HotSpot虚拟机的垃圾回收机制
uuhorse
javaHotSpotGC垃圾回收VM
官方资料,关于Java SE 6 HotSpot虚拟机的garbage Collection,非常全,英文。
http://www.oracle.com/technetwork/java/javase/gc-tuning-6-140523.html
Java SE 6 HotSpot[tm] Virtual Machine Garbage Collection Tuning
&