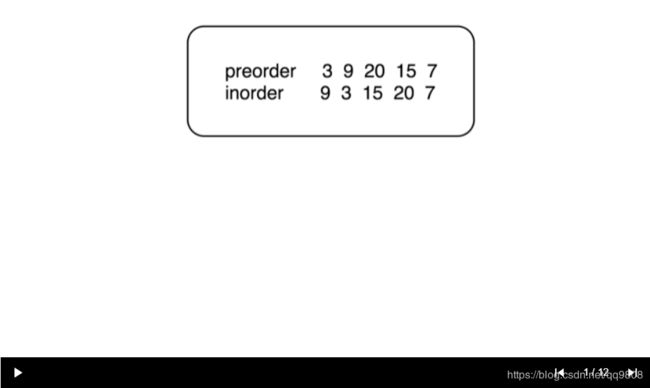
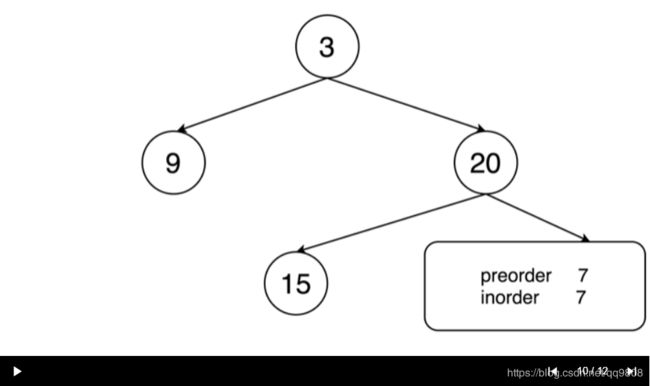
- day11 学习笔记
豆豆
学习笔记python
文章目录前言一、类方法二、静态方法三、构造方法四、魔术方法前言通过今天的学习,我掌握了更多Python中有关面向对象编程思想中方法的概念与操作,包括类方法,静态方法,构造方法,魔术方法一、类方法类方法是属于类的行为,一般使用类而非对象进行调用类方法需要使用@classmethod装饰器定义类方法至少有一个形参用于绑定类,约定为cls类和该类的实例都可以调用类方法,但一般不用实例进行调用类方法不能访
- k8s中PAUSE容器与init容器比较 local卷与hostpath卷比较
小刘爱喇石( ˝ᗢ̈˝ )
kubernetes容器云原生
目录一、PAUSE容器与INIT容器比较1.Pause容器作用特点示例2.Init容器作用特点示例3.Pause容器vsInit容器4.总结这两个哪个先启动呢?详细启动顺序为什么Pause容器最先启动?示例总结二、local卷与hostpath卷1.local卷定义特点配置示例2.hostPath卷定义特点配置示例3.local卷vshostPath卷4.选择建议一、PAUSE容器与INIT容器比
- RabbitMQ 与 Kafka:消息中间件的终极对比与选型指南
海上彼尚
node.jsrabbitmqkafka分布式node.js
引言在分布式系统架构中,消息中间件是异步通信的核心组件。RabbitMQ和Kafka作为两大主流技术,常被开发者拿来比较。本文深入解析两者的设计哲学、性能差异和典型场景,助你做出精准技术选型。目录引言一、核心设计差异1.定位与数据模型二、性能与架构对比1.吞吐量与延迟2.集群与扩展三、功能特性对决1.消息可靠性2.消息路由四、典型场景与选型决策1.优先选择Kafka的场景2.优先选择RabbitM
- 手机号码归属地API:助力数字化服务的利器
api
前言在当今数字化时代,手机号码已成为人们日常生活中不可或缺的一部分,而手机号码归属地API作为一种高效、便捷的网络服务接口,正逐渐成为众多行业提升用户体验和服务质量的重要工具。什么是手机号码归属地API?手机号码归属地API是一种允许开发者通过编程方式查询手机号码注册地信息的网络服务接口。它通常由第三方服务提供商通过HTTP请求的方式提供。通过这种API,开发者可以快速获取手机号码的归属地信息,包
- 外贸英语报价单制作步骤分享,可在线编辑
财务管理系统财务软件进销存系统
在国际贸易中,一份专业、清晰的外贸英语报价单是赢得客户信任的关键工具。它不仅需要准确传递产品信息与价格条款,还需符合国际商务规范。本文将深入解析外贸英语报价单的核心要素,并介绍如何通过ZohoBooks快速制作标准化模板,提升业务效率与竞争力。一、外贸英语报价单模板的核心要素一份完整的报价单需涵盖以下关键内容:1、基础信息标题与编号:明确标注“Quotation”或“ProformaInvoice
- 手机电脑如何通过跨平台远程控制工具来实现无缝互联
2501_90729959
RayLink远程控制工具远程控制软件智能手机电脑
在如今数字化办公和生活的场景里,远程控制工具已经成了连接各种设备的关键桥梁。不管是跨系统协作、远程技术支持,还是让移动端和电脑端高效联动,用户对这些工具的要求早就从“能用就行”变成了“得用得顺手”。接下来,我就从跨平台兼容性、画质性能、安全性和操作体验这四个主要方面,来聊聊现在主流的远程控制工具都有啥特点。重点说说RayLink远程控制软件,看看它是怎么通过技术创新,让手机和电脑实现无缝互联的。一
- 嵌入式硬件篇---WIFI模块
Ronin-Lotus
程序代码篇嵌入式硬件篇嵌入式硬件cWIFI
文章目录前言一、核心工作原理1.物理层(PHY)工作频段2.4GHz5GHz调制技术直接序列扩频正交频分复用高效数据编码2.协议栈架构MAC层Beacon帧4次握手3.核心工作模式二、典型应用场景1.智能家居系统远程控制环境监测视频监测2.工业物联网设备远程运维生产线监控仓储管理3.医疗设备远程诊疗医疗影像药品管理4.消费电子智能音箱游戏设备打印设备三、ESP32开发示例1.环境配置(Platfo
- 【C++】C++从入门到精通教程(持续更新...)
废人一枚
C++c++开发语言
前言最近在整理之前一些C++资料,重新整理出了一套C++从基础到实践的教程,包含概念、代码、运行结果以及知识点的扩展,感兴趣的后续大家持续关注。以下是更新的文章目录,文章之后整理了一个知识思维导图,看起来比较清楚点。目录1、C++基础知识C++基础知识一个简单的C++程序函数重载引用的概念引用与指针的区别引用作为函数参数引用作为返回值面向对象类的定义类的声明结构体与类的区别inline函数this
- day15 容器有好多东西需要记住的
想成为大佬的每一天
c++开发语言
Vectorvector数据结构和数组非常相似,也称为单端数组,与数组不同在于数组是静态空间,而vector可以动态扩展,动态扩展不是在原有空间之后续接空间,而是找更大的内存空间,将原数据拷贝到新空间,释放原空间。构造方式//vector构造方式vectorv1;//默认,无参构造vectorv2(v1.begin(),v1.end());//通过区间的方式进行构造vectorv3(5,20);/
- “四预”驱动数字孪生水利:让智慧治水守护山河安澜
GeoSaaS
实景三维智慧城市人工智能gis大数据安全
近年来,从黄河秋汛到海河特大洪水,从珠江流域性洪灾到长江罕见骤旱,极端天气频发让水安全问题备受关注。如何实现“治水于未发”?数字孪生水利以“预报、预警、预演、预案”(四预)为核心,正在掀起一场水利治理的智慧革命。一、数字孪生水利:从物理世界到虚拟镜像的跃迁数字孪生水利并非简单的“数字建模”,而是通过高精度传感器、大数据、人工智能等技术,在虚拟空间构建与物理流域完全映射的“数字分身”,实现水情、工情
- 低空航路:低空经济的基础设施
GeoSaaS
低空经济自动驾驶汽车信息可视化人工智能大数据
低空经济作为新兴产业,正逐渐成为推动城市高质量发展的新引擎。低空航路的构建是实现低空经济发展的关键,它涉及到无人机、电动垂直起降飞行器(eVTOL)等航空器的运行,对城市物流、旅游、农业、应急救援等领域产生深远影响。低空航路的内涵低空航路指的是在城市低空空域中为无人机和eVTOL等航空器规划的飞行路径。这些航路需要满足安全、高效、有序的飞行需求,同时还需考虑与城市建筑、人口密集区等的协调。构建低空
- 没有好的学历,Java开发未来的路应该怎么走?
全干程序员demo
技术热文java开发语言
没有好的学历,Java开发未来的路应该怎么走?在当今数字化时代,技术发展日新月异,大模型应用、鸿蒙系统等新兴技术领域正在蓬勃发展,为Java开发者带来了新的机遇和挑战。即使没有高学历,Java开发者依然可以通过以下路径在这些新兴领域找到自己的发展方向,实现职业突破。一、拥抱新兴技术,拓宽技术边界(一)大模型应用:从开发到优化大模型技术正在重塑软件开发的各个环节。对于Java开发者来说,可以从以下几
- TCP/IP协议——ARP
胡小冰
TCP/IP网络
ARP只要确定了IP地址,就可以向这个目标地址发送IP数据报。然而,在底层数据链路层,进行实际通信时确有必要了解每个IP地址所对应的MAC地址。一、ARP概要ARP是一种解决地址问题的协议。以目标IP地址为线索,用来定位下一个应该接受数据分包设备对应的MAC地址。如果目标主机不在同一个链路上时,可以通过ARP查找下一跳路由器的MAC地址。不过ARP只适用于IPv4,不能用于IPv6。IPv6中可以
- DeepSeek带来服务器与显卡需求激增的核心逻辑
DeepSeek+NAS
人工智能服务器运维网络安全计算机网络
随着DeepSeek等开源AI模型的普及,个人开发者和小型企业正加速构建私有化AI服务器,以处理敏感数据和定制化任务。这种趋势不仅重构了算力需求的结构,更推动服务器和显卡市场进入新一轮增长周期。以下从技术迭代、行业需求、市场格局三个维度展开论述。一、私有化部署:从数据安全到算力自主的核心驱动力数据隐私与合规性需求公共AI平台的数据泄露风险促使企业选择本地化部署。例如,医疗机构的患者数据、金融企业的
- Win NAS 的数据传输原理分析,超级详细!
DeepSeek+NAS
家用NASWinNAS飞牛NAS人工智能安卓NAS
WinNAS是一款运行在Windows系统上的NAS服务,允许用户通过手机客户端远程访问和管理电脑上的文件。为了实现这一功能,WinNAS与手机之间的数据传输过程涉及多个步骤,具体取决于手机和WinNAS电脑所处的网络环境。以下是整个数据传输过程的详细说明:1.权限认证与连接建立无论手机和WinNAS电脑是否在同一个局域网内,手机客户端在访问WinNAS之前,都需要先通过耘想公司的云服务器进行权限
- APP怎么抓取原生日志 - Android篇
大汉堡玩测试
android功能测试
文章目录前言为什么要抓原生页面的日志举一个抓取原生日志的例子AndroidDebugBridge(ADB)安装ADB连接设备验证连接抓取日志注意点总结前言好困~写点我觉得重要的吧,IOS和HarmonyOSNEXT这周写为什么要抓原生页面的日志原生日志能够捕捉到与操作系统和应用框架交互的关键信息,包括性能瓶颈、崩溃报告和安全事件等,而这些是纯H5日志无法提供的,确保了对应用行为的全面监控和精准调试
- Python 正则表达式小结1
大收藏家
Python正则表达式python
[声明]:本文参考了白夜黑雨老师的网页讲解。如有侵权,请与我联系!!!Python正则表达式小结11.正则表达式验证2.特殊元字符及含义3匹配某种字符类型4.正则表达式举例大收藏家说1.正则表达式验证提供两个网站用于正则表达式的验证,可以敲入文本与正则表达式。通过该网站,验证正则表达式的正确性。非常好用!英文网站中文网站2.特殊元字符及含义元字符含义.表示要匹配除了换行符之外的任何单个字符*星号-
- vr中的计算机知识,VR技术基本常识
淡庸
vr中的计算机知识
虚拟现实技术是仿真技术的一个重要方向是仿真技术与计算机图形学人机接口技术多媒体技术传感技术网络技术等多种技术的集合是一门富有挑战性的交叉技术前沿学科和研究领域。虚拟现实技术(VR)主要包括模拟环境、感知、自然技能和传感设备等方面。模拟环境是由计算机生成的、实时动态的三维立体逼真图像。感知是指理想的VR应该具有一切人所具有的感知。除计算机图形技术所生成的视觉感知外,还有听觉、触觉、力觉、运动等感知,
- RIP路由欺骗攻击与防御实验详解
w2361734601
智能路由器网络
一、基础网络配置1.路由器R1配置interfaceGigabitEthernet0/0/0ipaddress192.1.2.254255.255.255.0!interfaceGigabitEthernet0/0/1ipaddress192.1.3.254255.255.255.0!routerrip1version2network192.1.2.0network192.1.3.02.路由器R2
- 常见FUZZ姿势与工具实战:从未知目录到备份文件漏洞挖掘
w2361734601
web安全安全
本文仅供学习交流使用,严禁用于非法用途。未经授权,禁止对任何网站或系统进行未授权的测试或攻击。因使用本文所述技术造成的任何后果,由使用者自行承担。请严格遵守《网络安全法》及相关法律法规!目录本文仅供学习交流使用,严禁用于非法用途。未经授权,禁止对任何网站或系统进行未授权的测试或攻击。因使用本文所述技术造成的任何后果,由使用者自行承担。请严格遵守《网络安全法》及相关法律法规!一、FUZZ技术概述二、
- Webpack 中动态导入(Dynamic Import)的几种典型用法
混血哲谈
webpack前端node.js
这段代码展示了Webpack中动态导入(DynamicImport)的几种典型用法,并通过Webpack魔法注释(MagicComments)对打包行为进行精细控制。以下是逐段解析:1.单一目标模块的动态导入import(/*webpackChunkName:"my-chunk-name"*///指定生成的chunk名称/*webpackMode:"lazy"*///延迟加载模式/*webpack
- 如何使用webpack预加载 CSS 中定义的资源和预加载 CSS 文件
混血哲谈
webpackcss前端
在Webpack中预加载CSS文件及其内部定义的资源(如图片、字体等),可以通过资源预加载(Preloading)技术优化关键资源的加载优先级。以下是具体的实现方法和步骤:一、预加载CSS文件1.使用@vue/preload-webpack-plugin或手动注入Webpack默认不直接支持CSS文件的预加载,但可以通过插件或手动添加实现。方法一:通过@vue/preload-webpack-pl
- 基于AT89C52单片机的智能导盲杖报警设计
七月小卖铺
单片机单片机嵌入式硬件
点击链接获取Keil源码与ProjectBackups仿真图:https://download.csdn.net/download/qq_64505944/90498287?spm=1001.2014.3001.5503C+22部分参考设计如下:摘要超声波测距技术因其具有较强的指向性、低能耗、较长的传播距离等优点,已成为广泛应用于各类传感器技术和自动控制技术相结合的测距方案之一。超声波传感器利用声
- 【DeepSeek】 全方位使用指南————简版
諰.
人工智能aiAI写作
一、平台概述DeepSeek(深度求索)是专注实现AGI的中国的人工智能公司,提供多款AI产品:智能对话(Chat)文生图(Art)代码助手(Coder)API开发接口企业定制解决方案二、注册与登录2.1账号创建访问官网https://www.deepseek.com点击右上角「注册」支持三种方式:手机号+短信验证邮箱注册(需验证邮件)第三方登录(微信/Google账号)2.2订阅计划套餐类型免费
- tracert命令输出详解
learning-striving
eNSP智能路由器网络计算机网络
一、tracert命令输出C:\Users\xsq>tracertwww.xqnav.top通过最多30个跃点跟踪到www.xqnav.top[121.43.162.66]的路由:11ms2ms3ms10.16.0.121ms2ms1ms10.1.1.234ms3ms3ms49.9.17.58.adsl-pool.jx.chinaunicom.com[58.17.9.49]42ms2ms3ms21
- CVPR 2024 | 低分辨率引领方向:通过自监督学习提升超分辨率的泛化能力
小白学视觉
计算机顶会顶刊论文解读计算机视觉深度学习CVPR计算机顶会论文解读
论文信息题目:Low-ResLeadstheWay:ImprovingGeneralizationforSuper-ResolutionbySelf-SupervisedLearning低分辨率引领方向:通过自监督学习提升超分辨率的泛化能力作者:HaoyuChen,WenboLi,JinjinGu,JingjingRen,HaozeSun,XueyiZou,ZhensongZhang,Youlia
- OWL - 优化劳动力学习的通用智能体
小众AI
AI开源学习人工智能AI编程
GitHub:https://github.com/camel-ai/owl更多AI开源软件:发现分享好用的AI工具、AI开源软件、AI模型、AI变现-小众AIOWL是一个前沿的多智能体协作框架,推动任务自动化的边界,构建在CAMEL-AIFramework。愿景是彻底变革AI智能体协作解决现实任务的方式。通过利用动态智能体交互,OWL实现了跨多领域更自然、高效且稳健的任务自动化。OWL在GAIA
- 共筑智慧城市新生态!YashanDB与荣科科技完成兼容互认证
科技圈快讯
oracle数据库
近日,深圳计算科学研究院的崖山数据库系统YashanDB与荣科科技股份有限公司的智慧工程移动管理平台V1.0与不动产智能管理系统V1.0成功完成了兼容性互认证,标志着双方在智慧城市建设领域实现关键突破,以国产化高性能数据库技术为核心,为工程建设与不动产管理领域提供安全可控、高效稳定的数字化解决方案。在兼容性互认证过程中,双方针对功能、性能、兼容性以及稳定性等关键指标进行了多轮严格测试,验证了Ya
- 【Linux】Linux下调试器gdb的使用
安 度 因
Linuxlinux运维服务器测试工具调试
作者主页:@安度因学习社区:StackFrame专栏链接:Linux文章目录一、前言二、铺垫三、指令集和使用1、指令集2、演示四、结语如果无聊的话,就来逛逛我的博客栈吧!一、前言前几篇Linux博客中,我们分别学习了与编辑、编译、自动化构建代码、上传代码的工具。而今天,我们将学习最后一个工具——Linux下的调试器gdb
- YOLOv12优化:图像去噪 | AAAI2025 Transformer |一种基于Transformer的盲点网络(TBSN)架构 ,结合空间和通道自注意力层来增强网络能力
AI小怪兽
YOLOv12魔术师YOLOtransformer深度学习人工智能python
提出了一种基于Transformer的盲点网络(TBSN)架构,通过分析和重新设计Transformer运算符以满足盲点要求。TBSN遵循扩张BSN的架构原则,并结合空间和通道自注意力层来增强网络能力。如何使用:1)结合C3k2二次创新使用;2)结合A2C2f二次创新使用;亮点包括:1.提出了一种新的基于Transformer的盲点网络(TBSN)架构;2.引入了知识蒸馏策略来提高计算效率;3.在
- scala的option和some
矮蛋蛋
编程scala
原文地址:
http://blog.sina.com.cn/s/blog_68af3f090100qkt8.html
对于学习 Scala 的 Java™ 开发人员来说,对象是一个比较自然、简单的入口点。在 本系列 前几期文章中,我介绍了 Scala 中一些面向对象的编程方法,这些方法实际上与 Java 编程的区别不是很大。我还向您展示了 Scala 如何重新应用传统的面向对象概念,找到其缺点
- NullPointerException
Cb123456
androidBaseAdapter
java.lang.NullPointerException: Attempt to invoke virtual method 'int android.view.View.getImportantForAccessibility()' on a null object reference
出现以上异常.然后就在baidu上
- PHP使用文件和目录
天子之骄
php文件和目录读取和写入php验证文件php锁定文件
PHP使用文件和目录
1.使用include()包含文件
(1):使用include()从一个被包含文档返回一个值
(2):在控制结构中使用include()
include_once()函数需要一个包含文件的路径,此外,第一次调用它的情况和include()一样,如果在脚本执行中再次对同一个文件调用,那么这个文件不会再次包含。
在php.ini文件中设置
- SQL SELECT DISTINCT 语句
何必如此
sql
SELECT DISTINCT 语句用于返回唯一不同的值。
SQL SELECT DISTINCT 语句
在表中,一个列可能会包含多个重复值,有时您也许希望仅仅列出不同(distinct)的值。
DISTINCT 关键词用于返回唯一不同的值。
SQL SELECT DISTINCT 语法
SELECT DISTINCT column_name,column_name
F
- java冒泡排序
3213213333332132
java冒泡排序
package com.algorithm;
/**
* @Description 冒泡
* @author FuJianyong
* 2015-1-22上午09:58:39
*/
public class MaoPao {
public static void main(String[] args) {
int[] mao = {17,50,26,18,9,10
- struts2.18 +json,struts2-json-plugin-2.1.8.1.jar配置及问题!
7454103
DAOspringAjaxjsonqq
struts2.18 出来有段时间了! (貌似是 稳定版)
闲时研究下下! 貌似 sruts2 搭配 json 做 ajax 很吃香!
实践了下下! 不当之处请绕过! 呵呵
网上一大堆 struts2+json 不过大多的json 插件 都是 jsonplugin.34.jar
strut
- struts2 数据标签说明
darkranger
jspbeanstrutsservletScheme
数据标签主要用于提供各种数据访问相关的功能,包括显示一个Action里的属性,以及生成国际化输出等功能
数据标签主要包括:
action :该标签用于在JSP页面中直接调用一个Action,通过指定executeResult参数,还可将该Action的处理结果包含到本页面来。
bean :该标签用于创建一个javabean实例。如果指定了id属性,则可以将创建的javabean实例放入Sta
- 链表.简单的链表节点构建
aijuans
编程技巧
/*编程环境WIN-TC*/ #include "stdio.h" #include "conio.h"
#define NODE(name, key_word, help) \ Node name[1]={{NULL, NULL, NULL, key_word, help}}
typedef struct node { &nbs
- tomcat下jndi的三种配置方式
avords
tomcat
jndi(Java Naming and Directory Interface,Java命名和目录接口)是一组在Java应用中访问命名和目录服务的API。命名服务将名称和对象联系起来,使得我们可以用名称
访问对象。目录服务是一种命名服务,在这种服务里,对象不但有名称,还有属性。
tomcat配置
- 关于敏捷的一些想法
houxinyou
敏捷
从网上看到这样一句话:“敏捷开发的最重要目标就是:满足用户多变的需求,说白了就是最大程度的让客户满意。”
感觉表达的不太清楚。
感觉容易被人误解的地方主要在“用户多变的需求”上。
第一种多变,实际上就是没有从根本上了解了用户的需求。用户的需求实际是稳定的,只是比较多,也比较混乱,用户一般只能了解自己的那一小部分,所以没有用户能清楚的表达出整体需求。而由于各种条件的,用户表达自己那一部分时也有
- 富养还是穷养,决定孩子的一生
bijian1013
教育人生
是什么决定孩子未来物质能否丰盛?为什么说寒门很难出贵子,三代才能出贵族?真的是父母必须有钱,才能大概率保证孩子未来富有吗?-----作者:@李雪爱与自由
事实并非由物质决定,而是由心灵决定。一朋友富有而且修养气质很好,兄弟姐妹也都如此。她的童年时代,物质上大家都很贫乏,但妈妈总是保持生活中的美感,时不时给孩子们带回一些美好小玩意,从来不对孩子传递生活艰辛、金钱来之不易、要懂得珍惜
- oracle 日期时间格式转化
征客丶
oracle
oracle 系统时间有 SYSDATE 与 SYSTIMESTAMP;
SYSDATE:不支持毫秒,取的是系统时间;
SYSTIMESTAMP:支持毫秒,日期,时间是给时区转换的,秒和毫秒是取的系统的。
日期转字符窜:
一、不取毫秒:
TO_CHAR(SYSDATE, 'YYYY-MM-DD HH24:MI:SS')
简要说明,
YYYY 年
MM 月
- 【Scala六】分析Spark源代码总结的Scala语法四
bit1129
scala
1. apply语法
FileShuffleBlockManager中定义的类ShuffleFileGroup,定义:
private class ShuffleFileGroup(val shuffleId: Int, val fileId: Int, val files: Array[File]) {
...
def apply(bucketId
- Erlang中有意思的bug
bookjovi
erlang
代码中常有一些很搞笑的bug,如下面的一行代码被调用两次(Erlang beam)
commit f667e4a47b07b07ed035073b94d699ff5fe0ba9b
Author: Jovi Zhang <
[email protected]>
Date: Fri Dec 2 16:19:22 2011 +0100
erts:
- 移位打印10进制数转16进制-2008-08-18
ljy325
java基础
/**
* Description 移位打印10进制的16进制形式
* Creation Date 15-08-2008 9:00
* @author 卢俊宇
* @version 1.0
*
*/
public class PrintHex {
// 备选字符
static final char di
- 读《研磨设计模式》-代码笔记-组合模式
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
import java.util.ArrayList;
import java.util.List;
abstract class Component {
public abstract void printStruct(Str
- 利用cmd命令将.class文件打包成jar
chenyu19891124
cmdjar
cmd命令打jar是如下实现:
在运行里输入cmd,利用cmd命令进入到本地的工作盘符。(如我的是D盘下的文件有此路径 D:\workspace\prpall\WEB-INF\classes)
现在是想把D:\workspace\prpall\WEB-INF\classes路径下所有的文件打包成prpall.jar。然后继续如下操作:
cd D: 回车
cd workspace/prpal
- [原创]JWFD v0.96 工作流系统二次开发包 for Eclipse 简要说明
comsci
eclipse设计模式算法工作swing
JWFD v0.96 工作流系统二次开发包 for Eclipse 简要说明
&nb
- SecureCRT右键粘贴的设置
daizj
secureCRT右键粘贴
一般都习惯鼠标右键自动粘贴的功能,对于SecureCRT6.7.5 ,这个功能也已经是默认配置了。
老版本的SecureCRT其实也有这个功能,只是不是默认设置,很多人不知道罢了。
菜单:
Options->Global Options ...->Terminal
右边有个Mouse的选项块。
Copy on Select
Paste on Right/Middle
- Linux 软链接和硬链接
dongwei_6688
linux
1.Linux链接概念Linux链接分两种,一种被称为硬链接(Hard Link),另一种被称为符号链接(Symbolic Link)。默认情况下,ln命令产生硬链接。
【硬连接】硬连接指通过索引节点来进行连接。在Linux的文件系统中,保存在磁盘分区中的文件不管是什么类型都给它分配一个编号,称为索引节点号(Inode Index)。在Linux中,多个文件名指向同一索引节点是存在的。一般这种连
- DIV底部自适应
dcj3sjt126com
JavaScript
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/1999/xhtml&q
- Centos6.5使用yum安装mysql——快速上手必备
dcj3sjt126com
mysql
第1步、yum安装mysql
[root@stonex ~]# yum -y install mysql-server
安装结果:
Installed:
mysql-server.x86_64 0:5.1.73-3.el6_5 &nb
- 如何调试JDK源码
frank1234
jdk
相信各位小伙伴们跟我一样,想通过JDK源码来学习Java,比如collections包,java.util.concurrent包。
可惜的是sun提供的jdk并不能查看运行中的局部变量,需要重新编译一下rt.jar。
下面是编译jdk的具体步骤:
1.把C:\java\jdk1.6.0_26\sr
- Maximal Rectangle
hcx2013
max
Given a 2D binary matrix filled with 0's and 1's, find the largest rectangle containing all ones and return its area.
public class Solution {
public int maximalRectangle(char[][] matrix)
- Spring MVC测试框架详解——服务端测试
jinnianshilongnian
spring mvc test
随着RESTful Web Service的流行,测试对外的Service是否满足期望也变的必要的。从Spring 3.2开始Spring了Spring Web测试框架,如果版本低于3.2,请使用spring-test-mvc项目(合并到spring3.2中了)。
Spring MVC测试框架提供了对服务器端和客户端(基于RestTemplate的客户端)提供了支持。
&nbs
- Linux64位操作系统(CentOS6.6)上如何编译hadoop2.4.0
liyong0802
hadoop
一、准备编译软件
1.在官网下载jdk1.7、maven3.2.1、ant1.9.4,解压设置好环境变量就可以用。
环境变量设置如下:
(1)执行vim /etc/profile
(2)在文件尾部加入:
export JAVA_HOME=/home/spark/jdk1.7
export MAVEN_HOME=/ho
- StatusBar 字体白色
pangyulei
status
[[UIApplication sharedApplication] setStatusBarStyle:UIStatusBarStyleLightContent];
/*you'll also need to set UIViewControllerBasedStatusBarAppearance to NO in the plist file if you use this method
- 如何分析Java虚拟机死锁
sesame
javathreadoracle虚拟机jdbc
英文资料:
Thread Dump and Concurrency Locks
Thread dumps are very useful for diagnosing synchronization related problems such as deadlocks on object monitors. Ctrl-\ on Solaris/Linux or Ctrl-B
- 位运算简介及实用技巧(一):基础篇
tw_wangzhengquan
位运算
http://www.matrix67.com/blog/archives/263
去年年底写的关于位运算的日志是这个Blog里少数大受欢迎的文章之一,很多人都希望我能不断完善那篇文章。后来我看到了不少其它的资料,学习到了更多关于位运算的知识,有了重新整理位运算技巧的想法。从今天起我就开始写这一系列位运算讲解文章,与其说是原来那篇文章的follow-up,不如说是一个r
- jsearch的索引文件结构
yangshangchuan
搜索引擎jsearch全文检索信息检索word分词
jsearch是一个高性能的全文检索工具包,基于倒排索引,基于java8,类似于lucene,但更轻量级。
jsearch的索引文件结构定义如下:
1、一个词的索引由=分割的三部分组成: 第一部分是词 第二部分是这个词在多少