poj 1195(二维线段树||二维树状数组)
Mobile phones
| Time Limit: 5000MS | Memory Limit: 65536K | |
| Total Submissions: 17496 | Accepted: 8089 |
Description
Suppose that the fourth generation mobile phone base stations in the Tampere area operate as follows. The area is divided into squares. The squares form an S * S matrix with the rows and columns numbered from 0 to S-1. Each square contains a base station. The number of active mobile phones inside a square can change because a phone is moved from a square to another or a phone is switched on or off. At times, each base station reports the change in the number of active phones to the main base station along with the row and the column of the matrix.
Write a program, which receives these reports and answers queries about the current total number of active mobile phones in any rectangle-shaped area.
Write a program, which receives these reports and answers queries about the current total number of active mobile phones in any rectangle-shaped area.
Input
The input is read from standard input as integers and the answers to the queries are written to standard output as integers. The input is encoded as follows. Each input comes on a separate line, and consists of one instruction integer and a number of parameter integers according to the following table.
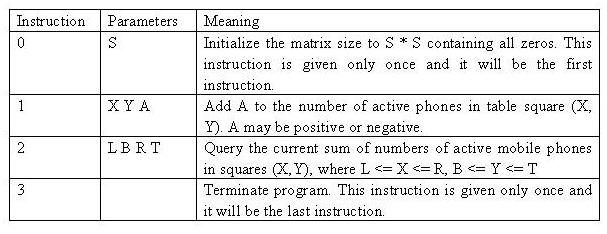
The values will always be in range, so there is no need to check them. In particular, if A is negative, it can be assumed that it will not reduce the square value below zero. The indexing starts at 0, e.g. for a table of size 4 * 4, we have 0 <= X <= 3 and 0 <= Y <= 3.
Table size: 1 * 1 <= S * S <= 1024 * 1024
Cell value V at any time: 0 <= V <= 32767
Update amount: -32768 <= A <= 32767
No of instructions in input: 3 <= U <= 60002
Maximum number of phones in the whole table: M= 2^30
The values will always be in range, so there is no need to check them. In particular, if A is negative, it can be assumed that it will not reduce the square value below zero. The indexing starts at 0, e.g. for a table of size 4 * 4, we have 0 <= X <= 3 and 0 <= Y <= 3.
Table size: 1 * 1 <= S * S <= 1024 * 1024
Cell value V at any time: 0 <= V <= 32767
Update amount: -32768 <= A <= 32767
No of instructions in input: 3 <= U <= 60002
Maximum number of phones in the whole table: M= 2^30
Output
Your program should not answer anything to lines with an instruction other than 2. If the instruction is 2, then your program is expected to answer the query by writing the answer as a single line containing a single integer to standard output.
Sample Input
0 4 1 1 2 3 2 0 0 2 2 1 1 1 2 1 1 2 -1 2 1 1 2 3 3
Sample Output
3 4
Source
IOI 2001
题意:
一个由数字构成的大矩阵,开始是全0,能进行两种操作
1) 对矩阵里的某个数加上一个整数(可正可负)
2) 查询某个子矩阵里所有数字的和
要求对每次查询,输出结果
题解:模板题,使用二维线段树或者二维树状数组数组
以下给出2种做法:
1:二维树状数组
#include
#include
#include
#include
#include
#include
#include
#include 2:二维线段树
#include
#include
#include
#include
#include
#include
#include
#include