- 打卡信奥刷题(1697)用C++实现信奥 P8244 [COCI 2013/2014 #3] KOLINJE
Loge编程生活
C++c++算法开发语言青少年编程数据结构
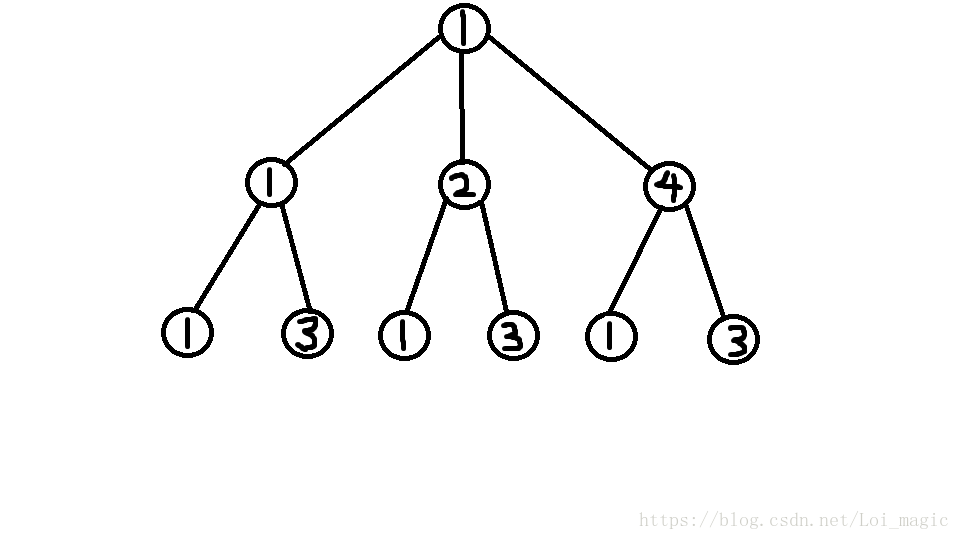
P8244[COCI2013/2014#3]KOLINJE题目描述在一年一度的生猪屠宰会上,屠夫Bajs正在进行活动的最后一个环节——将自己那条屡获殊荣的火腿拿出一部分,分给参加活动的所有人。今年的生猪屠宰会一共有nnn个人参加,其中第iii个人目前已经吃了aia_iai千克火腿。Bajs将自己的火腿按照b1:b2:⋯:bnb_1:b_2:\cdots:b_nb1:b2:⋯:bn的比例分给所有人,
- 打卡信奥刷题(1150)用C++实现信奥 P2085 最小函数值
P2085最小函数值题目描述有nnn个函数,分别为F1,F2,…,FnF_1,F_2,\dots,F_nF1,F2,…,Fn。定义Fi(x)=Aix2+Bix+Ci(x∈N∗)F_i(x)=A_ix^2+B_ix+C_i(x\in\mathbbN*)Fi(x)=Aix2+Bix+Ci(x∈N∗)。给定这些AiA_iAi、BiB_iBi和CiC_iCi,请求出所有函数的所有函数值中最小的mmm个(如
- 算法竞赛备考冲刺必刷题(C++) | 洛谷 P1179 数字统计
本文分享的必刷题目是从蓝桥云课、洛谷、AcWing等知名刷题平台精心挑选而来,并结合各平台提供的算法标签和难度等级进行了系统分类。题目涵盖了从基础到进阶的多种算法和数据结构,旨在为不同阶段的编程学习者提供一条清晰、平稳的学习提升路径。欢迎大家订阅我的专栏:算法题解:C++与Python实现!附上汇总贴:算法竞赛备考冲刺必刷题(C++)|汇总【题目来源】洛谷:P1179[NOIP2010普及组]数字
- 算法竞赛备考冲刺必刷题(C++) | 洛谷 P1109 学生分组
热爱编程的通信人
算法c++开发语言
本文分享的必刷题目是从蓝桥云课、洛谷、AcWing等知名刷题平台精心挑选而来,并结合各平台提供的算法标签和难度等级进行了系统分类。题目涵盖了从基础到进阶的多种算法和数据结构,旨在为不同阶段的编程学习者提供一条清晰、平稳的学习提升路径。欢迎大家订阅我的专栏:算法题解:C++与Python实现!附上汇总贴:算法竞赛备考冲刺必刷题(C++)|汇总【题目来源】洛谷:P1109学生分组-洛谷【题目描述】有n
- 算法竞赛备考冲刺必刷题(C++) | 洛谷 P1449 后缀表达式
热爱编程的通信人
算法c++开发语言
本文分享的必刷题目是从蓝桥云课、洛谷、AcWing等知名刷题平台精心挑选而来,并结合各平台提供的算法标签和难度等级进行了系统分类。题目涵盖了从基础到进阶的多种算法和数据结构,旨在为不同阶段的编程学习者提供一条清晰、平稳的学习提升路径。欢迎大家订阅我的专栏:算法题解:C++与Python实现!附上汇总贴:算法竞赛备考冲刺必刷题(C++)|汇总【题目来源】洛谷:P1449后缀表达式-洛谷【题目描述】所
- 【华为od刷题(C++)】HJ59 找出字符串中第一个只出现一次的字符
我的代码:#include//引入输入输出流,允许使用cin和cout进行输入输出操作#include//引入字符串类,允许使用string类型#include//引入map容器,允许使用map来存储键值对数据usingnamespacestd;/*使得可以直接使用标准库中的标识符(如cin,cout,string等)而不需要每次都写std::*/intmain(){stringstr;//定义一
- 【华为od刷题(C++)】HJ60 查找组成一个偶数最接近的两个素数
m0_64866459
华为odc++开发语言
我的代码:#include//用于输入输出操作(例如cin和cout)#include//用于动态数组操作,存储可能的质数对usingnamespacestd;//判断一个数字x是否是质数(素数)//质数是指只能被1和它本身整除的数boolisprime(intx){for(inti=2;i*i>even){//读取输入的偶数vectorvec;for(inti=2;i<=even/2;++i){
- 【牛客刷题】小红的与运算
字节卷动
牛客刷题java牛客算法
文章目录一、题目介绍1.1题目描述1.2输入描述1.3输出描述1.4示例二、解题思路2.1核心算法设计2.2性能优化关键2.3算法流程图三、解法实现3.1解法一:基础实现3.1.1初级版本分析3.2解法二:优化版本(推荐)3.2.1优化版本分析四、总结与拓展4.1关键优化技术4.2算法正确性证明一、题目介绍1.1题目描述小红拿到了一个数组,她想在其中选择kkk个数,使得这kk
- 华为OD机试统一考试D卷C卷 - 整数对最小和 python
打卡第二十六天#和牛牛一起刷题打卡#第一天第一天南京市电信有消息了吗有没有投递IT岗的兄弟啊树米科技嵌入式实习面经1在tcp三次握手中,假如第三次握手失败,客户端仍然发送数据到服务端,此时服务端和会怎么处理?发#牛客在线求职答疑中心(35799)##牛客在线求职答疑中心#三环到底是什么套路#牛客在线求职答疑中心(35799)##牛客在线求职答疑中心#国能技经院博士待遇#牛客在线求职答疑中心(357
- 【华为OD机试真题 2025B卷】784、跳格子2 | 机试真题+思路参考+代码解析(C++、Java、Py、C语言、JS)
KJ.JK
OJ+最新华为OD机试(C++JavaPyCJS)华为odc++java华为OD机试真题2025B卷跳格子2
文章目录一、题目题目描述输入输出样例1样例2样例3样例4二、代码与思路参考C++语言思路C++代码Java语言思路Java代码Python语言思路Python代码C语言思路C代码JS语言思路JS代码作者:KJ.JK订阅本专栏后即可解锁在线OJ刷题权限专栏介绍:最新的华为OD机试题目总结,使用C++、Java、Python、C语言、JS五种语言进行解答,每个题目的思路分析都非常详细,支持在线OJ评测
- 【华为OD机试真题 2025B卷】138、计算快递主站点 | 机试真题+思路参考+代码解析(C++、Java、Py、C语言、JS)
KFickle
最新华为OD机试(C++JavaPyCJS)+OJ华为odc++java华为OD机试真题javascript计算快递主站点
文章目录一、题目题目描述输入输出样例1样例2二、代码与思路参考C++代码Java代码Python代码C语言代码JS代码订阅本专栏后即可解锁在线OJ刷题权限个人博客首页:KFickle专栏介绍:最新的华为OD机试真题,使用C++,Java,Python,C语言,JS五种语言进行解答,每个题目都包含解题思路,五种语言的解法,每日持续更新中,订阅后支持开通在线OJ测试刷题!!!一次订阅永久享受更新,有代
- Leetcode刷题笔记——栈篇
code_lover_forever
Leetcode刷题笔记leetcode笔记算法python
Leetcode刷题笔记——栈篇栈的简介栈是一种先进后出的数据结构(FirstInLastOut),栈作为一种数据结构,是一种只能在一端进行插入和删除操作的特殊线性表,这里我不做过多介绍,栈的应用和练习算是面试中的高频考点了,接下来看下我们来看一下Leetcode关于栈的常见面试题题型,每道题都附上了简单明了的python解法,大家重点关注算法思想即可一、栈在括号匹配中的应用第一题:括号的最大嵌套
- C/C++ 知识总结
灿烂阳光g
后端
目录C/C++STL数据结构算法Problems操作系统计算机网络网络编程数据库设计模式链接装载库海量数据处理音视频其他书籍复习刷题网站招聘时间岗位面试题目经验C/C++const作用修饰变量,说明该变量不可以被改变;修饰指针,分为指向常量的指针和指针常量;常量引用,经常用于形参类型,即避免了拷贝,又避免了函数对值的修改;修饰成员函数,说明该成员函数内不能修改成员变量。使用const使用stati
- Leetcode链表刷题之:61.旋转链表
小小数据挖掘工程师
面试题LeetCode编程练习链表leetcode
题目描述:给定一个链表,旋转链表,将链表每个节点向右移动k个位置,其中k是非负数。示例1:输入:1->2->3->4->5->NULL,k=2输出:4->5->1->2->3->NULL解释:向右旋转1步:5->1->2->3->4->NULL向右旋转2步:4->5->1->2->3->NULL示例2:输入:0->1->2->NULL,k=4输出:2->0->1->NULL解释:向右旋转1步:2-
- 揭秘 LeetCode 数据结构与算法的高效学习方法
数据结构与算法学习
leetcode学习方法算法ai
揭秘LeetCode数据结构与算法的高效学习方法关键词:LeetCode、数据结构、算法、高效学习、解题技巧、知识体系、面试准备摘要:本文以“如何高效通过LeetCode学习数据结构与算法”为核心,结合新手常见痛点(如刷题没方向、刷完就忘、无法举一反三),通过生活化类比、具体案例拆解和可操作的学习方法,系统讲解从“入门到精通”的全流程。无论是求职准备的程序员,还是想提升编程能力的学习者,都能从中找
- 力扣刷题笔记 贪心篇
INlinKC
leetcode
总结先放在前面:贪心的本质是选择每一阶段的局部最优,从而达到全局最优。解答贪心时的一些小技巧与注意点:1.贪心问题在需要多维度进行考虑时,可以先从某一个维度开始贪心,然后再从另一个,比如NO.135.分发糖果中,既需要考虑左边孩子的糖果数又需要考虑右边孩子的糖果数,我们先从左往右保证右边一定大于左边,再从右往左保证左边一定大于右边(注意次数需要对比一下两次遍历中糖果数可能会不同,不同时需要去较大的
- leetcode-hot100 堆
迪滴第
java开发语言
目录Java堆相关知识点1.堆的初始化2.堆的相关函数3.堆的原理4.运用堆进行排序5.怎么用优先队列实现大顶堆:示例代码自定义比较器实现大顶堆总结刷题:数组中的第K个最大元素前K个高频元素数据流的中位数Java堆相关知识点1.堆的初始化在Java中,堆是一种数据结构,通常用于实现优先队列。堆可以通过PriorityQueue类来初始化。PriorityQueue是一个基于优先级的无界队列,底层实
- 来,C语言刷题(中)(保姆式详解)
白子寰
C语言题集c语言算法
目录关于VS2022调试技巧步骤一步骤二步骤三关于Debug和Release版本区别编程题1.计算求和2.水仙花数3.打印菱形4.喝汽水问题递归题组(1)关于递归的描述(2)打印一个整数的每一位(3)求阶层①递归方式②非递归方式(4)计算一个数的每位之和(5)n的k次方操作符讲解(1)进制位的转化(2)原码,反码,补码(3)按位异或^(4)按位或与&编程题(1)交换两个变量(2)统计二进制中1的个
- 【2025B卷专题】华为OD机试2025B卷统一考试题库清单,时间紧张就刷这个
专栏导读本专栏收录于《华为OD机试(JAVA)真题(B卷+A卷+C卷+D卷+E卷)》。刷的越多,抽中的概率越大,私信哪吒,备注华为OD,加入华为OD刷题交流群,每一题都有详细的答题思路、详细的代码注释、3个测试用例、为什么这道题采用XX算法、XX算法的适用场景,发现新题目,随时更新,全天CSDN在线答疑。2025年5月12日,华为官方已经将华为OD机试(A卷)切换为B卷。目前正在考的是B卷,按照华
- 【2025B卷专题】华为OD机试2025B卷统一考试题库清单,时间紧张就刷这个(Python/JS/C/C++)
哪 吒
搬砖工逆袭Java架构师华为odpythonjavascript华为OD机试2025B卷
专栏导读本专栏收录于《华为OD机试真题(Python/JS/C/C++)》。刷的越多,抽中的概率越大,私信哪吒,备注华为OD,加入华为OD刷题交流群,每一题都有详细的答题思路、详细的代码注释、3个测试用例、为什么这道题采用XX算法、XX算法的适用场景,发现新题目,随时更新。2025年5月12日,华为官方已经将华为OD机试(A卷)切换为B卷。目前正在考的是B卷,按照华为OD往常的操作,B卷题目是由往
- 【全网首发】华为OD机试 2025B卷 机考真题库清单(全真题库)含考点说明
哪 吒
搬砖工逆袭Java架构师华为odjava2025B卷华为OD机试
专栏导读本专栏收录于《华为OD机试(JAVA)真题(E卷+D卷+A卷+B卷+C卷)》。刷的越多,抽中的概率越大,私信哪吒,备注华为OD,加入华为OD刷题交流群,每一题都有详细的答题思路、详细的代码注释、3个测试用例、为什么这道题采用XX算法、XX算法的适用场景,发现新题目,随时更新,全天CSDN在线答疑。2025年5月12日,华为官方已经将华为OD机试(A卷)切换为B卷。目前正在考的是B卷,按照华
- 【2025B卷首发】华为OD机试真题+全流程解析+备考攻略+经验分享+Java最佳实现
专栏导读本专栏收录于《华为OD机试(JAVA)真题(E卷+D卷+A卷+B卷+C卷)》。刷的越多,抽中的概率越大,私信哪吒,备注华为OD,加入华为OD刷题交流群,每一题都有详细的答题思路、详细的代码注释、3个测试用例、为什么这道题采用XX算法、XX算法的适用场景,发现新题目,随时更新,全天CSDN在线答疑。2025年5月12日,华为官方已经将华为OD机试(A卷)切换为B卷。目前正在考的是B卷,按照华
- LeetCode 刷题:数据结构与算法的实战经验分享
LeetCode刷题:数据结构与算法的实战经验分享关键词:LeetCode、数据结构、算法、刷题经验、实战摘要:本文将围绕LeetCode刷题展开,深入探讨数据结构与算法在实际刷题过程中的应用。通过分享实战经验,帮助读者更好地理解和掌握数据结构与算法知识,提升解题能力。文章将从背景介绍入手,阐述刷题的目的和意义,接着详细解释核心概念,分析它们之间的关系,然后介绍核心算法原理和具体操作步骤,结合数学
- 2025B卷 - 华为OD机试七日集训第5期 - 按算法分类,由易到难,循序渐进,玩转OD
哪 吒
搬砖工逆袭Java架构师华为od算法华为OD机试2025B卷java
目录推荐刷题方法:一、适合人群二、本期训练时间三、如何参加四、七日集训第5期五、精心挑选21道高频100分经典题目,作为入门。第1天、逻辑分析第2天、逻辑分析第3天、逻辑分析第4天、字符串处理第5天、正则表达式第6天、深度优先搜索dfs第7天、深度优先搜索dfs六、集训总结国内直接使用ChatGPT4o、o3、o4-mini-high、GPT-4.5、GPT4.1、Gemini2.5pro0605
- 2025B卷 - 华为OD机试七日集训第4期 - 按算法分类,由易到难,循序渐进,玩转OD(Python/JS/C/C++)
哪 吒
搬砖工逆袭Java架构师华为od算法python华为OD机试2025B卷
目录推荐刷题方法:一、适合人群二、本期训练时间三、如何参加四、七日集训第4期五、精心挑选21道高频100分经典题目,作为入门。第1天、逻辑分析第2天、逻辑分析第3天、逻辑分析第4天、贪心算法第5天、二分查找第6天、字符串处理第7天、字符串处理六、集训总结国内直接使用ChatGPT4o、o3、o4-mini-high、GPT-4.5、GPT4.1、Gemini2.5pro0605、ClaudeSon
- 2025B卷 - 华为OD机试七日集训第2期 - 按算法分类,由易到难,循序渐进,玩转OD(Python/JS/C/C++)
目录推荐刷题方法:一、适合人群二、本期训练时间三、如何参加四、七日集训第2期五、精心挑选21道高频100分经典题目,作为入门。第1天、逻辑分析第2天、数组第3天、双指针第4天、贪心算法第5天、字符串处理第6天、深度优先搜索DFS第7天、动态规划六、集训总结国内直接使用ChatGPT4o、o3、o4-mini-high、GPT-4.5、GPT4.1、Gemini2.5pro0605、ClaudeSo
- 【华为od刷题(C++)】HJ52 计算字符串的编辑距离
m0_64866459
华为odc++开发语言
我的代码:#include//用于输入输出#include//包含一些常用算法,如min等#include//用于动态数组(二维数组)#include//用于处理字符串usingnamespacestd;intmain(){stringstr1,str2;//str1和str2是输入的两个字符串while(cin>>str1>>str2){intn=str1.size();intm=str2.si
- 暑假算法刷题日记 Day 10
mjh_yylx
算法刷题打卡算法
目录重点整理054、拼数题目描述输入格式输出格式输入输出样例核心思路代码055、求第k小的数题目描述输入格式输出格式输入输出样例核心思路代码总结这几天我们主要刷了洛谷上排序算法对应的一些题目,相对来说比较简单一共是13道题,对应我暑假刷题的043--055。当然这些题目相对来说比较简单,我们挑着重点的说。重点整理排序这一块的题目总体来看包括,1.基本的排序算法,像快速排序、分治排序,这些知识点我写
- 力扣刷题——位运算—只出现1次的数字
丢丢diu丢
力扣刷题思考leetcode
137.只出现一次的数字II(其他都出现了3次)题目分析1.每个整数都是32位的2进制;2.因为除了目标元素res外,其他元素都出现了3次,所以,其他元素们在同1个二进制位置上的加和sum对3取余因该是0,如果不是0,而是1,说明这是res所在的1;3.依次遍历32位,每1位都对nums数组中的元素求和classSolution{publicintsingleNumber(int[]nums){i
- 【C语言】学习过程教训与经验杂谈:思想准备、知识回顾(五)
个人主页:艾莉丝努力练剑❄专栏传送门:《C语言》、《数据结构与算法》、C语言刷题12天IO强训、LeetCode代码强化刷题学习方向:C/C++方向⭐️人生格言:为天地立心,为生民立命,为往圣继绝学,为万世开太平前言:我们在学习过程中会碰到很多很多问题,本系列文章不会博主不会额外再创建一个新的专栏来收录,因为这一系列文章创作的初心主要是针对回顾知识点(遵循遗忘曲线并且根据自身的实际情况可以做出一些
- C/C++Win32编程基础详解视频下载
择善Zach
编程C++Win32
课题视频:C/C++Win32编程基础详解
视频知识:win32窗口的创建
windows事件机制
主讲:择善Uncle老师
学习交流群:386620625
验证码:625
--
- Guava Cache使用笔记
bylijinnan
javaguavacache
1.Guava Cache的get/getIfPresent方法当参数为null时会抛空指针异常
我刚开始使用时还以为Guava Cache跟HashMap一样,get(null)返回null。
实际上Guava整体设计思想就是拒绝null的,很多地方都会执行com.google.common.base.Preconditions.checkNotNull的检查。
2.Guava
- 解决ora-01652无法通过128(在temp表空间中)
0624chenhong
oracle
解决ora-01652无法通过128(在temp表空间中)扩展temp段的过程
一个sql语句后,大约花了10分钟,好不容易有一个结果,但是报了一个ora-01652错误,查阅了oracle的错误代码说明:意思是指temp表空间无法自动扩展temp段。这种问题一般有两种原因:一是临时表空间空间太小,二是不能自动扩展。
分析过程:
既然是temp表空间有问题,那当
- Struct在jsp标签
不懂事的小屁孩
struct
非UI标签介绍:
控制类标签:
1:程序流程控制标签 if elseif else
<s:if test="isUsed">
<span class="label label-success">True</span>
</
- 按对象属性排序
换个号韩国红果果
JavaScript对象排序
利用JavaScript进行对象排序,根据用户的年龄排序展示
<script>
var bob={
name;bob,
age:30
}
var peter={
name;peter,
age:30
}
var amy={
name;amy,
age:24
}
var mike={
name;mike,
age:29
}
var john={
- 大数据分析让个性化的客户体验不再遥远
蓝儿唯美
数据分析
顾客通过多种渠道制造大量数据,企业则热衷于利用这些信息来实现更为个性化的体验。
分析公司Gartner表示,高级分析会成为客户服务的关键,但是大数据分析的采用目前仅局限于不到一成的企业。 挑战在于企业还在努力适应结构化数据,疲于根据自身的客户关系管理(CRM)系统部署有效的分析框架,以及集成不同的内外部信息源。
然而,面对顾客通过数字技术参与而产生的快速变化的信息,企业需要及时作出反应。要想实
- java笔记4
a-john
java
操作符
1,使用java操作符
操作符接受一个或多个参数,并生成一个新值。参数的形式与普通的方法调用不用,但是效果是相同的。加号和一元的正号(+)、减号和一元的负号(-)、乘号(*)、除号(/)以及赋值号(=)的用法与其他编程语言类似。
操作符作用于操作数,生成一个新值。另外,有些操作符可能会改变操作数自身的
- 从裸机编程到嵌入式Linux编程思想的转变------分而治之:驱动和应用程序
aijuans
嵌入式学习
笔者学习嵌入式Linux也有一段时间了,很奇怪的是很多书讲驱动编程方面的知识,也有很多书将ARM9方面的知识,但是从以前51形式的(对寄存器直接操作,初始化芯片的功能模块)编程方法,和思维模式,变换为基于Linux操作系统编程,讲这个思想转变的书几乎没有,让初学者走了很多弯路,撞了很多难墙。
笔者因此写上自己的学习心得,希望能给和我一样转变
- 在springmvc中解决FastJson循环引用的问题
asialee
循环引用fastjson
我们先来看一个例子:
package com.elong.bms;
import java.io.OutputStream;
import java.util.HashMap;
import java.util.Map;
import co
- ArrayAdapter和SimpleAdapter技术总结
百合不是茶
androidSimpleAdapterArrayAdapter高级组件基础
ArrayAdapter比较简单,但它只能用于显示文字。而SimpleAdapter则有很强的扩展性,可以自定义出各种效果
ArrayAdapter;的数据可以是数组或者是队列
// 获得下拉框对象
AutoCompleteTextView textview = (AutoCompleteTextView) this
- 九封信
bijian1013
人生励志
有时候,莫名的心情不好,不想和任何人说话,只想一个人静静的发呆。有时候,想一个人躲起来脆弱,不愿别人看到自己的伤口。有时候,走过熟悉的街角,看到熟悉的背影,突然想起一个人的脸。有时候,发现自己一夜之间就长大了。 2014,写给人
- Linux下安装MySQL Web 管理工具phpMyAdmin
sunjing
PHPInstallphpMyAdmin
PHP http://php.net/
phpMyAdmin http://www.phpmyadmin.net
Error compiling PHP on CentOS x64
一、安装Apache
请参阅http://billben.iteye.com/admin/blogs/1985244
二、安装依赖包
sudo yum install gd
- 分布式系统理论
bit1129
分布式
FLP
One famous theory in distributed computing, known as FLP after the authors Fischer, Lynch, and Patterson, proved that in a distributed system with asynchronous communication and process crashes,
- ssh2整合(spring+struts2+hibernate)-附源码
白糖_
eclipsespringHibernatemysql项目管理
最近抽空又整理了一套ssh2框架,主要使用的技术如下:
spring做容器,管理了三层(dao,service,actioin)的对象
struts2实现与页面交互(MVC),自己做了一个异常拦截器,能拦截Action层抛出的异常
hibernate与数据库交互
BoneCp数据库连接池,据说比其它数据库连接池快20倍,仅仅是据说
MySql数据库
项目用eclipse
- treetable bug记录
braveCS
table
// 插入子节点删除再插入时不能正常显示。修改:
//不知改后有没有错,先做个备忘
Tree.prototype.removeNode = function(node) {
// Recursively remove all descendants of +node+
this.unloadBranch(node);
// Remove
- 编程之美-电话号码对应英语单词
bylijinnan
java算法编程之美
import java.util.Arrays;
public class NumberToWord {
/**
* 编程之美 电话号码对应英语单词
* 题目:
* 手机上的拨号盘,每个数字都对应一些字母,比如2对应ABC,3对应DEF.........,8对应TUV,9对应WXYZ,
* 要求对一段数字,输出其代表的所有可能的字母组合
- jquery ajax读书笔记
chengxuyuancsdn
jQuery ajax
1、jsp页面
<%@ page language="java" import="java.util.*" pageEncoding="GBK"%>
<%
String path = request.getContextPath();
String basePath = request.getScheme()
- JWFD工作流拓扑结构解析伪码描述算法
comsci
数据结构算法工作活动J#
对工作流拓扑结构解析感兴趣的朋友可以下载附件,或者下载JWFD的全部代码进行分析
/* 流程图拓扑结构解析伪码描述算法
public java.util.ArrayList DFS(String graphid, String stepid, int j)
- oracle I/O 从属进程
daizj
oracle
I/O 从属进程
I/O从属进程用于为不支持异步I/O的系统或设备模拟异步I/O.例如,磁带设备(相当慢)就不支持异步I/O.通过使用I/O 从属进程,可以让磁带机模仿通常只为磁盘驱动器提供的功能。就好像支持真正的异步I/O 一样,写设备的进程(调用者)会收集大量数据,并交由写入器写出。数据成功地写出时,写入器(此时写入器是I/O 从属进程,而不是操作系统)会通知原来的调用者,调用者则会
- 高级排序:希尔排序
dieslrae
希尔排序
public void shellSort(int[] array){
int limit = 1;
int temp;
int index;
while(limit <= array.length/3){
limit = limit * 3 + 1;
- 初二下学期难记忆单词
dcj3sjt126com
englishword
kitchen 厨房
cupboard 厨柜
salt 盐
sugar 糖
oil 油
fork 叉;餐叉
spoon 匙;调羹
chopsticks 筷子
cabbage 卷心菜;洋白菜
soup 汤
Italian 意大利的
Indian 印度的
workplace 工作场所
even 甚至;更
Italy 意大利
laugh 笑
m
- Go语言使用MySQL数据库进行增删改查
dcj3sjt126com
mysql
目前Internet上流行的网站构架方式是LAMP,其中的M即MySQL, 作为数据库,MySQL以免费、开源、使用方便为优势成为了很多Web开发的后端数据库存储引擎。MySQL驱动Go中支持MySQL的驱动目前比较多,有如下几种,有些是支持database/sql标准,而有些是采用了自己的实现接口,常用的有如下几种:
http://code.google.c...o-mysql-dri
- git命令
shuizhaosi888
git
---------------设置全局用户名:
git config --global user.name "HanShuliang" //设置用户名
git config --global user.email "
[email protected]" //设置邮箱
---------------查看环境配置
git config --li
- qemu-kvm 网络 nat模式 (四)
haoningabc
kvmqemu
qemu-ifup-NAT
#!/bin/bash
BRIDGE=virbr0
NETWORK=192.168.122.0
GATEWAY=192.168.122.1
NETMASK=255.255.255.0
DHCPRANGE=192.168.122.2,192.168.122.254
TFTPROOT=
BOOTP=
function check_bridge()
- 不要让未来的你,讨厌现在的自己
jingjing0907
生活 奋斗 工作 梦想
故事one
23岁,他大学毕业,放弃了父母安排的稳定工作,独闯京城,在家小公司混个小职位,工作还算顺手,月薪三千,混了混,混走了一年的光阴。 24岁,有了女朋友,从二环12人的集体宿舍搬到香山民居,一间平房,二人世界,爱爱爱。偶然约三朋四友,打扑克搓麻将,日子快乐似神仙; 25岁,出了几次差,调了两次岗,薪水涨了不过百,生猛狂飙的物价让现实血淋淋,无力为心爱银儿购件大牌
- 枚举类型详解
一路欢笑一路走
enum枚举详解enumsetenumMap
枚举类型详解
一.Enum详解
1.1枚举类型的介绍
JDK1.5加入了一个全新的类型的”类”—枚举类型,为此JDK1.5引入了一个新的关键字enum,我们可以这样定义一个枚举类型。
Demo:一个最简单的枚举类
public enum ColorType {
RED
- 第11章 动画效果(上)
onestopweb
动画
index.html
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/
- Eclipse中jsp、js文件编辑时,卡死现象解决汇总
ljf_home
eclipsejsp卡死js卡死
使用Eclipse编辑jsp、js文件时,经常出现卡死现象,在网上百度了N次,经过N次优化调整后,卡死现象逐步好转,具体那个方法起到作用,不太好讲。将所有用过的方法罗列如下:
1、取消验证
windows–>perferences–>validation
把 除了manual 下面的全部点掉,build下只留 classpath dependency Valida
- MySQL编程中的6个重要的实用技巧
tomcat_oracle
mysql
每一行命令都是用分号(;)作为结束
对于MySQL,第一件你必须牢记的是它的每一行命令都是用分号(;)作为结束的,但当一行MySQL被插入在PHP代码中时,最好把后面的分号省略掉,例如:
mysql_query("INSERT INTO tablename(first_name,last_name)VALUES('$first_name',$last_name')");
- zoj 3820 Building Fire Stations(二分+bfs)
阿尔萨斯
Build
题目链接:zoj 3820 Building Fire Stations
题目大意:给定一棵树,选取两个建立加油站,问说所有点距离加油站距离的最大值的最小值是多少,并且任意输出一种建立加油站的方式。
解题思路:二分距离判断,判断函数的复杂度是o(n),这样的复杂度应该是o(nlogn),即使常数系数偏大,但是居然跑了4.5s,也是醉了。 判断函数里面做了3次bfs,但是每次bfs节点最多