Android 贝塞尔曲线

我们在实际开发中，肯定会遇到自定义控件，有时候我们也会遇到曲线的处理，今天我们就来学习下大名鼎鼎的贝塞尔曲线。

贝塞尔曲线(Bézier curve)叫贝兹曲线，是计算机图形学中非常重要的参数曲线。如qq消息提醒拖拽红点，阅读器翻书效果等等，在实际软件工具中，比如ps中的钢笔工具核心就是贝塞尔曲线。贝塞尔曲线常见的三种：一阶曲线，二阶曲线，三阶曲线。那我们怎么实现呢？

讲之前我们先看一下，android.graphics.Path类吧。看看具体的一些方法：

1.moveTo(float x, float y)设置绘制的起始点。

 /**
     * Set the beginning of the next contour to the point (x,y).
     */
    public void moveTo(float x, float y) {
        native_moveTo(mNativePath, x, y);
    }

2.lineTo(float x, float y) 画一条直线，终点为(x,y)，那起始点呢，如果你设置了moveTo，那就是从moveTo对应的点作为起始点，默认则从(0,0)点。

这个也就是我们常说的一阶贝塞尔曲线的形式，画直线。

 /**
     * Add a line from the last point to the specified point (x,y).
     * If no moveTo() call has been made for this contour, the first point is
     * automatically set to (0,0).
     */
    public void lineTo(float x, float y) {
        isSimplePath = false;
        native_lineTo(mNativePath, x, y);
    }

3.quadTo:根据两个点绘制贝塞尔曲线，我们在 二阶贝塞尔曲线时用到quadTo函数。根据函数描述，我们可以得到x1，y1是一个控制点，还有结束点x2，y2，结合上边第一个函数，moveTo是设置起始点，如果没有调用此函数，默认设置为(0，0)点。

 /**
     * Add a quadratic bezier from the last point, approaching control point
     * (x1,y1), and ending at (x2,y2). If no moveTo() call has been made for
     * this contour, the first point is automatically set to (0,0).
     *
     * @param x1 The x-coordinate of the control point on a quadratic curve
     * @param y1 The y-coordinate of the control point on a quadratic curve
     * @param x2 The x-coordinate of the end point on a quadratic curve
     * @param y2 The y-coordinate of the end point on a quadratic curve
     */
    public void quadTo(float x1, float y1, float x2, float y2) {
        isSimplePath = false;
        native_quadTo(mNativePath, x1, y1, x2, y2);
    }

4.cubicTo：同quadTo函数类似，只不过多了一个控制点，我们在三节贝塞尔曲线时用到此函数。

     * Add a cubic bezier from the last point, approaching control points
     * (x1,y1) and (x2,y2), and ending at (x3,y3). If no moveTo() call has been
     * made for this contour, the first point is automatically set to (0,0).
     *
     * @param x1 The x-coordinate of the 1st control point on a cubic curve
     * @param y1 The y-coordinate of the 1st control point on a cubic curve
     * @param x2 The x-coordinate of the 2nd control point on a cubic curve
     * @param y2 The y-coordinate of the 2nd control point on a cubic curve
     * @param x3 The x-coordinate of the end point on a cubic curve
     * @param y3 The y-coordinate of the end point on a cubic curve
     */
    public void cubicTo(float x1, float y1, float x2, float y2,
                        float x3, float y3) {
        isSimplePath = false;
        native_cubicTo(mNativePath, x1, y1, x2, y2, x3, y3);
    }

5.arcTo：画圆弧。

    /**
     * Append the specified arc to the path as a new contour. If the start of
     * the path is different from the path's current last point, then an
     * automatic lineTo() is added to connect the current contour to the
     * start of the arc. However, if the path is empty, then we call moveTo()
     * with the first point of the arc.
     *
     * @param oval        The bounds of oval defining shape and size of the arc
     * @param startAngle  Starting angle (in degrees) where the arc begins
     * @param sweepAngle  Sweep angle (in degrees) measured clockwise
     */
    public void arcTo(RectF oval, float startAngle, float sweepAngle) {
        arcTo(oval.left, oval.top, oval.right, oval.bottom, startAngle, sweepAngle, false);
    }

上述几个方法这是Path的一部分方法，其他自己看一下熟悉下。讲了Path类，那我们来看看贝塞尔曲线的原理图（均取自网络图片）：

1.一阶的其实就相对比较简单了。就是绘制直线。两点控制，默认起始点(0，0)

2.二阶贝塞尔曲线：

上边讲Path类时，说到了二阶曲线用到path对象的quadTo方法，P0是起始点，P2是结束点，P1是控制点，而红色描述的线就是最终的二阶贝塞尔曲线。根据P1的位置不同，在P0,P2中点之上或之下，也就绘制了不同的向下曲线或向上曲线。

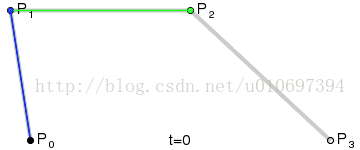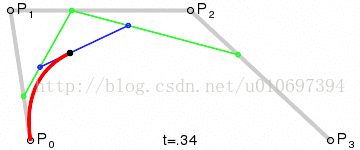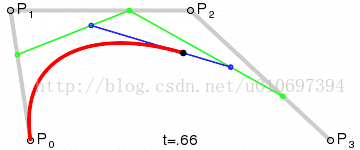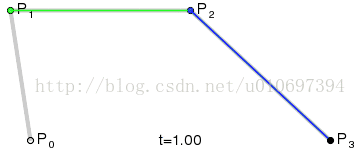
3.三阶贝塞尔曲线：

三阶贝塞尔曲线，相对二阶贝塞尔曲线，只不过多了一个控制点。其中p1和p2是控制点。