Partial differential equations and the finite element method 3-FEA1.md

This part is devoted to introduce the Galerkin method and its important special case, the Finite element method.

Consider the general framework

Let V V be a Hilbert space, a(.,.):V×V→R a ( . , . ) : V × V → R a bilinear form and l∈V′ l ∈ V ′ . It is our task to find u∈V u ∈ V such that
(1)

a(u,v)=l(v) a ( u , v ) = l ( v )

We assume that the bilinear form a(.,.) a ( . , . ) is bounded and V-elliptic, i.e., that there exist constants Cb,Cel>0 C b , C e l > 0 such that

|a(u,v)|≤Cb∥u∥V∥v∥V | a ( u , v ) | ≤ C b ∥ u ∥ V ∥ v ∥ V

and

a(u,v)≥Cel∥v∥2V a ( u , v ) ≥ C e l ∥ v ∥ V 2

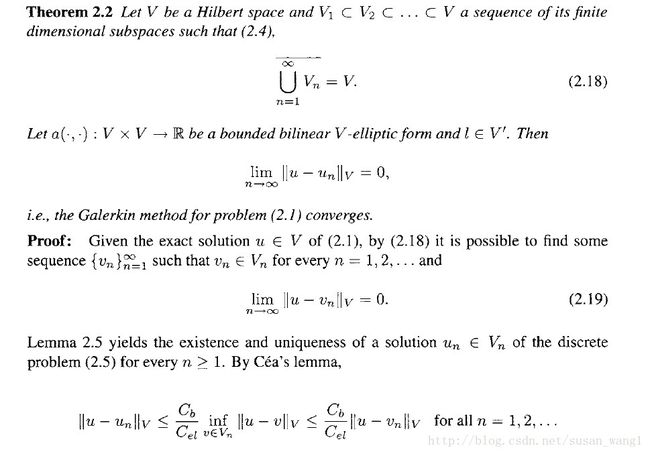
Recall that the weak problem (1) has a unique solution by the Lax-Milgram lemma.

---

The Galerkin method

As V V is infinite dim function space. The Galerkin method is based on a sequence of finite dimensional subspaces {Vn}∞n=1⊂V { V n } n = 1 ∞ ⊂ V , Vn⊂Vn+1 V n ⊂ V n + 1 , that fill the space V V in the limit. In each finite-dimensional space Vn V n , problem (1) is solved exactly. It can be shown that under suitable assumptions the sequence of the approximate solutions {un}∞n=1 { u n } n = 1 ∞ converges to the exact solution of problem (1).

Discrete problem

Find un∈Vn u n ∈ V n such that
(2)

a(un,v)=l(v),v∈Vn a ( u n , v ) = l ( v ) , v ∈ V n

Lemma 1 (Unique solvability) Problem (2) has a unique solution un∈Vn u n ∈ V n ,.

Proof: Recall Lax-Milgram lemma : Let V V be a Hilbert space, a(.,.):V×V→R a ( . , . ) : V × V → R a bounded V-elliptic bilinear form and l∈V′ l ∈ V ′ Then there exists a unique solution to the problem

a(u,v)=l(v) a ( u , v ) = l ( v )

.

Suppose space V V has a finite basis {vn}Nnn=1 { v n } n = 1 N n , then

un=∑j=1Nnyjvj u n = ∑ j = 1 N n y j v j

where yj y j s are unknown coefficients.

a(un,vi)=∑j=1Nnyja(vj,vi)=l(vI) a ( u n , v i ) = ∑ j = 1 N n y j a ( v j , v i ) = l ( v I )

Denote Sn={a(vj,vi)}Ni,j=1 S n = { a ( v j , v i ) } i , j = 1 N , Fn={l(vi)} F n = { l ( v i ) } , Yn={yj} Y n = { y j } , then
(3)

SnYn=Fn S n Y n = F n

.

Lemma 2 (Positive definiteness of Sn S n ) Let Vn V n be a Hilbert space and a(.,.):V×V→R a ( . , . ) : V × V → R a bilinear V-elliptic form. Then the stiffness matrix Sn S n , of the discrete problem (3) is positive definite.

About the error u−un u − u n

Lemma 3 (Orthogonality of error for elliptic problems) Let u∈V u ∈ V be the exact solution of the continuous problem (I ) and un u n the exact solution of the discrete problem (3).
Then the error en=u−un e n = u − u n , satisjies

a(u−un,v)=0,∀v∈Vn a ( u − u n , v ) = 0 , ∀ v ∈ V n

.
Proof:Because Vn⊂V V n ⊂ V , then

a(u−un,v)=a(u,v)−a(un,v)=0. a ( u − u n , v ) = a ( u , v ) − a ( u n , v ) = 0.

Remark 1 (Geometrical interpretation) If the bilinear form a(.,.) a ( . , . ) is symmetric, it induces an energetic inner product, then

(en,v)e=0,∀v∈Vn ( e n , v ) e = 0 , ∀ v ∈ V n

,

i.e., that the error of the Galerkin approximation en=u−un e n = u − u n is orthogonal to the Galerkin subspace Vn V n in the energetic inner product. Hence the approximate solution u, E V, is an orthogonal projection of the exact solution u u onto the Galerkin subspace Vn V n in the energetic inner product, and thus it is the nearest element in the space Vn V n to the exact solution u u in the energy norm,

∥u−un∥e=infv∈Vn∥u−v∥e ∥ u − u n ∥ e = inf v ∈ V n ∥ u − v ∥ e

---

FEA

Let Ω⊂Rd Ω ⊂ R d , where d d is the spatial dimension, be an open bounded set. If the Hilbert space V V consists of functions defined in Ω Ω and the Galerkin subspaces Vn V n comprise piecewise-polynomial functions, the Galerkin method is called the Finite element method (FEM).

Consider the model equation

−∇(a1∇u)+a0u=f, − ∇ ( a 1 ∇ u ) + a 0 u = f ,

where f∈L2(Ω) f ∈ L 2 ( Ω ) , in a bounded interval Ω=(a,b)⊂R Ω = ( a , b ) ⊂ R , equipped with the homogeneous Dirichlet boundary conditions. At the beginning let a1 a 1 and a0 a 0 be constants and assume a simple load function of the form f(x)=1 f ( x ) = 1 .

The Galerkin procedure assumes a sequence of finite-dimensional subspaces

V1⊂V2⊂…⊂V V 1 ⊂ V 2 ⊂ … ⊂ V

Consider a partition

a=x(n)0<x(n)1<…<x(n)Mn=b a = x 0 ( n ) < x 1 ( n ) < … < x M n ( n ) = b

and define the finite element mesh

\mathcal {T}_n=\{K_1^{(n),K_2^{(n),\ldots,K_{M_n}^{(n)\} \mathcal {T}_n=\{K_1^{(n),K_2^{(n),\ldots,K_{M_n}^{(n)\}

The open intervals

K_i^{(n)=(x_{i-1}^{(n)},x_i^{(n)}) K_i^{(n)=(x_{i-1}^{(n)},x_i^{(n)})

are called finite elements, and the value

h(n)=max(x(n)i−x(n)i−1) h ( n ) = m a x ( x i ( n ) − x i − 1 ( n ) )

is said to be the mesh diameter.

The piecewise linear basis functions vj v j , satisfy vj(xi)=δij v j ( x i ) = δ i j .

Then by (3), we can get un u n .