二分图最大匹配－匈牙利算法－HDU2063-HDU1068

这篇文章讲无权二分图（unweighted bipartite graph）的最大匹配（maximum matching）和完美匹配（perfect matching），以及用于求解匹配的匈牙利算法（Hungarian Algorithm）；不讲带权二分图的最佳匹配。

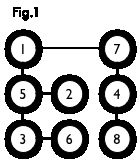
二分图：简单来说，如果图中点可以被分为两组，并且使得所有边都跨越组的边界，则这就是一个二分图。准确地说：把一个图的顶点划分为两个不相交集 U 和 V ，使得每一条边都分别连接 U 、 V 中的顶点。如果存在这样的划分，则此图为一个二分图。二分图的一个等价定义是：不含有「含奇数条边的环」的图。图 1 是一个二分图。为了清晰，我们以后都把它画成图 2 的形式。

匹配：在图论中，一个「匹配」（matching）是一个边的集合，其中任意两条边都没有公共顶点。例如，图 3、图 4 中红色的边就是图 2 的匹配。

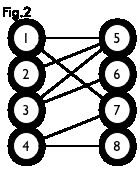
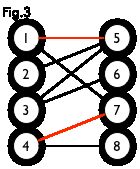
我们定义匹配点、匹配边、未匹配点、非匹配边，它们的含义非常显然。例如图 3 中 1、4、5、7 为匹配点，其他顶点为未匹配点；1-5、4-7为匹配边，其他边为非匹配边。

最大匹配：一个图所有匹配中，所含匹配边数最多的匹配，称为这个图的最大匹配。图 4 是一个最大匹配，它包含 4 条匹配边。

完美匹配：如果一个图的某个匹配中，所有的顶点都是匹配点，那么它就是一个完美匹配。图 4 是一个完美匹配。显然，完美匹配一定是最大匹配（完美匹配的任何一个点都已经匹配，添加一条新的匹配边一定会与已有的匹配边冲突）。但并非每个图都存在完美匹配。

举例来说：如下图所示，如果在某一对男孩和女孩之间存在相连的边，就意味着他们彼此喜欢。是否可能让所有男孩和女孩两两配对，使得每对儿都互相喜欢呢？图论中，这就是完美匹配问题。如果换一个说法：最多有多少互相喜欢的男孩/女孩可以配对儿？这就是最大匹配问题。

基本概念讲完了。求解最大匹配问题的一个算法是匈牙利算法，下面讲的概念都为这个算法服务。

交替路：从一个未匹配点出发，依次经过非匹配边、匹配边、非匹配边...形成的路径叫交替路。

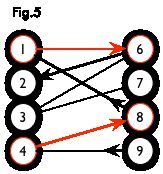
增广路：从一个未匹配点出发，走交替路，如果途径另一个未匹配点（出发的点不算），则这条交替路称为增广路（agumenting path）。例如，图 5 中的一条增广路如图 6 所示（图中的匹配点均用红色标出）：

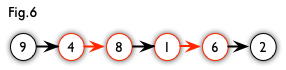
增广路有一个重要特点：非匹配边比匹配边多一条。因此，研究增广路的意义是改进匹配。只要把增广路中的匹配边和非匹配边的身份交换即可。由于中间的匹配节点不存在其他相连的匹配边，所以这样做不会破坏匹配的性质。交换后，图中的匹配边数目比原来多了 1 条。

我们可以通过不停地找增广路来增加匹配中的匹配边和匹配点。找不到增广路时，达到最大匹配（这是增广路定理）。匈牙利算法正是这么做的。在给出匈牙利算法 DFS 和 BFS 版本的代码之前，先讲一下匈牙利树。

匈牙利树一般由 BFS 构造（类似于 BFS 树）。从一个未匹配点出发运行 BFS（唯一的限制是，必须走交替路），直到不能再扩展为止。例如，由图 7，可以得到如图 8 的一棵 BFS 树：

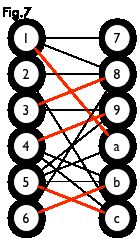
这棵树存在一个叶子节点为非匹配点（7 号），但是匈牙利树要求所有叶子节点均为匹配点，因此这不是一棵匈牙利树。如果原图中根本不含 7 号节点，那么从 2 号节点出发就会得到一棵匈牙利树。这种情况如图 9 所示（顺便说一句，图 8 中根节点 2 到非匹配叶子节点 7 显然是一条增广路，沿这条增广路扩充后将得到一个完美匹配）。

下面给出匈牙利算法的 DFS 和 BFS 版本的代码：

// 顶点、边的编号均从 0 开始
// 邻接表储存

struct Edge
{
    int from;
    int to;
    int weight;

    Edge(int f, int t, int w):from(f), to(t), weight(w) {}
};

vector G[__maxNodes]; /* G[i] 存储顶点 i 出发的边的编号 */
vector<Edge> edges;
typedef vector::iterator iterator_t;
int num_nodes;
int num_left;
int num_right;
int num_edges;

int matching[__maxNodes]; /* 存储求解结果 */
int check[__maxNodes];

bool dfs(int u)
{
    for (iterator_t i = G[u].begin(); i != G[u].end(); ++i) { // 对 u 的每个邻接点
        int v = edges[*i].to;
        if (!check[v]) {     // 要求不在交替路中
            check[v] = true; // 放入交替路
            if (matching[v] == -1 || dfs(matching[v])) {
                // 如果是未盖点，说明交替路为增广路，则交换路径，并返回成功
                matching[v] = u;
                matching[u] = v;
                return true;
            }
        }
    }
    return false; // 不存在增广路，返回失败
}

int hungarian()
{
    int ans = 0;
    memset(matching, -1, sizeof(matching));
    for (int u=0; u < num_left; ++u) {
        if (matching[u] == -1) {
            memset(check, 0, sizeof(check));
            if (dfs(u))
                ++ans;
        }
    }
    return ans;
}

queue Q;
int prev[__maxNodes];
int Hungarian()
{
    int ans = 0;
    memset(matching, -1, sizeof(matching));
    memset(check, -1, sizeof(check));
    for (int i=0; i<num_left; ++i) {
        if (matching[i] == -1) {
            while (!Q.empty()) Q.pop();
            Q.push(i);
            prev[i] = -1; // 设 i 为路径起点
            bool flag = false; // 尚未找到增广路
            while (!Q.empty() && !flag) {
                int u = Q.front();
                for (iterator_t ix = G[u].begin(); ix != G[u].end() && !flag; ++ix) {
                    int v = edges[*ix].to;
                    if (check[v] != i) {
                        check[v] = i;
                        Q.push(matching[v]);
                        if (matching[v] >= 0) { // 此点为匹配点
                            prev[matching[v]] = u;
                        } else { // 找到未匹配点，交替路变为增广路
                            flag = true;
                            int d=u, e=v;
                            while (d != -1) {
                                int t = matching[d];
                                matching[d] = e;
                                matching[e] = d;
                                d = prev[d];
                                e = t;
                            }
                        }
                    }
                }
                Q.pop();
            }
            if (matching[i] != -1) ++ans;
        }
    }
    return ans;
}

匈牙利算法的要点如下

从左边第 1 个顶点开始，挑选未匹配点进行搜索，寻找增广路。
1. 如果经过一个未匹配点，说明寻找成功。更新路径信息，匹配边数 +1，停止搜索。
2. 如果一直没有找到增广路，则不再从这个点开始搜索。事实上，此时搜索后会形成一棵匈牙利树。我们可以永久性地把它从图中删去，而不影响结果。
由于找到增广路之后需要沿着路径更新匹配，所以我们需要一个结构来记录路径上的点。DFS 版本通过函数调用隐式地使用一个栈，而 BFS 版本使用 prev 数组。

性能比较

两个版本的时间复杂度均为 O(V⋅E) 。DFS 的优点是思路清晰、代码量少，但是性能不如 BFS。我测试了两种算法的性能。对于稀疏图，BFS 版本明显快于 DFS 版本；而对于稠密图两者则不相上下。在完全随机数据 9000 个顶点 4,0000 条边时前者领先后者大约 97.6%，9000 个顶点 100,0000 条边时前者领先后者 8.6%, 而达到 500,0000 条边时 BFS 仅领先 0.85%。

补充定义和定理：

最大匹配数：最大匹配的匹配边的数目
最小点覆盖数：选取最少的点，使任意一条边至少有一个端点被选择
最大独立数：选取最多的点，使任意所选两点均不相连
最小路径覆盖数：对于一个 DAG（有向无环图），选取最少条路径，使得每个顶点属于且仅属于一条路径。路径长可以为 0（即单个点）。

定理1：最大匹配数 = 最小点覆盖数（这是 Konig 定理）
定理2：最大匹配数 = 最大独立数
定理3：最小路径覆盖数 = 顶点数 - 最大匹配数

二分图最大匹配－匈牙利算法－HDU2063-HDU1068－HDU1150-HDU2119

HDU2063-过山车

HDU1068-Girls and Boys

HDU1150Machine Schedule

你可能感兴趣的:(ACM,数学,算法分析与设计,C++)