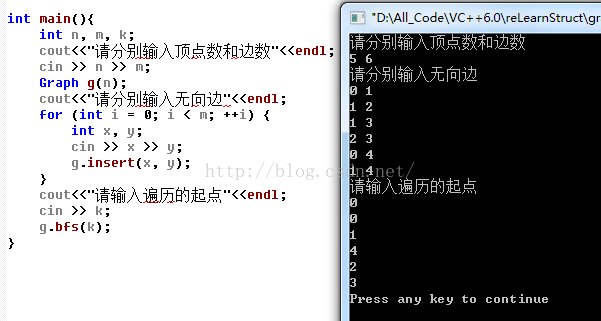
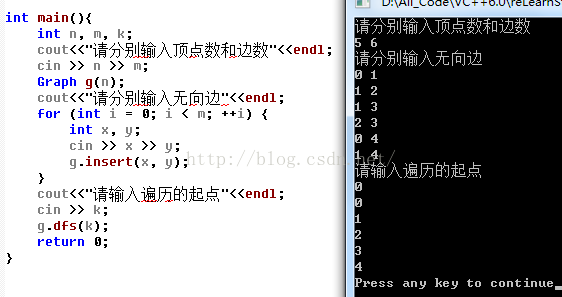
- 【Linux | 网络】socket编程 - 使用TCP实现服务端向客户端提供简单的服务
是阿建吖!
【网络】【Linux】网络linuxtcp/ip
目录一、Comm.hpp(公共数据)二、Log.hpp(日志)三、InetAddr.hpp(管理sockaddr_in相关信息)四、NoCopy.hpp(防拷贝)五、Lockguard.hpp(自动管理锁)六、Thread.hpp(封装线程)七、ThreadPool.hpp(线程池)八、dict.txt(配置文件、简单字典)九、Translate.hpp(提供翻译服务)十、Daemon.hpp(使
- Rust BSS段原理与实践解析
萧曵 丶
Rustrust开发语言后端内存模型
在Rust中,BSS段(BlockStartedbySymbol)是程序内存布局的关键部分,专门用于存储未初始化或零初始化的全局/静态变量。以下是从原理到实践的深入解析:一、BSS的核心特性零初始化BSS段中的所有变量在程序加载时自动初始化为0(或对应类型的零值:0、null、false等)。staticmutCOUNTER:usize=0;//实际存储在BSS段磁盘空间优化BSS段在可执行文件中
- OpenWebUI(8)源码学习-后端utils/telemetry追踪遥测模块
目录目录结构说明`constants.py`核心作用:主要功能:示例代码片段:`exporters.py`核心作用:主要类:`LazyBatchSpanProcessor`特点:技术亮点:`instrumentors.py`核心作用:插桩对象包括:钩子函数(Hooks):Instrumentor类:插桩流程:`setup.py`核心作用:主要功能:典型调用方式:✨总体架构与价值技术亮点总结✅开发建
- 一个实例用全创建型模式-优化(冗余消除)
科学的发展-只不过是读大自然写的代码
#c++ubuntu开发java算法前端
1.关联链接上一篇:一个实例用全创建型模式-CSDN博客目录:《一个实例讲完23种设计模式》2.内容当前:单件+抽象工厂+创建者+工厂方法+优化需求:坦克大战创建两种坦克坦克类型射程速度b7070米时/70公里b5050米时/50公里设计说明1.抽象工厂承担了创建部件的任务2.创建者承担了将部件组装的任务3.工厂方法类相当于创建者模式的导演,但是他是并未给用户提供选择创建者的接口。而是通过自己的多
- 西门子触摸屏与S7-200 SMART CPU的以太网通信
用户通过以下步骤可创建以太网接口的西门子触摸屏与S7-200SMARTCPU的以太网通信,在此以设备Smart1000IE为例。在WinCCflexible的主工作窗口中添加与CPU的连接与上文中RS485接口的西门子触摸屏类似,在此不再赘述。下面主要介绍连接参数设置及下载项目等。设置连接参数,首先选择Smart1000IE的接口为“以太网”,即触摸屏的以太网接口。选中该接口后,该接口的参数设置窗
- python汇率_用Python抓取汇率
抓取的是中行的数据:网址代码#-*-coding:utf-8-*-importreimporturllib.requesturl='http://www.boc.cn/sourcedb/whpj/index.html'#网址req=urllib.request.Request(url)response=urllib.request.urlopen(req)the_page=response.rea
- 计算机网络8832号答案,2013年4月份自考试计算机网络原理04741答案.doc
2013年4月份自考试计算机网络原理04741答案全国2013年4月高等教育自学考试计算机网络原理试题课程代码:04741请考生按规定用笔将所有试题的答案涂、写在答题纸上。选择题部分1.无线应用协议WAP的特点是A.支持手机上网B.不需要基站C.基于分组交换D.无固定路由器2.智能大厦及计算机网络的信息基础设施是A.通信自动化B.楼宇自动化C.结构化综合布线D.现代通信网络3.因特网工程特别任务组
- 数据结构实验解析(C++版)——实验一 复杂度分析
拯救三金
数据结构c++算法
目录一、实验例题例题1例题2二、实验原理与背景知识1、实验原理2、背景知识三、解题思路与算法1、解题思路2、算法四、代码实现例题1代码例题2代码五、实验结果分析与总结1、实验结果分析2、该实验与数据结构的联系一、实验例题例题1时间空间限制时间限制:1SEC空间限制:128MB问题描述分析以下代码:for(i=1;iusingnamespacestd;intmain(){longlongn;//输入
- vLLM 优化与调优:提升模型性能的关键策略
强哥之神
人工智能深度学习计算机视觉deepseek智能体vllm
在当今人工智能领域,大语言模型(LLM)的应用日益广泛,而优化和调优这些模型的性能成为了至关重要的任务。vLLM作为一种高效的推理引擎,提供了多种策略来提升模型的性能。本文将深入探讨vLLMV1的优化与调优策略,帮助读者更好地理解和应用这些技术。抢占式调度(Preemption)由于Transformer架构的自回归特性,有时键值缓存(KVcache)空间不足以处理所有批量请求。在这种情况下,vL
- S7-300 400与S7-200 SMART PLC以太网通讯(S7协议)资源文件介绍
滑展妙Bernice
S7-300400与S7-200SMARTPLC以太网通讯(S7协议)资源文件介绍【下载地址】S7-300400与S7-200SMARTPLC以太网通讯S7协议资源文件介绍本资源文件详细解析了S7-300400与S7-200SMARTPLC通过以太网进行通讯的技术细节,涵盖硬件连接、软件配置及通讯调试等关键环节。通过学习,您将掌握S7协议在PLC通讯中的实际应用,提升自动化与电气工程领域的专业技能
- Hive 事务表(ACID)问题梳理
文章目录问题描述分析原因什么是事务表概念事务表和普通内部表的区别相关配置事务表的适用场景注意事项设计原理与实现文件管理格式参考博客问题描述工作中需要使用pyspark读取Hive中的数据,但是发现可以获取metastore,外部表的数据可以读取,内部表数据有些表报错信息是:AnalysisException:org.apache.hadoop.hive.ql.metadata.HiveExcept
- 使用FinancialDatasets工具包进行财务数据分析
Zbb159
数据分析数据挖掘
##技术背景介绍在现代金融分析中,获取准确且及时的财务数据是至关重要的。FinancialDatasets提供了一个强大的API,可以获取超过16,000个股票的财务数据,时间跨度超过30年。通过与OpenAI的集成,我们能够创建智能化的财务分析助手,为投资者提供深度的市场洞察。##核心原理解析FinancialDatasets工具包通过RESTAPI接口访问财务数据,为每个公开交易的公司提供详细
- 【DeepSeek实战】24、LangGraph完全指南:从入门到实战,构建复杂AI工作流
无心水
人工智能LangGraph教程多Agent协作框架LangGraph实战案例复杂AI逻辑实现DeepSeek实战AI工作流开发
引言:为什么LangGraph是AI工作流的“下一代引擎”?当你需要构建一个能处理循环逻辑的AI客服系统——比如“用户投诉未解决时自动转人工,解决后发送满意度调查”——传统的链式框架(如LangChain基础链)会显得力不从心:它们难以实现分支跳转、状态保存和循环执行。而LangGraph的出现,正是为了解决这一痛点。LangGraph是LangChain团队推出的AI工作流引擎,专为复杂业务逻辑
- 让你的 AI 更聪明,这 7 个开源 MCP 项目不要错过
霍格沃兹测试开发学社
人工智能人工智能测试用例开发语言selenium驱动开发开源python
你还在用AI只是写写文档、改改代码?那你真的小看它了。现在,通过一套叫MCP(ModelControlPlane)的系统,AI不再只是“语言模型”,而是能直接操作网页、调用工具、自动化执行复杂任务的“智能助手”!今天整理了7个超实用的开源MCP项目,让你的AI立刻“开挂”。01|PagePublisherMCP:HTML页面一键上线还在发愁怎么把AI生成的HTML页部署上线?PagePublish
- 底层解剖ThreadLocal及其引发的内存泄漏问题
我认为可以!
jvmjavaThreadLocalThreadLocalMap内存泄漏
首先我们先明确一点,这里我们谈论的是比如线程池中的核心线程的情况,而不是普通的run完就销毁的线程。后面会继续说明为什么。关于ThreadLocal和ThreadLocalMap假设线程run()这样:publicvoidrun(){ThreadLocalthreadLocal=newThreadLocal()就只是new了一个这个工具类,其他的什么都没有发生。并不是每个线程都有一个它,不要混淆了
- 爬虫小结
Crescent_P
python小项目python数据分析
python爬虫小组作业上周布置了python的小组作业,每一组要求爬取老师指定的信息,本组抽到的题目如下:从中国银行网址:http://www.boc.cn/sourcedb/whpj/获取主要外汇(美元、欧元、英镑、加拿大元、澳大利亚元、日元、韩元、新台币、澳门元和港币)的牌价信息,计算出它们的每天平均价。要求把今年5月份每天平均价格保存到Excel文件中,每种外汇的数据保存在一个工作表中,并
- linux-权限管理
linux-权限管理一、权限的基本类型二、权限的表示方式1.字符形式(rwx)2.数字形式三、权限管理常用命令1.chmod2.chown3.chgrp四、隐藏权限1.lsattr2.chattr五、权限掩码六、特别权限位1.suid2.sgid3.StickyBit七、权限委托1.授权用户2.授权组里的用户3.使用命令别名授权八、ACL1.getfacl2.setfacl总结一、权限的基本类型读
- 怎样查询汇率实时行情,含汇率转换
大大的卷
接口python大数据前端
汇率转换接口支持多种货币之间的转换。只需简单的API调用,您就可以轻松获取最新的汇率信息。例如,您可以通过以下链接将人民币(CNY)转换为美元(USD):PHP请求案例:返回数据结果:{"code":1,"msg":"操作成功","data":{"from":"CNY","from_name":"人民币","to":"USD","to_name":"美元","exchange":"0.140583
- 【HCIA】TCP三次握手、4次断开详解
戏精亿点点菜
tcp/ip网络服务器
TCP(传输控制协议)是一种面向连接的、可靠的、基于字节流的传输层通信协议。在TCP/IP协议族中,TCP负责在两个网络实体之间建立、维护和终止连接。TCP连接的建立和终止分别通过三次握手和四次断开来完成。一、三次挥手TCP三次握手是建立TCP连接的过程,它确保了通信双方都准备好进行数据传输。过程如下:客户端->服务器:SYN,ISN=x服务器->客户端:SYN,ACK,ISN=y,ACK(x+1
- FPGA 47 ,MIG 内存接口生成器深度解析( FPGA 中的 MIG 技术 )
北城笑笑
fpga开发fpga
目录前言一、基础理论1.1MIG介绍1.2结构框架1.2.1主要模块①用户接口层(UserInterfaceLayer)②控制逻辑层(ControLogicLayer)③校准逻辑(CalibrationLogic)④初始化与时序控制(Initialization&TimingControl)⑤物理层接口(PHY–PhysicalLayer)⑥IO引脚驱动(引脚分配与IO配置:Pinout&IOSt
- Python爬虫实战:爬取ETF基金持仓变化
Python爬虫项目
python爬虫开发语言信息可视化数据分析
1.项目背景ETF(Exchange-TradedFund,交易型开放式指数基金)作为一种在交易所上市交易的基金,其持仓信息对于投资者具有重要参考价值。了解ETF的持仓变化,可以帮助投资者判断市场趋势和资金流向。本文将通过Python爬虫技术,自动化地获取ETF基金的持仓变化数据,进行存储和分析。2.技术选型与环境准备2.1技术选型编程语言:Python3.8+爬虫框架:Scrapy数据解析:Be
- 【数据结构】复杂度分析
目录一、算法1.基本概念2.描述方法3.算法效率二、算法的时间复杂度三、算法的空间复杂度一、算法1.基本概念通俗的讲,算法是解决问题的方法,比如在现实生活中一道菜谱,一个安装轮椅的操作指南等。严格的说,算法是对特定问题求解步骤的一种描述,是指令的有限序列。算法具有的基本特性有:(1)有穷性。一个算法必须总是在执行有穷步之后结束,且每一步都在有求时间内完成。(2)确定性。算法中的每一条指令必须有确切
- 【Python】(一)面试题和Py基础题
戏精亿点点菜
python开发语言
1.技术面试题(1)TCP与UDP的区别是什么?答:TCP(TransmissionControlProtocol,传输控制协议)提供的是面向连接,可靠的字节流服务。即客户和服务器交换数据前,必须现在双方之间建立一个TCP连接,之后才能传输数据。并且提供超时重发,丢弃重复数据,检验数据,流量控制等功能,保证数据能从一端传到另一端。UDP(UserDataProtocol,用户数据报协议)是一个简单
- linux-用户和组
2501_92004703
linux服务器运维
linux-用户和组前言一、用户管理1.用户账户类型2.主要命令2.1useradd2.2usedel2.3usermod2.4su3.查看用户登录信息3.1w3.2who3.3last3.4lastlog3.5lastb4.用户配置文件4.1/etc/passwd4.2/etc/shadow4.3/etc/login.defs5.手工新建用户二、组管理1.组分类2.组配置文件总结前言用户和组是进
- ResNet:深度卷积神经网络的里程碑
心想事“程”
小知识点cnn人工智能神经网络
一、引言在深度学习的发展历程中,深度卷积神经网络(CNN)不断演进,旨在提升对图像等数据的特征提取与分类能力。然而,随着网络层数的增加,传统CNN面临着梯度消失、梯度爆炸以及退化等棘手问题,训练变得愈发困难。2015年,由微软研究院提出的ResNet(ResidualNetworks,残差网络)横空出世,它以独特的残差学习思想,成功攻克了这些难题,在ImageNet竞赛中大放异彩,开创了深度神经网
- C语言指针进阶完全指南:从多级指针到函数指针的深度探索
给老吕螺丝
#C语言c语言开发语言
掌握指针基础后,你将开启C语言真正的力量之门。本文通过实战代码示例和内存布局图解,带你系统攻克指针进阶技术。一、指针核心回顾与进阶重点核心概念:指针本质:存储内存地址的变量间接访问:通过地址操作数据指针大小:64位系统固定8字节(与类型无关)进阶重点:多级指针:处理复杂间接关系动态内存管理:精准控制内存生命周期函数指针:实现代码抽象与回调复杂结构:构建链表等动态数据结构二、多级指针:指针的指针内存
- Go - 项目收藏
1、谷歌官方维护了一个基于go语言的开源项目列表:https://github.com/golang/go/wiki/Projects2、[知乎网]有哪些值得学习的Go语言开源项目?3、[知乎用户:hackstoic]看过awesome-go项目,汇总了很多go开源项目。但是awesome-go收集了太全了,而且每个项目没有描述。因此我自己根据go语言中文社区提供的资料,还有互联网企业架构设计中的
- 展锐平台(Android15)WLAN热点名称修改不生效问题分析
前言在展锐AndroidV项目开发中,需要修改softAp/P2P热点名称时,发现集成GMS后直接修改framework层代码无效。具体表现为:修改packages/modules/Wifi/WifiApConfigStore中的getDefaultApConfiguration方法编译烧录后修改不生效问题根源在于:Wi-Fi模块在AndroidS(12)及以上版本已纳入Mainline模块Mai
- 穿透硅层:模电数电如何重塑你的编程基因
还债大湿兄
模电数电
“不理解电子运动的程序员,永远在数字世界的表层流浪。”——吉恩·阿姆达尔(IBM360系统架构师)一、晶体管级视角:代码的物理载体1.CPU指令执行的硬件真相关键模电参数:阈值电压Vth:决定晶体管开关的电压临界点(典型值0.7V)跨导gm:栅压控制电流的能力(单位mS)米勒电容Cgd:限制开关速度的核心因素2.存储器操作的电子原理DRAM存储单元刷新过程://硬件级刷新伪代码voiddram_r
- 视觉算法之卷积神经网络
清风AI
深度学习算法详解及代码复现计算机视觉cnn神经网络深度学习python课程设计毕业设计
定义与特点卷积神经网络(ConvolutionalNeuralNetwork,CNN)是一种专为处理具有网格结构的数据而设计的深度学习模型。其独特的结构和功能使其在图像处理、语音识别等领域展现出卓越的性能:CNN的核心设计理念源于对生物视觉系统的模仿。通过模拟大脑皮层中视网膜和视觉皮层的层次化结构,CNN能够有效地捕捉图像中的局部特征并逐步抽象为高层语义信息。这种设计使得CNN特别擅长处理图像和音
- LeetCode[Math] - #66 Plus One
Cwind
javaLeetCode题解AlgorithmMath
原题链接:#66 Plus One
要求:
给定一个用数字数组表示的非负整数,如num1 = {1, 2, 3, 9}, num2 = {9, 9}等,给这个数加上1。
注意:
1. 数字的较高位存在数组的头上,即num1表示数字1239
2. 每一位(数组中的每个元素)的取值范围为0~9
难度:简单
分析:
题目比较简单,只须从数组
- JQuery中$.ajax()方法参数详解
AILIKES
JavaScriptjsonpjqueryAjaxjson
url: 要求为String类型的参数,(默认为当前页地址)发送请求的地址。
type: 要求为String类型的参数,请求方式(post或get)默认为get。注意其他http请求方法,例如put和 delete也可以使用,但仅部分浏览器支持。
timeout: 要求为Number类型的参数,设置请求超时时间(毫秒)。此设置将覆盖$.ajaxSetup()方法的全局
- JConsole & JVisualVM远程监视Webphere服务器JVM
Kai_Ge
JVisualVMJConsoleWebphere
JConsole是JDK里自带的一个工具,可以监测Java程序运行时所有对象的申请、释放等动作,将内存管理的所有信息进行统计、分析、可视化。我们可以根据这些信息判断程序是否有内存泄漏问题。
使用JConsole工具来分析WAS的JVM问题,需要进行相关的配置。
首先我们看WAS服务器端的配置.
1、登录was控制台https://10.4.119.18
- 自定义annotation
120153216
annotation
Java annotation 自定义注释@interface的用法 一、什么是注释
说起注释,得先提一提什么是元数据(metadata)。所谓元数据就是数据的数据。也就是说,元数据是描述数据的。就象数据表中的字段一样,每个字段描述了这个字段下的数据的含义。而J2SE5.0中提供的注释就是java源代码的元数据,也就是说注释是描述java源
- CentOS 5/6.X 使用 EPEL YUM源
2002wmj
centos
CentOS 6.X 安装使用EPEL YUM源1. 查看操作系统版本[root@node1 ~]# uname -a Linux node1.test.com 2.6.32-358.el6.x86_64 #1 SMP Fri Feb 22 00:31:26 UTC 2013 x86_64 x86_64 x86_64 GNU/Linux [root@node1 ~]#
- 在SQLSERVER中查找缺失和无用的索引SQL
357029540
SQL Server
--缺失的索引
SELECT avg_total_user_cost * avg_user_impact * ( user_scans + user_seeks ) AS PossibleImprovement ,
last_user_seek ,
- Spring3 MVC 笔记(二) —json+rest优化
7454103
Spring3 MVC
接上次的 spring mvc 注解的一些详细信息!
其实也是一些个人的学习笔记 呵呵!
- 替换“\”的时候报错Unexpected internal error near index 1 \ ^
adminjun
java“\替换”
发现还是有些东西没有刻子脑子里,,过段时间就没什么概念了,所以贴出来...以免再忘...
在拆分字符串时遇到通过 \ 来拆分,可是用所以想通过转义 \\ 来拆分的时候会报异常
public class Main {
/*
- POJ 1035 Spell checker(哈希表)
aijuans
暴力求解--哈希表
/*
题意:输入字典,然后输入单词,判断字典中是否出现过该单词,或者是否进行删除、添加、替换操作,如果是,则输出对应的字典中的单词
要求按照输入时候的排名输出
题解:建立两个哈希表。一个存储字典和输入字典中单词的排名,一个进行最后输出的判重
*/
#include <iostream>
//#define
using namespace std;
const int HASH =
- 通过原型实现javascript Array的去重、最大值和最小值
ayaoxinchao
JavaScriptarrayprototype
用原型函数(prototype)可以定义一些很方便的自定义函数,实现各种自定义功能。本次主要是实现了Array的去重、获取最大值和最小值。
实现代码如下:
<script type="text/javascript">
Array.prototype.unique = function() {
var a = {};
var le
- UIWebView实现https双向认证请求
bewithme
UIWebViewhttpsObjective-C
什么是HTTPS双向认证我已在先前的博文 ASIHTTPRequest实现https双向认证请求
中有讲述,不理解的读者可以先复习一下。本文是用UIWebView来实现对需要客户端证书验证的服务请求,网上有些文章中有涉及到此内容,但都只言片语,没有讲完全,更没有完整的代码,让人困扰不已。但是此知
- NoSQL数据库之Redis数据库管理(Redis高级应用之事务处理、持久化操作、pub_sub、虚拟内存)
bijian1013
redis数据库NoSQL
3.事务处理
Redis对事务的支持目前不比较简单。Redis只能保证一个client发起的事务中的命令可以连续的执行,而中间不会插入其他client的命令。当一个client在一个连接中发出multi命令时,这个连接会进入一个事务上下文,该连接后续的命令不会立即执行,而是先放到一个队列中,当执行exec命令时,redis会顺序的执行队列中
- 各数据库分页sql备忘
bingyingao
oraclesql分页
ORACLE
下面这个效率很低
SELECT * FROM ( SELECT A.*, ROWNUM RN FROM (SELECT * FROM IPAY_RCD_FS_RETURN order by id desc) A ) WHERE RN <20;
下面这个效率很高
SELECT A.*, ROWNUM RN FROM (SELECT * FROM IPAY_RCD_
- 【Scala七】Scala核心一:函数
bit1129
scala
1. 如果函数体只有一行代码,则可以不用写{},比如
def print(x: Int) = println(x)
一行上的多条语句用分号隔开,则只有第一句属于方法体,例如
def printWithValue(x: Int) : String= println(x); "ABC"
上面的代码报错,因为,printWithValue的方法
- 了解GHC的factorial编译过程
bookjovi
haskell
GHC相对其他主流语言的编译器或解释器还是比较复杂的,一部分原因是haskell本身的设计就不易于实现compiler,如lazy特性,static typed,类型推导等。
关于GHC的内部实现有篇文章说的挺好,这里,文中在RTS一节中详细说了haskell的concurrent实现,里面提到了green thread,如果熟悉Go语言的话就会发现,ghc的concurrent实现和Go有点类
- Java-Collections Framework学习与总结-LinkedHashMap
BrokenDreams
LinkedHashMap
前面总结了java.util.HashMap,了解了其内部由散列表实现,每个桶内是一个单向链表。那有没有双向链表的实现呢?双向链表的实现会具备什么特性呢?来看一下HashMap的一个子类——java.util.LinkedHashMap。
- 读《研磨设计模式》-代码笔记-抽象工厂模式-Abstract Factory
bylijinnan
abstract
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
package design.pattern;
/*
* Abstract Factory Pattern
* 抽象工厂模式的目的是:
* 通过在抽象工厂里面定义一组产品接口,方便地切换“产品簇”
* 这些接口是相关或者相依赖的
- 压暗面部高光
cherishLC
PS
方法一、压暗高光&重新着色
当皮肤很油又使用闪光灯时,很容易在面部形成高光区域。
下面讲一下我今天处理高光区域的心得:
皮肤可以分为纹理和色彩两个属性。其中纹理主要由亮度通道(Lab模式的L通道)决定,色彩则由a、b通道确定。
处理思路为在保持高光区域纹理的情况下,对高光区域着色。具体步骤为:降低高光区域的整体的亮度,再进行着色。
如果想简化步骤,可以只进行着色(参看下面的步骤1
- Java VisualVM监控远程JVM
crabdave
visualvm
Java VisualVM监控远程JVM
JDK1.6开始自带的VisualVM就是不错的监控工具.
这个工具就在JAVA_HOME\bin\目录下的jvisualvm.exe, 双击这个文件就能看到界面
通过JMX连接远程机器, 需要经过下面的配置:
1. 修改远程机器JDK配置文件 (我这里远程机器是linux).
- Saiku去掉登录模块
daizj
saiku登录olapBI
1、修改applicationContext-saiku-webapp.xml
<security:intercept-url pattern="/rest/**" access="IS_AUTHENTICATED_ANONYMOUSLY" />
<security:intercept-url pattern=&qu
- 浅析 Flex中的Focus
dsjt
htmlFlexFlash
关键字:focus、 setFocus、 IFocusManager、KeyboardEvent
焦点、设置焦点、获得焦点、键盘事件
一、无焦点的困扰——组件监听不到键盘事件
原因:只有获得焦点的组件(确切说是InteractiveObject)才能监听到键盘事件的目标阶段;键盘事件(flash.events.KeyboardEvent)参与冒泡阶段,所以焦点组件的父项(以及它爸
- Yii全局函数使用
dcj3sjt126com
yii
由于YII致力于完美的整合第三方库,它并没有定义任何全局函数。yii中的每一个应用都需要全类别和对象范围。例如,Yii::app()->user;Yii::app()->params['name'];等等。我们可以自行设定全局函数,使得代码看起来更加简洁易用。(原文地址)
我们可以保存在globals.php在protected目录下。然后,在入口脚本index.php的,我们包括在
- 设计模式之单例模式二(解决无序写入的问题)
come_for_dream
单例模式volatile乱序执行双重检验锁
在上篇文章中我们使用了双重检验锁的方式避免懒汉式单例模式下由于多线程造成的实例被多次创建的问题,但是因为由于JVM为了使得处理器内部的运算单元能充分利用,处理器可能会对输入代码进行乱序执行(Out Of Order Execute)优化,处理器会在计算之后将乱序执行的结果进行重组,保证该
- 程序员从初级到高级的蜕变
gcq511120594
框架工作PHPandroidhtml5
软件开发是一个奇怪的行业,市场远远供不应求。这是一个已经存在多年的问题,而且随着时间的流逝,愈演愈烈。
我们严重缺乏能够满足需求的人才。这个行业相当年轻。大多数软件项目是失败的。几乎所有的项目都会超出预算。我们解决问题的最佳指导方针可以归结为——“用一些通用方法去解决问题,当然这些方法常常不管用,于是,唯一能做的就是不断地尝试,逐个看看是否奏效”。
现在我们把淫浸代码时间超过3年的开发人员称为
- Reverse Linked List
hcx2013
list
Reverse a singly linked list.
/**
* Definition for singly-linked list.
* public class ListNode {
* int val;
* ListNode next;
* ListNode(int x) { val = x; }
* }
*/
p
- Spring4.1新特性——数据库集成测试
jinnianshilongnian
spring 4.1
目录
Spring4.1新特性——综述
Spring4.1新特性——Spring核心部分及其他
Spring4.1新特性——Spring缓存框架增强
Spring4.1新特性——异步调用和事件机制的异常处理
Spring4.1新特性——数据库集成测试脚本初始化
Spring4.1新特性——Spring MVC增强
Spring4.1新特性——页面自动化测试框架Spring MVC T
- C# Ajax上传图片同时生成微缩图(附Demo)
liyonghui160com
1.Ajax无刷新上传图片,详情请阅我的这篇文章。(jquery + c# ashx)
2.C#位图处理 System.Drawing。
3.最新demo支持IE7,IE8,Fir
- Java list三种遍历方法性能比较
pda158
java
从c/c++语言转向java开发,学习java语言list遍历的三种方法,顺便测试各种遍历方法的性能,测试方法为在ArrayList中插入1千万条记录,然后遍历ArrayList,发现了一个奇怪的现象,测试代码例如以下:
package com.hisense.tiger.list;
import java.util.ArrayList;
import java.util.Iterator;
- 300个涵盖IT各方面的免费资源(上)——商业与市场篇
shoothao
seo商业与市场IT资源免费资源
A.网站模板+logo+服务器主机+发票生成
HTML5 UP:响应式的HTML5和CSS3网站模板。
Bootswatch:免费的Bootstrap主题。
Templated:收集了845个免费的CSS和HTML5网站模板。
Wordpress.org|Wordpress.com:可免费创建你的新网站。
Strikingly:关注领域中免费无限的移动优
- localStorage、sessionStorage
uule
localStorage
W3School 例子
HTML5 提供了两种在客户端存储数据的新方法:
localStorage - 没有时间限制的数据存储
sessionStorage - 针对一个 session 的数据存储
之前,这些都是由 cookie 完成的。但是 cookie 不适合大量数据的存储,因为它们由每个对服务器的请求来传递,这使得 cookie 速度很慢而且效率也不