【golang】leetcode中级-多数元素

第一题 多数元素

题目

简单的思路

简单的想法是使用哈希表或者排序完成

遍历一次数组，将每个数出现的次数存入哈希表，当次数大于n/2时返回即可
然而该方案空间复杂度为O（n），不符合要求O（1）

将数组排成有序数组，计算每个元素出现的次数，当次数大于n/2时返回即可
然而排序的时间复杂度为O（nlogn），大于题目要求O（n）

随机法

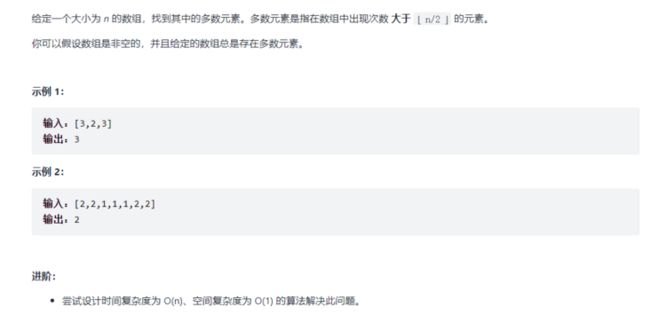
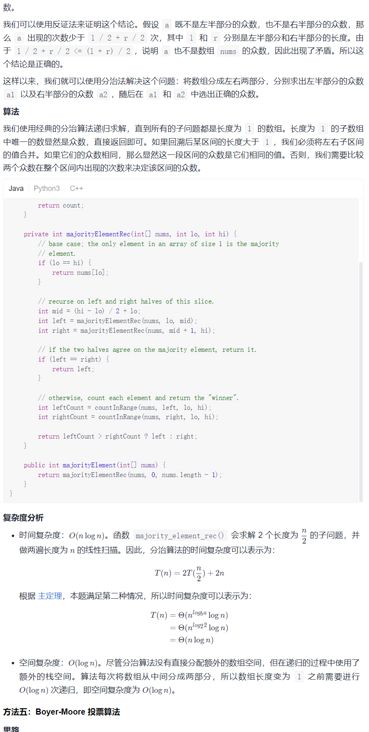
分治法

代码.3

func majorityElement(nums []int) int {
    return majorityElementRec(nums, 0, len(nums) - 1)
}

//判断众数
func countInRange(nums []int,  target int,lo int,  hi int) int{
    count := 0
    for  i := lo; i <= hi; i++ {
        if nums[i] == target {
            count++
        }
    }
    return count
}

//二分查找
func majorityElementRec( nums []int,  lo int,  hi int) int{
    //左边界等于右边界，只有一个元素，即为该数组众数
    if lo == hi {
        return nums[lo]
    }
    mid := (lo + hi) / 2
    //左右众数
    leftMajority := majorityElementRec(nums, lo, mid)
    rightMajority := majorityElementRec(nums, mid + 1, hi)
    //返回真正的众数
    if countInRange(nums, leftMajority, lo, hi) > (hi - lo + 1) / 2 {
        return leftMajority
    }
    if countInRange(nums, rightMajority, lo, hi) > (hi - lo + 1) / 2 {
        return rightMajority
    }
    return -1
}

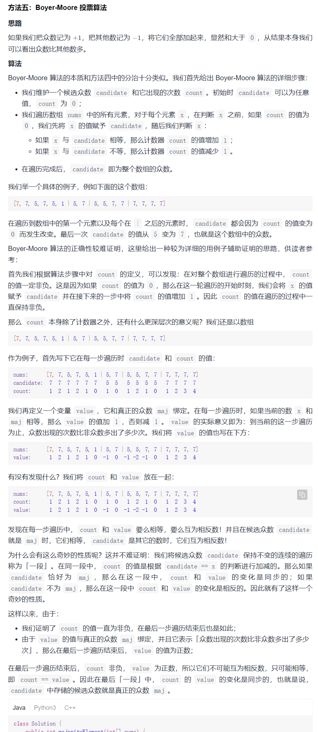
摩尔投票法

代码

func majorityElement(nums []int) int {
    //初始化
    candidate := -1
    count := 0

    for _, num :=range nums {
        //如果相等，计数器加一
        if num == candidate {
            count++
        }else {
            //否则计数器减一，消去一个非众数
            count--
        }
        //初始化候选众数
        if count < 0 {
            candidate = num
            count = 1
        }
    }
    return candidate
}

效果

复杂度分析

时间复杂度：O(n)。Boyer-Moore 算法只对数组进行了一次遍历。

空间复杂度：O(1)。Boyer-Moore 算法只需要常数级别的额外空间。