用Python画科赫雪花
文章目录
-
- 科赫曲线
- 飘雪
- 三维飘雪
科赫曲线
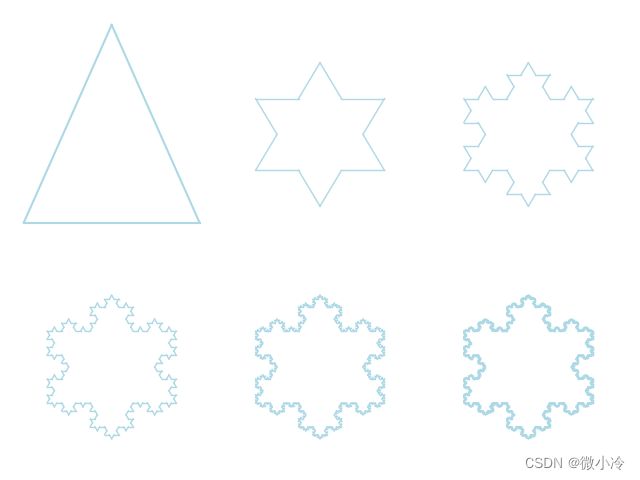
科赫曲线因为十分像雪花,所以被成为雪花曲线,生成方式十分简单,总共分两步
- 画一个正三角形
- 将正三角形的每个边三等分,然后以中间的那份为边,再画出个三角形。
- 重复第二步。
那么难点无非是三等分后如何新画一个三角形,更进一步,新三角形的那个新顶点在哪里?
设已知两点 ( x 1 , y 1 ) , ( x 2 , y 2 ) (x_1,y_1),(x_2,y_2) (x1,y1),(x2,y2),设正三角形的第三点为 ( x , y ) (x,y) (x,y),则将坐标系平移到 ( x 1 , y 1 ) (x_1,y_1) (x1,y1)后对 ( x 2 , y 2 ) (x_2,y_2) (x2,y2)旋转60°即可。
[ x y ] = [ x 1 y 1 ] + [ cos π 3 − sin π 3 sin π 3 cos π 3 ] ⋅ [ x 2 − x 1 y 2 − y 1 ] = [ x 1 + 1 2 ( x 2 − x 1 ) − 3 2 ( y 2 − y 1 ) y 1 + 3 2 ( x 2 − x 1 ) + 1 2 ( y 2 − y 1 ) ] = [ 1 2 ( x 2 + x 1 ) − 3 2 ( y 2 − y 1 ) 1 2 ( y 2 + y 1 ) + 3 2 ( x 2 − x 1 ) ] \begin{aligned} \begin{bmatrix}x\\ y\end{bmatrix}&= \begin{bmatrix}x_1\\ y_1\end{bmatrix}+ \begin{bmatrix}\cos\frac{\pi}{3} &-\sin\frac{\pi}{3}\\ \sin\frac{\pi}{3}&\cos\frac{\pi}{3}\end{bmatrix}\cdot \begin{bmatrix}x_2-x_1\\ y_2-y_1\end{bmatrix}\\ &=\begin{bmatrix} x_1 + \frac{1}{2}(x_2-x_1)-\frac{\sqrt 3}{2}(y_2-y_1)\\ y_1 + \frac{\sqrt{3}}{2}(x_2-x_1)+\frac{1}{2}(y_2-y_1)\end{bmatrix}\\ &=\begin{bmatrix} \frac{1}{2}(x_2+x_1)-\frac{\sqrt 3}{2}(y_2-y_1)\\ \frac{1}{2}(y_2+y_1)+\frac{\sqrt{3}}{2}(x_2-x_1)\end{bmatrix} \end{aligned} [xy]=[x1y1]+[cos3πsin3π−sin3πcos3π]⋅[x2−x1y2−y1]=[x1+21(x2−x1)−23(y2−y1)y1+23(x2−x1)+21(y2−y1)]=[21(x2+x1)−23(y2−y1)21(y2+y1)+23(x2−x1)]
写成Python就是
import numpy as np
import matplotlib.pyplot as plt
from numpy.random import rand,randint
# n>=1,生成科赫雪花的方法
def Koch(L,n=1):
if n<1 : return L
newL = [] #(x,y)的列表
k = np.sqrt(3)/2
for i in range(len(L)-1):
d = (L[i+1]-L[i])/3
mid = (L[i]+L[i+1])/2
xy = [mid[0]-k*d[1], mid[1]+k*d[0]]
newL += [L[i], L[i]+d, xy, L[i]+d*2]
newL.append(L[-1])
return np.array(Koch(newL,n-1))
接下来,设置初始三角形,并做一个绘图函数
L0 = np.array([[0,0],
[0.5,np.sqrt(3)/2], [1,0], [0,0] ])
fig = plt.figure()
for i in range(6):
L = Koch(L0, i)
ax = fig.add_subplot(2,3,i+1)
ax.plot(*L.T, c='lightblue', lw=1)
plt.axis('off') # 隐藏坐标轴
plt.show()
从而得到
飘雪
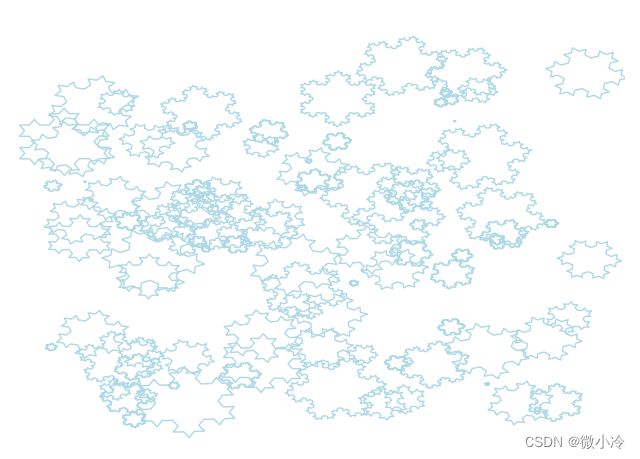
既然有许多个雪花,那么可能就会有那种飘雪的感觉,这里只需用多个随机数生成多组科赫曲线
#n为雪花数量,low,high为最低和最高koch雪花阶数
def RandKoch(n,low,high):
randKochs = []
rMax = np.sqrt(1/n)
for _ in range(n):
cx,cy,t0 = rand(3)
r = rand()*rMax
L0 = [np.array([np.cos(t),np.sin(t)])*r+[cx,cy]
for t in (t0-np.arange(4)*np.pi*2/3)]
randKochs.append(Koch(L0,randint(low,high)))
return randKochs
Ls = RandKoch(100, 2,4)
for L in Ls:
plt.plot(*L.T, c='lightblue', lw=1)
plt.axis('off')
plt.show()
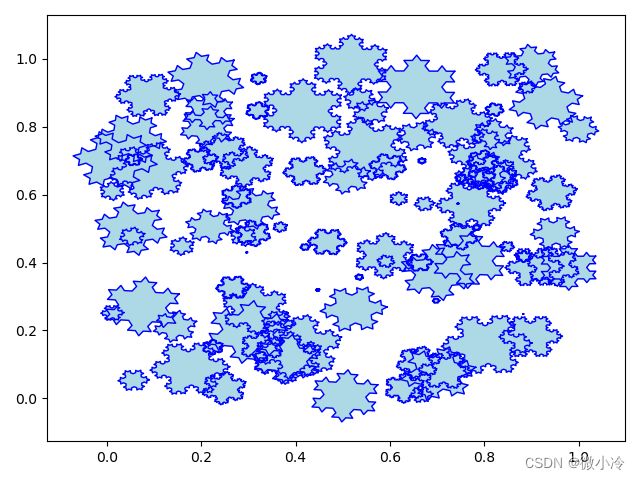
plt.figure(2)
for L in Ls:
plt.plot(*L.T, c='blue', lw=1)
plt.fill(*L.T, c='lightblue', lw=1)
plt.show()
其中,plt.fill可以填充绘制内容,其结果为
 |
 |
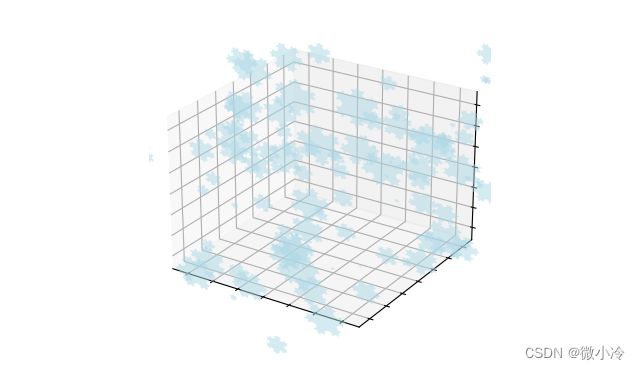
三维飘雪
如果想要那种飘雪的感觉,至少得有个3D的图,这很简单,只要加个三维的坐标就可以了。
#导入PolyCollection绘制实心的3D图形
from mpl_toolkits.mplot3d.art3d import PolyCollection
ax = plt.subplot(projection='3d')
p3d = PolyCollection(Ls,facecolors=np.repeat('lightblue',len(Ls)),alpha=0.5)
ax.add_collection3d(p3d,zs=rand(len(Ls)),zdir='y')
# 取消坐标轴的刻度值
for axset in [ax.set_xticks, ax.set_xticks, ax.set_xticks]:
axset(np.arange(10)/10, ['']*10)
plt.show()