【深入浅出密码学】离散对数
群相关知识点
离散对数相关
对于解决 α x = β \alpha^{x} =\beta αx=β,我们不断地选取合适地 x x x,计算 a x a^x ax,直到找到满足这个等式的 x x x,时间复杂度 O ( ∣ G ∣ ) O(|G|) O(∣G∣).
Baby-Step Giant-Step
对于解决 α x = β m o d ( p ) \alpha^x=\beta\ mod\ (p) αx=β mod (p),我们将 x x x表示为 x = x g ∗ p + x b x=x_g*\sqrt{p}+x_b x=xg∗p+xb.这里的 x b , x g ∈ [ 0 , p ] x_b,x_g \in[0,\sqrt{p}] xb,xg∈[0,p]
现将问题转化为,求解 a x g ∗ p + x b = β m o d ( p ) a^{x_g*\sqrt{p}+x_b}=\beta \ mod(p) axg∗p+xb=β mod(p),此时我们只需要寻找满足等式的 ( x g , x b ) (x_g,x_b) (xg,xb),
将等式等价变换成 a x g ∗ p = β ∗ a − x b m o d ( p ) a^{x_g*\sqrt{p}}=\beta*a^{-x_b}\ mod(p) axg∗p=β∗a−xb mod(p).
在Baby-Step阶段,我们枚举 x b x_b xb的取值,并采用哈希表或者字典等数据结构记录 ( x b , β ∗ a − x b ) (x_b,\beta*a^{-x_b}) (xb,β∗a−xb).
在Giant-Step阶段,我们枚举 x g x_g xg,计算 a x g ∗ p a^{x_g*\sqrt{p}} axg∗p,并且在哈希表当中查找,直到出现相等的值。
这个算法的时间和空间复杂度都是 O ( P ) O(\sqrt{P}) O(P)
Pollards’s Rho
在整数分解当中的应用:
问题描述:
设大整数为 n n n,我们需要找到 n n n的一个因子 p p p
我们设想存在两个整数 x , x ′ ∈ Z n x,x^{'}\in Z_n x,x′∈Zn,满足 x = x ′ m o d ( p ) x=x^{'}\ mod\ (p) x=x′ mod (p),这样的话就有 p ∣ ( x − x ′ ) p|(x-x^{'}) p∣(x−x′),又因为 p ∣ n p|n p∣n,那么 g c d ( x − x ′ , n ) gcd(x-x',n) gcd(x−x′,n)一定是 n n n的非平凡因子。问题归结为:如何找到满足条件的 x , x ′ x,x' x,x′
解释: x − x ′ = k ∗ p , n = k ∗ p , 那么 g c d ( x − x ′ , n ) = p x-x'=k*p,n=k*p,那么gcd(x-x',n)=p x−x′=k∗p,n=k∗p,那么gcd(x−x′,n)=p
我们选定一个随机的序列 X ⊂ Z n X \subset Z_n X⊂Zn, x = { x 1 , x 2 , . . , x l } x=\{x_1,x_2,..,x_l\} x={x1,x2,..,xl},这里的 l = n 1 / 4 l=n^{1/4} l=n1/4,我们从中随机选择两个数计算 g c d ( x − x ′ , n ) gcd(x-x',n) gcd(x−x′,n),我们需要进行 C l 2 C_l^2 Cl2次 g c d gcd gcd运算,这时的计算效率并不高,需要后续的优化。
这里我们选定一个伪随机数生成器,让我们每次的选择看上去好像是随机的。
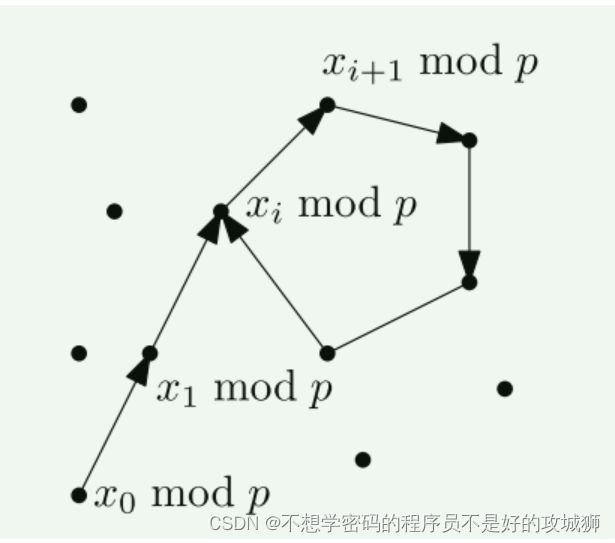
f ( x ) = x 2 + 1 m o d n f(x)=x^2+1\ mod \ n f(x)=x2+1 mod n,随机选择一个 x 0 ∈ Z n x_0\in Z_n x0∈Zn,然后计算 x 1 = f ( x 0 ) , x 2 = f ( x 1 ) , . . x i = f ( x i − 1 ) x_1=f(x_0),x_2=f(x_1),..x_i=f(x_{i-1}) x1=f(x0),x2=f(x1),..xi=f(xi−1).
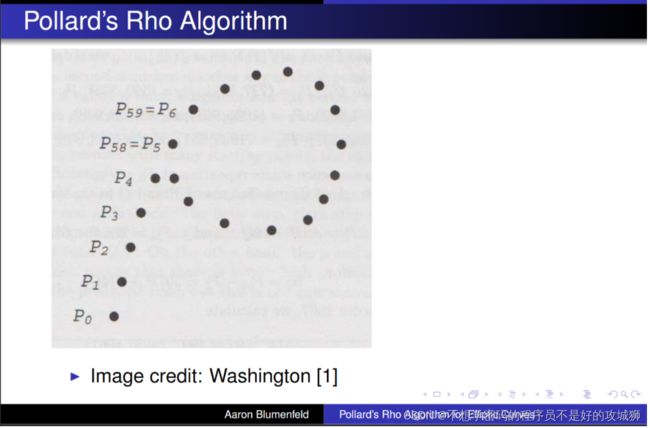
我们画出 Z p Z_p Zp的图像
1. L e t y 0 = x 0 1.Let\ y_0=x_0 1.Let y0=x0
2. F o r i = 1 , 2 , . . . 2.For\ i=1,2,... 2.For i=1,2,...
( a ) x i = f ( x i − 1 ) (a)\ x_i=f(x_{i-1}) (a) xi=f(xi−1)
( b ) y i = f ( f ( y i − 1 ) ) (b)\ y_i=f(f(y_{i-1})) (b) yi=f(f(yi−1))
( c ) I F g c d ( x i − y i , n ) ≠ 1 r e t u r n t h i s d i s c o v e r e d f a c t o r (c)\ IF\ gcd(x_i-y_i,n)\neq\ 1\ return\ this\ discovered\ factor (c) IF gcd(xi−yi,n)= 1 return this discovered factor
在离散对数当中的应用:
def pollard_rho(g, y, p):
q = (p-1) // 2
def new_xab(x, a, b, g, y, p, q):
subset = x % 3
if subset == 0:
return ((x*x) % p, (a*2) % q, (b*2) % q)
if subset == 1:
return ((x*g) % p, (a+1) % q, b )
if subset == 2:
return ((x*y) % p, a , (b+1) % q)
x, a, b = 1, 0, 0
X, A, B = x, a, b
for i in range(1, p):
x, a, b = new_xab(x, a, b, g, y, p, q)
X, A, B = new_xab(X, A, B, g, y, p, q)
X, A, B = new_xab(X, A, B, g, y, p, q)
if x == X:
break
res = ((a - A) * pow(B - b, -1, q)) % q
if pow(g, res, p) == y:
return res
if pow(g, res + q, p) == y:
return res + q
return None
g =
y =
p =
x = pollard_rho(g, y, p)
print(x)
print(pow(g, x, p) == y)
Pollards’s Hellman
适用条件:p是一个素数,p-1包含的质因子较小并且比较少
对于 a x = b m o d ( p ) a^x=b\ mod\ (p) ax=b mod (p),记其原根为 g g g,记 a = g a i , b = g b i a=g^{a_i},b=g^{b_i} a=gai,b=gbi,此时得到 g a i x = g b i m o d ( p ) g^{a_ix}=g^{b_i}\ mod\ (p) gaix=gbi mod (p),所以 a i x = b i m o d ( p − 1 ) a_ix=b_i\ mod\ (p-1) aix=bi mod (p−1),如果我们得到 a i , b i a_i,b_i ai,bi那么 x x x易得。
#Sage Code 1
p =
a =
b =
gx =
gy =
px =
py =
E = EllipticCurve(GF(p), [a, b])
G = E(gx, gy)
n = E.order()
QA = E(px, py)
factors = list(factor(n))
m = 1
moduli = []
remainders = []
print(f"[+] Running Pohlig Hellman")
print(factors)
for i, j in factors:
if i > 10**9:
print(i)
break
mod = i**j
g2 = G*(n//mod)
q2 = QA*(n//mod)
r = discrete_log(q2, g2, operation='+')
remainders.append(r)
moduli.append(mod)
m *= mod
r = crt(remainders, moduli)
print(r)