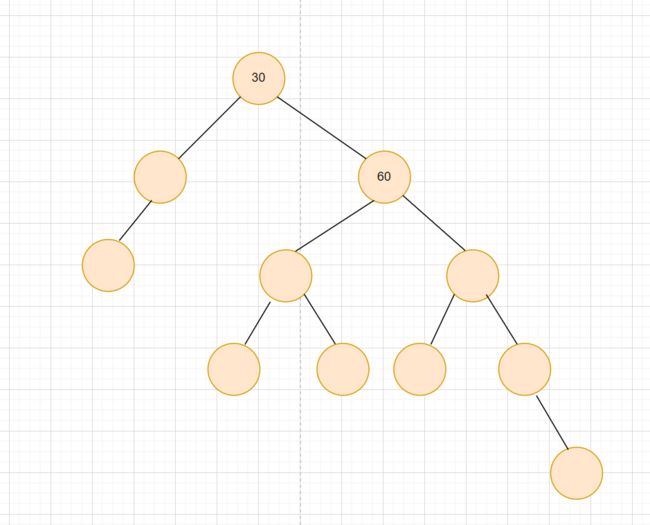
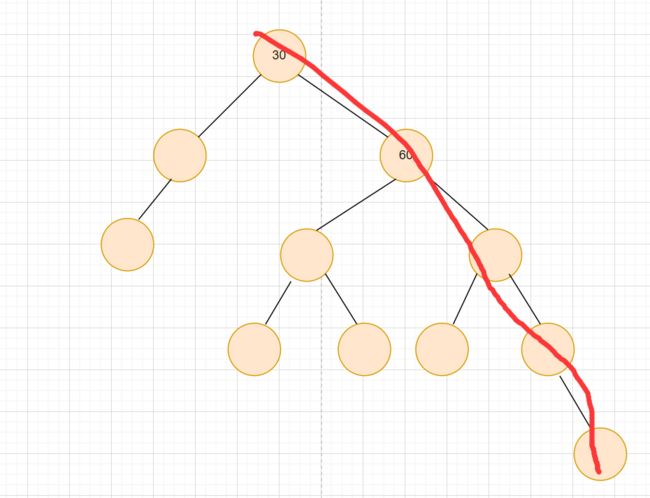
- C++数据结构 数组加链表
哈儿1号
数据结构C++c++
#includeusingnamespacestd;//对于线性表有必要执行的操作://创建,撤销//确定线性表是否为空//确定线性表的长度//按索引查找一个元素//按元素查找索引。//按索引删除元素//按索引插入元素//从左到右的顺序输出线性表元素//这是个老祖宗templateclasslinearList{public:virtual~linearList(){};virtualboolem
- c++基础
冰凉的保温瓶
c++开发c++
extern关键字https://www.cnblogs.com/honernan/p/13431431.html定义和声明在介绍extern之前,我们需要了解一下变量的声明和定义。变量的声明指向程序表名变量的类型和名字,即使得名字为程序所知,一个文件如果想使用别处定义的名字则必须包含对那个名字的声明。而变量的定义指申请存储空间,并将其与变量名相关联,除此之外,还可以为变量指定初始值。在程序中变量
- Java、Python、PHP、Go:网站开发语言全维度对比与选择指南
生信天地
开发语言javapython
在数字化转型浪潮中,网站开发技术的选择直接影响着项目的成败。Java、Python、PHP、Go四门语言凭借各自特性,在不同场景中展现出独特的竞争力。根据Statista2024年开发者调查报告,Java仍以34%的企业级应用占比位居榜首,而Go以27%的增速成为云原生领域新宠。本文基于技术特性、行业案例及发展趋势,深度解析四大语言的优劣势,助您做出精准技术选型。一、性能与并发能力:高负载场景的生
- C++核心编程手册
易方达蓝筹
Cor计算机网络c++开发语言后端
C++核心编程本阶段主要针对C++面向对象编程技术做详细讲解,探讨C++中的核心和精髓。1内存分区模型C++程序在执行时,将内存大方向划分为4个区域代码区:存放函数体的二进制代码,由操作系统进行管理的全局区:存放全局变量和静态变量以及常量栈区:由编译器自动分配释放,存放函数的参数值,局部变量等堆区:由程序员分配和释放,若程序员不释放,程序结束时由操作系统回收内存四区意义:不同区域存放的数据,赋予不
- Springboot乐动健身房管理系统6xl64计算机毕业设计-课程设计-期末作业-毕设程序代做
含宇网络
springbootjava后端
Springboot乐动健身房管理系统6xl64计算机毕业设计-课程设计-期末作业-毕设程序代做【免费赠送源码】Springboot乐动健身房管理系统6xl64计算机毕业设计-课程设计-期末作业-毕设程序代做本源码技术栈:项目架构:B/S架构开发语言:Java语言开发软件:ideaeclipse前端技术:Layui、HTML、CSS、JS、JQuery等技术后端技术:JAVA运行环境:Win10、
- C++智能指针:从内存裸奔到安全驾驶(附保姆级代码示例)
灰灰的C旅程
随时随地C++C/C++c++安全开发语言
大家好呀,我是灰灰,上期咱们聊完引用,不少小伙伴在评论区哭诉内存泄漏的惨痛经历。今天咱们就来解锁C++的"自动驾驶"神器——智能指针!从此告别new/delete的手动挡时代,系好安全带,发车啦!一、智能指针是什么?为什么需要它?1.1手动管理内存的痛void作死示例(){int*裸指针=newint[10086];//申请//...一顿操作猛如虎...if(rand()%2)return;//5
- C或C++中实现数据结构课程中的链表、数组、树和图案例
小弟有话说1.0
数据结构c语言c++
1.双向链表(DoublyLinkedList)-----支持双向遍历。C++实现#includestructNode{intdata;Node*prev;Node*next;};classDoublyLinkedList{private:Node*head;public:DoublyLinkedList():head(nullptr){}//在链表末尾插入节点voidappend(intdata
- C++徒手搓国密SM算法!从青铜到王者の硬核修炼手册
skyksksksksks
C++个人杂记物联网c++算法开发语言国密算法国密c语言
当代码遇上中国密码标准(掏出祖传键盘)家人们谁懂啊!今天我们要用C++光膀子手撕国密四件套!不靠任何第三方库,就像用树枝钻木取火一样原始硬核!先上全家桶参数对比表(建议截图保存):算法杀伤力密钥长度核心装备必杀技SM2非对称核弹256bit椭圆曲线方程数字签名+密钥交换二合一SM3哈希冲击波256bit压缩函数套娃数据粉碎成量子态SM4对称加特林128bitFeistel网络32轮位操作旋风斩SM
- C++ 预处理器
阳光向日葵向阳
c++开发语言
预处理器是一些指令,指示编译器在实际编译之前所需完成的预处理。所有的预处理器指令都是以井号(#)开头,只有空格字符可以出现在预处理指令之前。预处理指令不是C++语句,所以它们不会以分号(;)结尾。我们已经看到,之前所有的实例中都有#include指令。这个宏用于把头文件包含到源文件中。C++还支持很多预处理指令,比如#include、#define、#if、#else、#line等,让我们一起看看
- 2024年第十五届蓝桥杯大赛软件类省赛C/C++大学B组真题——好数
小黄Calm
蓝桥杯c语言c++
题目:一个整数如果按从低位到高位的顺序,奇数位(个位、百位、万位···)上的数字是奇数,偶数位(十位、千位、十万位···)上的数字是偶数,我们就称之为“好数”。给定一个正整数N,请计算从1到N一共有多少个好数。由于题目中最高只有七位数,可以通过多少位数来分别判断:#includeintmain(){intnum1,count=0,n=0;scanf("%d",&num1);for(intnum=1
- 宝石组合 第十五届蓝桥杯大赛软件赛省赛C/C++ 大学 B 组
Geometry Fu
蓝桥杯蓝桥杯c语言c++
宝石组合题目来源第十五届蓝桥杯大赛软件赛省赛C/C++大学B组原题链接蓝桥杯宝石组合https://www.lanqiao.cn/problems/19711/learning/问题描述P10426[蓝桥杯2024省B]宝石组合题目描述在一个神秘的森林里,住着一个小精灵名叫小蓝。有一天,他偶然发现了一个隐藏在树洞里的宝藏,里面装满了闪烁着美丽光芒的宝石。这些宝石都有着不同的颜色和形状,但最引人注目
- 用VSCode做前端开发
北子ALF
杂谈vscodeide编辑器
vscode写前端和记markdown还是很好用的,虽然在C++,Java和Python大型项目开发的体验不如vs,idea和pycharm自动生成html骨架打个感叹号预览网页:liveserver插件
- 好数 第十五届蓝桥杯大赛软件赛省赛C/C++ 大学 B 组
Geometry Fu
蓝桥杯蓝桥杯c语言c++
好数题目来源第十五届蓝桥杯大赛软件赛省赛C/C++大学B组原题链接蓝桥杯好数问题描述P10424[蓝桥杯2024省B]好数题目描述一个整数如果按从低位到高位的顺序,奇数位(个位、百位、万位……)上的数字是奇数,偶数位(十位、千位、十万位……)上的数字是偶数,我们就称之为“好数”。给定一个正整数NNN,请计算从111到NNN一共有多少个好数。输入格式一个整数NNN。输出格式一个整数代表答案。输入输出
- JAVA毕业设计河南口腔医疗机构线上服务系统计算机源码+lw文档+系统+调试部署+数据库
煦洋cxsj985
javajvm开发语言
JAVA毕业设计河南口腔医疗机构线上服务系统计算机源码+lw文档+系统+调试部署+数据库JAVA毕业设计河南口腔医疗机构线上服务系统计算机源码+lw文档+系统+调试部署+数据库本源码技术栈:项目架构:B/S架构开发语言:Java语言开发软件:ideaeclipse前端技术:Layui、HTML、CSS、JS、JQuery等技术后端技术:JAVA运行环境:Win10、JDK1.8数据库:MySQL5
- 详解c++的编译过程,如何从源文件到可执行文件到
飞鼠_
C++c++开发语言
本节详细介绍c++的编译过程,c++从代码到可执行文件有四个阶段:预处理运行以#好开头的代码,引入头文件,做预处理定义常量等编译对代码进行优化,进行词法与语法的分析,生成与平台无关的中间表示,再将中间代码转换为目标平台的汇编代码。汇编将汇编代码转换为机器码(二进制格式)。链接将目标文件中的未定义符号(如printf)与库文件中的定义匹配。预处理我们可以使用g++-Emain.cpp-omain.i
- QT中的宏
m0_55576290
qtqt开发语言
Q_UNUSED(event);是Qt提供的一个宏,用于标记某个变量或参数在当前作用域中未被使用。它的主要作用是避免编译器发出“未使用变量”的警告。背景在C++中,如果一个函数参数或变量在代码中没有被使用,编译器会发出警告,例如:voidsomeFunction(intunusedParam){//参数unusedParam没有被使用}编译器可能会报出类似以下警告:warning:unusedpa
- protubuf序列化和反序列化原理
要好好养胃
c++11c++开发语言算法linux服务器
文章目录protubuf序列化和反序列化原理序列化:将数据结构或者对象转换成二进制字节流判断每个字段是否有设置值,有值才进行编码根据字段表示号与实际类型将字段值通过不容的编码方式进行编码将编码后的数据块按照字段类型采用不同的存储方式封装成二进制数据流反序列化:将二进制字节流转换回数据结构或者对象解析读取的二进制字节数据流将解析出来的数据存储到c++、java等对应的数据结构中varint编码:整形
- 7.7:C++的 STL迭代器的分类和使用!(课程共7300字,8个代码举例)
小兔子平安
C++完整学习全解答c++开发语言
例子1:使用输入迭代器读取文件中的数据例子2:使用输出迭代器将数据写入文件例子3:使用双向迭代器反转容器中的元素例子4:使用随机访问迭代器进行二分查找例子1:使用输入迭代器读取文件中的数据下面的代码演示了如何使用输入迭代器从文件中读取数据,并计算其平均值。#include#include#include#includeintmain(){std::ifstreamfile("data.txt");
- C++中map和set的详解
黑猫Teng
c++算法开发语言
C++中map和set的介绍与使用在C++编程中,map和set是标准模板库(STL)中两种非常重要的关联容器。它们基于平衡二叉搜索树(通常是红黑树)的数据结构来实现,提供了高效的数据存储和检索功能。本文将详细介绍map和set的特点、用法以及一些常见的操作示例。一、map的介绍与使用1.map的基本概念map是一个键值对容器,其中每个键都是唯一的,且按照升序排序。map的内部结构是红黑树,这使得
- C++内存管理秘籍:深入解析与实战代码示例
萱萱199504
c++java开发语言
C++内存管理秘籍:深入解析与实战代码示例一、内存管理的基本概念二、内存泄漏与野指针三、智能指针:现代C++的内存管理利器四、实战代码示例示例1:传统动态内存管理示例2:使用`std::unique_ptr`示例3:使用`std::shared_ptr`五、总结在C++编程的世界里,内存管理是一项既基础又核心的技能。它直接关系到程序的性能、稳定性和可维护性。不同于一些高级语言自动管理内存的特性,C
- [RA-L 2023] Coco-LIC:基于非均匀 B 样条的连续时间紧密耦合 LiDAR-惯性-相机里程计
十年一梦实验室
c++
这段代码是一个基于C++的均匀B样条(UniformB-spline)实现,专门用于表示SE(3)变换(即三维空间中的刚体变换,包括旋转和平移)。以下是对代码的总结:1.许可证和版权使用BSD3-ClauseLicense,允许在满足条件的情况下自由分发和修改。版权归VladyslavUsenko和NikolausDemmel所有,属于Basalt项目的一部分。2.功能概述文件定义了一个模板类Se
- C++ Map 查询时的两个小细节:`map[id]` 与 `map.value(id, nullptr)` 的区别
誰能久伴不乏
c++java开发语言
文章目录C++Map查询时的两个小细节:`map[id]`与`map.value(id,nullptr)`的区别1.`map[id]`—直接访问和自动插入新元素示例代码:关键点:适用场景:2.`map.value(id,nullptr)`—安全查询并避免插入新元素示例代码:关键点:适用场景:3.对比:`map[id]`与`map.value(id,nullptr)`的区别4.总结:选择合适的方式5
- Python与C ++开发匿名捐赠1对1管理APP
Geeker-2025
pythonc++
开发一款用于**匿名捐赠1对1管理**的App,结合Python和C++的优势,可以实现高效的后端数据处理、实时的捐赠监控以及用户友好的前端界面。以下是一个详细的开发方案,涵盖技术选型、功能模块、开发步骤等内容。##技术选型###后端(Python)-**编程语言**:Python-**Web框架**:Django或Flask-**数据库**:PostgreSQL或MySQL-**实时通信**:W
- 【数据结构之树】
武帝为此
数据结构数据结构
文章目录一、前言二、树的基本概念1.什么是树?2.树的常见分类(1)普通树(2)二叉树(BinaryTree)(3)满二叉树(FullBinaryTree)(4)完全二叉树(CompleteBinaryTree)(5)二叉搜索树(BinarySearchTree,BST)(6)平衡二叉树(AVL树)(7)红黑树(Red-BlackTree)三、树的基本操作及代码示例1.二叉树的基本实现(C++)运
- C/C++高效编译工具ccache
工头阿乐
C/C++c语言c++java
C/C++文章目录C/C++前言一、引入ccache二、ccache基本原理三、安装前言在处理一些规模相对较大的工程时,编译花费的时间可能会很长。有时候我们会经常一遍一遍地编译相同的程序,此时,有了ccache情况就好多了。它将在第一遍编译时多花几秒钟,但接下来就会使编译成倍(5-10倍)的提速。ccache的基本原理是通过将头文件高速缓存到源文件之中而改进了构建性能,因而通过减少每一步编译时添加
- C++高频面试问题
HJ_sky
C++
C++常见面试问题汇总一、指针和引用的区别1.指针有自己的一块空间,而引用只是一个别名;2.使用sizeof看一个指针的大小是4,而引用则是被引用对象的大小;3.指针可以被初始化为NULL,而引用必须被初始化且必须是一个已有对象的引用;4.作为参数传递时,指针需要被解引用才可以对对象进行操作,而直接对引用的修改都会改变引用所指向的对象;5.可以有const指针,但是没有const引用;6.指针在使
- 【华为OD-E卷 -123 判断一组不等式是否满足约束并输出最大差 100分(python、java、c++、js、c)】
CodeClimb
算法题华为od(A+B+C+D+E卷)收录分享华为odpythonjavajavascriptc++
【华为OD-E卷-判断一组不等式是否满足约束并输出最大差100分(python、java、c++、js、c)】题目给定一组不等式,判断是否成立并输出不等式的最大差(输出浮点数的整数部分)要求:不等式系数为double类型,是一个二维数组不等式的变量为int类型,是一维数组;不等式的目标值为double类型,是一维数组不等式约束为字符串数组,只能是:“>”,“>=”,“<”,“<=”,“=”,例如,
- 【华为OD-E卷 -122 字符统计及重排 100分(python、java、c++、js、c)】
CodeClimb
算法题华为od(A+B+C+D+E卷)收录分享python华为odjavac++javascript
【华为OD-E卷-字符统计及重排100分(python、java、c++、js、c)】题目给出一个仅包含字母的字符串,不包含空格,统计字符串中各个字母(区分大小写)出现的次数,并按照字母出现次数从大到小的顺序。输出各个字母及其出现次数。如果次数相同,按照自然顺序进行排序,且小写字母在大写字母之前输入描述输入一行,为一个仅包含字母的字符串输出描述按照字母出现次数从大到小的顺序输出各个字母和字母次数,
- 【华为OD-E卷-02 最多提取子串数目100分(python、java、c++、js、c)】
CodeClimb
算法题华为od(A+B+C+D+E卷)收录分享华为odpythonjavac++javascript
【华为OD-E卷-最多提取子串数目100分(python、java、c++、js、c)】题目给定[a-z],26个英文字母小写字符串组成的字符串A和B,其中A可能存在重复字母,B不会存在重复字母,现从字符串A中按规则挑选一些字母,可以组成字符串B。挑选规则如下:同一个位置的字母只能挑选一次被挑选字母的相对先后顺序不能被改变求最多可以同时从A中挑选多少组能组成B的字符串。输入描述输入为2行,第1行输
- C++高频(二)
HUZ_小Z
c++课程设计笔记经验分享
C++面试高频(二)1.知道动态链接与静态链接吗?两者有什么区别动态链接和静态链接的区别:动态链接:在程序运行时进行链接,加载共享库文件。节省空间,可多个程序共享库文件。灵活性高,可以动态加载不同版本的库文件。维护方便,只需更新库文件本身。静态链接:在编译时进行链接,将库函数复制到可执行文件中。独立的可执行文件,不依赖外部库文件。可执行文件较大,可能会有冗余代码。维护复杂,更新库函数需重新编译和分
- log4j对象改变日志级别
3213213333332132
javalog4jlevellog4j对象名称日志级别
log4j对象改变日志级别可批量的改变所有级别,或是根据条件改变日志级别。
log4j配置文件:
log4j.rootLogger=ERROR,FILE,CONSOLE,EXECPTION
#log4j.appender.FILE=org.apache.log4j.RollingFileAppender
log4j.appender.FILE=org.apache.l
- elk+redis 搭建nginx日志分析平台
ronin47
elasticsearchkibanalogstash
elk+redis 搭建nginx日志分析平台
logstash,elasticsearch,kibana 怎么进行nginx的日志分析呢?首先,架构方面,nginx是有日志文件的,它的每个请求的状态等都有日志文件进行记录。其次,需要有个队 列,redis的l
- Yii2设置时区
dcj3sjt126com
PHPtimezoneyii2
时区这东西,在开发的时候,你说重要吧,也还好,毕竟没它也能正常运行,你说不重要吧,那就纠结了。特别是linux系统,都TMD差上几小时,你能不痛苦吗?win还好一点。有一些常规方法,是大家目前都在采用的1、php.ini中的设置,这个就不谈了,2、程序中公用文件里设置,date_default_timezone_set一下时区3、或者。。。自己写时间处理函数,在遇到时间的时候,用这个函数处理(比较
- js实现前台动态添加文本框,后台获取文本框内容
171815164
文本框
<%@ page language="java" import="java.util.*" pageEncoding="UTF-8"%>
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://w
- 持续集成工具
g21121
持续集成
持续集成是什么?我们为什么需要持续集成?持续集成带来的好处是什么?什么样的项目需要持续集成?... 持续集成(Continuous integration ,简称CI),所谓集成可以理解为将互相依赖的工程或模块合并成一个能单独运行
- 数据结构哈希表(hash)总结
永夜-极光
数据结构
1.什么是hash
来源于百度百科:
Hash,一般翻译做“散列”,也有直接音译为“哈希”的,就是把任意长度的输入,通过散列算法,变换成固定长度的输出,该输出就是散列值。这种转换是一种压缩映射,也就是,散列值的空间通常远小于输入的空间,不同的输入可能会散列成相同的输出,所以不可能从散列值来唯一的确定输入值。简单的说就是一种将任意长度的消息压缩到某一固定长度的消息摘要的函数。
- 乱七八糟
程序员是怎么炼成的
eclipse中的jvm字节码查看插件地址:
http://andrei.gmxhome.de/eclipse/
安装该地址的outline 插件 后重启,打开window下的view下的bytecode视图
http://andrei.gmxhome.de/eclipse/
jvm博客:
http://yunshen0909.iteye.com/blog/2
- 职场人伤害了“上司” 怎样弥补
aijuans
职场
由于工作中的失误,或者平时不注意自己的言行“伤害”、“得罪”了自己的上司,怎么办呢?
在职业生涯中这种问题尽量不要发生。下面提供了一些解决问题的建议:
一、利用一些轻松的场合表示对他的尊重
即使是开明的上司也很注重自己的权威,都希望得到下属的尊重,所以当你与上司冲突后,最好让不愉快成为过去,你不妨在一些轻松的场合,比如会餐、联谊活动等,向上司问个好,敬下酒,表示你对对方的尊重,
- 深入浅出url编码
antonyup_2006
应用服务器浏览器servletweblogicIE
出处:http://blog.csdn.net/yzhz 杨争
http://blog.csdn.net/yzhz/archive/2007/07/03/1676796.aspx
一、问题:
编码问题是JAVA初学者在web开发过程中经常会遇到问题,网上也有大量相关的
- 建表后创建表的约束关系和增加表的字段
百合不是茶
标的约束关系增加表的字段
下面所有的操作都是在表建立后操作的,主要目的就是熟悉sql的约束,约束语句的万能公式
1,增加字段(student表中增加 姓名字段)
alter table 增加字段的表名 add 增加的字段名 增加字段的数据类型
alter table student add name varchar2(10);
&nb
- Uploadify 3.2 参数属性、事件、方法函数详解
bijian1013
JavaScriptuploadify
一.属性
属性名称
默认值
说明
auto
true
设置为true当选择文件后就直接上传了,为false需要点击上传按钮才上传。
buttonClass
”
按钮样式
buttonCursor
‘hand’
鼠标指针悬停在按钮上的样子
buttonImage
null
浏览按钮的图片的路
- 精通Oracle10编程SQL(16)使用LOB对象
bijian1013
oracle数据库plsql
/*
*使用LOB对象
*/
--LOB(Large Object)是专门用于处理大对象的一种数据类型,其所存放的数据长度可以达到4G字节
--CLOB/NCLOB用于存储大批量字符数据,BLOB用于存储大批量二进制数据,而BFILE则存储着指向OS文件的指针
/*
*综合实例
*/
--建立表空间
--#指定区尺寸为128k,如不指定,区尺寸默认为64k
CR
- 【Resin一】Resin服务器部署web应用
bit1129
resin
工作中,在Resin服务器上部署web应用,通常有如下三种方式:
配置多个web-app
配置多个http id
为每个应用配置一个propeties、xml以及sh脚本文件
配置多个web-app
在resin.xml中,可以为一个host配置多个web-app
<cluster id="app&q
- red5简介及基础知识
白糖_
基础
简介
Red5的主要功能和Macromedia公司的FMS类似,提供基于Flash的流媒体服务的一款基于Java的开源流媒体服务器。它由Java语言编写,使用RTMP作为流媒体传输协议,这与FMS完全兼容。它具有流化FLV、MP3文件,实时录制客户端流为FLV文件,共享对象,实时视频播放、Remoting等功能。用Red5替换FMS后,客户端不用更改可正
- angular.fromJson
boyitech
AngularJSAngularJS 官方APIAngularJS API
angular.fromJson 描述: 把Json字符串转为对象 使用方法: angular.fromJson(json); 参数详解: Param Type Details json
string
JSON 字符串 返回值: 对象, 数组, 字符串 或者是一个数字 示例:
<!DOCTYPE HTML>
<h
- java-颠倒一个句子中的词的顺序。比如: I am a student颠倒后变成:student a am I
bylijinnan
java
public class ReverseWords {
/**
* 题目:颠倒一个句子中的词的顺序。比如: I am a student颠倒后变成:student a am I.词以空格分隔。
* 要求:
* 1.实现速度最快,移动最少
* 2.不能使用String的方法如split,indexOf等等。
* 解答:两次翻转。
*/
publ
- web实时通讯
Chen.H
Web浏览器socket脚本
关于web实时通讯,做一些监控软件。
由web服务器组件从消息服务器订阅实时数据,并建立消息服务器到所述web服务器之间的连接,web浏览器利用从所述web服务器下载到web页面的客户端代理与web服务器组件之间的socket连接,建立web浏览器与web服务器之间的持久连接;利用所述客户端代理与web浏览器页面之间的信息交互实现页面本地更新,建立一条从消息服务器到web浏览器页面之间的消息通路
- [基因与生物]远古生物的基因可以嫁接到现代生物基因组中吗?
comsci
生物
大家仅仅把我说的事情当作一个IT行业的笑话来听吧..没有其它更多的意思
如果我们把大自然看成是一位伟大的程序员,专门为地球上的生态系统编制基因代码,并创造出各种不同的生物来,那么6500万年前的程序员开发的代码,是否兼容现代派的程序员的代码和架构呢?
- oracle 外部表
daizj
oracle外部表external tables
oracle外部表是只允许只读访问,不能进行DML操作,不能创建索引,可以对外部表进行的查询,连接,排序,创建视图和创建同义词操作。
you can select, join, or sort external table data. You can also create views and synonyms for external tables. Ho
- aop相关的概念及配置
daysinsun
AOP
切面(Aspect):
通常在目标方法执行前后需要执行的方法(如事务、日志、权限),这些方法我们封装到一个类里面,这个类就叫切面。
连接点(joinpoint)
spring里面的连接点指需要切入的方法,通常这个joinpoint可以作为一个参数传入到切面的方法里面(非常有用的一个东西)。
通知(Advice)
通知就是切面里面方法的具体实现,分为前置、后置、最终、异常环
- 初一上学期难记忆单词背诵第二课
dcj3sjt126com
englishword
middle 中间的,中级的
well 喔,那么;好吧
phone 电话,电话机
policeman 警察
ask 问
take 拿到;带到
address 地址
glad 高兴的,乐意的
why 为什么
China 中国
family 家庭
grandmother (外)祖母
grandfather (外)祖父
wife 妻子
husband 丈夫
da
- Linux日志分析常用命令
dcj3sjt126com
linuxlog
1.查看文件内容
cat
-n 显示行号 2.分页显示
more
Enter 显示下一行
空格 显示下一页
F 显示下一屏
B 显示上一屏
less
/get 查询"get"字符串并高亮显示 3.显示文件尾
tail
-f 不退出持续显示
-n 显示文件最后n行 4.显示头文件
head
-n 显示文件开始n行 5.内容排序
sort
-n 按照
- JSONP 原理分析
fantasy2005
JavaScriptjsonpjsonp 跨域
转自 http://www.nowamagic.net/librarys/veda/detail/224
JavaScript是一种在Web开发中经常使用的前端动态脚本技术。在JavaScript中,有一个很重要的安全性限制,被称为“Same-Origin Policy”(同源策略)。这一策略对于JavaScript代码能够访问的页面内容做了很重要的限制,即JavaScript只能访问与包含它的
- 使用connect by进行级联查询
234390216
oracle查询父子Connect by级联
使用connect by进行级联查询
connect by可以用于级联查询,常用于对具有树状结构的记录查询某一节点的所有子孙节点或所有祖辈节点。
来看一个示例,现假设我们拥有一个菜单表t_menu,其中只有三个字段:
- 一个不错的能将HTML表格导出为excel,pdf等的jquery插件
jackyrong
jquery插件
发现一个老外写的不错的jquery插件,可以实现将HTML
表格导出为excel,pdf等格式,
地址在:
https://github.com/kayalshri/
下面看个例子,实现导出表格到excel,pdf
<html>
<head>
<title>Export html table to excel an
- UI设计中我们为什么需要设计动效
lampcy
UIUI设计
关于Unity3D中的Shader的知识
首先先解释下Unity3D的Shader,Unity里面的Shaders是使用一种叫ShaderLab的语言编写的,它同微软的FX文件或者NVIDIA的CgFX有些类似。传统意义上的vertex shader和pixel shader还是使用标准的Cg/HLSL 编程语言编写的。因此Unity文档里面的Shader,都是指用ShaderLab编写的代码,
- 如何禁止页面缓存
nannan408
htmljspcache
禁止页面使用缓存~
------------------------------------------------
jsp:页面no cache:
response.setHeader("Pragma","No-cache");
response.setHeader("Cache-Control","no-cach
- 以代码的方式管理quartz定时任务的暂停、重启、删除、添加等
Everyday都不同
定时任务管理spring-quartz
【前言】在项目的管理功能中,对定时任务的管理有时会很常见。因为我们不能指望只在配置文件中配置好定时任务就行了,因为如果要控制定时任务的 “暂停” 呢?暂停之后又要在某个时间点 “重启” 该定时任务呢?或者说直接 “删除” 该定时任务呢?要改变某定时任务的触发时间呢? “添加” 一个定时任务对于系统的使用者而言,是不太现实的,因为一个定时任务的处理逻辑他是不
- EXT实例
tntxia
ext
(1) 增加一个按钮
JSP:
<%@ page language="java" import="java.util.*" pageEncoding="UTF-8"%>
<%
String path = request.getContextPath();
Stri
- 数学学习在计算机研究领域的作用和重要性
xjnine
Math
最近一直有师弟师妹和朋友问我数学和研究的关系,研一要去学什么数学课。毕竟在清华,衡量一个研究生最重要的指标之一就是paper,而没有数学,是肯定上不了世界顶级的期刊和会议的,这在计算机学界尤其重要!你会发现,不论哪个领域有价值的东西,都一定离不开数学!在这样一个信息时代,当google已经让世界没有秘密的时候,一种卓越的数学思维,绝对可以成为你的核心竞争力. 无奈本人实在见地