二维平面扭曲的python实现及思路
二维平面扭曲的python实现及思路
- 缘起
- 原理
- 实现
- 代码
缘起
工作需要,需要一个尝试改变设备布点的方法,在csdn闲逛时,偶然间发现这样的一篇文章 二维扭曲,参考这位博主的文章,我对其内容进行复现和进一步挖掘。若有侵权或被侵权,请及时联系我。

原理
基本原理见文章 二维扭曲,核心公式就是
X 1 ′ = X 0 + O D × cos ( θ ′ ) Y 1 ′ = Y 0 + O D × sin ( θ ′ ) X_1'= X_0+ OD×\cos(θ')\\ Y_1'= Y_0+ OD×\sin(θ') X1′=X0+OD×cos(θ′)Y1′=Y0+OD×sin(θ′)
其中,
O D = ( X 1 − X 0 ) 2 + ( Y 1 − Y 0 ) 2 ,也即该点到中心的距离 OD=\sqrt{(X_1-X_0)^2 +(Y_1-Y_0)^2},也即该点到中心的距离 OD=(X1−X0)2+(Y1−Y0)2,也即该点到中心的距离
而
θ ′ = f ( θ , O D ) , 也即是与原来的角度、距离有关的一个函数 \theta' = f(\theta, OD) ,也即是与原来的角度、距离有关的一个函数 θ′=f(θ,OD),也即是与原来的角度、距离有关的一个函数
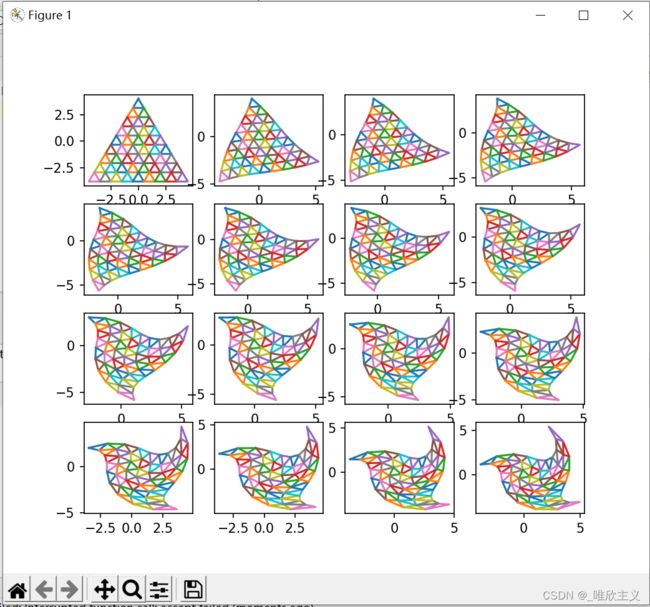
可以看到最令人迷惑的地方在于 f 究竟要怎么取?
这就是一个很有意思的地方了,像上面那幅图,可以观察到 点越远离旋转中心,它的扭曲程度就越大。这句话可以理解为 点越远离旋转中心, θ ′ \theta' θ′ 与 θ \theta θ 的相异程度越大,远离可简单由OD作为评判标准,因此,我们可以构造一个随着 OD变大,、 θ ′ \theta' θ′变化越大的这样一个f
θ ′ = f ( θ , O D ) = θ + k ∗ O D , ( k > 0 ) \theta' = f(\theta, OD) =\theta + k * OD,~~~~(k > 0) θ′=f(θ,OD)=θ+k∗OD, (k>0)
实现
我们使用的工具是 matplotlib 下 pyplot,先把原来的大三角形画出来
代码
from matplotlib import pyplot as plt
from copy import deepcopy
import math
def triangle_struct(layer=8, length=1):
fig = plt.figure()
x = list()
y = list()
height = length * (3 ** 0.5) / 2
top_height = height * (layer + 1) // 2
x.append([0])
y.append(top_height)
for i in range(1, layer):
tmp = list()
for j in x[-1]:
tmp.append(j - length / 2)
tmp.append(x[-1][-1] + length / 2)
x.append(tmp)
top_height -= height
y.append(top_height)
# print(x_axis)
# print(y_axis)
return [x, y]
def plot(x, y, row, col, num, fig):
layer = len(y)
axis = fig.add_subplot(row, col, num)
for i in range(1, layer):
for j in range(i):
plt.plot([x[i - 1][j], x[i][j], x[i][j + 1], x[i - 1][j]],
[y[i - 1], y[i], y[i], y[i - 1]])
def plot3(x, y, row, col, num, fig):
layer = len(y)
axis = fig.add_subplot(row, col, num)
for i in range(1, layer):
for j in range(i):
axis.plot([x[i - 1][j], x[i][j], x[i][j + 1], x[i - 1][j]],
[y[i - 1][j], y[i][j], y[i][j + 1], y[i - 1][j]])
def distort(x_list, y_list, k=1):
g = lambda theta, distance, k: theta + 0.02 * k * distance
new_x = deepcopy(x_list)
new_y = deepcopy(x_list)
for index in range(len(x_list)):
y = y_list[index]
for j in range(index + 1):
angle, distance = get_polar_para(x_list[index][j], y)
new_x[index][j] = distance * math.cos(g(angle, distance, k))
new_y[index][j] = distance * math.sin(g(angle, distance, k))
return [new_x, new_y]
x_axis, y_axis = triangle_struct(layer=10)
fig = plt.figure(num=1, figsize=(18, 10))
plot(x_axis, y_axis, 4, 4, 1, fig)
for i in range(2, 17):
_x, _y = distort(x_axis, y_axis, k=i)
plot3(_x, _y, 4, 4, i, fig)
plt.show()