数据结构 | 堆【图解】

数据结构 | 堆【图解】

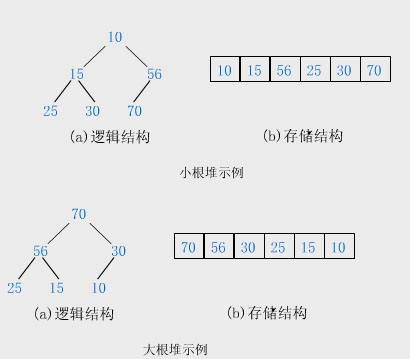
堆的概念及结构

堆（heap）： 一种有特殊用途的数据结构——用来在一组变化频繁（发生增删查改的频率较高）的数据集中查找最值。

堆在物理层面上：表现为一组连续的数组区间：long[] array ；将整个数组看作是堆。

堆在逻辑结构上：一般被视为是一颗完全二叉树。

满足任意结点的值都大于其子树中结点的值，叫做大堆，或者大根堆，或者最大堆；反之，则是小堆，或者小根堆，或者最小堆。当一个堆为大堆时，它的每一棵子树都是大堆。

• 堆一般是数组数据看做一颗完全二叉树
  • 小堆要求：任意一个父亲<=孩子
  • 大堆要求：任意一个父亲>=孩子

这里是没有中堆的！

堆的实现

Heap.h

• 需要实现堆的函数

#pragma once

#include
#include
#include
#include


typedef int HPDataType;

typedef struct Heap
{
	HPDataType* a;
	int size;
	int capacity;
}Heap;

// 堆的构建
void HeapInit(Heap* hp);
// 堆的插入
void HeapPush(Heap* hp, HPDataType x);
// 堆的删除
void HeapPop(Heap* hp);
// 取堆顶的数据
HPDataType HeapTop(Heap* hp);
// 堆的数据个数
int HeapSize(Heap* hp);
// 堆的判空
bool HeapEmpty(Heap* hp);
// 堆的销毁
void HeapDestory(Heap* hp);

---

• 接下来我们就开始实现堆~~

堆的初始化

• 这里直接初始化，不多介绍

void HeapInit(Heap* hp)
{
	assert(hp);
	hp->a = NULL;
	hp->capacity = hp->size = 0;
}

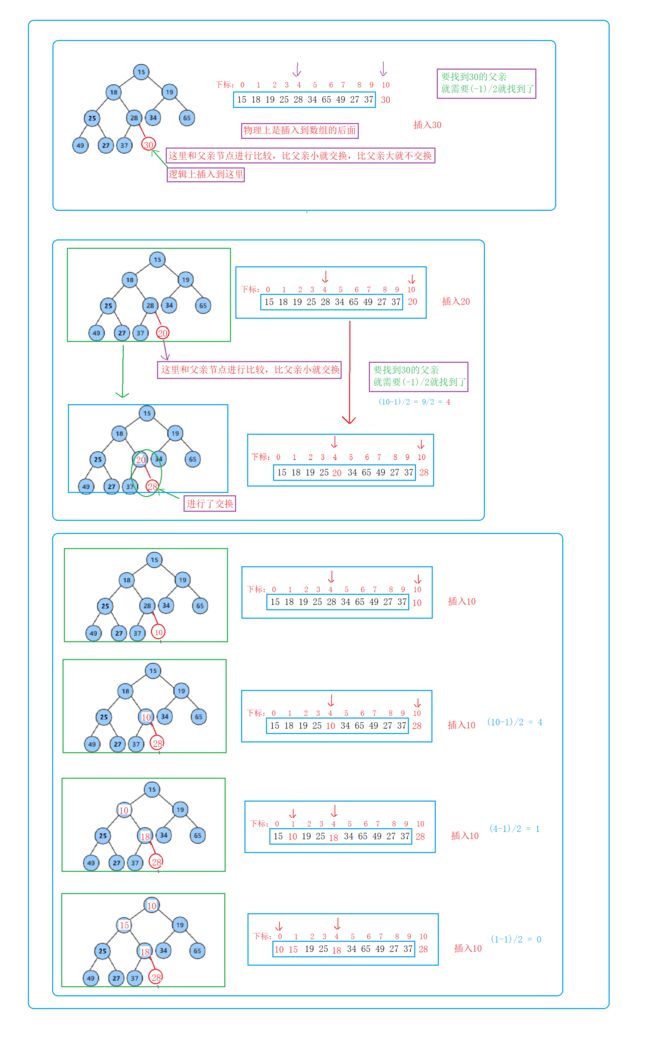
堆的插入【重点】

• 检查空间是否满了，满了就扩容
• 然后将值插入到最后
• 最后向上调整

向上调整算法，依次pk

• 这里的size是size-1，而不是size，因为是放完数据后size++了一下，然后要取size-1的位置
• 如果孩子节点小于父亲节点就交换
• 然后再将父亲节点给了孩子节点，再进行(-1)/2
• 如果大于等于父亲就跳出循环
• 跳出的条件是child > 0
• 这里的向上时间复杂度是O(logN)

//交换
void Swap(int* p1, int* p2)
{
	HPDataType tmp = *p1;
	*p1 = *p2;
	*p2 = tmp;
}
//向上调整
void AjustUp(HPDataType* a, HPDataType child)
{
	HPDataType parent = (child - 1) / 2;
	while (child > 0)
	{
		if (a[child] < a[parent])
		{
			Swap(&a[child], &a[parent]);
			child = parent;
			parent = (parent - 1) / 2;
		}
		else
		{
			break;
		}
	}
}

// 堆的插入
void HeapPush(Heap* hp, HPDataType x)
{
	assert(hp);
	if (hp->capacity == hp->size)
	{
		HPDataType newcapacity = hp->capacity == 0 ? 4 : hp->capacity * 2;
		HPDataType* tmp = (HPDataType*)realloc(hp->a, sizeof(HPDataType) * newcapacity);
		if (tmp == NULL)
		{
			perror("realloc fail\n");
			exit(-1);
		}
		hp->a = tmp;
		hp->capacity = newcapacity;
	}

	hp->a[hp->size] = x;
	hp->size++;

	AjustUp(hp->a, hp->size - 1);
}

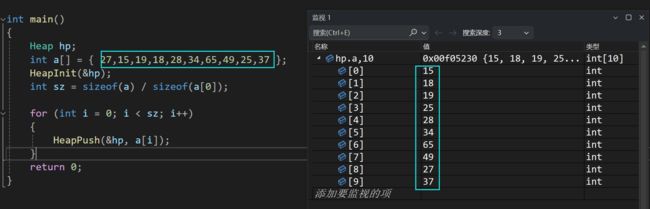
• 我们测试一下~~

int main()
{
	Heap hp;
	int a[] = { 27,15,19,18,28,34,65,49,25,37 };
	HeapInit(&hp);
	int sz = sizeof(a) / sizeof(a[0]);
	
	for (int i = 0; i < sz; i++)
	{
		HeapPush(&hp, a[i]);
	}
	return 0;
}

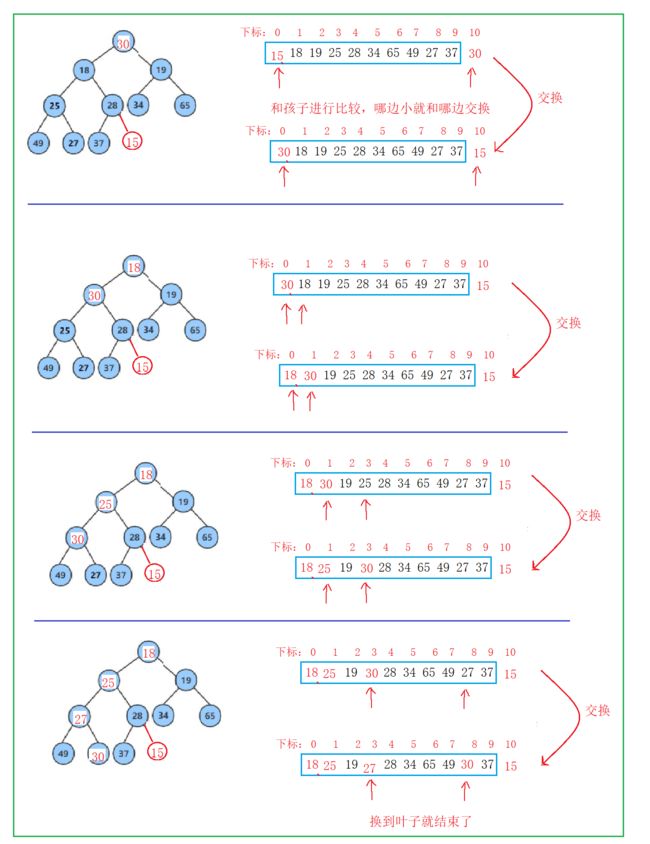
堆的删除【重点】

• 堆的删除是堆顶上的数据，而不是删除根节点，删除最下面的那个数据是没有意义的~~

• 删除后要进行调整

步骤一：

交换

步骤二：

向下调整算法

这里的向下时间复杂度是O(logN)，和向上一样，调整高度次

//向下调整
void AdjustDown(int* a, int size, int parent)
{
	//假设左孩子小，假设错了就更新
	int child = parent * 2 + 1;
	while (child < size)
	{
		if (a[child + 1] < a[child])
		{
			++child;
		}
		if (a[child] < a[parent])
		{
			Swap(&a[child], &a[parent]);
			parent = child;
			child = parent * 2 + 1;
		}
		else
		{
			break;
		}
	}
}
// 堆的删除
void HeapPop(Heap* hp)
{
	assert(hp);
	assert(hp->size > 0);

	//首尾交换
	Swap(&hp->a[0], &hp->a[hp->size - 1]);
	hp->size--;

	//从根向下调整
	AdjustDown(hp->a, hp->size, 0);
}

取堆顶的数据

• 这里直接取数组第一个元素就可以了

HPDataType HeapTop(Heap* hp)
{
	assert(hp);
	assert(hp->size > 0);

	return hp->a[0];
}

堆的数据个数

• 这里也一样，取size

int HeapSize(Heap* hp)
{
	assert(hp);
	return hp->size;
}

堆的判空

• 判断size是否为0

bool HeapEmpty(Heap* hp)
{
	assert(hp);
	return hp->size == 0;
}

堆的销毁

• 销毁也不多说了，很简单

void HeapDestory(Heap* hp)
{
	assert(hp);
	free(hp->a);
	hp->capacity = hp->size = 0;
}

全部代码

//小堆算法
// 堆的构建
void HeapInit(Heap* hp)
{
	assert(hp);
	hp->a = NULL;
	hp->capacity = hp->size = 0;
}

//交换
void Swap(int* p1, int* p2)
{
	HPDataType tmp = *p1;
	*p1 = *p2;
	*p2 = tmp;
}


//向上调整
void AdjustUp(HPDataType* a, HPDataType child)
{
	HPDataType parent = (child - 1) / 2;
	while (child > 0)
	{
		if (a[child] < a[parent])
		{
			Swap(&a[child], &a[parent]);
			child = parent;
			parent = (parent - 1) / 2;
		}
		else
		{
			break;
		}
	}
}

// 堆的插入
void HeapPush(Heap* hp, HPDataType x)
{
	assert(hp);
	if (hp->capacity == hp->size)
	{
		int newcapacity = hp->capacity == 0 ? 4 : hp->capacity * 2;
		HPDataType* tmp = (HPDataType*)realloc(hp->a, sizeof(HPDataType) * newcapacity);
		if (tmp == NULL)
		{
			perror("realloc fail\n");
			exit(-1);
		}
		hp->a = tmp;
		hp->capacity = newcapacity;
	}

	hp->a[hp->size] = x;
	hp->size++;

	AdjustUp(hp->a, hp->size - 1);
}

//向下调整
void AdjustDown(int* a, int size, int parent)
{
	//假设左孩子小，假设错了就更新
	int child = parent * 2 + 1;
	while (child < size)
	{
		if (child + 1 < size && a[child + 1] < a[child])
		{
			++child;
		}
		if (a[child] < a[parent])
		{
			Swap(&a[child], &a[parent]);
			parent = child;
			child = parent * 2 + 1;
		}
		else
		{
			break;
		}
	}
}
// 堆的删除
void HeapPop(Heap* hp)
{
	assert(hp);
	assert(hp->size > 0);

	//首尾交换
	Swap(&hp->a[0], &hp->a[hp->size - 1]);
	hp->size--;

	//从根向下调整
	AdjustDown(hp->a, hp->size, 0);
}
// 取堆顶的数据
HPDataType HeapTop(Heap* hp)
{
	assert(hp);
	assert(hp->size > 0);

	return hp->a[0];
}
// 堆的数据个数
int HeapSize(Heap* hp)
{
	assert(hp);

	return hp->size;
}
// 堆的判空
bool HeapEmpty(Heap* hp)
{
	assert(hp);

	return hp->size == 0;
}

// 堆的销毁
void HeapDestory(Heap* hp)
{
	assert(hp);

	free(hp->a);
	hp->capacity = hp->size = 0;
}

以上是小堆的算法，大堆也是一样的，只需要改几个符号就可以了~~

堆的介绍就到这里结束了，感谢收看~~