算法基础12 —— 树进阶(二叉搜索树 + 堆—优先队列)

二叉搜索树

定义：
二叉搜索树，又叫二叉排序树、二叉查找树(Binary Search Tree)

它或者是一棵空树，或者是具有下列性质的二叉树：

• 若它的左子树不空，则左子树上所有结点的值均小于它的根结点的值；
• 若它的右子树不空，则右子树上所有结点的值均大于它的根结点的值；
• 它的左、右子树也分别为二叉排序树

性质：二叉搜索树的中序遍历结果为一个无重复数据的升序序列

判断方法：将待判断的二叉树采用中序遍历，结果保存在vector中，然后遍历vector，判断是否满足是升序并且无重复数据，不满足则不是二叉搜索树，反之则是搜索二叉树。
时间空间复杂度分析： 时间复杂度为O(N)，空间复杂度为O(N)

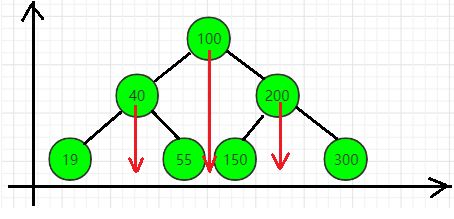
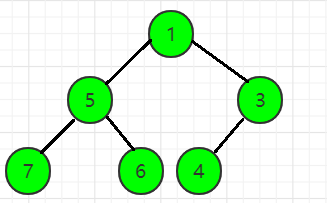
下图就是一棵二叉搜索树

其先序遍历为(根左右)：100 40 19 55 200 150 300
其中序遍历为(左根右)：19 40 55 100 150 200 300
其后序遍历为(左右根)：19 55 40 150 300 200 100
不难发现，其中序序列递增
另外，对二叉搜索树进行垂直投影，可以直接得到其中序序列

代码实现：

堆 —— 优先队列

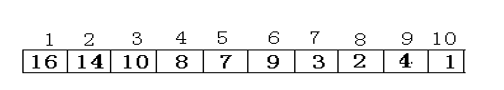
问题引入：假如有14个数，分别是99、5、36、7、22、17、46、12、2、19、25、28、1和92，请找出这14个数中最小的数。
暴力法：时间复杂度是O(N)

for(int i = 1;i <= 14;i++)
{
	if (a[i] < min)   min = a[i];
}

• 现在我们需要删除其中最小的数，并增加一个新数23，再次求这N个数中最小的一个数
• 该怎么办？
• 假设有N次这样的操作，那么整个时间复杂度为O(NxN)。
• 有没有效率更高的办法呢？
• 堆来解决，时间复杂度O(nlogn)

什么是堆？

定义以及存储方式：
堆结构是一种数组对象，它可以被视为一棵完全二叉树。如下图是一个大根堆：

利用数组进行顺序存储：

堆的性质：

对于每个结点 i

• 如果每个结点都大于等于他的孩子结点，称为大根堆
• 反之，如果每个结点都小于等于他的孩子结点，称为小根堆

堆的操作(建堆):

一、往堆中加入一个元素的算法(put)如下：
(1) 在堆尾加入一个元素，并把这个结点置为当前结点
(2) 比较当前结点和它父结点的大小

• 如果当前结点小于父结点，则交换它们的值，并把父结点置为当前结点。转(2)
• 如果当前结点大于等于父结点，则转(3)

(3) 结束
重复n次put操作，即可建立一个小根堆。
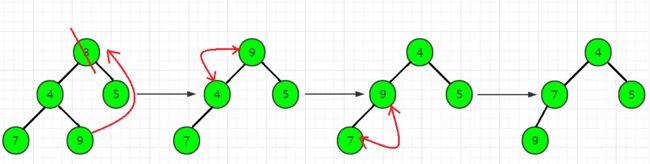
例：设n = 10，10个数依次为：3、5、1、7、6、4、2、5、4、1。建小根堆的过程如下：
第1步，读入一个数x = 3，len = 1，heap[len] = x，堆中只有一个数，必定会满足堆的性质：


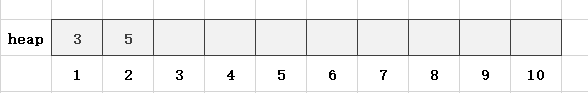
第2步，读入一个数x = 5，len = 2，heap[len] = x，依然满足堆的性质：


第3步，读入一个数x = 1，len = 3，heap[len] = x，此时heap[1] = 3，heap[3] = 1，不满足小根堆的性质，故需要交换heap[1]和heap[3]：


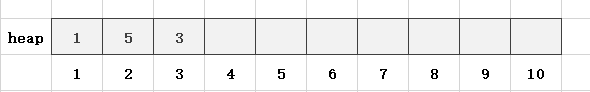
第4步，读入一个数x = 7，len = 4，heap[len] = x，满足堆的性质：

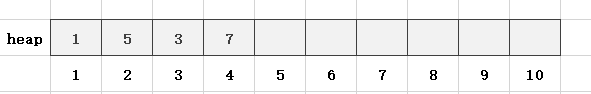
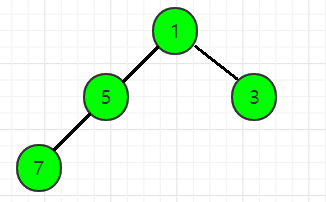
第5步，读入一个数x = 6，len = 5，heap[len] = x，满足堆的性质：


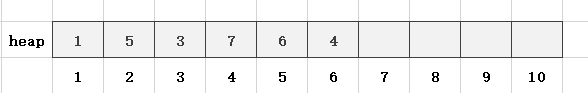
第6步，读入一个数x = 4，len = 6，heap[len] = x，满足堆的性质：


第7步，读入一个数x = 2，len = 7，heap[len] = x，此时heap[7] = 2，heap[3] = 3，不满足小根堆的性质，故需要交换heap[7]和heap[3]：


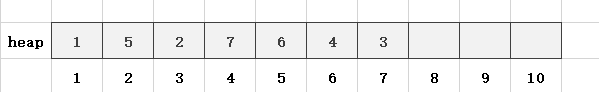
第8步，读入一个数x = 5，len = 8，heap[len] = x，此时heap[8] = 5，heap[4] = 7，不满足小根堆的性质，故需要交换heap[8]和heap[4]：


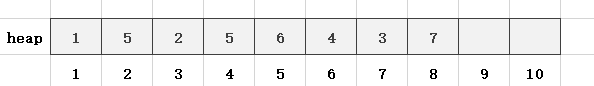
第9步，读入一个数x = 4，len = 9，heap[len] = x，此时heap[9] = 4，heap[4] = 7，不满足小根堆的性质，故需要先交换heap[9]和heap[4]：


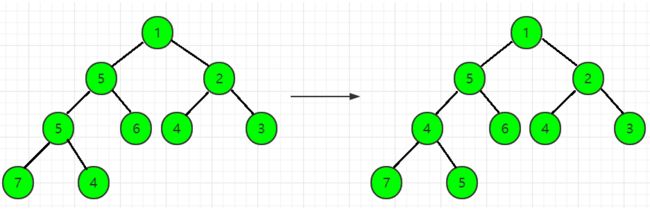
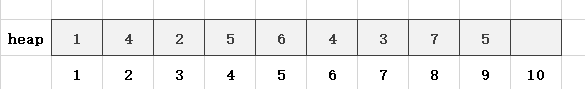
但是，经过上一步的转换之后，此时heap[2] = 5，heap[4] = 4，仍然不满足小根堆的性质，故需要继续交换heap[2]和heap[4]：


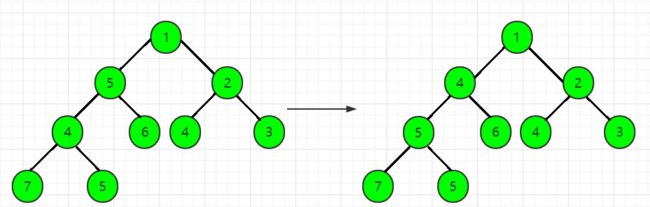
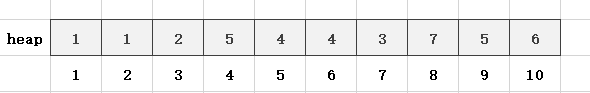
第10步，读入一个数x = 1，len = 10，heap[len] = x，先把1从heap[10]调整到heap[5]，再把1从heap[5]调整到heap[2]，得到最后的小根堆，如图所示：


代码实现建立小根堆：

#include 
#include 
#include 

using namespace std;

const int N = 100;
int heap[N];//保存堆
int heapSize;//表示堆的长度

void put(int d)//建堆模板
{
	int now,next;//now表示当前结点,next表示父亲结点
	heap[++heapSize] = d;
	now = heapSize;
	while (now > 1)
	{
		next = now >> 1;//父亲结点的位置=当前结点的位置/2
		if (heap[now] >= heap[next]) break;//当前结点大于等于父亲结点
		else swap(heap[now],heap[next]);
		now = next;//将当前结点设置为父亲结点
	}
}

int main()
{
    int n,x;
	cin >> n;//10
	for (int i = 1;i <= n;i++)
	{
		cin >> x;//3 5 1 7 6 4 2 5 4 1
		put(x);
	}
	for (int i = 1;i <= n;i++) cout << heap[i] << " ";
	return 0;
}

输入
10
3 5 1 7 6 4 2 5 4 1
输出
1 1 2 5 4 4 3 7 5 6

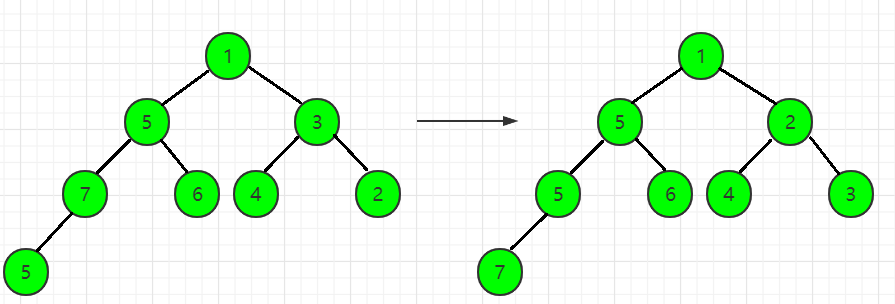
堆的堆顶元素具有最值问题，例如对于一个小根堆，其堆顶元素即为最小值。同理，大根堆的堆顶元素即为最大值。当取出堆顶元素之后，剩下的ｎ－１个结点如何继续构成一个小根堆，是一个很重要的问题。

二、从堆中取出并删除一个元素的算法(get)如下：

(1) 取出堆的根结点heap[1]的值。
(2) 把堆的最后一个结点(heap[heapSize])放到根的位置上，把根覆盖掉。把堆的长度减一
(3) 把根结点置为当前父结点pa
(4) 如果pa无儿子(2 * pa > len)，则转(6)；否则，把pa的两(或一)个儿子中值最小的那个置为当前的子结点son
(5) 比较pa与son的值：

• 如果pa的值小于或等于son，则转(6)
• 否则，交换这两个结点的值，把pa指向son，转(4)

(6) 结束

举例：

代码实现：

int get()//取出堆顶元素,heap[1]为堆顶
{
	int now = 1,res = heap[1];
	int next;
	heap[1] = heap[heapSize];//将最后一个元素置于堆顶
	heapSize --;
	//重新调整为小根堆
	while (now * 2 <= heapSize)//存在左孩子
	{
		next = now * 2;
		//next < heapSize说明heapSize+1存在,然后找出左右孩子中较小的那个
		if (next < heapSize && heap[next + 1] < heap[next]) next++;
		if (heap[now] <= heap[next]) break;//当前结点小于等于左右孩子中较小的那个
		else swap(heap[now],heap[next]);
		now = next;
	}
	return res;
}

堆排序(heapsort)

假设n个数存放在a[1… …n]中，我们可以利用堆将它们从小到大进行排序，这种排序方法称为“堆排序”。将建堆与获取堆顶元素组合起来，就实现了堆排序。

#include 
#include 
#include 

using namespace std;

const int N = 100;
int heap[N];//保存堆
int heapSize;//表示堆的长度

void put(int d)//建堆
{
	int now,next;//now表示当前结点,next表示父亲结点
	heap[++heapSize] = d;
	now = heapSize;
	while (now > 1)
	{
		next = now >> 1;//父亲结点的位置=当前结点的位置/2   
		if (heap[now] >= heap[next]) break;//当前结点大于等于父亲结点
		else swap(heap[now],heap[next]);
		now = next;//将当前结点设置为父亲结点
	}
}

int get()//取出堆顶元素,heap[1]为堆顶
{
	int now = 1,res = heap[1];
	int next;
	heap[1] = heap[heapSize];//将最后一个元素置于堆顶
	heapSize --;
	//重新调整为小根堆
	while (now * 2 <= heapSize)//存在左孩子 2 ^ x = n ——> O(logn)
	{
		next = now * 2;
		//next < heapSize说明heapSize+1存在,然后找出左右孩子中较小的那个
		if (next < heapSize && heap[next + 1] < heap[next]) next++;
		if (heap[now] <= heap[next]) break;//当前结点小于等于左右孩子中较小的那个
		else swap(heap[now],heap[next]);
		now = next;
	}
	return res;
}

int main()
{
    int n,x;
	cin >> n;//10
	for (int i = 1;i <= n;i++)
	{
		cin >> x;//3 5 1 7 6 4 2 5 4 1
		put(x);
	}
	//输出堆
	for (int i = 1;i <= n;i++) cout << heap[i] << " ";
	//堆排序
	cout << endl;
	int result = 0;
	for (int i = 1;i <= n;i++)//O(n) 
	{
	    result = get();//O(logn)
	    cout << result << " ";
	}
	return 0;
}

输入
10
3 5 1 7 6 4 2 5 4 1
输出
1 1 2 5 4 4 3 7 5 6
1 1 2 3 4 4 5 5 6 7

堆排序的时间复杂度为O(nlogn)