【leetcode题解C++】101.对称二叉树 and 111.二叉树的最小深度 and 222.完全二叉树的节点个数 and 110.平衡二叉树
101. 对称二叉树
给你一个二叉树的根节点 root , 检查它是否轴对称。
示例 1:
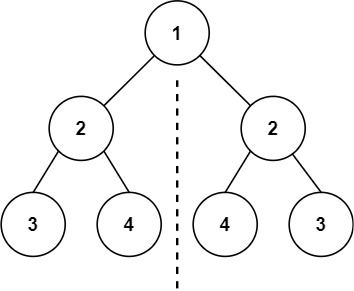
输入:root = [1,2,2,3,4,4,3] 输出:true
示例 2:
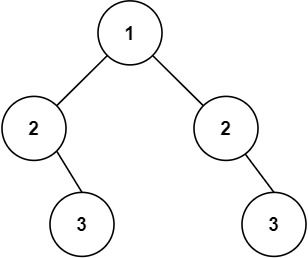
输入:root = [1,2,2,null,3,null,3] 输出:false
思路:想到了用队列(迭代),把每一对结点入队,判定的条件有val的值是否相等,还有某某结点是否存在,需要注意的是入队的顺序,要符合对称的判定。leetcode官方题解也有一种递归的方法,代码很简短,下面也会给出。
代码实现1:迭代
class Solution {
public:
bool isSymmetric(TreeNode* root) {
queue que;
que.push(root->left);
que.push(root->right);
while(!que.empty()) {
TreeNode *node1 = que.front(); que.pop();
TreeNode *node2 = que.front(); que.pop();
if(!node1 && !node2) continue;
if(!node1 || !node2 || node1->val != node2->val) return false;
que.push(node1->left);
que.push(node2->right);
que.push(node1->right);
que.push(node2->left);
}
return true;
}
}; 代码实现2:递归
class Solution {
public:
bool check(TreeNode *p, TreeNode *q) {
if (p==nullptr && q==nullptr) return true;
if (p==nullptr || q==nullptr) return false;
return p->val == q->val && check(p->left, q->right) && check(p->right, q->left);
}
bool isSymmetric(TreeNode* root) {
return check(root, root);
}
};111. 二叉树的最小深度(最大深度类似)
给定一个二叉树,找出其最小深度。
最小深度是从根节点到最近叶子节点的最短路径上的节点数量。
说明:叶子节点是指没有子节点的节点。
示例 1:
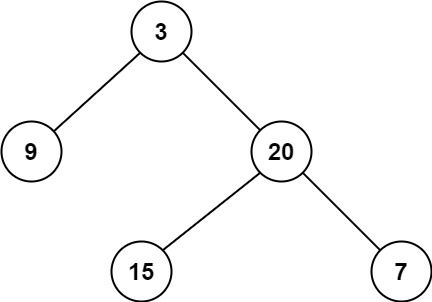
输入:root = [3,9,20,null,null,15,7] 输出:2
示例 2:
输入:root = [2,null,3,null,4,null,5,null,6] 输出:5
思路:用一个队列层序遍历即可,return的时机是:出现第一个节点,它的左右孩子都为空。(如果是记录最大深度,把这个中途return的判定去掉即可)
代码实现:
class Solution {
public:
int minDepth(TreeNode* root) {
queue que;
int depth = 0;
if(!root) return depth;
que.push(root);
TreeNode *node;
while(!que.empty()) {
int size = que.size();
++depth;
for(int i = 0; i < size; ++i) {
node = que.front();
que.pop();
if(node->left) que.push(node->left);
if(node->right) que.push(node->right);
if(!node->right && !node->left) return depth; //中途判定
}
}
return depth;
}
}; 222. 完全二叉树的节点个数
给你一棵 完全二叉树 的根节点 root ,求出该树的节点个数。
完全二叉树的定义如下:在完全二叉树中,除了最底层节点可能没填满外,其余每层节点数都达到最大值,并且最下面一层的节点都集中在该层最左边的若干位置。若最底层为第 h 层,则该层包含 1~ 2h 个节点。
示例 1:
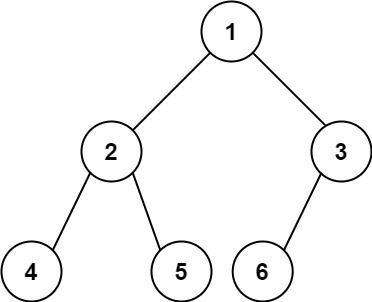
输入:root = [1,2,3,4,5,6] 输出:6
示例 2:
输入:root = [] 输出:0
示例 3:
输入:root = [1] 输出:1
思路:注意到,完全二叉树,那么使用队列来层序遍历即可,用ret来记录节点数,每多一个节点,ret++。
class Solution {
public:
int countNodes(TreeNode* root) {
queue que;
int ret = 0;
if(!root) return ret;
que.push(root);
TreeNode *node;
while(!que.empty()) {
int size = que.size();
for(int i = 0; i< size; ++i) {
node = que.front();
que.pop();
++ret;
if(node->left) que.push(node->left);
if(node->right) que.push(node->right);
}
}
return ret;
}
}; 110. 平衡二叉树
给定一个二叉树,判断它是否是高度平衡的二叉树。
本题中,一棵高度平衡二叉树定义为:
一个二叉树每个节点 的左右两个子树的高度差的绝对值不超过 1 。
示例 1:
输入:root = [3,9,20,null,null,15,7] 输出:true
示例 2:
输入:root = [1,2,2,3,3,null,null,4,4] 输出:false
示例 3:
输入:root = [] 输出:true
思路:一开始就想用层序遍历记录最小和最大深度,然后来相减,后来一想要是二叉树是只有一支的话,实现不了,单独判定也不好判定。遂学习...,找到了递归的方法,判断每一个节点的左右子树是否为平衡的。
代码实现:
class Solution {
public:
int getHeight(TreeNode *node) {
if(!node) return 0;
int leftHeight = getHeight(node->left);
if(leftHeight == -1) return -1;
int rightHeight = getHeight(node->right);
if(rightHeight == -1) return -1;
return abs(leftHeight - rightHeight) > 1 ?
-1 : max(leftHeight, rightHeight) + 1;
}
bool isBalanced(TreeNode* root) {
return getHeight(root) == -1 ? false : true;
}
};