1. Goal: Simulate the motion of N moving particles that behave according to the laws of elastic collision.
Hard disc model:
- Moving particles interact via elastic collisions with each other and walls.
- Each particle is a disc with known position, velocity, mass, and radius.
- No other forces.
2. N bouncing balls in the unit square without considering balls colliding with each other
public class Ball
{
private double rx, ry; // position
private double vx, vy; // velocity
private final double radius; // radius
public Ball(...)
{ /* initialize position and velocity */ }
public void move(double dt)
{
if ((rx + vx*dt < radius) || (rx + vx*dt > 1.0 - radius)) {
rx = 1.0 - radius - vx * dt + (1.0 - radius - rx);
vx = -vx;
} else {
rx = rx + vx * dt;
}
if ((ry + vy*dt < radius) || (ry + vy*dt > 1.0 - radius)) {
ry = 1.0 - radius - vy * dt + (1.0 - radius - ry);
vy = -vy;
} else {
ry = ry + vy*dt;
}
}
public void draw()
{
StdDraw.filledCircle(rx, ry, radius);
}
}
public class BouncingBalls
{
public static void main(String[] args)
{
int N = Integer.parseInt(args[0]);
Ball[] balls = new Ball[N];
for (int i = 0; i < N; i++)
balls[i] = new Ball();
while(true)
{
StdDraw.clear();
for (int i = 0; i < N; i++)
{
balls[i].move(0.5);
balls[i].draw();
}
StdDraw.show(50);
}
}
}
3. Naive Implementation for handling collision:
-- Discretize time in quanta of size dt.
-- Update the position of each particle after every dt units of time, and check for overlaps.
-- If overlap, roll back the clock to the time of the collision, update the velocities of the colliding particles, and continue the simulation.
Main drawbacks:
-- ~ N^2 / 2 overlap checks per time quantum.
-- Simulation is too slow if dt is very small.
-- May miss collisions if dt is too large. (if colliding particles fail to overlap when we are looking)
4. Event-Driven Simulation :
Change state only when something happens.
-- Between collisions, particles move in straight-line trajectories.
-- Focus only on times when collisions occur.
-- Maintain PQ of collision events, prioritized by time.
-- Remove the min = get next collision.
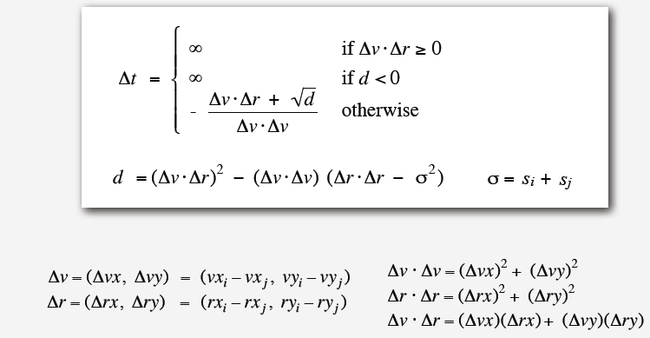
Collision prediction.
-- Particle i: radius si, position (rxi, ryi), velocity (vxi, vyi).
-- Particle j: radius sj, position (rxj, ryj), velocity (vxj, vyj).
-- Will particles i and j collide? If so, when?
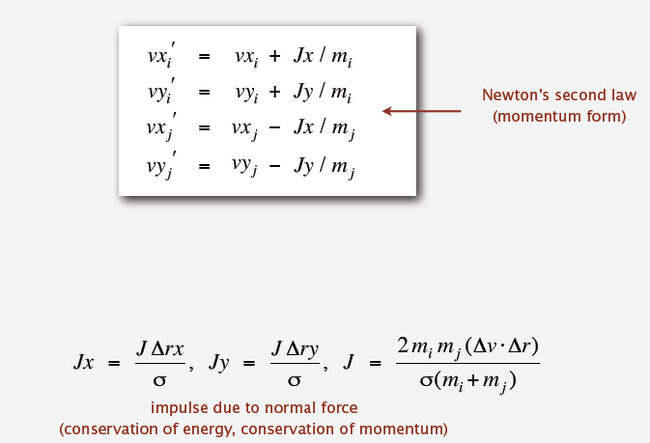
Collision resolution.
-- If collision occurs, update colliding particle(s) according to laws of elastic collisions.
5. Collision system :
Initialization:
-- Fill PQ with all potential particle-wall collisions.
-- Fill PQ with all potential particle-particle collisions.
Main loop:
-- Delete the impending event from PQ (min priority = t).
-- If the event has been invalidated, ignore it.
-- Advance all particles to time t, on a straight-line trajectory.
-- Update the velocities of the colliding particle(s).
-- Predict future particle-wall and particle-particle collisions involving the colliding particle(s) and insert events onto PQ.
/**
Conventions.
-- Neither particle null ⇒ particle-particle collision.
-- One particle null ⇒ particle-wall collision.
-- Both particles null ⇒ redraw event.
*/
private class Event implements Comparable<Event>
{
private double time; // time of event
private Particle a, b; // particles involved in event
private int countA, countB; // collision counts for a and b, used to check whether the collsion is still valid
public Event(double t, Particle a, Particle b) { }
public int compareTo(Event that)
{return this.time - that.time; }
public boolean isValid() { }
}
public class Particle
{
private double rx, ry; // position
private double vx, vy; // velocity
private final double radius; // radius
private final double mass; // mass
private int count; // number of collisions
public Particle(...) { }
public void move(double dt) { }
public void draw() { }
public double timeToHit(Particle that) {
if (this == that) return INFINITY;
double dx = that.rx - this.rx, dy = that.ry - this.ry;
double dvx = that.vx - this.vx; dvy = that.vy - this.vy;
double dvdr = dx*dvx + dy*dvy;
if( dvdr > 0) return INFINITY;
double dvdv = dvx*dvx + dvy*dvy;
double drdr = dx*dx + dy*dy;
double sigma = this.radius + that.radius;
double d = (dvdr*dvdr) - dvdv * (drdr - sigma*sigma);
if (d < 0) return INFINITY;
return -(dvdr + Math.sqrt(d)) / dvdv;
}
public double timeToHitVerticalWall() { }
public double timeToHitHorizontalWall() { }
public void bounceOff(Particle that) {
double dx = that.rx - this.rx, dy = that.ry - this.ry;
double dvx = that.vx - this.vx, dvy = that.vy - this.vy;
double dvdr = dx*dvx + dy*dvy;
double dist = this.radius + that.radius;
double J = 2 * this.mass * that.mass * dvdr / ((this.mass + that.mass) * dist);
double Jx = J * dx / dist;
double Jy = J * dy / dist;
this.vx += Jx / this.mass;
this.vy += Jy / this.mass;
that.vx -= Jx / that.mass;
that.vy -= Jy / that.mass;
this.count++;
that.count++;
}
public void bounceOffVerticalWall() { }
public void bounceOffHorizontalWall() { }
}
public class CollisionSystem
{
private MinPQ<Event> pq; // the priority queue
private double t = 0.0; // simulation clock time
private Particle[] particles; // the array of particles
public CollisionSystem(Particle[] particles) { }
private void predict(Particle a)
{
if (a == null) return;
for (int i = 0; i < N; i++)
{
double dt = a.timeToHit(particles[i]);
pq.insert(new Event(t + dt, a, particles[i]));
}
pq.insert(new Event(t + a.timeToHitVerticalWall() , a, null));
pq.insert(new Event(t + a.timeToHitHorizontalWall(), null, a));
}
private void redraw() { }
public void simulate() {
pq = new MinPQ<Event>();
for(int i = 0; i < N; i++) predict(particles[i]);
pq.insert(new Event(0, null, null));
while(!pq.isEmpty())
{
Event event = pq.delMin();
if(!event.isValid()) continue;
Particle a = event.a;
Particle b = event.b;
for(int i = 0; i < N; i++)
particles[i].move(event.time - t);
t = event.time;
if (a != null && b != null) a.bounceOff(b);
else if (a != null && b == null) a.bounceOffVerticalWall();
else if (a == null && b != null) b.bounceOffHorizontalWall();
else if (a == null && b == null) redraw();
predict(a);
predict(b);
}
}
}