HDU 1693 Eat the Trees (插头DP,闭合路径)
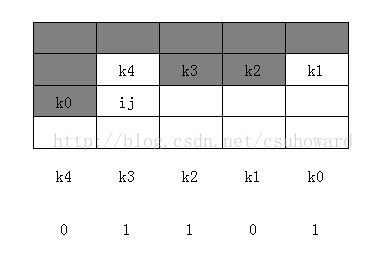
之前对于经典骨牌的轮廓线DP的位压缩,采用的如下形状的位压缩:
当ij判断放置哪种骨牌时,是根据k4k3k2k1k0判断的,在填入了骨牌后,转移到的状态也是由k3k2k1k0ij这样排布的,就是原状态左移一下,如果横着放骨牌就会影响到k0位,竖着放和不放都是把ij从右边附加到原状态上。
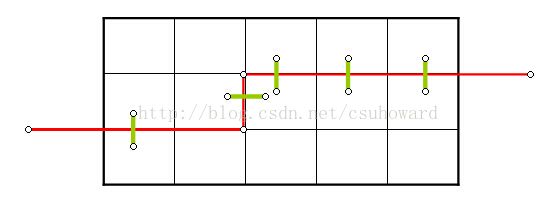
本题的插头DP是保存插头的状态,采用如下的状态:
红线就是轮廓线,轮廓线上有插头。有插头就记为1,没有插头就记为0。上图就是k0k1k2k3k4k5=101111。竖的红线右侧就是当前位ij。
初始状态当然是一个插头都没有就是k0k1k2k3k4k5=000000,结束状态也是000000。
转移时只要判当前格子(本图为第二行第三列的格子,当前状态是101111)左插头和上插头的状态,就能知道能够转移到哪些状态去。
现在就转移到了100011这种状态。
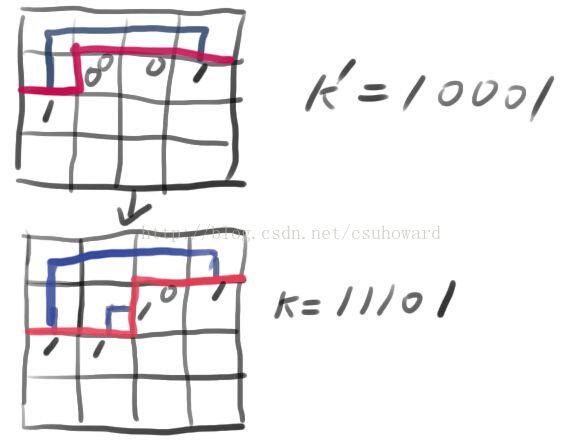
假如知道现在的状态,也可以通过先前的状态推得现在状态组数。
枚举现在的状态,枚举到第2行第2列的11101状态(我的状态左边是低位,11101存的时候就按10111存),当前状态可以由第2行第1列的10001转移得到。
其实用滚动数组可以只保存2*(1<<12)个状态就够了,时间复杂度为n*m*(1<<m)。
#include <cstdio>
#include <iostream>
#include <algorithm>
#include <cstring>
using namespace std;
typedef long long LL;
int const maxn = 12;
int g[maxn][maxn];
LL dp[2][1<<maxn];
int n,m;
void read(){
scanf("%d%d",&n,&m);
for(int i=1;i<=n;i++){
for(int j=1;j<=m;j++){
scanf("%d",&g[i][j]);
}
}
}
LL solve(){
read();
int cur=0;
memset(dp,0,sizeof dp);
dp[cur^1][0]=1;
for(int i=1;i<=n;i++){
memset(dp[cur],0,sizeof dp[cur]);
for(int k=(1<<m)-1;k>=0;k--){
dp[cur][k<<1]=dp[cur^1][k];
}
cur^=1;
for(int j=1;j<=m;j++,cur^=1){
int b=1<<(j-1);
int r=1<<j;
for(int k=(1<<(m+1))-1;k>=0;k--){
if(g[i][j]){
if(j!=m){
if((r&k) && (b&k)){//下方和右方都有插头
dp[cur][k]=dp[cur^1][k^b^r];
}else if((r&k) || (b&k)){//下方或右方一方有插头
dp[cur][k]=dp[cur^1][k]+dp[cur^1][k^b^r];
}else{//下方和右方都没插头
dp[cur][k]=dp[cur^1][k^b^r];
}
}else if(!(r&k)){
if(b&k){
dp[cur][k]=dp[cur^1][k]+dp[cur^1][k^b^r];
}else{
dp[cur][k]=dp[cur^1][k^r^b];
}
}else{
dp[cur][k]=0;
}
}else{
if((r&k)||(b&k)){//下方或右方有插头
dp[cur][k]=0;
}else{//没有插头
dp[cur][k]=dp[cur^1][k];
}
}
}
}
}
return dp[cur^1][0];
}
int main(){
int T;
LL ans;
scanf("%d",&T);
for(int cas=1;cas<=T;cas++){
ans = solve();
printf("Case %d: There are %I64d ways to eat the trees.\n",cas,ans);
}
return 0;
}