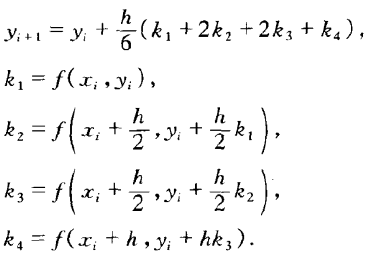matlab练习程序（龙格库塔法）

非刚性常微分方程的数值解法通常会用四阶龙格库塔算法，其matlab函数对应ode45。

对于dy/dx = f(x,y)，y(0)=y0。

其四阶龙格库塔公式如下：

对于通常计算，四阶已经够用，四阶以上函数f(x,y)计算工作量大大增加而精度提高较慢。

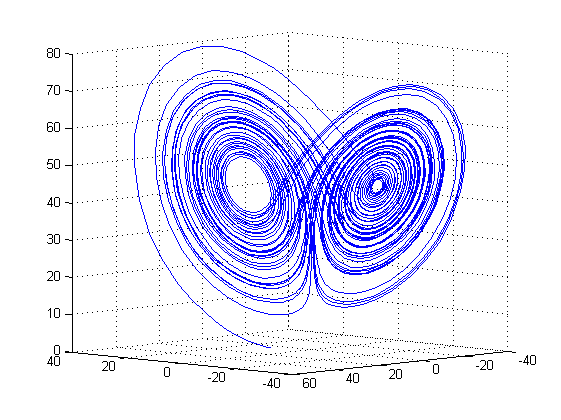
下面以龙格库塔法解洛伦兹方程为例：

matlab代码如下：

main.m：

clear all;
close all;
clc;

%系统龙格库塔法
[t,h] = ode45(@test_fun,[0 40],[12 4 0]);
plot3(h(:,1),h(:,2),h(:,3));
grid on;

%自定义龙格库塔法
[t1,h1]=runge_kutta(@test_fun,[12 4 0],0.01,0,40);
figure;
plot3(h1(1,:),h1(2,:),h1(3,:),'r')
grid on;

runge_kutta.m（函数参考网络）：

%参数表顺序依次是微分方程组的函数名称，初始值向量，步长，时间起点，时间终点（参数形式参考了ode45函数）
function [x,y]=runge_kutta(ufunc,y0,h,a,b)
n=floor((b-a)/h);       %步数
x(1)=a;                 %时间起点
y(:,1)=y0;              %赋初值，可以是向量，但是要注意维数
for i=1:n               %龙格库塔方法进行数值求解    
    x(i+1)=x(i)+h;    
    k1=ufunc(x(i),y(:,i));  
    k2=ufunc(x(i)+h/2,y(:,i)+h*k1/2);    
    k3=ufunc(x(i)+h/2,y(:,i)+h*k2/2);   
    k4=ufunc(x(i)+h,y(:,i)+h*k3);   
    y(:,i+1)=y(:,i)+h*(k1+2*k2+2*k3+k4)/6;
end

test_fun（洛伦兹方程）：

%构造微分方程
function dy=test_fun(t,y)
a = 16;
b = 4;
c = 45;

dy=[a*(y(2)-y(1));
    c*y(1)-y(1)*y(3)-y(2);
    y(1)*y(2)-b*y(3)];

得到很经典的洛伦兹吸引子，结果如下：

参考：

https://wenku.baidu.com/view/8211fbd428ea81c758f57893.html