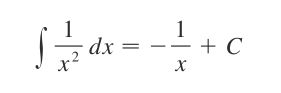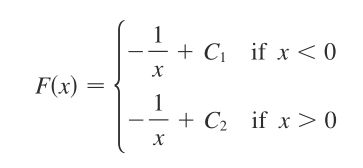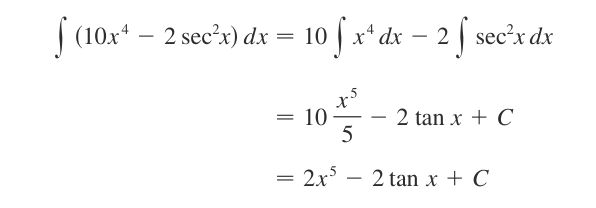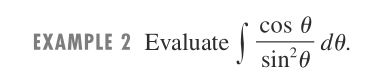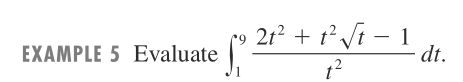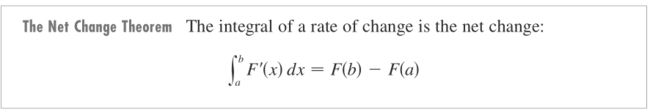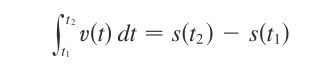- react native 之自定义顶部导航栏,实现标题居中可控
铁柱同学
ReactNativereactnative基础讲解react-native导航栏标题居中
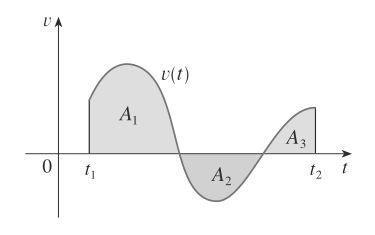
本来一直用的都是RN自带的组件react-navigation,但是后面需求有变,需要顶部导航栏目的标题可以自己控制,同一页面的标题根据数据库查出来的内容变化。因此自定义了一个导航栏目,实现需求。关于RN自带的顶部导航栏以及底部导航栏目,请看我的另一篇博客,地址:http://blog.csdn.net/ljfphp/article/details/77940284一、背景1、根据需求,要求点击列
- 2007. 从双倍数组中还原原数组
【算法题解析】还原双倍数组—从打乱的数组恢复原数组题目描述给定一个整数数组changed,该数组是通过对一个原始数组original的每个元素乘以2并打乱顺序后得到的。你的任务是判断给定的changed是否为某个original数组的双倍数组,并返回该原数组。具体来说,存在一个数组original,使得对original中的每个元素x,changed中都包含x和2*x两个元素(顺序可能被打乱)。如
- ASP .NET Core 8实现实时Web功能
发粪的屎壳郎
ASP.NETCore8后端服务框架搭建asp.netcore8signalr
ASP.NETCoreSignalR是一个开放源代码库,可用于简化向应用添加实时Web功能。实时Web功能使服务器端代码能够将内容推送到客户端。以下是ASP.NETCoreSignalR的一些主要功能:自动处理连接管理同时向所有连接的客户端发送消息。例如聊天室向特定客户端或客户端组发送消息SignalR支持以下用于处理实时通信的技术(按正常回退的顺序):WebSocketServer-Sent事件
- js运行,控制台显示乱码
wangsrc
javascriptlinux开发语言
"code-runner.executorMap":{ "javascript":"C:\\ProgramFiles\\nodejs\\node.exe" //node.js的安装路径}参考文章:https://blog.csdn.net/m0_63785629/article/details/129432129
- js 记录七 vscode+iis中文乱码
WMY10001
前端
1.其实就是html没有加2.其他配置的话,iis>.net全球化>文件utf-83.iis的localhost文件夹里的web.config,修改为:4.vscode右下角utf-85.点击vscode右下角utf-8,选择通过编码保存其实试了就第一条有效
- 复杂场景检测失效?陌讯多模态算法在千万级监控网的落地实战
2501_92473061
算法视觉检测安全计算机视觉
开篇痛点:安防监控的检测困境"明明人就在画面里,系统却毫无反应!"——这是某智慧园区安防负责人的吐槽。传统目标检测模型在安防监控场景面临三大死穴:漏报:夜间、遮挡场景下召回率骤降(实测ResNet50漏报率>40%)误报:树叶晃动、光影变化引发的误报占比超35%延迟:1080P视频流检测延迟普遍>100ms,难以满足实时响应需求技术解析:陌讯算法的三阶优化架构陌讯视觉算法采用多模态特征金字塔(MM
- Kubernetes K8S之资源控制器RC、RS、Deployment详解
LightZhang666
kubernetesci/cddevopskubernetesk8sdevopsci/cddocker
Kubernetes的资源控制器ReplicationController(RC)、ReplicaSet(RS)、Deployment(Deploy)详解与示例主机配置规划服务器名称(hostname)系统版本配置内网IP外网IP(模拟)k8s-masterCentOS7.72C/4G/20G172.16.1.11010.0.0.110k8s-node01CentOS7.72C/4G/20G172
- 设置第三方窗口置顶(SetWindowPos方法,vb.net)
大Mod_abfun
vb.net窗体活动调用.netvb.net窗口置顶
起源在日常办公、游戏时,我们经常需要一些窗口处于置顶状态,而这些窗口往往是网页端(浏览器)、办公软件(wps、office等),这些需要置顶的窗口往往自身没有明显的置顶开关,因此,想要让窗口一直处于顶端我们介绍一种有效的方法。在自己窗体内部Me.TopMost=True那么我们需要在第三方窗口呢?思路step1获取窗口的句柄,我们可以通过窗口的坐标来判断窗口的句柄,使用WindowFromPoin
- Kubernetes 常用命令
程序员的世界你不懂
《容器》kubernetes容器云原生
Kubernetes通过KubeApiserver作为整个集群管理的入口。Apiserver是整个集群的主管理节点,用户通过Apiserver配置和组织集群,同时集群中各个节点同etcd存储的交互也是通过Apiserver进行交互。Apiserver实现了一套RESTful的接口,用户可以直接使用API同Apiserver交互。另外官方还提供了一个客户端kubectl随工具集打包,用于可直接通过k
- Tcpdump使用
一介绍tcpdump,是Linux/Unix系统下强大的网络抓包工具,能够捕获和分析网络流量。用简单的语言概括就是dumpthetrafficonanetwork,是一个运行在linux平台可以根据使用者需求对网络上传输的数据包进行捕获的抓包工具,windows平台有sniffer等工具,tcpdump可以将网络中传输的数据包的“包头”全部捕获过来进程分析,其支持网络层、特定的传输协议、数据发送和
- # 检测 COM 服务器在线状态
胡八一、
报错解决服务器qt运维
适用场景OPCDA/OPCAE等基于DCOM的工业软件巡检自动化部署脚本中批量验证远程COM组件是否可用Windows服务开机自检1.背景在工业控制与运维场景下,我们经常需要判断某台机器上的COM/DCOM服务器(例如OPCServer)是否存活,并在掉线时及时告警或自动重连。.NET自带的System.Type.GetTypeFromProgID/Activator.CreateInstance
- Jfinal+SQLite java工具类复制mysql表数据到 *.sqlite
秋林辉
sqlitejavamysql
处理了时间类型packagechangeDataBase;importjava.sql.*;importjava.util.ArrayList;importjava.util.Iterator;importjava.util.List;publicclassMySQLToSQLiteMigration{privatestaticfinalStringMYSQL_URL="jdbc:mysql://
- 容器化技术:Kubernetes(k8s)、Pod、Docker容器
人工干智能
Docker的高级知识kubernetesdocker容器
三个相关的容器化技术Kubernetes(k8s)、Pod、Docker容器在容器化技术领域各自扮演着不同的角色,它们之间既存在区别又相互联系。Kubernetes(k8s)定义:Kubernetes是一个开源的容器编排平台,用于自动化部署、扩展和管理容器化应用程序。功能:提供了强大的工具和功能,如服务发现、负载均衡、自动伸缩、滚动更新等,帮助用户更高效地管理复杂的容器环境。架构:基于控制论和反馈
- Linux检测远程端口是否打开的六种方法
岚天start
运维网络linuxssh网络
Linux检测远程端口是否打开的六种方法服务器环境:[root@java-test-server~]#cat/etc/redhat-releaseCentOSLinuxrelease7.6.1810(Core)方法一:Telnet命令【yum-yinstalltelnet】#端口通显示如下(实验IP为自定义IP)[root@java-test-server~]#telnet88.88.88.888
- 【C# in .NET】18. 探秘接口:契约精神
阿蒙Armon
C#in.NETc#.netjava
探秘接口:契约精神在C#类型系统中,接口扮演着“契约”与“能力”的双重角色,它既是代码抽象的核心工具,也是.NET运行时(CLR)类型系统的重要组成部分。本文将穿透接口的语法表象,从IL代码结构、CLR类型系统实现、方法调度机制三个维度,全面揭示接口的底层工作原理,并结合框架设计实践提炼接口使用的精髓。一、接口的底层本质:并非只是“纯抽象类”接口在C#语法中表现为方法签名的集合,但在CLR层面有着
- 三步解锁.NET Conf Student Zone:免费资源+实战项目全攻略!学生党必看!
关注墨瑾轩,带你探索编程的奥秘!超萌技术攻略,轻松晋级编程高手技术宝库已备好,就等你来挖掘订阅墨瑾轩,智趣学习不孤单即刻启航,编程之旅更有趣.NETConfStudentZone三步称王第一步:注册与资源获取——“领取你的魔法钥匙”目标:用StudentZone的免费资源,告别“资源散落”困境。步骤1:注册账号(1分钟搞定!)//模拟注册流程(伪代码,实际需访问官网)stringemail="yo
- 观察者模式
午觉不眠Orz
一.概念当对象间存在一对多关系时,则使用观察者模式(ObserverPattern)。比如,当一个对象被修改时,则会自动通知它的依赖对象。定义:Defineaone-to-manydependencybetweenobjectssothatwhenoneobjectchangesstate,allitsdependentsarenotifiedandupdatedautomatically.意图:
- 深度学习——CNN(3)
飘涯
前言:前面介绍了最基本的Lenet,下面介绍几种其他的网络结构CNN-AlexNet网络结构如下图:从图中可以看出,采用双gpu训练增加LRN归一化层:本质上,这个层也是为了防止激活函数的饱和的。采用dropout防止过拟合基于AlexNet进行微调,诞生了ZF-netCNN-GoogleNetGoogLeNet借鉴了NIN的特性,在原先的卷积过程中附加了11的卷积核加上ReLU激活。这不仅仅提升
- 【C# in .NET】17. 探秘类成员-构造函数与析构函数:对象生命周期管理
阿蒙Armon
C#in.NETc#.netjava
探秘类成员-构造函数与析构函数:对象生命周期管理在C#的类成员体系中,构造函数与析构函数承担着对象生命周期管理的核心职责。它们看似简单,却蕴含着与.NET运行时(CLR)深度交互的底层逻辑。本文将从IL代码解析、内存操作机制、CLR调度原理三个维度,全面揭秘这对特殊成员的工作原理,并结合实战场景提炼最佳实践。一、构造函数:对象诞生的幕后推手构造函数是类实例化过程中执行初始化操作的特殊方法,它的底层
- 【C# in .NET】9. 探秘委托:函数抽象的底层机制
阿蒙Armon
C#in.NETc#.netjava
探秘委托:函数抽象的底层机制在C#的类型系统中,委托(Delegate)作为函数的抽象容器,架起了面向对象与函数式编程的桥梁。它不仅是事件驱动编程的核心,更是LINQ、异步编程等现代C#特性的基础。与类和结构体相比,委托的底层实现融合了引用类型的内存管理与函数指针的调用特性,涉及CLR对方法调度的深度优化。本文将从IL指令解析到JIT编译细节,全面揭示委托的本质机制,带你理解这一特殊类型如何在.N
- 【C# in .NET】2. 探秘数据类型:构建程序的基石
阿蒙Armon
C#in.NETc#.netjava
探秘数据类型:构建程序的基石在C#编程中,数据类型是构建程序的基石。无论是简单的整数计算还是复杂的对象交互,都离不开对数据类型的精准运用。然而,大多数开发者对数据类型的理解往往停留在表面语法层面,忽视了其在.NET框架底层的运行机制。本文将从CLR(公共语言运行时)的视角,深入剖析C#数据类型的本质、内存布局与核心机制,带你看透数据类型背后的底层逻辑。一、数据类型的本质:CLR类型系统的双轨制.N
- 久远的操作系统笔记3
锦绣拾年
信号变量,条件变量,互斥锁解析来自:http://blog.chinaunix.net/space.php?uid=23061624&do=blog&cuid=2127853http://blog.163.com/huangguoqiang_123@126/blog/static/141043114201349112849554/信号量用在多线程多任务同步的,一个线程完成了某一个动作就通过信号量告
- 尝试is制作轮播图
鸡蛋卷1619
图片转换2letarr=["http://p1.music.126.net/wBMu9w9U8o7k4CDssm5FDg==/109951166684652624.jpg?imageView&quality=89","http://p1.music.126.net/82byaQmflAHb77TFu5l5HQ==/109951166682139804.jpg?imageView&quality=8
- Docker+Kubernetes落地指南:从单机到集群的平滑迁移
sg_knight
dockerdockerkubernetes容器javaspringcloud
一、为何必须升级到Kubernetes?1.1单机Docker的瓶颈单机环境痛点:├─资源利用率不均衡(CPU飙高vs内存闲置)├─服务扩容需手动操作├─零宕机更新难以实现└─网络配置复杂(跨主机通信困难)企业级需求:┌───────────┬───────────────┐|场景|Kubernetes方案||───────────|───────────────||滚动更新|Deployment
- gin数据解析和绑定
rit8432499
giniphoneios
go代码packagemainimport("net/http""github.com/gin-gonic/gin")//定义接收JSON数据的结构体typeLoginstruct{Usernamestring`form:"username"json:"username"uri:"username"xml:"username"binding:"required"`Passwordstring`fo
- 通过 Docker 和 Kubernetes 部署前后端代码到服务器
目录通过Docker和Kubernetes部署前后端代码到服务器一、准备工作二、创建Docker镜像三、部署到Kubernetes四、访问应用程序五、总结在现代软件开发中,Docker和Kubernetes已成为部署应用程序的强大工具。它们提供了一种可靠、可扩展和高效的方式来将前后端代码部署到服务器上。本文将介绍如何使用Docker和Kubernetes来部署前后端代码。一、准备工作安装Docke
- IoC容器深度解析:架构、原理与实现
liulilittle
MarkdownExtensionC#架构网络c#IoCDI依赖倒置依赖注入
IoC容器深度解析:架构、原理与实现引用:.NETIoC容器原理与实现等巫山的雲彩都消散撒下的碧色如何看淡一、引言:从服务定位器到IoC的演进类签名修改集中式管理依赖反转+动态注入强耦合直接依赖全量代码重构服务定位器模式圈复杂度指数级增长IoC容器方案低耦合+高扩展性历史痛点在早期软件开发中(如2000年代JavaEE体系),开发者面临两大核心问题:强耦合依赖链:上层模块直接实例化下层对象(new
- HPE携手迈阿密自由公园,为迈阿密国际足球俱乐部打造极致球迷体验
近日,慧与科技(NYSE:HPE)宣布与迈阿密自由公园(MiamiFreedomPark)及迈阿密国际足球俱乐部(InterMiamiCF,后简称迈阿密国际)正式达成合作协议。HPE将成为迈阿密自由公园的首个创始合作伙伴和官方技术合作伙伴,及迈阿密国际在网络基础设施、解决方案、混合云等领域的独家合作伙伴。届时,HPE将凭借HPEArubaNetworking、HPEAlletraStorage、H
- Python - 数据分析三剑客之Pandas
MinggeQingchun
PythonPythonPandas
阅读前可参考NumPy文章https://blog.csdn.net/MinggeQingchun/article/details/148253682https://blog.csdn.net/MinggeQingchun/article/details/148253682Pandas是Python中一个强大的开源数据分析库,专门用于处理结构化数据(如表格、时间序列等),其核心数据结构为Seri
- python网络爬虫(第一章/共三章:网络爬虫库、robots.txt规则(防止犯法)、查看获取网页源代码)
python网络爬虫(第一章/共三章:网络爬虫库、robots.txt规则(防止犯法)、查看获取网页源代码)学习python网络爬虫的完整路径:(第一章即此篇文章)(第二章)python网络爬虫(第二章/共三章:安装浏览器驱动,驱动浏览器加载网页、批量下载资源)-CSDN博客https://blog.csdn.net/2302_78022640/article/details/149431071?
- mysql主从数据同步
林鹤霄
mysql主从数据同步
配置mysql5.5主从服务器(转)
教程开始:一、安装MySQL
说明:在两台MySQL服务器192.168.21.169和192.168.21.168上分别进行如下操作,安装MySQL 5.5.22
二、配置MySQL主服务器(192.168.21.169)mysql -uroot -p &nb
- oracle学习笔记
caoyong
oracle
1、ORACLE的安装
a>、ORACLE的版本
8i,9i : i是internet
10g,11g : grid (网格)
12c : cloud (云计算)
b>、10g不支持win7
&
- 数据库,SQL零基础入门
天子之骄
sql数据库入门基本术语
数据库,SQL零基础入门
做网站肯定离不开数据库,本人之前没怎么具体接触SQL,这几天起早贪黑得各种入门,恶补脑洞。一些具体的知识点,可以让小白不再迷茫的术语,拿来与大家分享。
数据库,永久数据的一个或多个大型结构化集合,通常与更新和查询数据的软件相关
- pom.xml
一炮送你回车库
pom.xml
1、一级元素dependencies是可以被子项目继承的
2、一级元素dependencyManagement是定义该项目群里jar包版本号的,通常和一级元素properties一起使用,既然有继承,也肯定有一级元素modules来定义子元素
3、父项目里的一级元素<modules>
<module>lcas-admin-war</module>
<
- sql查地区省市县
3213213333332132
sqlmysql
-- db_yhm_city
SELECT * FROM db_yhm_city WHERE class_parent_id = 1 -- 海南 class_id = 9 港、奥、台 class_id = 33、34、35
SELECT * FROM db_yhm_city WHERE class_parent_id =169
SELECT d1.cla
- 关于监听器那些让人头疼的事
宝剑锋梅花香
画图板监听器鼠标监听器
本人初学JAVA,对于界面开发我只能说有点蛋疼,用JAVA来做界面的话确实需要一定的耐心(不使用插件,就算使用插件的话也没好多少)既然Java提供了界面开发,老师又要求做,只能硬着头皮上啦。但是监听器还真是个难懂的地方,我是上了几次课才略微搞懂了些。
- JAVA的遍历MAP
darkranger
map
Java Map遍历方式的选择
1. 阐述
对于Java中Map的遍历方式,很多文章都推荐使用entrySet,认为其比keySet的效率高很多。理由是:entrySet方法一次拿到所有key和value的集合;而keySet拿到的只是key的集合,针对每个key,都要去Map中额外查找一次value,从而降低了总体效率。那么实际情况如何呢?
为了解遍历性能的真实差距,包括在遍历ke
- POJ 2312 Battle City 优先多列+bfs
aijuans
搜索
来源:http://poj.org/problem?id=2312
题意:题目背景就是小时候玩的坦克大战,求从起点到终点最少需要多少步。已知S和R是不能走得,E是空的,可以走,B是砖,只有打掉后才可以通过。
思路:很容易看出来这是一道广搜的题目,但是因为走E和走B所需要的时间不一样,因此不能用普通的队列存点。因为对于走B来说,要先打掉砖才能通过,所以我们可以理解为走B需要两步,而走E是指需要1
- Hibernate与Jpa的关系,终于弄懂
avords
javaHibernate数据库jpa
我知道Jpa是一种规范,而Hibernate是它的一种实现。除了Hibernate,还有EclipseLink(曾经的toplink),OpenJPA等可供选择,所以使用Jpa的一个好处是,可以更换实现而不必改动太多代码。
在play中定义Model时,使用的是jpa的annotations,比如javax.persistence.Entity, Table, Column, OneToMany
- 酸爽的console.log
bee1314
console
在前端的开发中,console.log那是开发必备啊,简直直观。通过写小函数,组合大功能。更容易测试。但是在打版本时,就要删除console.log,打完版本进入开发状态又要添加,真不够爽。重复劳动太多。所以可以做些简单地封装,方便开发和上线。
/**
* log.js hufeng
* The safe wrapper for `console.xxx` functions
*
- 哈佛教授:穷人和过于忙碌的人有一个共同思维特质
bijian1013
时间管理励志人生穷人过于忙碌
一个跨学科团队今年完成了一项对资源稀缺状况下人的思维方式的研究,结论是:穷人和过于忙碌的人有一个共同思维特质,即注意力被稀缺资源过分占据,引起认知和判断力的全面下降。这项研究是心理学、行为经济学和政策研究学者协作的典范。
这个研究源于穆来纳森对自己拖延症的憎恨。他7岁从印度移民美国,很快就如鱼得水,哈佛毕业
- other operate
征客丶
OSosx
一、Mac Finder 设置排序方式,预览栏 在显示-》查看显示选项中
二、有时预览显示时,卡死在那,有可能是一些临时文件夹被删除了,如:/private/tmp[有待验证]
--------------------------------------------------------------------
若有其他凝问或文中有错误,请及时向我指出,
我好及时改正,同时也让我们一
- 【Scala五】分析Spark源代码总结的Scala语法三
bit1129
scala
1. If语句作为表达式
val properties = if (jobIdToActiveJob.contains(jobId)) {
jobIdToActiveJob(stage.jobId).properties
} else {
// this stage will be assigned to "default" po
- ZooKeeper 入门
BlueSkator
中间件zk
ZooKeeper是一个高可用的分布式数据管理与系统协调框架。基于对Paxos算法的实现,使该框架保证了分布式环境中数据的强一致性,也正是基于这样的特性,使得ZooKeeper解决很多分布式问题。网上对ZK的应用场景也有不少介绍,本文将结合作者身边的项目例子,系统地对ZK的应用场景进行一个分门归类的介绍。
值得注意的是,ZK并非天生就是为这些应用场景设计的,都是后来众多开发者根据其框架的特性,利
- MySQL取得当前时间的函数是什么 格式化日期的函数是什么
BreakingBad
mysqlDate
取得当前时间用 now() 就行。
在数据库中格式化时间 用DATE_FORMA T(date, format) .
根据格式串format 格式化日期或日期和时间值date,返回结果串。
可用DATE_FORMAT( ) 来格式化DATE 或DATETIME 值,以便得到所希望的格式。根据format字符串格式化date值:
%S, %s 两位数字形式的秒( 00,01,
- 读《研磨设计模式》-代码笔记-组合模式
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
import java.util.ArrayList;
import java.util.List;
abstract class Component {
public abstract void printStruct(Str
- 4_JAVA+Oracle面试题(有答案)
chenke
oracle
基础测试题
卷面上不能出现任何的涂写文字,所有的答案要求写在答题纸上,考卷不得带走。
选择题
1、 What will happen when you attempt to compile and run the following code? (3)
public class Static {
static {
int x = 5; // 在static内有效
}
st
- 新一代工作流系统设计目标
comsci
工作算法脚本
用户只需要给工作流系统制定若干个需求,流程系统根据需求,并结合事先输入的组织机构和权限结构,调用若干算法,在流程展示版面上面显示出系统自动生成的流程图,然后由用户根据实际情况对该流程图进行微调,直到满意为止,流程在运行过程中,系统和用户可以根据情况对流程进行实时的调整,包括拓扑结构的调整,权限的调整,内置脚本的调整。。。。。
在这个设计中,最难的地方是系统根据什么来生成流
- oracle 行链接与行迁移
daizj
oracle行迁移
表里的一行对于一个数据块太大的情况有二种(一行在一个数据块里放不下)
第一种情况:
INSERT的时候,INSERT时候行的大小就超一个块的大小。Oracle把这行的数据存储在一连串的数据块里(Oracle Stores the data for the row in a chain of data blocks),这种情况称为行链接(Row Chain),一般不可避免(除非使用更大的数据
- [JShop]开源电子商务系统jshop的系统缓存实现
dinguangx
jshop电子商务
前言
jeeshop中通过SystemManager管理了大量的缓存数据,来提升系统的性能,但这些缓存数据全部都是存放于内存中的,无法满足特定场景的数据更新(如集群环境)。JShop对jeeshop的缓存机制进行了扩展,提供CacheProvider来辅助SystemManager管理这些缓存数据,通过CacheProvider,可以把缓存存放在内存,ehcache,redis,memcache
- 初三全学年难记忆单词
dcj3sjt126com
englishword
several 儿子;若干
shelf 架子
knowledge 知识;学问
librarian 图书管理员
abroad 到国外,在国外
surf 冲浪
wave 浪;波浪
twice 两次;两倍
describe 描写;叙述
especially 特别;尤其
attract 吸引
prize 奖品;奖赏
competition 比赛;竞争
event 大事;事件
O
- sphinx实践
dcj3sjt126com
sphinx
安装参考地址:http://briansnelson.com/How_to_install_Sphinx_on_Centos_Server
yum install sphinx
如果失败的话使用下面的方式安装
wget http://sphinxsearch.com/files/sphinx-2.2.9-1.rhel6.x86_64.rpm
yum loca
- JPA之JPQL(三)
frank1234
ormjpaJPQL
1 什么是JPQL
JPQL是Java Persistence Query Language的简称,可以看成是JPA中的HQL, JPQL支持各种复杂查询。
2 检索单个对象
@Test
public void querySingleObject1() {
Query query = em.createQuery("sele
- Remove Duplicates from Sorted Array II
hcx2013
remove
Follow up for "Remove Duplicates":What if duplicates are allowed at most twice?
For example,Given sorted array nums = [1,1,1,2,2,3],
Your function should return length
- Spring4新特性——Groovy Bean定义DSL
jinnianshilongnian
spring 4
Spring4新特性——泛型限定式依赖注入
Spring4新特性——核心容器的其他改进
Spring4新特性——Web开发的增强
Spring4新特性——集成Bean Validation 1.1(JSR-349)到SpringMVC
Spring4新特性——Groovy Bean定义DSL
Spring4新特性——更好的Java泛型操作API
Spring4新
- CentOS安装Mysql5.5
liuxingguome
centos
CentOS下以RPM方式安装MySQL5.5
首先卸载系统自带Mysql:
yum remove mysql mysql-server mysql-libs compat-mysql51
rm -rf /var/lib/mysql
rm /etc/my.cnf
查看是否还有mysql软件:
rpm -qa|grep mysql
去http://dev.mysql.c
- 第14章 工具函数(下)
onestopweb
函数
index.html
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/
- POJ 1050
SaraWon
二维数组子矩阵最大和
POJ ACM第1050题的详细描述,请参照
http://acm.pku.edu.cn/JudgeOnline/problem?id=1050
题目意思:
给定包含有正负整型的二维数组,找出所有子矩阵的和的最大值。
如二维数组
0 -2 -7 0
9 2 -6 2
-4 1 -4 1
-1 8 0 -2
中和最大的子矩阵是
9 2
-4 1
-1 8
且最大和是15
- [5]设计模式——单例模式
tsface
java单例设计模式虚拟机
单例模式:保证一个类仅有一个实例,并提供一个访问它的全局访问点
安全的单例模式:
/*
* @(#)Singleton.java 2014-8-1
*
* Copyright 2014 XXXX, Inc. All rights reserved.
*/
package com.fiberhome.singleton;
- Java8全新打造,英语学习supertool
yangshangchuan
javasuperword闭包java8函数式编程
superword是一个Java实现的英文单词分析软件,主要研究英语单词音近形似转化规律、前缀后缀规律、词之间的相似性规律等等。Clean code、Fluent style、Java8 feature: Lambdas, Streams and Functional-style Programming。
升学考试、工作求职、充电提高,都少不了英语的身影,英语对我们来说实在太重要