- 初试牛刀 - 使用 Chaos Mesh 进行第一次混沌实验
weixin_42587823
混沌混沌工程
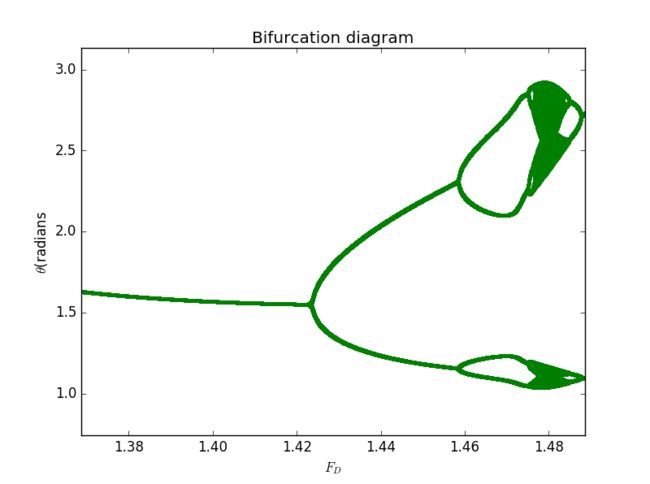
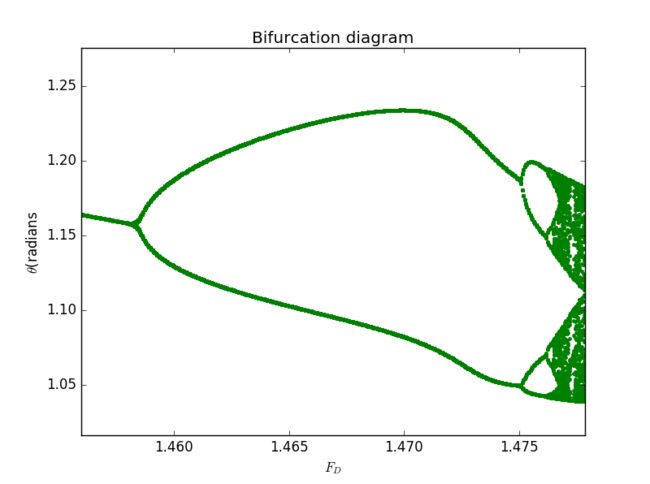
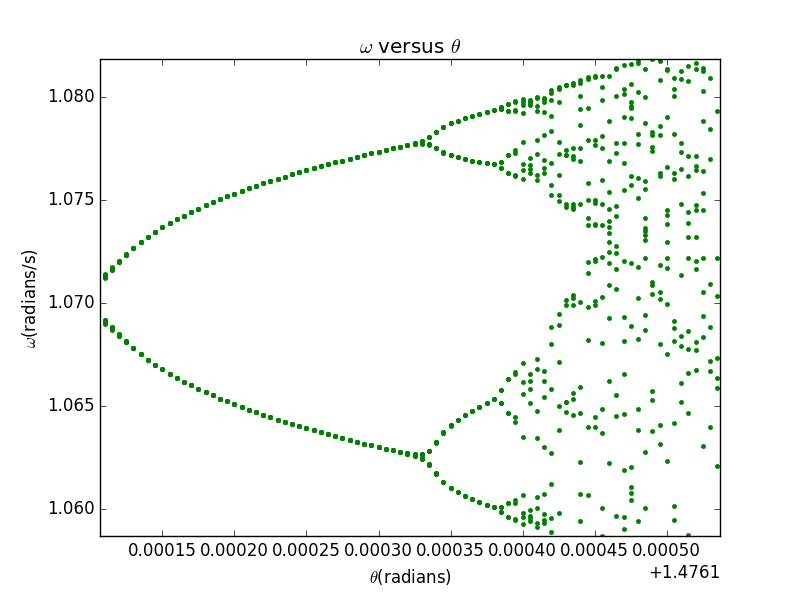
初试牛刀-使用ChaosMesh进行第一次混沌实验第一步:准备实验环境我们的“混沌实验室”需要三个核心组件:一个Kubernetes集群、ChaosMesh平台、以及一个用来做实验的应用。A.安装ChaosMesh我们将使用Helm来安装ChaosMesh,这是官方推荐的最简单的方式。添加ChaosMesh的Helm仓库:helmrepoaddchaos-meshhttps://charts.ch
- 【Pandas】pandas DataFrame resample
liuweidong0802
DataFramepandas
Pandas2.2DataFrameTimeSeries-related方法描述DataFrame.asfreq(freq[,method,how,…])用于**将时间序列数据转换为指定频率(resampletofrequency)**的方法DataFrame.asof(where[,subset])用于查找时间序列中最接近指定时间点的非NaN值的方法DataFrame.shift([period
- Pandas 中的 Period 对象
闯闯桑
pythonnumpy开发语言
在Pandas中,Period对象用于表示特定的时间区间(如某个月、某个季度),而不是时间点(后者用Timestamp)。它是处理时间序列数据的重要工具,尤其适合需要按固定周期(如月度、季度)分析的场景。核心概念与Timestamp的区别:Timestamp表示具体时刻(如2023-01-0100:00:00)。Period表示时间区间(如2023年1月整月)。关键属性:start_time:周期
- 建议收藏,22个Python迷你项目(附源码)_python做的简单项目
2401_84141293
程序员python开发语言
alarm_hour=alarm_time[0:2]alarm_minute=alarm_time[3:5]alarm_seconds=alarm_time[6:8]alarm_period=alarm_time[9:11].upper()print(“Settingupalarm…”)whileTrue:now=datetime.now()current_hour=now.strftime(“%
- 《vue3基础知识》学习笔记Day34-(Period2)进阶 API
sameen1900
学习vue.jsjavascript
渲染函数APIh()创建虚拟DOM节点(vnode)。第一个参数既可以是一个字符串(用于原生元素)也可以是一个Vue组件定义。第二个参数是要传递的prop,第三个参数是子节点。当创建一个组件的vnode时,子节点必须以插槽函数进行传递。如果组件只有默认槽,可以使用单个插槽函数进行传递。否则,必须以插槽函数的对象形式来传递。为了方便阅读,当子节点不是插槽对象时,可以省略prop参数。import{h
- esp32+IDF V5.1.1版本编译freertos报错
嵌入式软硬件叶玄
ESP32入门教程esp32
error:'portTICK_RATE_MS'undeclared(firstuseinthisfunction);didyoumean'portTICK_PERIOD_MS解决方法:使用命令idf.pymenuconfig打开配置界面配置freeRtos使能configENABLE_BACKWARD_COMPATIBLITY
- [Timer] 02.QEMU 定时器 Ptimer
MaoXian_n
QEMU嵌入式硬件驱动开发linux
目录1.简介2.定时器策略-PTIMER_POLICY3.定时器结构-structptimer_state4.创建定时器-ptimer_init()5.设置时钟周期5.1.按固定值设置时钟周期-ptimer_set_period()5.2.按频率设置时钟周期-ptimer_set_freq()5.3.从已有时钟获取周期-ptimer_set_period_from_clock()6.定时器接口6.
- grafana/loki-stack 设置日志保存时间及自动清理
时空无限
lokigrafana
Loki本身不提供命令行或HTTPAPI删除特定日志,但你可以通过配置retention_period来实现日志自动清理。loki:config:table_manager:retention_deletes_enabled:trueretention_period:168h#保留7天适用于boltdb-shipper或chunkstore。如果你用的是filesystem模式,还可以这样:lok
- MySQL日期时间及字符串处理函数全面解析
May Wei
MySQL日期时间处理字符串操作函数数据格式化
MySQL日期时间及字符串处理函数全面解析MySQL是一个功能强大的关系型数据库管理系统,它提供了丰富的函数来处理日期时间以及字符串,从而满足数据存储、查询和格式化的各种需求。本文将探讨MySQL中的一些基本且常用的日期时间与字符串处理函数,并通过实例加深理解。MySQL中的日期时间处理函数MySQL提供了多种处理日期时间的函数,包括但不限于以下几种:1.计算日期时间差PERIOD_DIFF函数用
- 如何在ccs快速使用感为八路灰度传感器
不想干活的电工张
c语言
声明:此文章方便新手快速使用感为灰度传感器,代码是从官方文章和例程总结下来的。官方提供三种方法,第一种用的gpio过多,第三种iic对于新手不友好,第二种,串行读取数据,我个人认为最方便上手。1、在系统文件上配置gpio口(CLK,DAT),gpio口可以随便选,CLK输出,DAT输入,其他默认选项。打开嘀嗒计时器(systick),配置到1us(period=32)。2、配置board.c文件,
- java读取通达信day_通达信,获得当前日期(示例代码)
联想小新笔记本
java读取通达信day
DRAWTEXT(ISNEWDAY,P_OBV,VAR2STR(DATE+19000000-20170000,0));函数名简称详解用法PERIOD周期取得周期类型.结果从0到11,依次分别是1/5/15/30/60分钟,日/周/月,多分钟,多日,季,年.DATE日期取得该周期从1900以来的的年月日.DATE例如函数返回1000101,表示2000年1月1日,DATE+19000000后才是真正
- mysql sql 解锁表_SQL 解锁表
夜空中的UFO恋曲
mysqlsql解锁表
JavaBasic-Generics什么是类型擦除?类型擦除指的是通过类型参数合并,将泛型类型实例关联到同一份字节码上.编译器只为泛型类型生成一份字节码,并将其实例关联到这份字节码上.类型擦除的关键在于从泛型类型中清除类型参数的相关信...[iOSUI进阶-6.1]核心动画CoreAnimationA.基本知识1.概念CoreAnimation是一组非常强大的
- 【java】jdk8及以后的时间类总结
jstart千语
Javajava开发语言时间类
目录1.LocalDate2.LocalTime4.ZonedDateTime5.Duration6.Period7.DateTimeFormatter1.LocalDate说明:表示不带时区的日期(年、月、日),不可变且线程安全。importjava.time.LocalDate;publicclassLocalDateExample{publicstaticvoidmain(String[]a
- Java—— 常见API介绍 第五期
举一个梨子zz
java开发语言APIintellij-idea
JDK8以后新增的时间相关类Date类ZoneId:时区Instant:时间戳ZoneDateTime:带时区的时间日期格式化类SimpleDateFormatDateTimeFormatter:用于时间的格式化和解析日历类CalendarLocalDate:年、月、日LocalTime:时、分、秒LocalDateTime:年、月、日、时、分、秒工具类Period:时间间隔(年,月,日)Dura
- 华为OD机试 - 批量处理任务 - 二分查找(Python/JS/C/C++ 2025 A卷 100分)
哪 吒
华为odpythonjavascript华为OD机试2025A卷
华为OD机试2025A卷题库疯狂收录中,刷题点这里专栏导读本专栏收录于《华为OD机试真题(Python/JS/C/C++)》。刷的越多,抽中的概率越大,私信哪吒,备注华为OD,加入华为OD刷题交流群,每一题都有详细的答题思路、详细的代码注释、3个测试用例、为什么这道题采用XX算法、XX算法的适用场景,发现新题目,随时更新。一、题目描述某实验室计算机待处理任务以[start,end,period]格
- html字母转化ascii码,HTML中特殊字符和与之对应的ASCII代码
weixin_39693662
html字母转化ascii码
【转载】App.config/Web.config中特殊字符的处理写一个网站,遇到一个问题,发布以后,提示错误,但是即使打开错误提示(在web.config中打开),还是只提示错误,没提示什么地方错误,这让我知道了:是webconfig本身的错误,经过排除,是链接字...App.config/Web.config中特殊字符的处
- ExoPlayer 中的 Timeline、Period 和 Window
说码解字
实时音视频
ExoPlayer的Timeline系统设计用于抽象化媒体内容的组织方式,无论是点播视频、直播流还是多内容播放列表。Timeline提供了一个统一的方式来描述内容的时间范围、结构和元数据,而Timeline.Period和Timeline.Window分别是其更细粒度的子结构。TimelineTimeline是ExoPlayer的抽象基类,表示媒体内容的整体时间轴结构。它包含一组窗口(Window
- 时间工具类(简单版)拿来就用
假客套
java工具类java开发语言
importjava.text.ParseException;importjava.text.SimpleDateFormat;importjava.time.LocalDate;importjava.time.Period;importjava.util.Date;importstaticcn.hutool.core.date.LocalDateTimeUtil.parseDate;/***时间
- UE5 Chaos :官方文献总结 + 渲染网格体 (Render Mesh) 和模拟网格体 是如何关联的?为什么模拟网格体 可以驱动渲染网格体?
子燕若水
3DUE5前端
官方文献:https://dev.epicgames.com/community/learning/tutorials/pv7x/unreal-engine-panel-cloth-editor1.流程概述本文档介绍了如何通过面板编辑器(PanelEditor)在UnrealEngine中生成基于面板的布料资源。流程主要包含从MarvelousDesigner导出静态网格(StaticMesh),
- spring断言和java断言区别_Spring-----Assert断言工具类
weixin_39743414
Spring的断言工具类Assert的基本使用org.springframework.util.Assert;Assert工具类,通常用于数据合法性检查.平时做判断通常都是这样写:if(message==null||message....java编程中的断言工具类(org.springframework.util.Assert)转自:https://blo
- linux c语言打印hello,C语言——打印“Hello World!”,这么简单?
彭浩翔
linuxc语言打印hello
iOS开发系列--C语言之数组和字符串概览数组在C语言中有着特殊的地位,它有很多特性,例如它的存储是连续的,数组的名称就是数组的地址等.而在C语言中是没有String类型的,那么如果要表示一个字符串,就必须使用字符数组.今天主要就介绍如...java.lang.NoClassDefFoundError:异常信息:十一月10,20165:20:15下午org.
- Chaos Mesh实战:手把手教你用“混沌魔法”暴打K8s集群
码农技术栈
kubernetes容器云原生springbootspringcloud微服务架构
从网络延迟到Pod爆炸,这个开源工具让你的分布式系统真正“抗揍”一、为什么你的系统需要“混沌工程”?想象一下:你的Kubernetes集群运行着核心业务,突然某个节点宕机、网络延迟飙升、Pod莫名其妙消失…如果线上真发生这些故障,你的系统能扛住吗?混沌工程(ChaosEngineering)就是通过主动注入故障,提前暴露系统脆弱点的“压力测试”。而ChaosMesh(由PingCAP开源)是目前最
- Julia 日期和时间
jeeper88
julia开发语言
Julia通过Dates模块提供了以下三个函数来处理日期和时间:Date:表示日期,精确到日,只显示日期。DateTime:表示日期和时间,精确到毫秒。DateTime:表示日时间,精确到纳秒,代表一天24小时中的特定时刻。使用前,我们需要先导入Dates模块:importDatesDate和DateTime类型可以通过整数或Period类型解析。Period基于日期值,表示年、月、日等:Peri
- 10个工具让你秒变数字艺术家,小白也能画出百万点赞神图
ivwdcwso
AIAI绘画副业变现Midjourney教程
一、新手必看:3步出神图的核心心法1.咒语炼金术(Prompt魔法公式)#万能结构:画风+主体+细节+神级后缀"8kCG,(赛博朋克美少女:1.3),霓虹机械臂,雨夜东京街景,宫崎骏风格--ar16:9--niji6--style4b"2.参数调教秘籍参数效果推荐值--ar画面比例9:16(手机壁纸)--chaos创意随机性30-80(越高越抽象)--stylize艺术化程度500-1000(漫画
- phoenix无法连接hbase shell创建表失败_报错_PleaseHoldException: Master is initializing---记录020_大数据工作笔记0180
添柴程序猿
hbase连接报错phoenix连接hbasephoenixPleaseHoldExcep
今天发现,我的phoenix,去连接hbase集群,怎么也连不上了,奇怪了...弄了一晚上org.apache.hadoop.hbase.PleaseHoldException:Masterisinitializing[root@hadoop120bin]#ll总用量184-rwxr-xr-x.1rootroot36371月222020chaos-daemon.sh-rwxr-xr-x.1root
- STM32学习笔记——TIM_Period 和 TIM_Prescaler
优雅的造轮狮
嵌入式学习笔记单片机嵌入式硬件
概念TIM_TimeBaseStructure.TIM_Period和TIM_TimeBaseStructure.TIM_Prescaler是STM32定时器(Timer)的两个重要参数。TIM_Period:这个参数代表的是定时器的自动重装载值(Auto-reloadvalue)。当定时器的计数值达到这个值时,定时器就会产生一个中断或更新事件。这个参数可以用来控制定时器中断的间隔时间。例如,如果
- 如何在MySQL中计算两个日期的间隔天数
m0_74824552
面试学习路线阿里巴巴mysql数据库
目录1.DATEDIFF函数2.TIMESTAMPDIFF函数3.PERIOD_DIFF函数4.函数对比在MySQL5.7中,计算两个日期之间的间隔天数是一项常见的任务。1.DATEDIFF函数DATEDIFF函数可以直接计算两个日期之间的天数差异。--计算2024年1月1日和2024年1月10日之间的天数差异SELECTDATEDIFF('2024-01-10','2024-01-01')ASd
- OINH系列(自编)---> <Page 1-[2]>题库原题
玄湖白虎
C++YACSNOIP算法OINH系列
不同难度的会以3~8道题为单位,可能略过一些简单的(漏掉的会以下篇展出)OINH系列即为oneinninth/eighth每次只展出总题数的九分之一或八分之一1013题目描述读入n(1usingnamespacestd;intmain(){doubles=0,m;intn,a;cin>>n;for(inti=0;i>a;s+=a;}m=s/n;printf("%.0lf",s);coutusing
- 【go】time.after内存泄漏
还没入门的大菜狗
golang
funcworker(){select{case0.0{jitteredPeriod=Jitter(period,jitterFactor)}if!sliding{t=resetOrReuseTimer(t,jitteredPeriod,sawTimeout)}func(){f()}()ifsliding{t=resetOrReuseTimer(t,jitteredPeriod,sawTimeou
- 通达信,获得当前日期
weixin_30588675
DRAWTEXT(ISNEWDAY,P_OBV,VAR2STR(DATE+19000000-20170000,0));函数名简称详解用法PERIOD周期取得周期类型.结果从0到11,依次分别是1/5/15/30/60分钟,日/周/月,多分钟,多日,季,年.DATE日期取得该周期从1900以来的的年月日.DATE例如函数返回1000101,表示2000年1月1日,DATE+19000000后才是真正
- Js函数返回值
_wy_
jsreturn
一、返回控制与函数结果,语法为:return 表达式;作用: 结束函数执行,返回调用函数,而且把表达式的值作为函数的结果 二、返回控制语法为:return;作用: 结束函数执行,返回调用函数,而且把undefined作为函数的结果 在大多数情况下,为事件处理函数返回false,可以防止默认的事件行为.例如,默认情况下点击一个<a>元素,页面会跳转到该元素href属性
- MySQL 的 char 与 varchar
bylijinnan
mysql
今天发现,create table 时,MySQL 4.1有时会把 char 自动转换成 varchar
测试举例:
CREATE TABLE `varcharLessThan4` (
`lastName` varchar(3)
) ;
mysql> desc varcharLessThan4;
+----------+---------+------+-
- Quartz——TriggerListener和JobListener
eksliang
TriggerListenerJobListenerquartz
转载请出自出处:http://eksliang.iteye.com/blog/2208624 一.概述
listener是一个监听器对象,用于监听scheduler中发生的事件,然后执行相应的操作;你可能已经猜到了,TriggerListeners接受与trigger相关的事件,JobListeners接受与jobs相关的事件。
二.JobListener监听器
j
- oracle层次查询
18289753290
oracle;层次查询;树查询
.oracle层次查询(connect by)
oracle的emp表中包含了一列mgr指出谁是雇员的经理,由于经理也是雇员,所以经理的信息也存储在emp表中。这样emp表就是一个自引用表,表中的mgr列是一个自引用列,它指向emp表中的empno列,mgr表示一个员工的管理者,
select empno,mgr,ename,sal from e
- 通过反射把map中的属性赋值到实体类bean对象中
酷的飞上天空
javaee泛型类型转换
使用过struts2后感觉最方便的就是这个框架能自动把表单的参数赋值到action里面的对象中
但现在主要使用Spring框架的MVC,虽然也有@ModelAttribute可以使用但是明显感觉不方便。
好吧,那就自己再造一个轮子吧。
原理都知道,就是利用反射进行字段的赋值,下面贴代码
主要类如下:
import java.lang.reflect.Field;
imp
- SAP HANA数据存储:传统硬盘的瓶颈问题
蓝儿唯美
HANA
SAPHANA平台有各种各样的应用场景,这也意味着客户的实施方法有许多种选择,关键是如何挑选最适合他们需求的实施方案。
在 《Implementing SAP HANA》这本书中,介绍了SAP平台在现实场景中的运作原理,并给出了实施建议和成功案例供参考。本系列文章节选自《Implementing SAP HANA》,介绍了行存储和列存储的各自特点,以及SAP HANA的数据存储方式如何提升空间压
- Java Socket 多线程实现文件传输
随便小屋
javasocket
高级操作系统作业,让用Socket实现文件传输,有些代码也是在网上找的,写的不好,如果大家能用就用上。
客户端类:
package edu.logic.client;
import java.io.BufferedInputStream;
import java.io.Buffered
- java初学者路径
aijuans
java
学习Java有没有什么捷径?要想学好Java,首先要知道Java的大致分类。自从Sun推出Java以来,就力图使之无所不包,所以Java发展到现在,按应用来分主要分为三大块:J2SE,J2ME和J2EE,这也就是Sun ONE(Open Net Environment)体系。J2SE就是Java2的标准版,主要用于桌面应用软件的编程;J2ME主要应用于嵌入是系统开发,如手机和PDA的编程;J2EE
- APP推广
aoyouzi
APP推广
一,免费篇
1,APP推荐类网站自主推荐
最美应用、酷安网、DEMO8、木蚂蚁发现频道等,如果产品独特新颖,还能获取最美应用的评测推荐。PS:推荐简单。只要产品有趣好玩,用户会自主分享传播。例如足迹APP在最美应用推荐一次,几天用户暴增将服务器击垮。
2,各大应用商店首发合作
老实盯着排期,多给应用市场官方负责人献殷勤。
3,论坛贴吧推广
百度知道,百度贴吧,猫扑论坛,天涯社区,豆瓣(
- JSP转发与重定向
百合不是茶
jspservletJava Webjsp转发
在servlet和jsp中我们经常需要请求,这时就需要用到转发和重定向;
转发包括;forward和include
例子;forwrad转发; 将请求装法给reg.html页面
关键代码;
req.getRequestDispatcher("reg.html
- web.xml之jsp-config
bijian1013
javaweb.xmlservletjsp-config
1.作用:主要用于设定JSP页面的相关配置。
2.常见定义:
<jsp-config>
<taglib>
<taglib-uri>URI(定义TLD文件的URI,JSP页面的tablib命令可以经由此URI获取到TLD文件)</tablib-uri>
<taglib-location>
TLD文件所在的位置
- JSF2.2 ViewScoped Using CDI
sunjing
CDIJSF 2.2ViewScoped
JSF 2.0 introduced annotation @ViewScoped; A bean annotated with this scope maintained its state as long as the user stays on the same view(reloads or navigation - no intervening views). One problem w
- 【分布式数据一致性二】Zookeeper数据读写一致性
bit1129
zookeeper
很多文档说Zookeeper是强一致性保证,事实不然。关于一致性模型请参考http://bit1129.iteye.com/blog/2155336
Zookeeper的数据同步协议
Zookeeper采用称为Quorum Based Protocol的数据同步协议。假如Zookeeper集群有N台Zookeeper服务器(N通常取奇数,3台能够满足数据可靠性同时
- Java开发笔记
白糖_
java开发
1、Map<key,value>的remove方法只能识别相同类型的key值
Map<Integer,String> map = new HashMap<Integer,String>();
map.put(1,"a");
map.put(2,"b");
map.put(3,"c"
- 图片黑色阴影
bozch
图片
.event{ padding:0; width:460px; min-width: 460px; border:0px solid #e4e4e4; height: 350px; min-heig
- 编程之美-饮料供货-动态规划
bylijinnan
动态规划
import java.util.Arrays;
import java.util.Random;
public class BeverageSupply {
/**
* 编程之美 饮料供货
* 设Opt(V’,i)表示从i到n-1种饮料中,总容量为V’的方案中,满意度之和的最大值。
* 那么递归式就应该是:Opt(V’,i)=max{ k * Hi+Op
- ajax大参数(大数据)提交性能分析
chenbowen00
WebAjax框架浏览器prototype
近期在项目中发现如下一个问题
项目中有个提交现场事件的功能,该功能主要是在web客户端保存现场数据(主要有截屏,终端日志等信息)然后提交到服务器上方便我们分析定位问题。客户在使用该功能的过程中反应点击提交后反应很慢,大概要等10到20秒的时间浏览器才能操作,期间页面不响应事件。
根据客户描述分析了下的代码流程,很简单,主要通过OCX控件截屏,在将前端的日志等文件使用OCX控件打包,在将之转换为
- [宇宙与天文]在太空采矿,在太空建造
comsci
我们在太空进行工业活动...但是不太可能把太空工业产品又运回到地面上进行加工,而一般是在哪里开采,就在哪里加工,太空的微重力环境,可能会使我们的工业产品的制造尺度非常巨大....
地球上制造的最大工业机器是超级油轮和航空母舰,再大些就会遇到困难了,但是在空间船坞中,制造的最大工业机器,可能就没
- ORACLE中CONSTRAINT的四对属性
daizj
oracleCONSTRAINT
ORACLE中CONSTRAINT的四对属性
summary:在data migrate时,某些表的约束总是困扰着我们,让我们的migratet举步维艰,如何利用约束本身的属性来处理这些问题呢?本文详细介绍了约束的四对属性: Deferrable/not deferrable, Deferred/immediate, enalbe/disable, validate/novalidate,以及如
- Gradle入门教程
dengkane
gradle
一、寻找gradle的历程
一开始的时候,我们只有一个工程,所有要用到的jar包都放到工程目录下面,时间长了,工程越来越大,使用到的jar包也越来越多,难以理解jar之间的依赖关系。再后来我们把旧的工程拆分到不同的工程里,靠ide来管理工程之间的依赖关系,各工程下的jar包依赖是杂乱的。一段时间后,我们发现用ide来管理项程很不方便,比如不方便脱离ide自动构建,于是我们写自己的ant脚本。再后
- C语言简单循环示例
dcj3sjt126com
c
# include <stdio.h>
int main(void)
{
int i;
int count = 0;
int sum = 0;
float avg;
for (i=1; i<=100; i++)
{
if (i%2==0)
{
count++;
sum += i;
}
}
avg
- presentModalViewController 的动画效果
dcj3sjt126com
controller
系统自带(四种效果):
presentModalViewController模态的动画效果设置:
[cpp]
view plain
copy
UIViewController *detailViewController = [[UIViewController al
- java 二分查找
shuizhaosi888
二分查找java二分查找
需求:在排好顺序的一串数字中,找到数字T
一般解法:从左到右扫描数据,其运行花费线性时间O(N)。然而这个算法并没有用到该表已经排序的事实。
/**
*
* @param array
* 顺序数组
* @param t
* 要查找对象
* @return
*/
public stati
- Spring Security(07)——缓存UserDetails
234390216
ehcache缓存Spring Security
Spring Security提供了一个实现了可以缓存UserDetails的UserDetailsService实现类,CachingUserDetailsService。该类的构造接收一个用于真正加载UserDetails的UserDetailsService实现类。当需要加载UserDetails时,其首先会从缓存中获取,如果缓存中没
- Dozer 深层次复制
jayluns
VOmavenpo
最近在做项目上遇到了一些小问题,因为架构在做设计的时候web前段展示用到了vo层,而在后台进行与数据库层操作的时候用到的是Po层。这样在业务层返回vo到控制层,每一次都需要从po-->转化到vo层,用到BeanUtils.copyProperties(source, target)只能复制简单的属性,因为实体类都配置了hibernate那些关联关系,所以它满足不了现在的需求,但后发现还有个很
- CSS规范整理(摘自懒人图库)
a409435341
htmlUIcss浏览器
刚没事闲着在网上瞎逛,找了一篇CSS规范整理,粗略看了一下后还蛮有一定的道理,并自问是否有这样的规范,这也是初入前端开发的人一个很好的规范吧。
一、文件规范
1、文件均归档至约定的目录中。
具体要求通过豆瓣的CSS规范进行讲解:
所有的CSS分为两大类:通用类和业务类。通用的CSS文件,放在如下目录中:
基本样式库 /css/core
- C++动态链接库创建与使用
你不认识的休道人
C++dll
一、创建动态链接库
1.新建工程test中选择”MFC [dll]”dll类型选择第二项"Regular DLL With MFC shared linked",完成
2.在test.h中添加
extern “C” 返回类型 _declspec(dllexport)函数名(参数列表);
3.在test.cpp中最后写
extern “C” 返回类型 _decls
- Android代码混淆之ProGuard
rensanning
ProGuard
Android应用的Java代码,通过反编译apk文件(dex2jar、apktool)很容易得到源代码,所以在release版本的apk中一定要混淆一下一些关键的Java源码。
ProGuard是一个开源的Java代码混淆器(obfuscation)。ADT r8开始它被默认集成到了Android SDK中。
官网:
http://proguard.sourceforge.net/
- 程序员在编程中遇到的奇葩弱智问题
tomcat_oracle
jquery编程ide
现在收集一下:
排名不分先后,按照发言顺序来的。
1、Jquery插件一个通用函数一直报错,尤其是很明显是存在的函数,很有可能就是你没有引入jquery。。。或者版本不对
2、调试半天没变化:不在同一个文件中调试。这个很可怕,我们很多时候会备份好几个项目,改完发现改错了。有个群友说的好: 在汤匙
- 解决maven-dependency-plugin (goals "copy-dependencies","unpack") is not supported
xp9802
dependency
解决办法:在plugins之前添加如下pluginManagement,二者前后顺序如下:
[html]
view plain
copy
<build>
<pluginManagement