DFS + 回溯专题
17. 电话号码的字母组合
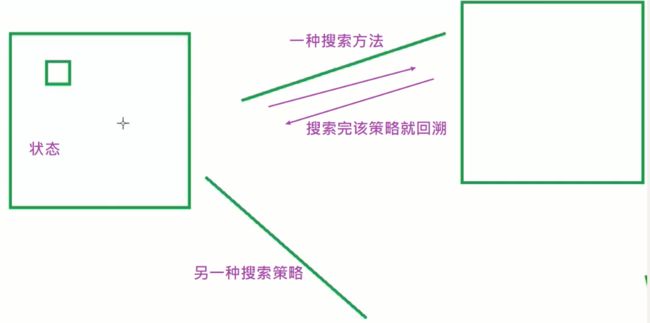
迭代也可以实现搜索
循环改写dfs搜索的写法:
例如
C++写法
class Solution {
public:
vector letterCombinations(string digits) {
string alp[8] = {"abc","def","ghi","jkl","mno","pqrs","tuv","wxyz"};
vector state;
if(digits.empty()) return state;
state.push_back("");
for(int i=0;i now;
for(int j=0;j C++11+ forEach遍历,新语法写法
class Solution {
public:
vector letterCombinations(string digits) {
string alp[8] = {"abc","def","ghi","jkl","mno","pqrs","tuv","wxyz"};
vector state;
if(digits.empty()) return state;
state.push_back("");
for(auto digit : digits){ //对digits进行遍历 取得每一个按键数字
vector now;
for(auto c : alp[digit - '2']){ //对数字对应的string能表示的形式遍历
for(auto s : state){ //对state集合里的状态进行遍历
now.push_back(s + c);
}
}
state = now;
}
return state;
}
};
两种写法耗时对比:
79. 单词搜索
搜索题
考虑顺序:
1.枚举起点 n×m
2.从起点开始依次搜索下一个点的位置 上下左右走3种
3.在枚举的过程中要保证和目标单词匹配
时间复杂度 n×m × 3^k,k为单词字符串word的长度
class Solution {
public:
int n,m;
int dx[4] = {1,-1,0,0},dy[4] = {0,0,-1,1}; //上下左右4个方向
bool exist(vector>& board, string word) {
if(board.empty() || board[0].empty()) return false;
n = board.size(),m = board[0].size();
for(int i=0;i>& board,int x,int y,string word,int k){
if(board[x][y] != word[k]) return false;
//递归出口 当最后一个字符到达
if(k == word.size() - 1) return true;
board[x][y] = '.'; //标记已访问过 用过
for(int i=0;i<4;i++){ //枚举4个方向
int nx = x + dx[i],ny = y + dy[i];
if(nx >= 0 && nx < n && ny >=0 && ny < m){
//递归搜索单词的下一个字符
if(dfs(board,nx,ny,word,k+1)){
return true;
}
}
}
board[x][y] = word[k]; //回溯
return false;
}
};
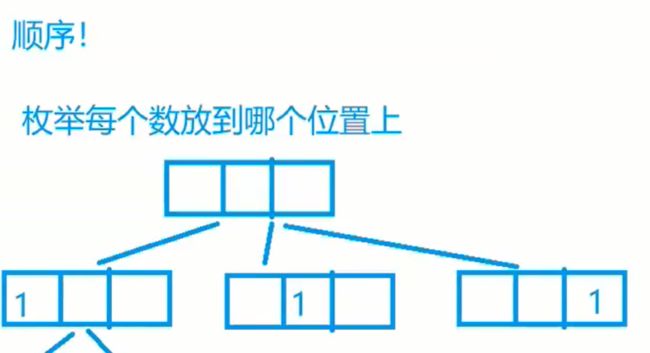
46. 全排列
全排列,先考虑顺序问题,不能重复
全排列有两种方案
第一种方案,枚举每个位置上放哪个数字,每次dfs搜索时枚举这个数标记、回溯
class Solution {
public:
int n;
vector vis;
vector path;
vector > result;
vector> permute(vector& nums) {
n = nums.size();
vis = vector(n,false); //初始化bool容器
dfs(nums,0);
return result;
}
//枚举第k个数该放哪个数字 即枚举数字
void dfs(vector& nums,int k){
if(k == n){
result.push_back(path);
return;
}
//枚举当前第k个位置上该放哪个数字 枚举这个数字的下标
for(int i=0;i 47. 全排列 II
与leetcode46比较,这题数字可能重复
需要判重
A.采用枚举每个数在哪个位置
B.判重的思路:
1.对nums数组进行排序
2.dfs搜索的时候需要保证值相同的数不在同一个位置上(从下一个位置开始)搜索
class Solution {
public:
int n;
vector> result;
vector path;
vector visit;
vector> permuteUnique(vector& nums) {
n = nums.size();
visit = vector(n);
path = vector(n);
sort(nums.begin(),nums.end());
dfs(nums,0,0);
return result;
}
//对一个数枚举该放哪个位置 即枚举位置
void dfs(vector& nums,int k,int start){
if(k == n){ //递归出口
result.push_back(path);
return;
}
//枚举num[k]放的位置在哪
for(int i=start;i 78. 子集
给定一组不含重复元素的整数数组 nums,返回该数组所有可能的子集(幂集)。
递归考虑顺序:按顺序,枚举每个数两种状态:选、不选
方法一:dfs
class Solution {
public:
int n;
vector path;
vector> result;
vector> subsets(vector& nums) {
n = nums.size();
dfs(0,nums);
return result;
}
void dfs(int k,vector& nums){
if(k == n){
result.push_back(path);
return;
}
dfs(k+1,nums);
path.push_back(nums[k]);
dfs(k+1,nums);
path.pop_back();
}
};
方法2:二进制枚举:i从0到2^n-1,位置上是1的时候选,0的时候不选
class Solution {
public:
vector> subsets(vector& nums) {
vector> result;
//二进制枚举 每个数代表一种方案
for(int i=0;i< 1< now;
//枚举每一位 位数一共nums.size()
for(int j=0;j> j & 1){
now.push_back(nums[j]);
}
}
result.push_back(now);
}
return result;
}
};
90. 子集 II
与leetcode78的区别:数字可以重复
枚举的时候,再枚举这个数的选多少个
class Solution {
public:
int n;
vector> result;
vector path;
vector> subsetsWithDup(vector& nums) {
n = nums.size();
sort(nums.begin(),nums.end()); //先排序
dfs(nums,0);
return result;
}
void dfs(vector& nums,int k){
if(k == n){
result.push_back(path);
return;
}
//统计后面与当前元素相同的个数
int cnt = 0;
while(k + cnt < n && nums[k + cnt] == nums[k]) cnt++;
for(int i=0;i<=cnt;i++) {
dfs(nums,k + cnt);
path.push_back(nums[k]);
}
for(int i=0;i<=cnt;i++) path.pop_back();
}
};
216. 组合总和 III
找出所有相加之和为 n 的 k个数的组合
组合中只允许含有 1 - 9 的正整数
并且每种组合中不存在重复的数字。
class Solution {
public:
vector> result;
vector path;
vector> combinationSum3(int k, int n) {
dfs(k,1,n);
return result;
}
//当前还剩k个没选 选到了数字start上 总和还需要n
void dfs(int k,int start,int n){
if(k == 0){
if(n == 0) result.push_back(path);
return;
}
for(int i = start; i <= 9; i++ ){
path.push_back(i);
//剩k-1个没选 选到i+1了 总和还需要n-i
dfs(k-1,i+1,n-i);
path.pop_back();
}
}
};
剪枝:因为还需要选k个,i<=9即最大值可以替换为 i<=9 + 1 - k个
for(int i = start; i <= 10-k; i++ )
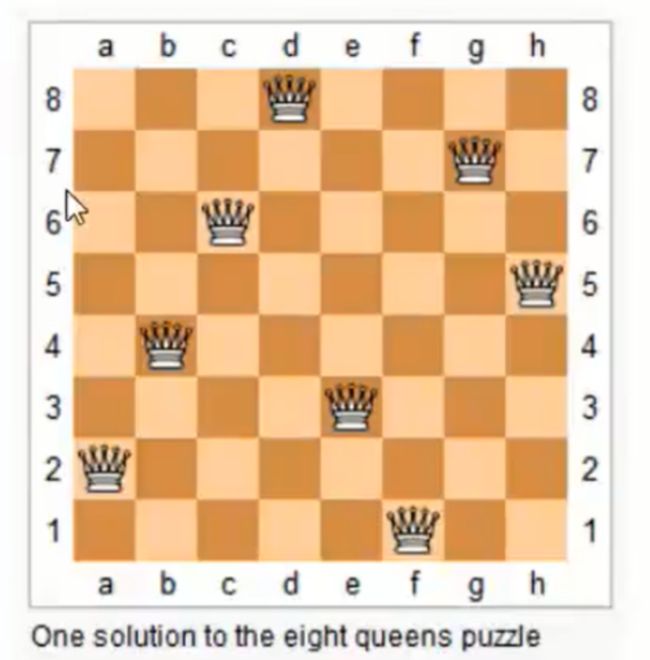
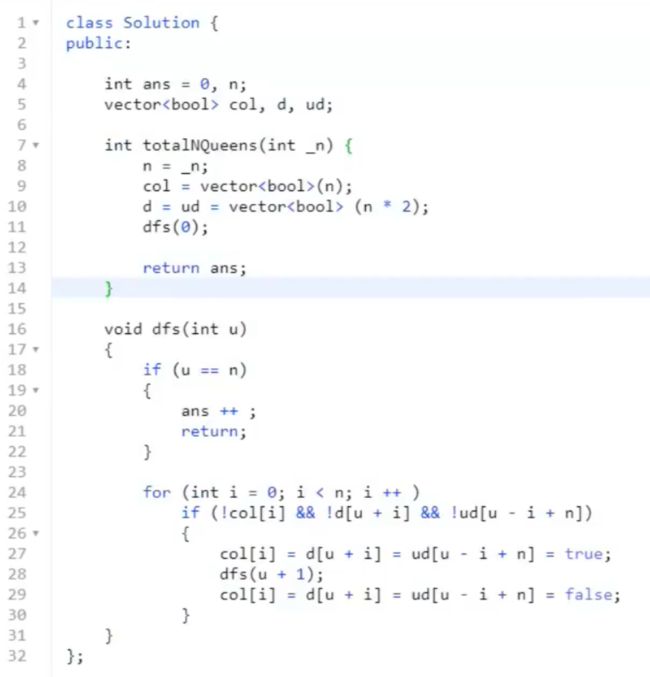
52. N皇后 II
方法一:枚举每一个坐标放不放棋子
class Solution {
public:
int ans;
vector col,row,diag,anti_diag;
int totalNQueens(int n) {
row = col = vector(n,false);
diag = anti_diag = vector(2*n,false);
ans = 0;
dfs(0,0,0,n);
return ans;
}
//当前搜索到的坐标x,y 当前已选择的皇后个数
void dfs(int x,int y,int k,int n){
if(y == n) x++, y = 0;
if(x == n){
if(k == n) ans++;
return;
}
dfs(x,y+1,k,n); //不选这个位置
if(!row[x] && !col[y] && !diag[x+y] && !anti_diag[n+x-y]){
row[x] = col[y] = diag[x+y] = anti_diag[n+x-y] = true;
dfs(x,y+1,k+1,n);
row[x] = col[y] = diag[x+y] = anti_diag[n+x-y] = false;
}
}
};
方法二:枚举每一行放在哪个位置,只需枚举行
37. 解数独
开三个标记数组,分别标记行、列、所在九宫格是否用过某个数
1.先初始化,统计原棋盘已经放的元素。
2.dfs搜索每一个坐标点,枚举该坐标点下能放哪个数字,要求所在行、所在列、所在九宫格都没有这个数字,
3.递归下一个子节点,递归完true就结束程序;否则回溯这个点
52和37的小结:
Dancing Links解决 精确覆盖问题
十字链表
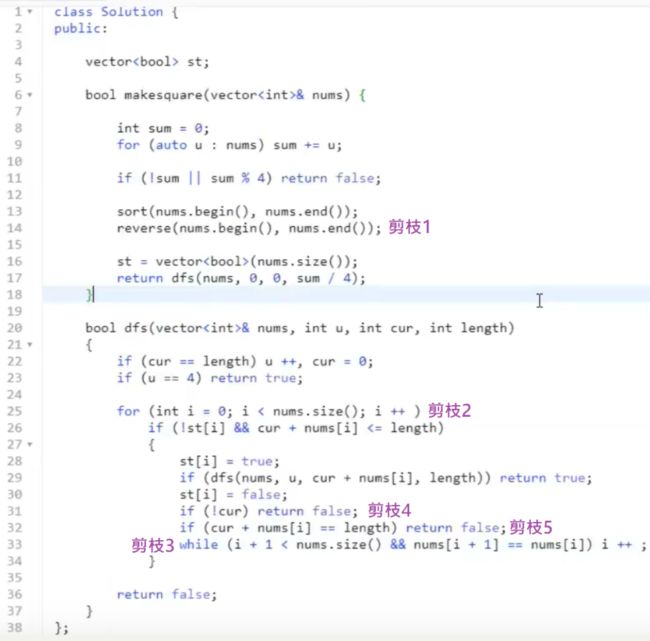
473. 火柴拼正方形
剪枝优化题
超时代码:时间复杂度n^4
class Solution {
public:
int n;
int ave;
vector vis;
bool makesquare(vector& nums) {
n = nums.size();
vis = vector(n,false);
sort(nums.begin(),nums.end());
reverse(nums.begin(),nums.end());
long long sum = 0;
int cnt = 0;
for(int i=0;i sum/4) return false;
if(nums[i] == sum/4) {
cnt++;
vis[i] = true;
}
}
if(cnt == 4 && n == 4) return true;
for(int i=0;i= sum/4) continue;
}
ave = sum/4;
return dfs(4-cnt,0,nums);
}
bool dfs(int k,int ans,vector& nums){
if(k == 0){ //递归出口
for(int i=0;i ave) return false; //剪枝
if(ans == ave) return dfs(k-1,0,nums);
//枚举这一轮选的数
for(int i=0;i 剪枝优化代码: