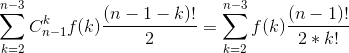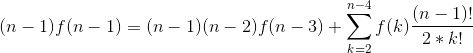- 上位机知识篇---SD卡&U盘镜像
常用的镜像烧录软件balenaEtcherbalenaEtcher是一个开源的、跨平台的工具,用于将操作系统镜像文件(如ISO和IMG文件)烧录到SD卡和USB驱动器中。以下是其使用方法、使用场景和使用注意事项的介绍:使用方法下载安装:根据自己的操作系统,从官方网站下载对应的安装包。Windows系统下载.exe文件后双击安装;Linux系统若下载的是.deb文件,可在终端执行“sudodpkg-
- kube-scheduler 抢占机制
分享放大价值
kubernetes源码分析kuberneteskube-scheduler抢占
当pod调度失败后,会在PostFilter扩展点执行抢占流程,下面分析相关的代码实现抢占接口//PodNominatorabstractsoperationstomaintainnominatedPods.typePodNominatorinterface{//将pod加入抢占成功的node中AddNominatedPod(pod*PodInfo,nodeNamestring)//将pod从no
- 无面试无offer? 你需要AI 求职co-pilot的帮助!
大家好啊,我写的开源免费求职AIco-pilot工具发布了v3.0.0,欢迎大家参与、使用!https://github.com/weicanie/prisma-ai一、项目介绍开源免费的求职co-pilot,自动化简历准备至offer到手的整个流程。优化您的项目、定制您的简历、为您匹配工作,并帮助您做好面试准备。二、核心价值prisma-ai旨在解决求职者在准备简历和寻找工作时最头疼的3个问题:
- 系统迁移从CentOS7.9到Rocky8.9
我有两台阿里云上的服务器是CentOS7.9,由于CentOS7已经停止支持,后续使用的话会有安全漏洞,所以需要尽快迁移,个人使用的话目前兼容性好的还是RockyLinux8,很多脚本改改就能用了。一、盘点系统和迁移应用查看当前系统发行版版本cat/etc/os-release盘点迁移清单服务器应用部署方式docker镜像来源v1wordpressdockerdockerhubv1zdirdock
- 【unity编辑器开发与拓展EditorGUILayoyt和GUILayoyt】
死也不注释
Unity编辑器开发与拓展笔记unity编辑器游戏引擎
EditorGUILayout与GUILayout的核心区别及使用场景详解一、对比表特性GUILayoutEditorGUILayout命名空间UnityEngineUnityEditor使用场景运行时UI+编辑器扩展仅限编辑器扩展控件风格基础游戏风格(无编辑器优化)原生Unity编辑器风格布局复杂度基础流式布局高级自动布局(带标签对齐/间距优化)序列化支持❌不支持✅直接支持SerializedP
- c++ 编译链接时 报错找不到 某个函数,如何排查?
sun007700
c++chrome开发语言
在C++开发中,链接时出现“undefinedreferenceto”错误是常见问题,以下是系统化的排查流程和解决方案:1.确认基础问题(30秒检查)#检查函数声明是否存在grep"function_name"include/*.hsrc/*.cpp#检查是否包含实现文件ls-lsrc/#确认包含实现的.cpp文件在编译列表中2.签名匹配检查(最常见问题)//头文件声明-voidprocess_d
- 具身语义导航算法总揽
Shilong Wang
具身导航算法算法
端到端方法小脑大脑GNMNavDPNaVILAViNTNomadNavidStreamVLNMapNavNavGPTUni-NavidOctoNavNavGPT2模仿学习行为克隆BCDAgger模块化方法GOATVLFMSayPlanLM-NavETPNavVoroNavEmbodiedRAGVL-NavStairwaytoSuccess业内大佬北大王鹤NavidUni-NavidOctoNav吴
- 7. TCP 和 UDP 的区别
yqcoder
前端面试-服务协议网络网络协议http
总结TCP面向连接,需要三次握手建立连接,UDP无连接,不需要握手,直接发送数据。UDP有较好的实时性,效率比TCP高。TCP面向字节流,实际上是TCP把数据看成一连串无结构的字节流,UDP是面向报文的,一次交付一个完整的报文,报文不可分割,报文是UDP数据报处理的最小单位。每一条TCP连接时一对一的,UDP可以一对多,多对一,多对多。UDP分组首部开销小,八个字节,TCP首部开销大约20字节。U
- 2.4 基于dpdk的用户态协议栈的实现
百亿苍狗
高性能网络设计专栏开发语言网络
操作系统PosixAPI所提供的网络接口,数据收发是基于用户态与内核态的频繁切换实现。而dpdk实现了绕过内核监管,直接在用户态访问网络硬件,避免频繁状态切换。DPDK安装与配置虚拟机环境配置检查是否支持多队列网卡cat/proc/interrupts|grepens33(获取整个机器的终端),结果19:4202120IO-APIC19-fasteoiens33,不支持多队列网卡。虚拟机关机,修改
- dpdk-testpmd 统计显示
背景最近在做测试的发现testpmdshowport统计的Tx-packets是个极大值,很不符合预期。硬件同学说,这个是软件统计,一定是软件问题。我大概知道它是个硬件统计,但是并不能确定,于是,做了一下代码的分析。testpmd>showportstats0########################NICstatisticsforport0########################R
- TCP和UDP协议区别+应用场景+优缺点+常用协议
马拉萨的春天
一天一读基础知识点tcp/ipudp网络
文章目录1.TCP协议特点应用场景优点缺点运行于TCP协议之上的协议2.UDP协议特点应用场景优点缺点运行于UDP协议之上的协议TCP(TransmissionControlProtocol)和UDP(UserDatagramProtocol)是两种常用的传输层协议,它们在网络通信中扮演不同的角色,各有优缺点。1.TCP协议特点提供面向连接的、可靠的数据传输服务。使用三次握手建立连接,四次挥手断开
- C语言手写简易 DNS 客户端(接收部分)
(Charon)
c语言开发语言
本文通过纯C语言手动构造DNS请求报文,使用UDP协议发送到公共DNS服务器,并接收响应,完整演示DNS请求流程。主流程:dns_client_commit()这是整个流程的核心函数,下面我们按顺序拆解每一步的逻辑,尤其突出发送sendto与接收recvfrom的设计思路和实现。第一步:创建UDP套接字intsockfd=socket(AF_INET,SOCK_DGRAM,0);if(sockfd
- 8个Java TCP/UDP框架:优缺点及应用场景全解析!
技术男老张
#编程语言-JAVA编程语言javatcp/ipudpssl网络协议websockethttp
JavaTCP框架在现代网络编程中扮演着至关重要的角色,尤其是在需要高效、稳定且可扩展的网络通信解决方案时。本文将深入探讨一些主流的JavaTCP/UDP框架,分析它们的优缺点以及适用场景,旨在为开发者提供一份详尽的指南。一、NettyNetty是一个异步事件驱动的网络应用框架,用于快速开发高性能、高可靠性的网络IO程序。Netty的设计目标是简化网络编程的复杂性,同时提高网络应用的性能和可扩展性
- 基于TCP/UDP的应用层协议
huangxy10
面试专题——网络知识
1,基于TCP的有:Telnet(TeletypeovertheNetwork,网络电传),通过一个终端(terminal)登陆到网络
- UDP并发服务器之多进程并发
一、常见的服务器类型在网络程序里面,通常都是一个服务器处理多个客户端。为了处理多个客户端的请求,服务器端程序有不同的处理方式。1.迭代服务器大多数UDP都是迭代运行,服务器等待客户端的数据,收到数据后处理该数据,送回其应答,在等待下一个客户端请求。2.并发服务器并发服务器是指在同一个时刻可以响应多个客户端的请求本质是创建多进程/多线程,对多数用户的信息进行处理UDP协议一般默认是不支持多线程并发的
- UDP服务器的优缺点都包含哪些?
wanhengidc
udp服务器网络协议
UDP协议不需要像TCP协议那样进行复杂的连接建立与拆除过程,在进行传输数据信息的过程中,应用层将数据交给UDP层,UDP层直接加上首部就发往网络层,极大地减少了处理时间和资源消耗。例如在一些简单的网络监控程序中,只是定期发送一些状态信息,对数据准确性的要求不高时,企业可以选择使用UDP服务器,能够实现快速传输数据的功能。由于UDP服务器不需要连接建立过程和重传机制的束缚,UDP数据能够快速地从发
- 【代码学习】扩散模型原理+代码
李加号pluuuus
CV基础代码学习扩散模型机器学习算法学习
来源:超详细的扩散模型(DiffusionModels)原理+代码-知乎(zhihu.com)代码:drizzlezyk/DDPM-MindSpore(github.com)DDPM1.Unet1.1正弦位置编码classSinusoidalPosEmb(nn.Cell):def__init__(self,dim):super().__init__()half_dim=dim//2#将给定的维度除
- 深入解析:UPF/PGW-U如何通过PPP/L2TP隧道实现终端PAP/CHAP接入
码农老gou
5G5G网络
在移动网络承载企业VPN或ISP接入的幕后,UPF/PGW-U化身智能PPP客户端,在L2TP隧道中完成鉴权与IP分配,为终端构筑透明传输通道。传统移动网络中,终端通常通过IPPDP类型直接获取IP地址访问互联网或IMS。但在特定场景(如企业VPN接入、某些ISP的宽带接入)中,需要终端采用PAP/CHAP鉴权并通过L2TP隧道连接到企业内网或ISP后台系统。本文将详细解析在4G/5G网络中,UP
- QT5使用cmakelists引入Qt5Xlsx库并使用
1、首先需要已经有了Qt5Xlsx的头文件和库,并拷贝到程序exe路径下(以xxx.exe/3rdparty/qtxlsx路径为例,Qt5Xlsx版本为0.3.0);2、cmakelist中:#设置QtXlsx路径set(QTXLSX_ROOT_DIR${CMAKE_CURRENT_SOURCE_DIR}/3rdparty/qtxlsx)set(QTXLSX_INCLUDE_DIR${QTXLSX
- 2025年UDP洪水攻击防护实战全解析:从T级流量清洗到AI智能防御
上海云盾商务经理杨杨
udp人工智能网络协议
一、2025年UDP洪水攻击的新特征AI驱动的自适应攻击攻击者利用生成式AI动态调整UDP报文特征(如载荷内容、发送频率),攻击流量与正常业务流量差异率低至0.5%,传统指纹过滤规则失效。反射放大攻击升级黑客通过劫持物联网设备(如摄像头、传感器)构建僵尸网络,利用DNS/NTP协议漏洞发起反射攻击,1Gbps请求可放大至50-500倍流量,峰值突破8Tbps。混合协议打击70%的UDP攻击伴随TC
- fps透视基础-d3d绘制-绘制文字-绘制方框-绘制连线
程序员陈子青
逆向工程DirectXfps透视画方框画文字
↓↓↓↓↓↓↓↓↓↓↓↓↓↓↓↓↓#include#include#pragmacomment(lib,"d3d9.lib")#pragmacomment(lib,"d3dx9.lib")staticLPDIRECT3D9g_pD3D=NULL;staticD3DPRESENT_PARAMETERSg_d3dpp={};staticLPDIRECT3DDEVICE9g_pd3dDevice=NUL
- IDEA远程联调Linux部署的Java项目
远程联调Linux部署的项目环境及工具Linux操作系统:[root@hl-db~]#cat/etc/os-releaseNAME="CentOSLinux"VERSION="7(Core)"端口开放状态:[root@hl-db~]#lsof-i:8086COMMANDPIDUSERFDTYPEDEVICESIZE/OFFNODENAMEjava32110root5uIPv44879980790t
- 查找子节点路径
一只一只妖
前端javascript
//查找子节点路径exportfunctionfindPath(nodes,targetId,path=[]){for(constnodeofnodes){constcurrentPath=[...path,node];if(node.id===targetId)returncurrentPath;if(node.children&&node.children.length){constchild
- 【译】2018 前端性能优化清单 —— 第一部分
qq_36320160
前端前端
原文地址:Front-EndPerformanceChecklist2018-Part1原文作者:VitalyFriedman译文出自:掘金翻译计划本文永久链接:https://github.com/xitu/gold-miner/blob/master/TODO/front-end-performance-checklist-2018-1.md译者:tvChan校对者:mysterytonyry
- 2024年运维最新分布式存储ceph osd 常用操作_ceph查看osd对应硬盘(1),2024年最新Linux运维编程基础教程
2401_83944328
程序员运维分布式ceph
最全的Linux教程,Linux从入门到精通======================linux从入门到精通(第2版)Linux系统移植Linux驱动开发入门与实战LINUX系统移植第2版Linux开源网络全栈详解从DPDK到OpenFlow第一份《Linux从入门到精通》466页====================内容简介====本书是获得了很多读者好评的Linux经典畅销书**《Linu
- 前端开发常见问题
技术文章大纲性能优化问题页面加载速度慢的常见原因及解决方案渲染阻塞资源的处理方法图片与媒体文件优化策略懒加载与代码分割的实现方式浏览器兼容性问题不同浏览器对CSS特性的支持差异JavaScriptAPI的兼容性处理方案Polyfill的使用场景与实现方法自动化测试工具在兼容性测试中的应用响应式设计挑战移动端与桌面端布局适配问题媒体查询的最佳实践方案视口单位与相对单位的正确使用高DPI屏幕的图像处理
- 【解决问题】HBuilderX窗口文字太小
书山有路勤为径~
物联网-手机APPuni-app
系列文章目录文章目录系列文章目录前言1右键HBuilderX,点击属性2选中兼容性3点击“更改高DPI设置”4勾选5解决总结前言在安装HBuilderX软件以后,默认字体非常小,因此需要设置1右键HBuilderX,点击属性2选中兼容性3点击“更改高DPI设置”4勾选5解决总结
- UDP协议深度解析:从原理到应用全面剖析
⭐小白苦学IT的博客主页⭐⭐初学者必看:Linux操作系统入门⭐⭐代码仓库:Linux代码仓库⭐❤关注我一起讨论和学习Linux系统前言随着互联网的蓬勃发展,网络通信协议成为了支撑其稳定运行的关键。UDP协议作为网络通信协议中的重要一员,以其高效、简洁的特点在网络通信中发挥着重要作用。本文将带您深入了解UDP协议的原理、特点以及应用,帮助您更好地掌握这一网络通信的核心技术。udp协议概念和工作原理
- 在Debian 11/Debian 10上安装MySQL 5.7
骑台风走
debianmysql运维
本文借鉴如何在Debian11/Debian10上安装MySQL5.7|https://cn.linux-console.net/?p=20728下载安装存储库安装根据提示选择mysql5.7即可(会车键选择)wgethttps://dev.mysql.com/get/mysql-apt-config_0.8.16-1_all.debsudodpkg-imysql-apt-config_0.8.1
- debian 安装 mysql5.7
你会忘记吃饭吗
debian运维
cd/usr/local/src:wgethttps://downloads.mysql.com/archives/get/p/23/file/mysql-server_5.7.29-1debian10_amd64.deb-bundle.tartar-xvfxx.tarcdxx:执行dpkg-imysql-community-client_5.7.29-1debian10_amd64.deb返回S
- 桌面上有多个球在同时运动,怎么实现球之间不交叉,即碰撞?
换个号韩国红果果
html小球碰撞
稍微想了一下,然后解决了很多bug,最后终于把它实现了。其实原理很简单。在每改变一个小球的x y坐标后,遍历整个在dom树中的其他小球,看一下它们与当前小球的距离是否小于球半径的两倍?若小于说明下一次绘制该小球(设为a)前要把他的方向变为原来相反方向(与a要碰撞的小球设为b),即假如当前小球的距离小于球半径的两倍的话,马上改变当前小球方向。那么下一次绘制也是先绘制b,再绘制a,由于a的方向已经改变
- 《高性能HTML5》读后整理的Web性能优化内容
白糖_
html5
读后感
先说说《高性能HTML5》这本书的读后感吧,个人觉得这本书前两章跟书的标题完全搭不上关系,或者说只能算是讲解了“高性能”这三个字,HTML5完全不见踪影。个人觉得作者应该首先把HTML5的大菜拿出来讲一讲,再去分析性能优化的内容,这样才会有吸引力。因为只是在线试读,没有机会看后面的内容,所以不胡乱评价了。
- [JShop]Spring MVC的RequestContextHolder使用误区
dinguangx
jeeshop商城系统jshop电商系统
在spring mvc中,为了随时都能取到当前请求的request对象,可以通过RequestContextHolder的静态方法getRequestAttributes()获取Request相关的变量,如request, response等。 在jshop中,对RequestContextHolder的
- 算法之时间复杂度
周凡杨
java算法时间复杂度效率
在
计算机科学 中,
算法 的时间复杂度是一个
函数 ,它定量描述了该算法的运行时间。这是一个关于代表算法输入值的
字符串 的长度的函数。时间复杂度常用
大O符号 表述,不包括这个函数的低阶项和首项系数。使用这种方式时,时间复杂度可被称为是
渐近 的,它考察当输入值大小趋近无穷时的情况。
这样用大写O()来体现算法时间复杂度的记法,
- Java事务处理
g21121
java
一、什么是Java事务 通常的观念认为,事务仅与数据库相关。 事务必须服从ISO/IEC所制定的ACID原则。ACID是原子性(atomicity)、一致性(consistency)、隔离性(isolation)和持久性(durability)的缩写。事务的原子性表示事务执行过程中的任何失败都将导致事务所做的任何修改失效。一致性表示当事务执行失败时,所有被该事务影响的数据都应该恢复到事务执行前的状
- Linux awk命令详解
510888780
linux
一. AWK 说明
awk是一种编程语言,用于在linux/unix下对文本和数据进行处理。数据可以来自标准输入、一个或多个文件,或其它命令的输出。它支持用户自定义函数和动态正则表达式等先进功能,是linux/unix下的一个强大编程工具。它在命令行中使用,但更多是作为脚本来使用。
awk的处理文本和数据的方式:它逐行扫描文件,从第一行到
- android permission
布衣凌宇
Permission
<uses-permission android:name="android.permission.ACCESS_CHECKIN_PROPERTIES" ></uses-permission>允许读写访问"properties"表在checkin数据库中,改值可以修改上传
<uses-permission android:na
- Oracle和谷歌Java Android官司将推迟
aijuans
javaoracle
北京时间 10 月 7 日,据国外媒体报道,Oracle 和谷歌之间一场等待已久的官司可能会推迟至 10 月 17 日以后进行,这场官司的内容是 Android 操作系统所谓的 Java 专利权之争。本案法官 William Alsup 称根据专利权专家 Florian Mueller 的预测,谷歌 Oracle 案很可能会被推迟。 该案中的第二波辩护被安排在 10 月 17 日出庭,从目前看来
- linux shell 常用命令
antlove
linuxshellcommand
grep [options] [regex] [files]
/var/root # grep -n "o" *
hello.c:1:/* This C source can be compiled with:
- Java解析XML配置数据库连接(DOM技术连接 SAX技术连接)
百合不是茶
sax技术Java解析xml文档dom技术XML配置数据库连接
XML配置数据库文件的连接其实是个很简单的问题,为什么到现在才写出来主要是昨天在网上看了别人写的,然后一直陷入其中,最后发现不能自拔 所以今天决定自己完成 ,,,,现将代码与思路贴出来供大家一起学习
XML配置数据库的连接主要技术点的博客;
JDBC编程 : JDBC连接数据库
DOM解析XML: DOM解析XML文件
SA
- underscore.js 学习(二)
bijian1013
JavaScriptunderscore
Array Functions 所有数组函数对参数对象一样适用。1.first _.first(array, [n]) 别名: head, take 返回array的第一个元素,设置了参数n,就
- plSql介绍
bijian1013
oracle数据库plsql
/*
* PL/SQL 程序设计学习笔记
* 学习plSql介绍.pdf
* 时间:2010-10-05
*/
--创建DEPT表
create table DEPT
(
DEPTNO NUMBER(10),
DNAME NVARCHAR2(255),
LOC NVARCHAR2(255)
)
delete dept;
select
- 【Nginx一】Nginx安装与总体介绍
bit1129
nginx
启动、停止、重新加载Nginx
nginx 启动Nginx服务器,不需要任何参数u
nginx -s stop 快速(强制)关系Nginx服务器
nginx -s quit 优雅的关闭Nginx服务器
nginx -s reload 重新加载Nginx服务器的配置文件
nginx -s reopen 重新打开Nginx日志文件
- spring mvc开发中浏览器兼容的奇怪问题
bitray
jqueryAjaxspringMVC浏览器上传文件
最近个人开发一个小的OA项目,属于复习阶段.使用的技术主要是spring mvc作为前端框架,mybatis作为数据库持久化技术.前台使用jquery和一些jquery的插件.
在开发到中间阶段时候发现自己好像忽略了一个小问题,整个项目一直在firefox下测试,没有在IE下测试,不确定是否会出现兼容问题.由于jquer
- Lua的io库函数列表
ronin47
lua io
1、io表调用方式:使用io表,io.open将返回指定文件的描述,并且所有的操作将围绕这个文件描述
io表同样提供三种预定义的文件描述io.stdin,io.stdout,io.stderr
2、文件句柄直接调用方式,即使用file:XXX()函数方式进行操作,其中file为io.open()返回的文件句柄
多数I/O函数调用失败时返回nil加错误信息,有些函数成功时返回nil
- java-26-左旋转字符串
bylijinnan
java
public class LeftRotateString {
/**
* Q 26 左旋转字符串
* 题目:定义字符串的左旋转操作:把字符串前面的若干个字符移动到字符串的尾部。
* 如把字符串abcdef左旋转2位得到字符串cdefab。
* 请实现字符串左旋转的函数。要求时间对长度为n的字符串操作的复杂度为O(n),辅助内存为O(1)。
*/
pu
- 《vi中的替换艺术》-linux命令五分钟系列之十一
cfyme
linux命令
vi方面的内容不知道分类到哪里好,就放到《Linux命令五分钟系列》里吧!
今天编程,关于栈的一个小例子,其间我需要把”S.”替换为”S->”(替换不包括双引号)。
其实这个不难,不过我觉得应该总结一下vi里的替换技术了,以备以后查阅。
1
所有替换方案都要在冒号“:”状态下书写。
2
如果想将abc替换为xyz,那么就这样
:s/abc/xyz/
不过要特别
- [轨道与计算]新的并行计算架构
comsci
并行计算
我在进行流程引擎循环反馈试验的过程中,发现一个有趣的事情。。。如果我们在流程图的每个节点中嵌入一个双向循环代码段,而整个流程中又充满着很多并行路由,每个并行路由中又包含着一些并行节点,那么当整个流程图开始循环反馈过程的时候,这个流程图的运行过程是否变成一个并行计算的架构呢?
- 重复执行某段代码
dai_lm
android
用handler就可以了
private Handler handler = new Handler();
private Runnable runnable = new Runnable() {
public void run() {
update();
handler.postDelayed(this, 5000);
}
};
开始计时
h
- Java实现堆栈(list实现)
datageek
数据结构——堆栈
public interface IStack<T> {
//元素出栈,并返回出栈元素
public T pop();
//元素入栈
public void push(T element);
//获取栈顶元素
public T peek();
//判断栈是否为空
public boolean isEmpty
- 四大备份MySql数据库方法及可能遇到的问题
dcj3sjt126com
DBbackup
一:通过备份王等软件进行备份前台进不去?
用备份王等软件进行备份是大多老站长的选择,这种方法方便快捷,只要上传备份软件到空间一步步操作就可以,但是许多刚接触备份王软件的客用户来说还原后会出现一个问题:因为新老空间数据库用户名和密码不统一,网站文件打包过来后因没有修改连接文件,还原数据库是好了,可是前台会提示数据库连接错误,网站从而出现打不开的情况。
解决方法:学会修改网站配置文件,大多是由co
- github做webhooks:[1]钩子触发是否成功测试
dcj3sjt126com
githubgitwebhook
转自: http://jingyan.baidu.com/article/5d6edee228c88899ebdeec47.html
github和svn一样有钩子的功能,而且更加强大。例如我做的是最常见的push操作触发的钩子操作,则每次更新之后的钩子操作记录都会在github的控制板可以看到!
工具/原料
github
方法/步骤
- ">的作用" target="_blank">JSP中的作用
蕃薯耀
JSP中<base href="<%=basePath%>">的作用
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
- linux下SAMBA服务安装与配置
hanqunfeng
linux
局域网使用的文件共享服务。
一.安装包:
rpm -qa | grep samba
samba-3.6.9-151.el6.x86_64
samba-common-3.6.9-151.el6.x86_64
samba-winbind-3.6.9-151.el6.x86_64
samba-client-3.6.9-151.el6.x86_64
samba-winbind-clients
- guava cache
IXHONG
cache
缓存,在我们日常开发中是必不可少的一种解决性能问题的方法。简单的说,cache 就是为了提升系统性能而开辟的一块内存空间。
缓存的主要作用是暂时在内存中保存业务系统的数据处理结果,并且等待下次访问使用。在日常开发的很多场合,由于受限于硬盘IO的性能或者我们自身业务系统的数据处理和获取可能非常费时,当我们发现我们的系统这个数据请求量很大的时候,频繁的IO和频繁的逻辑处理会导致硬盘和CPU资源的
- Query的开始--全局变量,noconflict和兼容各种js的初始化方法
kvhur
JavaScriptjquerycss
这个是整个jQuery代码的开始,里面包含了对不同环境的js进行的处理,例如普通环境,Nodejs,和requiredJs的处理方法。 还有jQuery生成$, jQuery全局变量的代码和noConflict代码详解 完整资源:
http://www.gbtags.com/gb/share/5640.htm jQuery 源码:
(
- 美国人的福利和中国人的储蓄
nannan408
今天看了篇文章,震动很大,说的是美国的福利。
美国医院的无偿入院真的是个好措施。小小的改善,对于社会是大大的信心。小孩,税费等,政府不收反补,真的体现了人文主义。
美国这么高的社会保障会不会使人变懒?答案是否定的。正因为政府解决了后顾之忧,人们才得以倾尽精力去做一些有创造力,更造福社会的事情,这竟成了美国社会思想、人
- N阶行列式计算(JAVA)
qiuwanchi
N阶行列式计算
package gaodai;
import java.util.List;
/**
* N阶行列式计算
* @author 邱万迟
*
*/
public class DeterminantCalculation {
public DeterminantCalculation(List<List<Double>> determina
- C语言算法之打渔晒网问题
qiufeihu
c算法
如果一个渔夫从2011年1月1日开始每三天打一次渔,两天晒一次网,编程实现当输入2011年1月1日以后任意一天,输出该渔夫是在打渔还是在晒网。
代码如下:
#include <stdio.h>
int leap(int a) /*自定义函数leap()用来指定输入的年份是否为闰年*/
{
if((a%4 == 0 && a%100 != 0
- XML中DOCTYPE字段的解析
wyzuomumu
xml
DTD声明始终以!DOCTYPE开头,空一格后跟着文档根元素的名称,如果是内部DTD,则再空一格出现[],在中括号中是文档类型定义的内容. 而对于外部DTD,则又分为私有DTD与公共DTD,私有DTD使用SYSTEM表示,接着是外部DTD的URL. 而公共DTD则使用PUBLIC,接着是DTD公共名称,接着是DTD的URL.
私有DTD
<!DOCTYPErootSYST
![]()
![]()
![]()