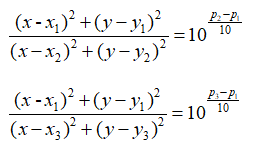C#---最速下降+牛顿迭代求二元非线性方程组

求解非线性方程组

即

Newton.cs

using System;
using System.Collections.Generic;
using System.Linq;
using System.Text;

namespace NewtonIteration
{

    class Newton
    {
        int _MaxIterativeTime = 1000000;      // 最大迭代次数
        double _Precision = 0.0001;           // 精度
        public double[] result;

        double xk, yk;
        double p1, p2, p3, x1, x2, x3, y1, y2, y3, c1, c2;

        public Newton(double p1, double p2, double p3, double x1, double x2, double x3, double y1, double y2, double y3) 
        {
            this.p1 = p1;
            this.p2 = p2;
            this.p3 = p3;
            this.x1 = x1;
            this.x2 = x2;
            this.x3 = x3;
            this.y1 = y1;
            this.y2 = y2;
            this.y3 = y3;

            this.c1 = Math.Pow(10, (p2 - p1) / 10.0);
            this.c2 = Math.Pow(10, (p3 - p1) / 10.0);
        }

        private double F(double x, double y)
        {
            return (Math.Pow(x - x1, 2) + Math.Pow(y - y1, 2)) / (Math.Pow(x - x2, 2) + Math.Pow(y - y2, 2)) - c1;
        }

        private double Fx(double x)
        {
            return 2.0 * x * (1 - c1) + 2.0 * (-x1 + c1 * x2);
        }

        private double Fy(double y)
        {
            return 2.0 * y * (1 - c1) + 2.0 * (-y1 + c1 * y2);
        }

        private double G(double x, double y)
        {
            return (Math.Pow(x - x1, 2) + Math.Pow(y - y1, 2)) / (Math.Pow(x - x3, 2) + Math.Pow(y - y3, 2)) - c2;
        }

        private double Gx(double x)
        {
            return 2.0 * x * (1 - c2) + 2.0 * (-x1 + c2 * x3);
        }

        private double Gy(double y)
        {
            return 2.0 * y * (1 - c2) + 2.0 * (-y1 + c2 * y3);
        }

        // 最速下降
        private void ZSXJ()
        {
            double m = 0.0001;

            for(int i=0; i<1000; i++)
            {
                double f = m * 2 * (F(xk, yk) * Fx(xk) + G(xk, yk) * Gx(xk));
                double g = m * 2 * (F(xk, yk) * Fy(yk) + G(xk, yk) * Gy(yk));

                xk -= f;
                yk -= g;

                double a = f * Fx(xk) + g * Fy(yk);
                double b = f * Gx(xk) + g * Gy(yk);
                m = (F(xk, yk) * a + G(xk, yk) * b) / (Math.Pow(a, 2) + Math.Pow(b, 2));

                if (Math.Pow(F(xk, yk), 2) + Math.Pow(G(xk, yk), 2) < 0.001)
                    break;
            }
        }

        public void run()
        {
            xk = (x1 + x2 + x3) / 3.0;
            yk = (y1 + y2 + y3) / 3.0;

            Console.WriteLine(xk);
            Console.WriteLine(yk);

            // 最速下降
            ZSXJ();

            Console.WriteLine(xk);
            Console.WriteLine(yk);

            double lastX = xk;
            double lastY = yk;

            // 牛顿迭代
            for (int i = 0; i < _MaxIterativeTime; i++)
            {
                xk += (F(xk, yk) * Gy(yk) - G(xk, yk) * Fy(yk)) / (Gx(xk) * Fy(yk) - Fx(xk) * Gy(yk));
                yk += (G(xk, yk) * Fx(yk) - F(xk, yk) * Gx(xk)) / (Gx(xk) * Fy(yk) - Fx(xk) * Gy(yk));

                if (Math.Abs(xk - lastX) < _Precision && Math.Abs(yk - lastY) < _Precision)
                    break;

                lastX = xk;
                lastY = yk;
            }

            Console.WriteLine(xk);
            Console.WriteLine(yk);

            result = new double[2];
            result[0] = xk;
            result[1] = yk;

            Console.ReadLine();
        }
    }
}

Program.cs

using System;
using System.Collections.Generic;
using System.Linq;
using System.Text;

namespace NewtonIteration
{
    class Program
    {
        static void Main(string[] args)
        {
            double[] x = { 668565.725, 668199.829, 667936.921 };
            double[] y = { 3548174.709, 3547598.824, 3548511.284 };
            double[] p = { -50.13, -47.71, -47.88 };

            Newton newton = new Newton(p[0], p[1], p[2], x[0], x[1], x[2], y[0], y[1], y[2]);
            newton.run(); 

        }
    }
}

原理
最速下降：https://wenku.baidu.com/view/85892d3c10661ed9ad51f3b7.html
牛顿迭代：http://blog.sina.com.cn/s/blog_14ecf9fa60102wtjq.html
http://www.doc88.com/p-3137553224119.html