floyd算法（循环问题）

floyd算法，又称插点法，多用于求给定加权图任意两点间的最短路径，比较简洁易懂的算法，但是复杂度高。用于解决点少的情况很适用。

核心代码：

for(int k=1; k<=n; k++)
for(int i=1; i<=n; i++)
for(int j=1; j<=n; j++)
    if(maze[i][j] > maze[i][k] + maze[k][j])
        maze[i][j] = maze[i][k] + maze[k][j];

三层循环的作用是暴力枚举所有中点，起点和终点，分别用 k , i , j 表示。

必须注意的是外层循环必须为 k 的枚举，考虑算法更新边权的方式，用起点到中点到终点的边权与起点到终点的边权取小。

~ 如果 k 写在第三层，也就是在确定了 i 和 j 的位置之后枚举 k，这样每组 i 和 j 只会枚举一次 k ，而 k 处的点都是没有更新过的，所以用 k 枚举一次来确定当前的 maze[ i ] [ j ] 是不能得到最优解的。

~ 如果 k 写在第二层，也就是在确定了 i 和 k的位置之后枚举 j，看似可以确定了 k 的最优解，但是同理，j 出的所有点也都是没有更新过的，所以用 j 枚举一次来确定 k 的最优解也是不能得到最优解的。

~ 如果 k 写在最外层，可以确定任意一组 i j 以任意一个点作为 k 的最优解，也就是可以求得任意一组 i j 的最优解。

~ 理解循环关键是要了解状态转移的原理。

例题  HDU 1690

Bus System

Time Limit: 2000/1000 MS (Java/Others)    Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 7611    Accepted Submission(s): 1978

Problem Description

Because of the huge population of China, public transportation is very important. Bus is an important transportation method in traditional public transportation system. And it’s still playing an important role even now.
The bus system of City X is quite strange. Unlike other city’s system, the cost of ticket is calculated based on the distance between the two stations. Here is a list which describes the relationship between the distance and the cost.



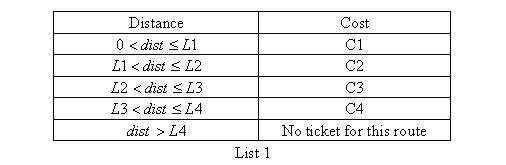
Your neighbor is a person who is a really miser. He asked you to help him to calculate the minimum cost between the two stations he listed. Can you solve this problem for him?
To simplify this problem, you can assume that all the stations are located on a straight line. We use x-coordinates to describe the stations’ positions.

 

Input

The input consists of several test cases. There is a single number above all, the number of cases. There are no more than 20 cases.
Each case contains eight integers on the first line, which are L1, L2, L3, L4, C1, C2, C3, C4, each number is non-negative and not larger than 1,000,000,000. You can also assume that L1<=L2<=L3<=L4.
Two integers, n and m, are given next, representing the number of the stations and questions. Each of the next n lines contains one integer, representing the x-coordinate of the ith station. Each of the next m lines contains two integers, representing the start point and the destination.
In all of the questions, the start point will be different from the destination.
For each case,2<=N<=100,0<=M<=500, each x-coordinate is between -1,000,000,000 and 1,000,000,000, and no two x-coordinates will have the same value.

 

Output

For each question, if the two stations are attainable, print the minimum cost between them. Otherwise, print “Station X and station Y are not attainable.” Use the format in the sample.

 

Sample Input

2 1 2 3 4 1 3 5 7 4 2 1 2 3 4 1 4 4 1 1 2 3 4 1 3 5 7 4 1 1 2 3 10 1 4

 

Sample Output

Case 1: The minimum cost between station 1 and station 4 is 3. The minimum cost between station 4 and station 1 is 3. Case 2: Station 1 and station 4 are not attainable.

#include"iostream"
#include"cmath"
#include"cstdio"
#include"string"
#include"cstring"

#define MAXN 1005
#define INF 0xfffffffffff

using namespace std;

int poi[MAXN];
long long maze[MAXN][MAXN];
int n, m, t, l1, l2, l3, l4, c1, c2, c3, c4;

void init()
{
    for(int i=1; i <= n; i++)
    for(int j=1; j <= n; j++)
    {
        if(i == j)  maze[i][j] = 0;
        else        maze[i][j] = INF;
    }
}

int main(void)
{
    scanf("%d",&t);
    int T = 1;
    while(t--)
    {
        scanf("%d%d%d%d%d%d%d%d", &l1, &l2, &l3, &l4, &c1, &c2, &c3, &c4);
        cin>>n>>m;
        init();
        for(int i=1; i<=n; i++) scanf("%d",&poi[i]);
        for(int i=1; i<=n; i++)
        {
            for(int j=i+1; j <= n; j++)
            {
                int len = abs(poi[i] - poi[j]);
                if(len>0 && len<=l1)        maze[i][j] = maze[j][i] = c1;
                else if(l1 maze[i][k] + maze[k][j])
                maze[i][j] = maze[i][k] + maze[k][j];
        printf("Case %d:\n",T++);
        while(m--)
        {
            int st, en;
            cin>>st>>en;
            if(maze[st][en] != INF)
                printf("The minimum cost between station %d and station %d is %lld.\n",st,en,maze[st][en]);
            else
                printf("Station %d and station %d are not attainable.\n", st, en);
        }
    }
}