使用jQuery读取本地json绑定表格

由于js安全不允许读写本地文件，采用引用脚本方式读取json

equation.js

var edata = [
    {
        "eid": "1",
        "etype": "RATH",
        "ename": "RATH",
        "equation": [
            "diff(u(x,t),t)+u(x,t)*diff(u(x,t),x)+p*diff(u(x,t),x$3)=0",
            "diff(u(x,t),t)+u(x,t)*diff(u(x,t),x)-p*diff(u(x,t),x$2)+q*diff(u(x,t),x$3)=0",
            "diff(u(x,t),t)+alpha*diff(u(x,t),t)*diff(u(x,t),x$2)+beta*u(x,t)*diff(u(x,t),x$3)=0",
            "diff(u(x,t),t)+u(x,t)*diff(u(x,t),x$3)+p*diff(u(x,t),x)*diff(u(x,t),x$2)+q*u(x,t)^2*diff(u(x,t),x)+diff(u(x,t),x$5)=0"
        ],
        "desc": "Real Automated TanH-function method"
    },
    {
        "eid": "2",
        "etype": "IRATH",
        "ename": "IRATH",
        "equation": [
            "diff(u(x,t),t)+u(x,t)*diff(u(x,t),x)+p*diff(u(x,t),x$3)=0",
            "diff(u(x,t),t)+u(x,t)*diff(u(x,t),x)-p*diff(u(x,t),x$2)+q*diff(u(x,t),x$3)=0",
            "diff(u(x,t),t)+alpha*diff(u(x,t),t)*diff(u(x,t),x$2)+beta*u(x,t)*diff(u(x,t),x$3)=0",
            "diff(u(x,t),t)+u(x,t)*diff(u(x,t),x$3)+p*diff(u(x,t),x)*diff(u(x,t),x$2)+q*u(x,t)^2*diff(u(x,t),x)+diff(u(x,t),x$5)=0"
        ],
        "desc": "Improved Real Automated TanH-function method"
    },
    {
        "eid": "3",
        "etype": "RAEEM",
        "ename": "RAEEM",
        "equation": [
            "[diff(u(x,y,z,t),t)+u(x,y,z,t)^2*diff(u(x,y,z,t),x)+diff(u(x,y,z,t),x$3)+diff(u(x,y,z,t),x,y,y)+diff(u(x,y,z,t),x,z,z)=0],3,3",
            "[diff(u(x,t),t)+3*v(x,t)*diff(v(x,t),x)=0,diff(v(x,t),t)+2*diff(v(x,t),x$3)+2*u(x,t)*diff(v(x,t),x)+diff(u(x,t),x)*v(x,t)=0]",
            "[diff(u(x,y,z,t),t)+u(x,y,z,t)^2*diff(u(x,y,z,t),x)+diff(u(x,y,z,t),x$3)+diff(u(x,y,z,t),x,y,y)+diff(u(x,y,z,t),x,z,z)=0],3,3"
        ],
        "desc": "Real Automated Elliptic Equation Method"
    },
    {
        "eid": "4",
        "etype": "SEMPS",
        "ename": "SEMPS",
        "equation": [
            "[diff(u(x,t),t)+u(x,t)*diff(u(x,t),x)+p*diff(u(x,t),x$3)=0],[diff(f(x),x)=R*g(x)^2,diff(g(x),x)=mu*g(x)*f(x),g(x)^2=s+R*f(x)^2],[f(x),g(x)]",
            "[diff(u(x, t), t)+u(x, t)*(diff(u(x, t), x))+p*diff(u(x, t), x$3) = 0],[diff(f(x), x) = g(x)*h(x), diff(g(x), x) = -f(x)*h(x), diff(h(x), x) = -n^2*g(x)*f(x), g(x)^2 = 1-f(x)^2, h(x)^2 = 1-n^2*f(x)^2],[f(x), g(x), h(x)]"
        ],
        "desc": "Sub Eq Method and Polynomial Solutions"
    },
    {
        "eid": "5",
        "etype": "AutoBT",
        "ename": "AutoBT",
        "equation": [
            "diff(w(x,t),t)-6*w(x,t)*diff(w(x,t),x)+diff(w(x,t),x$3)",
            "diff(w(x,t),t)+p*w(x,t)^2*diff(w(x,t),x)+diff(w(x,t),x$3)"
        ],
        "desc": "Automated Bäcklund Transformation method"
    },
    {
        "eid": "6",
        "etype": "CRE",
        "ename": "CRE",
        "equation": [
            "[diff(u(x,t),t)+6*u(x,t)*diff(u(x,t),x)+diff(u(x,t),x$3)=0]",
            "[diff(u(x,t),t)+u(x,t)*diff(u(x,t),x)+diff(v(x,t),x)=0,diff(v(x,t),t)+diff(u(x,t),x)+diff(u(x,t)*v(x,t),x)+diff(u(x,t),x,x,x)=0]"
        ],
        "desc": "Automated Consistent Riccati Expansion Method"
    },
    {
        "eid": "7",
        "etype": "ADSP",
        "ename": "ADSP",
        "equation": [
            "[diff(u(x, t), t)+6*u(x, t)*diff(u(x, t), x)+diff(u(x, t), x$3) = 0]",
            "[diff(u(x, t), t$2)-diff(u(x, t), x$2)-diff(u(x, t), x$4)-3*diff(u(x, t)^2, x$2) = 0]",
            "[diff(u(x, t), t)-(1/4)*diff(u(x, t), x$5)-5*diff(u(x, t), x)*diff(u(x, t), x$2)-(5/2)*u(x, t)*diff(u(x, t), x$3)-(15/2)*u(x, t)^2*diff(u(x, t), x) = 0]",
            "[diff(u(x, t), t)-6*u(x, t)*diff(u(x, t), x)+diff(u(x, t), x, x, x)-6*v(x, t)*diff(v(x, t), x) = 0, diff(v(x, t), t)-6*diff(u(x, t)*v(x, t), x)+diff(v(x, t), x, x, x) = 0]"
        ],
        "desc": "Automated Derivation Solutions for PDE"
    },
    {
        "eid": "8",
        "etype": "ADMP",
        "ename": "ADMP",
        "equation": [
            "[diff(y(x),x$2)=3/4*y(x)+y(x/2)-x^2+2],[y(0)=0,D(y)(0)=0],[y(x)],output=plot,err=true,x=0..1,y=0..1,index=15,pade=[7,7]",
            "[diff(y(t),t$alpha)+y(t)=0],[y(0)=1,D(y)(0)=0],alpha=1.3,index=50,output=plot,t=0..20,y=-0.2..1,pade=[150,150]"
        ],
        "desc": "Adomian Decomposition Method Package"
    },
    {
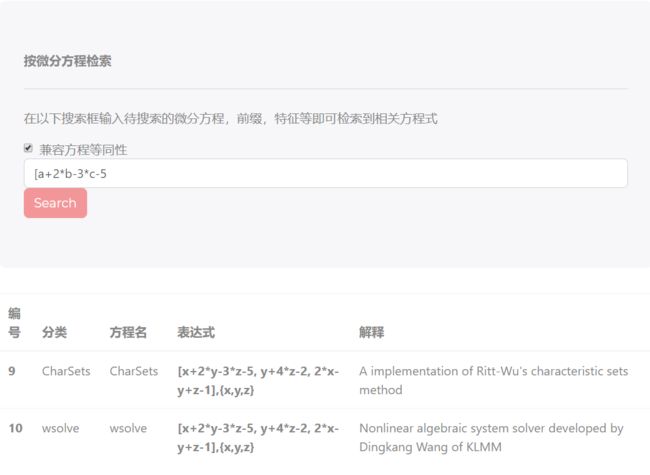
        "eid": "9",
        "etype": "CharSets",
        "ename": "CharSets",
        "equation": [
            "[x+2*y-3*z-5,  y+4*z-2,  2*x-y+z-1],{x,y,z}",
            "[2*x^2+x*y-y+1, -3*x*y+2*y^2-x-2, -3*x*y^2+2*y^3+2*x^2-3*y+1],[x,y]"
        ],
        "desc": "A implementation of Ritt-Wu's characteristic sets method"
    },
    {
        "eid": "10",
        "etype": "wsolve",
        "ename": "wsolve",
        "equation": [
            "[x+2*y-3*z-5,  y+4*z-2,  2*x-y+z-1],{x,y,z}",
            "[2*x^2+x*y-y+1, -3*x*y+2*y^2-x-2, -3*x*y^2+2*y^3+2*x^2-3*y+1],[x,y]"
        ],
        "desc": "Nonlinear algebraic system solver developed by Dingkang Wang of KLMM"
    }
]

1、读取并绑定表格

$("#btnOverview").click(function () {;
    var eHtml = ' ';
    for (var i = 0; i < edata.length; i++) {
        console.log(edata[i]);
        console.log(eHtml);
        if (i == 0)
            eHtml += '';
        else
            eHtml += '';

        eHtml += '' + edata[i].eid + ''
        eHtml += '' + edata[i].etype + ''
        eHtml += '' + edata[i].ename + ''
        eHtml += '' + edata[i].equation[0] + ''
        eHtml += '' + edata[i].desc + ''
        eHtml += ''
    }

    $("#indextbody").html(eHtml);
})

2、按类型查询

$("#btnSearch").click(function () {
    console.log("btnSearch");
    var searchType = $("#sType").val();
    console.log(searchType);

    var eHtml = ' ';
    for (var i = 0; i < edata.length; i++) {
        if (edata[i].etype == searchType) {
            for (var j = 0; j < edata[i].equation.length; j++) {
                if (i == 0)
                    eHtml += '';
                else
                    eHtml += '';
                eHtml += '' + edata[i].eid + ''
                eHtml += '' + edata[i].etype + ''
                eHtml += '' + edata[i].ename + ''

                eHtml += '' + edata[i].equation[j] + ''

                eHtml += '' + edata[i].desc + ''
                eHtml += ''
            }
        }

    }
    $("#lookuptable").html(eHtml);
})

3、录入新数据条目

$("#btnSave").click(function () {
    console.log("btnSearch");
    var saveType = $("#sType").val();
    var saveName = $("#sName").val();
    var saveEqua = $("#sEqua").val();
    var saveDesc = $("#sDesc").val();

    var saveJSON = {
        "eid": edata.length+1,
        "etype": saveType,
        "ename": saveName,
        "equation": saveEqua,
        "desc": saveDesc
    }

    edata.push(saveJSON);
    alert("微分公式插入完成");
})

---

fake function 方程等同性检索