- AI大模型产品经理学习路线,2025最新,从AI产品经理零基础入门到精通,非常详细收藏我这一篇够了!
AGI-杠哥
人工智能产品经理学习语言模型agi自然语言处理
随着人工智能技术的发展,尤其是大模型(LargeModel)的兴起,越来越多的企业开始重视这一领域的投入。作为大模型产品经理,你需要具备一系列跨学科的知识和技能,以便有效地推动产品的开发、优化和市场化。以下是一份详细的大模型产品经理学习路线,旨在帮助你构建所需的知识体系,从零基础到精通。一、基础知识阶段1.计算机科学基础数据结构与算法:理解基本的数据结构(如数组、链表、树、图等)和常用算法(如排序
- Linux内核中的数据结构与算法(三)哈希链表
木木0o0欧尼
Linux链表数据结构linux
四,哈希链表谈到链表就不得不谈Linux内核中另外一个重要的结构,哈希链表。讨论这个结构前,你需要对哈希的最基本的概念要清楚哦,由于我们已经讲过Linux内核中的普通链表的结构,这里我们对比他们的区别来了解哈希链表会直观一些。Linux链表认为双指针表头双循环链表对于HASH表来说过于浪费,因而设计了一套用于HASH表的hlist的数据结构,单指针表头双循环链表。hlish表头仅有一个指向首节点的
- Java架构师成长之路
hweiyu00
分享spring微服务springcloudjava
概述本教程主要从6个方面,全面讲解Java技术栈的知识。1.性能调优深入理解MySQL底层原理、索引逻辑,数据结构与算法。使用Explain进行优化分析MVCC原理剖析日志机制解析2.框架源码掌握Spring底层原理带你手写一个Spring解析IOC、AOP源码、以及事务原理3.并发编程剖析Java底层锁机制CAS、JUC工具使用、AQS源码分析以及并发的集合类的讲解4.分布式开发剖析分布式中使用
- 《Hello 算法》火了!!!一本写给算法初学者的入门算法书籍
遇码
分享算法hellohello算法算法书籍
曾经也放出豪言壮语,决心要刷遍力扣上的所有算法题目。然而现实就很快啪啪的打脸。不知道多少人和我有过一样的经历。在读到《Hello算法》的序中,作者靳宇栋给了我们一个“台阶”。随后就表达了针对我们的现状,他特地写了《Hello算法》这本书,代表广大算法初学者表示感激涕零。《Hello算法》为什么适合入门动画图解、一键运行的数据结构与算法教程全书采用动画图解,内容清晰易懂、学习曲线平滑,引导初学者探索
- 大二下开始学数据结构与算法--07,单项循环链表的实现
爱我的你不说话
链表数据结构
自习所完成的任务单向循环链表代码的实现和测验任务学课程到p28复现相关代码感悟其实这个教程上的观念,跟我刚开始理解想的并不一样,我以为会是:头节点使实例化的节点的循环链表,但是,教程给的更像是存在头节点,但头节点没有实际意义的添加了尾节点单项循环链表(跟之前单向不循环链表相比,更像是只多了一尾节点)。#include#include#includeusingnamespacestd;//存在头节点
- 大二下开始学数据结构与算法--06,判断两个节点是否相交,删除链表倒数第K个节点
爱我的你不说话
链表数据结构
自习所完成的任务完成函数判断单项链表是否相交的代码编写和测试。完成函数删除倒数第K个节点的代码编写和测试。感悟其实这篇是昨天晚上写的,但是昨天下午在实验室呆了一下,然后写完这些代码后感觉脑袋昏沉,晚上十点就回宿舍了,想着看会儿书,但是,没看成,还是玩手机了。感觉坚持做一件事,还挺难的,老是为自己找逃避的借口,比如说周三晚上跟舍友出去吃,就放下了写代码的每日任务。我在想,是不是应该改变一下观念,以进
- 华为OD机试九日集训第2期 - 按算法分类,由易到难,循序渐进,提升编程能力和解题技巧,从而提高机试通过率
哪 吒
搬砖工逆袭Java架构师华为od算法九日集训Java
目录一、适合人群二、本期训练时间三、如何参加四、数据结构与算法大纲五、华为OD九日集训第1期第1天、逻辑分析第2天、队列第3天、双指针第4天栈第5天滑动窗口第6天、二叉树第7天、并查集第8天、矩阵第9天、贪心算法六、国内直接使用满血ChatGPT4o、o1、o3-mini-high、Claude3.7Sonnet、满血DeepSeekR11、纯原版ChatGPT、Claude2、技术支持3、支持所
- 数据结构与算法——栈和队列
深度学习&目标检测实战项目
算法数据结构java开发语言
目录第三章:栈和队列第一节:栈(Stack)1.1:栈的基本运算:1.2:栈的存储结构和基本运算第二节:队列2.1:定义及基本运算2.2:队列的存储结构和基本运算本章小结:第三章:栈和队列第一节:栈(Stack)是限制在表一端进行插入和删除操作的线性表。允许进行插入、删除操作的这一端称为栈顶(Top),另一个固定端称为栈底。例如栈中有三个元素,近栈的顺序是a1、a2、a3,当需要出栈时顺序为a3,
- Ada语言的数据结构与算法
尤宸翎
包罗万象golang开发语言后端
Ada语言的数据结构与算法引言在计算机科学的领域里,数据结构与算法是核心的组成部分,围绕着如何高效地存储和处理数据。这些概念不仅是程序设计的重要基础,也是提高程序性能的关键。Ada是一种强类型、结构化的编程语言,早在20世纪80年代就被设计用于军用和实时系统。由于其高可靠性和可维护性,Ada逐渐在航空航天、军事和其他需要高安全性的领域获得了广泛应用。本文将探讨Ada语言中的数据结构和算法,包括常见
- 成为编程大佬!!----->数据结构与算法(2)——顺序表!!
Elnaij
算法数据结构c语言
前言:线性表是数据结构与算法的重中之重,所有具有线性逻辑结构的数据结构,都能称为线性表。这篇文章我们先来讨论线性表中的顺序表,顺序表和线性表都是后续实现栈,树,串和图等等结构的重要基础。目录❀简单介绍线性表❀顺序表❀顺序表的存储❀动态存储❀静态存储❀静态存储与动态存储的优缺点❀顺序表操作❀1.初始化顺序表❀2.销毁顺序表❀3.插入数据❀插入数据之判断已满否❀插入操作之尾插❀插入操作之头插❀插入数据
- 数据结构与算法:单调栈
WBluuue
c++算法数据结构leetcode
前言单调栈是一种维护数组当前位置左右两侧比它小或大的最近的数的一种数据结构。一、经典用法单调栈的经典用法就是找数组当前位置的数左右两侧比它小或大的最近的数。1.模板——单调栈结构(进阶)#includeusingnamespacestd;voidfindSmall(vector&arr){stackindex;vector>ans(1000001,vector(2,0));//存下标intcur;
- 数据结构与算法:洪水填充
WBluuue
c++算法leetcode数据结构深度优先剪枝图论
前言洪水填充是一种用在图上的搜索算法,其过程就像洪水或病毒一样逐渐蔓延整个区域,继而达到遍历和统计相同属性的连通区域的功能,中间也可以通过每走过一个节点就设置路径信息的方法来达到剪枝的效果。一、岛屿数量——洪水填充方法classSolution{public:intnumIslands(vector>&grid){returnsolve2(grid);}//洪水填充方法intsolve2(vect
- 数据结构与算法——二叉树,多叉树的递归遍历、层序遍历,DFS与BFS
Book_熬夜!
数据结构与算法深度优先宽度优先算法数据结构广度优先
文章目录二叉树1.递归遍历2.层序遍历3.多叉树遍历二叉树【子节点】:每个节点下方相连的节点【父节点】:每个节点上方相连的节点【根节点】:最上方没有父节点的节点【叶子节点】:最下方没有子节点的节点【最大深度】:树的最大层数【高度】:节点数减一,即枝数。【满二叉树(PerfectBinaryTree)】:深度为h,则总节点数:2^h-1FullBinaryTree是指一棵二叉树的所有节点要么没有孩子
- 数据结构与算法——二叉搜索树,使用TreeMap将键值对存储在一棵二叉搜索树的节点
Book_熬夜!
数据结构与算法算法javascript数据结构
二叉搜索树【二叉搜索树(BST)】:对于树中的每个节点,其左子树的每个节点的值都要小于这个节点的值,右子树的每个节点的值都要大于这个节点的值。左小右大。中序遍历结果是有序的,会从小到大排序。7/\49/\\1810(不符合)可以使用TreeMap把键值对存储在一棵二叉搜索树的节点里通过遍历这棵二叉搜索树,比遍历普通的二叉树能更快实现增删查改classTreeNode{constructor(key
- 02、数据结构与算法 - 基础:数组 - 吊打面试官
星星学霸
数据结构与算法-吊打面试官python开发语言java算法数据结构
更多系列教程,每天更新更多教程关注:xxxueba.com星星学霸本篇博客我们介绍数据结构的鼻祖------数组,可以说数组几乎能表示一切的数据结构,在每一门编程语言中,数组都是重要的数据结构,当然每种语言对数组的实现和处理也不相同,但是本质是都是用来存放数据的的结构,这里我们以Java语言为例,来详细介绍Java语言中数组的用法。Java中数组的介绍在Java中,数组是用来存放同一种数据类型的集
- 数据结构与算法-图(绪论 图论基本概念)
可爱的野指针
数据结构图论算法数据结构有向图欧拉回路
昨天我的的树就分享完了,树的概念很多吧,二叉树,满二叉树,完全二叉树,赫夫曼树,孩子,双亲……多不?哈哈哈,这算不了什么,我们接下来要看到的图的概念才叫多,没关系,勤奋和时间会让你记住他们,内心只需要告诉自己,加油,我能行,就一定能学会图。不知道有没有看过或者学过离散数学,如果学过,那么恭喜啦,离散数学里的图论就是这一章的基础,图论学的还不错的话,压力就小了。先介绍的是图的定义,图-V个顶点和E条
- 数据结构与算法-图论-二分图
一个人在码代码的章鱼
#图论算法学习图论算法
关押罪犯(贪心+二分答案+染色法判定二分图/扩展域并查集)题目描述S城现有两座监狱,一共关押着N名罪犯,编号分别为1∼N。他们之间的关系自然也极不和谐。很多罪犯之间甚至积怨已久,如果客观条件具备则随时可能爆发冲突。我们用“怨气值”(一个正整数值)来表示某两名罪犯之间的仇恨程度,怨气值越大,则这两名罪犯之间的积怨越多。如果两名怨气值为c的罪犯被关押在同一监狱,他们俩之间会发生摩擦,并造成影响力为c的
- 数据结构与算法——数据结构4
写代码写到手抽筋
数据结构与算法数据结构
程序员没有稳定一说,目前学习数据结构,其实不难,最近在学习,系统性的总结下,便于后续复习和使用。主要是把线性表,全名为线性存储结构。使用线性表存储数据的方式可以这样理解,即“把所有数据用一根线儿串起来,再存储到物理空间中”。分为顺序表和单链表。顺序表单链表同时还要知道顺序表和链表的优缺点【待补充】还要知道链表反转,知道迭代法和递归法就可以【】还需要知道单链表相交的思路【】后边了解静态链表的原理静态
- 「QT」布局类 之 QHBoxLayout 水平布局类
何曾参静谧
「QT」QT5程序设计qt开发语言
✨博客主页何曾参静谧的博客(✅关注、点赞、⭐收藏、转发)文章专栏「QT」QT5程序设计全部专栏(专栏会有变化,以最新发布为准)「Win」Windows程序设计「IDE」集成开发环境「UG/NX」BlockUI集合「C/C++」C/C++程序设计「DSA」数据结构与算法「UG/NX」NX二次开发「QT」QT5程序设计「File」数据文件格式「UG/NX」NX定制开发「Py」Python程序设计「Ma
- Java线程协作式中断机制
超人汪小建(seaboat)
线程协作式中断机制jvm
跟着作者的65节课彻底搞懂Java并发原理专栏,一步步彻底搞懂Java并发原理。作者简介:笔名seaboat,擅长工程算法、人工智能算法、自然语言处理、计算机视觉、架构、分布式、高并发、大数据和搜索引擎等方面的技术,大多数编程语言都会使用,但更擅长Java、Python和C++。平时喜欢看书写作、运动、画画。崇尚技术自由,崇尚思想自由。出版书籍:《Tomcat内核设计剖析》、《图解数据结构与算法》
- Python实现数据结构与算法——反转字符串
Mantana
数据结构与算法字符串算法数据结构递归法
题目描述:编写一个函数,其作用是将输入的字符串反转过来。输入字符串以字符数组char[]的形式给出。不要给另外的数组分配额外的空间,你必须原地修改输入数组、使用O(1)的额外空间解决这一问题。你可以假设数组中的所有字符都是ASCII码表中的可打印字符。示例1:输入:["h","e","l","l","o"]输出:["o","l","l","e","h"]示例2:输入:["H","a"
- 数据结构与算法——哈希表,数组加强哈希表,双链表加强哈希表
Book_熬夜!
数据结构与算法散列表哈希算法数据结构javascript算法
文章目录哈希表1.数组实现hash表2.双链表实现hash表哈希表key是唯一的,value可以重复哈希表和我们常说的Map(键值映射)不是同一个东西。【Map】是一个Java接口,仅声明了若干个方法,并没有给出方法的具体实现;HashMap这种数据结构根据自身特点实现了这些操作。可以说hashmap的get、put、remove等方法复杂度为O(1),但是map接口的复杂度不一定,需要看他底层数
- 数据结构与算法(java版)
future-2002
算法数据结构
一、初识数据结构与算法1.1数据结构与算法数据结构是指在计算机中组织和存储数据的方式。它关注数据的逻辑关系、操作和存储方式,以及如何有效地访问和修改数据。常见的数据结构包括数组、链表、栈、队列、树、图等。算法是解决问题的一系列步骤或规则。它描述了如何通过输入数据来产生所需的输出结果。算法可以用来执行各种计算任务,如排序、搜索、图形处理等。好的算法应该具有正确性、可读性、高效性和健壮性。数据结构和算
- 机器狗监控系统软件工程师面试题
道亦无名
机器人面试机器狗
大部分企业会使用的面试题一、基础知识编程语言方面请简述C++中多态的实现方式,在机器狗监控系统中,哪里可能会用到多态来提高代码的扩展性?例如不同型号机器狗的运动控制模块。Python作为脚本语言在系统开发中有诸多应用,说说Python的GIL(全局解释锁)对多线程性能的影响,以及在实时数据采集与处理场景下如何规避。数据结构与算法若要实现机器狗的路径规划,你会选择哪种数据结构来存储地图信息,比如栅格
- Python高级开发工程师
巴啦啦小魔仙变身
python开发语言
Python高级开发工程师通常会围绕技术能力、项目经验、问题解决能力等方面展开,以下为你详细介绍面试的常见内容、准备方式及注意事项:常见面试内容技术基础语言特性:深入理解Python的高级特性,如装饰器、元类、描述符等的原理和应用场景。例如,面试官可能会要求你现场编写一个装饰器来实现函数执行时间的统计。数据结构与算法:熟悉常见的数据结构(如列表、字典、集合、堆、栈、队列、链表、树、图等)和算法(如
- 刷题前必学!二叉树!用JavaScript学数据结构与算法
JavaScript算法与数据结构-HowieCong务必要熟悉JavaScript使用再来学!一、树是什么?数据结构中的树,对于现实世界中的树简化——树根抽象为“根节点”,树枝抽象为“边”,树枝的两个端点抽象为“结点”,树叶抽象为“叶子结点”计算机中的树如下:二、树的重点树的层次计算规则:根结点所在的那一层为第一层,其子节点为第二层,以此类推结点和树的高度计算规则:叶子结点高度为1,每向上一层
- 2025年大模型AI产品经理学习路线图:零基础到精通,一篇收藏,开启学习之旅!悄悄努力然后惊艳所有人
AGI大模型老王
人工智能产品经理学习AI大模型大模型学习大模型AI产品经理
随着人工智能技术的发展,尤其是大模型(LargeModel)的兴起,越来越多的企业开始重视这一领域的投入。作为大模型产品经理,你需要具备一系列跨学科的知识和技能,以便有效地推动产品的开发、优化和市场化。以下是一份详细的大模型产品经理学习路线,旨在帮助你构建所需的知识体系,从零基础到精通。一、基础知识阶段1.计算机科学基础数据结构与算法:理解基本的数据结构(如数组、链表、树、图等)和常用算法(如排序
- 给求职者的建议:软件工程师
追寻向上
pythonjavac语言软件工程
一、编程基础:构建核心能力语言选择与学习首推Python:语法简洁,适合入门。推荐书籍《Python编程:从入门到实践》,重点掌握列表推导、装饰器、文件操作。Java/C++进阶:理解内存管理(如JVM垃圾回收)、多线程编程(synchronized关键字)。推荐《Java核心技术卷Ⅰ》。辅助语言:JavaScript(必学)、Go或Rust(扩展视野)。数据结构与算法基础必刷:数组、链表、哈希表
- 字节跳动C++客户端开发实习生内推-抖音基础技术
飞300
业界资讯c++
智能手机爱好者和使用者,追求良好的用户体验;具有良好的编程习惯,代码结构清晰,命名规范;熟练掌握数据结构与算法、计算机网络、操作系统、编译原理等课程;熟练掌握C/C++/OC/Swift一种或多种语言,理解基本的设计模式;有深度参与开源项目或者自己独立开发过App上架App商城优先。内推链接(校招与实习均含):https://job.toutiao.com/campus/m/position?ex
- 数据结构与算法(两两交换链表中的结点)
银迢迢
算法笔记链表数据结构
原题24.两两交换链表中的节点-力扣(LeetCode)给你一个链表,两两交换其中相邻的节点,并返回交换后链表的头节点。你必须在不修改节点内部的值的情况下完成本题(即,只能进行节点交换)。示例1:输入:head=[1,2,3,4]输出:[2,1,4,3]示例2:输入:head=[]输出:[]示例3:输入:head=[1]输出:[1]解答建立一个虚拟结点virtual指向head,cur=virtu
- Dom
周华华
JavaScripthtml
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/1999/xhtml&q
- 【Spark九十六】RDD API之combineByKey
bit1129
spark
1. combineByKey函数的运行机制
RDD提供了很多针对元素类型为(K,V)的API,这些API封装在PairRDDFunctions类中,通过Scala隐式转换使用。这些API实现上是借助于combineByKey实现的。combineByKey函数本身也是RDD开放给Spark开发人员使用的API之一
首先看一下combineByKey的方法说明:
- msyql设置密码报错:ERROR 1372 (HY000): 解决方法详解
daizj
mysql设置密码
MySql给用户设置权限同时指定访问密码时,会提示如下错误:
ERROR 1372 (HY000): Password hash should be a 41-digit hexadecimal number;
问题原因:你输入的密码是明文。不允许这么输入。
解决办法:用select password('你想输入的密码');查询出你的密码对应的字符串,
然后
- 路漫漫其修远兮 吾将上下而求索
周凡杨
学习 思索
王国维在他的《人间词话》中曾经概括了为学的三种境界古今之成大事业、大学问者,罔不经过三种之境界。“昨夜西风凋碧树。独上高楼,望尽天涯路。”此第一境界也。“衣带渐宽终不悔,为伊消得人憔悴。”此第二境界也。“众里寻他千百度,蓦然回首,那人却在灯火阑珊处。”此第三境界也。学习技术,这也是你必须经历的三种境界。第一层境界是说,学习的路是漫漫的,你必须做好充分的思想准备,如果半途而废还不如不要开始。这里,注
- Hadoop(二)对话单的操作
朱辉辉33
hadoop
Debug:
1、
A = LOAD '/user/hue/task.txt' USING PigStorage(' ')
AS (col1,col2,col3);
DUMP A;
//输出结果前几行示例:
(>ggsnPDPRecord(21),,)
(-->recordType(0),,)
(-->networkInitiation(1),,)
- web报表工具FineReport常用函数的用法总结(日期和时间函数)
老A不折腾
finereport报表工具web开发
web报表工具FineReport常用函数的用法总结(日期和时间函数)
说明:凡函数中以日期作为参数因子的,其中日期的形式都必须是yy/mm/dd。而且必须用英文环境下双引号(" ")引用。
DATE
DATE(year,month,day):返回一个表示某一特定日期的系列数。
Year:代表年,可为一到四位数。
Month:代表月份。
- c++ 宏定义中的##操作符
墙头上一根草
C++
#与##在宏定义中的--宏展开 #include <stdio.h> #define f(a,b) a##b #define g(a) #a #define h(a) g(a) int main() { &nbs
- 分析Spring源代码之,DI的实现
aijuans
springDI现源代码
(转)
分析Spring源代码之,DI的实现
2012/1/3 by tony
接着上次的讲,以下这个sample
[java]
view plain
copy
print
- for循环的进化
alxw4616
JavaScript
// for循环的进化
// 菜鸟
for (var i = 0; i < Things.length ; i++) {
// Things[i]
}
// 老鸟
for (var i = 0, len = Things.length; i < len; i++) {
// Things[i]
}
// 大师
for (var i = Things.le
- 网络编程Socket和ServerSocket简单的使用
百合不是茶
网络编程基础IP地址端口
网络编程;TCP/IP协议
网络:实现计算机之间的信息共享,数据资源的交换
协议:数据交换需要遵守的一种协议,按照约定的数据格式等写出去
端口:用于计算机之间的通信
每运行一个程序,系统会分配一个编号给该程序,作为和外界交换数据的唯一标识
0~65535
查看被使用的
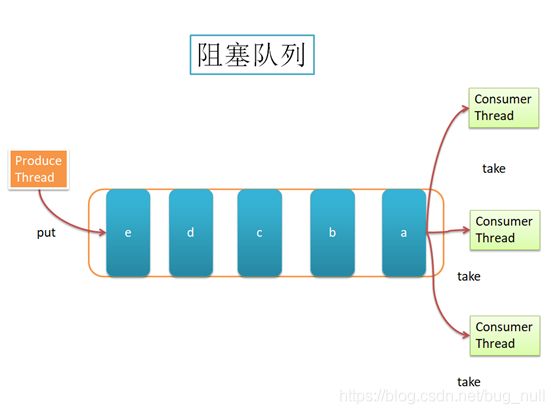
- JDK1.5 生产消费者
bijian1013
javathread生产消费者java多线程
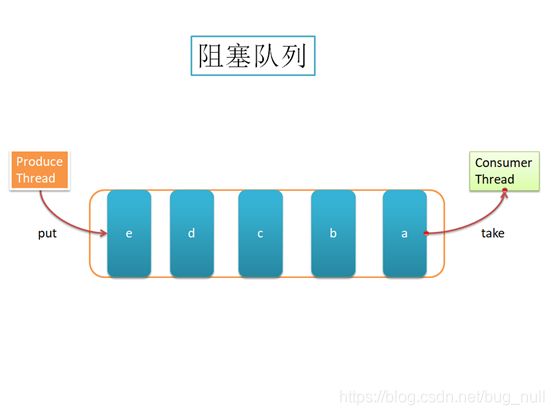
ArrayBlockingQueue:
一个由数组支持的有界阻塞队列。此队列按 FIFO(先进先出)原则对元素进行排序。队列的头部 是在队列中存在时间最长的元素。队列的尾部 是在队列中存在时间最短的元素。新元素插入到队列的尾部,队列检索操作则是从队列头部开始获得元素。
ArrayBlockingQueue的常用方法:
- JAVA版身份证获取性别、出生日期及年龄
bijian1013
java性别出生日期年龄
工作中需要根据身份证获取性别、出生日期及年龄,且要还要支持15位长度的身份证号码,网上搜索了一下,经过测试好像多少存在点问题,干脆自已写一个。
CertificateNo.java
package com.bijian.study;
import java.util.Calendar;
import
- 【Java范型六】范型与枚举
bit1129
java
首先,枚举类型的定义不能带有类型参数,所以,不能把枚举类型定义为范型枚举类,例如下面的枚举类定义是有编译错的
public enum EnumGenerics<T> { //编译错,提示枚举不能带有范型参数
OK, ERROR;
public <T> T get(T type) {
return null;
- 【Nginx五】Nginx常用日志格式含义
bit1129
nginx
1. log_format
1.1 log_format指令用于指定日志的格式,格式:
log_format name(格式名称) type(格式样式)
1.2 如下是一个常用的Nginx日志格式:
log_format main '[$time_local]|$request_time|$status|$body_bytes
- Lua 语言 15 分钟快速入门
ronin47
lua 基础
-
-
单行注释
-
-
[[
[多行注释]
-
-
]]
-
-
-
-
-
-
-
-
-
-
-
1.
变量 & 控制流
-
-
-
-
-
-
-
-
-
-
num
=
23
-
-
数字都是双精度
str
=
'aspythonstring'
- java-35.求一个矩阵中最大的二维矩阵 ( 元素和最大 )
bylijinnan
java
the idea is from:
http://blog.csdn.net/zhanxinhang/article/details/6731134
public class MaxSubMatrix {
/**see http://blog.csdn.net/zhanxinhang/article/details/6731134
* Q35
求一个矩阵中最大的二维
- mongoDB文档型数据库特点
开窍的石头
mongoDB文档型数据库特点
MongoDD: 文档型数据库存储的是Bson文档-->json的二进制
特点:内部是执行引擎是js解释器,把文档转成Bson结构,在查询时转换成js对象。
mongoDB传统型数据库对比
传统类型数据库:结构化数据,定好了表结构后每一个内容符合表结构的。也就是说每一行每一列的数据都是一样的
文档型数据库:不用定好数据结构,
- [毕业季节]欢迎广大毕业生加入JAVA程序员的行列
comsci
java
一年一度的毕业季来临了。。。。。。。。
正在投简历的学弟学妹们。。。如果觉得学校推荐的单位和公司不适合自己的兴趣和专业,可以考虑来我们软件行业,做一名职业程序员。。。
软件行业的开发工具中,对初学者最友好的就是JAVA语言了,网络上不仅仅有大量的
- PHP操作Excel – PHPExcel 基本用法详解
cuiyadll
PHPExcel
导出excel属性设置//Include classrequire_once('Classes/PHPExcel.php');require_once('Classes/PHPExcel/Writer/Excel2007.php');$objPHPExcel = new PHPExcel();//Set properties 设置文件属性$objPHPExcel->getProperties
- IBM Webshpere MQ Client User Issue (MCAUSER)
darrenzhu
IBMjmsuserMQMCAUSER
IBM MQ JMS Client去连接远端MQ Server的时候,需要提供User和Password吗?
答案是根据情况而定,取决于所定义的Channel里面的属性Message channel agent user identifier (MCAUSER)的设置。
http://stackoverflow.com/questions/20209429/how-mca-user-i
- 网线的接法
dcj3sjt126com
一、PC连HUB (直连线)A端:(标准568B):白橙,橙,白绿,蓝,白蓝,绿,白棕,棕。 B端:(标准568B):白橙,橙,白绿,蓝,白蓝,绿,白棕,棕。 二、PC连PC (交叉线)A端:(568A): 白绿,绿,白橙,蓝,白蓝,橙,白棕,棕; B端:(标准568B):白橙,橙,白绿,蓝,白蓝,绿,白棕,棕。 三、HUB连HUB&nb
- Vimium插件让键盘党像操作Vim一样操作Chrome
dcj3sjt126com
chromevim
什么是键盘党?
键盘党是指尽可能将所有电脑操作用键盘来完成,而不去动鼠标的人。鼠标应该说是新手们的最爱,很直观,指哪点哪,很听话!不过常常使用电脑的人,如果一直使用鼠标的话,手会发酸,因为操作鼠标的时候,手臂不是在一个自然的状态,臂肌会处于绷紧状态。而使用键盘则双手是放松状态,只有手指在动。而且尽量少的从鼠标移动到键盘来回操作,也省不少事。
在chrome里安装 vimium 插件
- MongoDB查询(2)——数组查询[六]
eksliang
mongodbMongoDB查询数组
MongoDB查询数组
转载请出自出处:http://eksliang.iteye.com/blog/2177292 一、概述
MongoDB查询数组与查询标量值是一样的,例如,有一个水果列表,如下所示:
> db.food.find()
{ "_id" : "001", "fruits" : [ "苹
- cordova读写文件(1)
gundumw100
JavaScriptCordova
使用cordova可以很方便的在手机sdcard中读写文件。
首先需要安装cordova插件:file
命令为:
cordova plugin add org.apache.cordova.file
然后就可以读写文件了,这里我先是写入一个文件,具体的JS代码为:
var datas=null;//datas need write
var directory=&
- HTML5 FormData 进行文件jquery ajax 上传 到又拍云
ileson
jqueryAjaxhtml5FormData
html5 新东西:FormData 可以提交二进制数据。
页面test.html
<!DOCTYPE>
<html>
<head>
<title> formdata file jquery ajax upload</title>
</head>
<body>
<
- swift appearanceWhenContainedIn:(version1.2 xcode6.4)
啸笑天
version
swift1.2中没有oc中对应的方法:
+ (instancetype)appearanceWhenContainedIn:(Class <UIAppearanceContainer>)ContainerClass, ... NS_REQUIRES_NIL_TERMINATION;
解决方法:
在swift项目中新建oc类如下:
#import &
- java实现SMTP邮件服务器
macroli
java编程
电子邮件传递可以由多种协议来实现。目前,在Internet 网上最流行的三种电子邮件协议是SMTP、POP3 和 IMAP,下面分别简单介绍。
◆ SMTP 协议
简单邮件传输协议(Simple Mail Transfer Protocol,SMTP)是一个运行在TCP/IP之上的协议,用它发送和接收电子邮件。SMTP 服务器在默认端口25上监听。SMTP客户使用一组简单的、基于文本的
- mongodb group by having where 查询sql
qiaolevip
每天进步一点点学习永无止境mongo纵观千象
SELECT cust_id,
SUM(price) as total
FROM orders
WHERE status = 'A'
GROUP BY cust_id
HAVING total > 250
db.orders.aggregate( [
{ $match: { status: 'A' } },
{
$group: {
- Struts2 Pojo(六)
Luob.
POJOstrust2
注意:附件中有完整案例
1.采用POJO对象的方法进行赋值和传值
2.web配置
<?xml version="1.0" encoding="UTF-8"?>
<web-app version="2.5"
xmlns="http://java.sun.com/xml/ns/javaee&q
- struts2步骤
wuai
struts
1、添加jar包
2、在web.xml中配置过滤器
<filter>
<filter-name>struts2</filter-name>
<filter-class>org.apache.st