java之实现十大排序算法,时间复杂度以及横向对比
数据结构十大排序算法的java实现
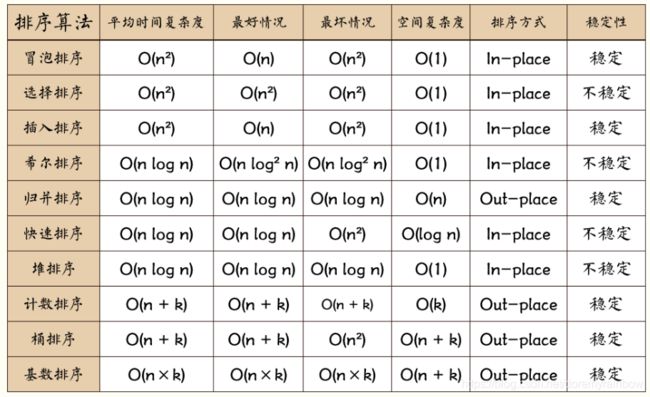
十大排序算法:冒泡排序,选择排序,插入排序,希尔排序,归并排序,快速排序,堆排序,计数排序,桶排序,基数排序。
排序又分内部排序和外部排序
内部排序:所有排序操作都在内存中完成;
外部排序:由于数据太大,因此把数据放在磁盘中,而排序通过磁盘和内存的数据传输才能进行
文章目录
- 数据结构十大排序算法的java实现
- 1.冒泡排序
- 1.1冒泡排序算法
- 1.2冒泡排序过程动态图演示
- 1.3Java代码实现冒泡排序
- 2.选择排序
- 1.1选择排序
- 1.2选择排序动态图演示
- 1.3选择排序java实现
- 3.插入排序
- 3.1插入排序
- 3.2插入排序算法动图演示
- 3.3插入排序算法Java实现
- 4.希尔排序
- 4.1.希尔排序
- 4.2希尔排序算法Java实现
- 5.归并排序
- 5.1归并排序算法
- 5.2归并排序算法Java实现
- 6.快速排序
- 6.1快速排序算法
- 6.2.快速排序算法动图演示
- 6.3.快速排序算法Java实现
- 7.堆排序
- 7.1堆排序算法
- 7.2堆排序算法Java实现
- 8.计数排序
- 8.1计数排序算法
- 8.2计数排序算法动图演示
- 8.2.计数排序算法java实现
- 9.桶排序
- 9.1.桶排序算法
- 9.2.桶排序算法Java实现
- 10.基数排序
- 10.1.基数排序算法
- 10.2.基数排序算法Java实现
- 十大排序算法时间空间复杂度
1.冒泡排序
1.1冒泡排序算法
冒泡排序是一种简单的排序算法,一次比较两个相邻元素,如果它们的顺序错误就换过来,最终将数组按一定顺序(大到小,或者小到大)列出来
1.2冒泡排序过程动态图演示
1.3Java代码实现冒泡排序
package tensort;
public class bub {
/*
* 冒泡排序算法
*/
public static void bubling(int[] a){
int t=0,i,j;
for(i = 0;i < a.length;i++){
for(j = 0;j < a.length-1-i;j++){
if(a[j+1] < a[j]) {
t = a[j+1];
a[j+1] = a[j];
a[j] = t;
}
}
}
for(i = 0; i < a.length; i++){
if(i==a.length-1){
System.out.print(a[i]);
}else{
System.out.print(a[i]+",");
}
}
}
}
package tensort;
public class bubling {
public static void main(String[] args) {
int a[]={3,44,38,5,47,15,36,26,27,2,46,4,19,50,48};
bub b = new bub();
b.bubling(a);
}
}
2.选择排序
1.1选择排序
选择排序是一种简单直观的排序算法,这个算法是从一个未排序的数组里面找出一个最大值或者最小值放在一端,然后以此类推,完成排序的原理。
1.2选择排序动态图演示
1.3选择排序java实现
package tensort;
public class select {
/*
* 选择排序算法
*/
public static void select(int[] a){
int i,j,k = 0,t;
for(j = 0;j < a.length;j++){
a[k] = a[j];
for(i = j+1; i < a.length;i++){
if(a[k]>a[i]){
a[k] = a[i];
}
}
k++;
}
for(i = 0; i < a.length; i++){
if(i==a.length-1){
System.out.print(a[i]);
}else{
System.out.print(a[i]+",");
}
}
}
}
package tensort;
public class bubling {
public static void main(String[] args) {
int a[]={3,44,38,5,47,15,36,26,27,2,46,4,19,50,48};
select s = new select();
s.select(a);
}
}
}
3.插入排序
3.1插入排序
插入排序是一种比较简单的排序算法,其过程主要是在一个数组中,默认第二个数为最大值或者最小值,然后跟后面的元素比较,如果排序不对就换过来,如果正确的就往后比较一直比较找出最大值或者最小值,以此类推获得排序好了的数组
3.2插入排序算法动图演示
3.3插入排序算法Java实现
package tensort;
public class insert {
/*
* 插入排序算法
*/
public static void insert(int[] a) {
int s,i,index;
for (i = 0; i < a.length - 1; i++) {
s = a[i + 1];
index = i;
while (index >= 0 && s < a[index]) {
a[index + 1] = a[index];
index--;
}
a[index + 1] = s;
}
for(i = 0; i < a.length; i++){
if(i==a.length-1){
System.out.print(a[i]);
}else{
System.out.print(a[i]+",");
}
}
}
}
package tensort;
public class bubling {
public static void main(String[] args) {
insert i = new insert();
i.insert(a);
}
}
4.希尔排序
4.1.希尔排序
希尔排序也是一种插入排序,它是简单插入排序经过改进之后的一个更高效的版本,也称为缩小增量排序。它与插入排序的不同之处在于,它会优先比较距离较远的元素。希尔排序又叫缩小增量排序。
4.2希尔排序算法Java实现
public class Shell{
/*
* 插入排序算法
*/
public static int[] Shell(int[] a) {
int i,temp, gap,index = a.length / 2;
while (gap > 0) {
for (i = gap; i < a.length; i++) {
temp = a[i];
index= i - gap;
while (index>= 0 && a[index] > temp) {
a[index + gap] = a[index];
index-= gap;
}
a[index+ gap] = temp;
}
gap /= 2;
}
}
for(i = 0; i < a.length; i++){
if(i==a.length-1){
System.out.print(a[i]);
}else{
System.out.print(a[i]+",");
}
}
}
5.归并排序
5.1归并排序算法
归并排序和选择排序一样,归并排序的性能不受输入数据的影响,但表现比选择排序好的多。但是需要额外的内存空间。
归并排序是建立在归并操作上的一种有效的排序算法。该算法是采用分治法的一个非常典型的应用。归并排序是一种稳定的排序方法。将已有序的子序列合并,得到完全有序的序列;即先使每个子序列有序,再使子序列段间有序。若将两个有序表合并成一个有序表,称为2-路归并。
5.2归并排序算法Java实现
/**
* 归并排序
*/
public static void MergeSort(int[] array) {
if (array.length < 2) return array;
int mid = array.length / 2;
int[] left = Arrays.copyOfRange(array, 0, mid);
int[] right = Arrays.copyOfRange(array, mid, array.length);
return merge(MergeSort(left), MergeSort(right));
}
/**
* 归并排序——将两段排序好的数组结合成一个排序数组
*/
public static int[] merge(int[] left, int[] right) {
int[] result = new int[left.length + right.length];
for (int index = 0, i = 0, j = 0; index < result.length; index++) {
if (i >= left.length)
result[index] = right[j++];
else if (j >= right.length)
result[index] = left[i++];
else if (left[i] > right[j])
result[index] = right[j++];
else
result[index] = left[i++];
}
}
6.快速排序
6.1快速排序算法
快速排序的基本思想:通过一趟排序将待排记录分隔成独立的两部分,其中一部分记录的关键字均比另一部分的关键字小,则可分别对这两部分记录继续进行排序,以达到整个序列有序。
6.2.快速排序算法动图演示
6.3.快速排序算法Java实现
/**
* 快速排序方法
*/
public static void QuickSort(int[] array, int start, int end) {
if (array.length < 1 || start < 0 || end >= array.length || start > end) return null;
int smallIndex = partition(array, start, end);
if (smallIndex > start)
QuickSort(array, start, smallIndex - 1);
if (smallIndex < end)
QuickSort(array, smallIndex + 1, end);
}
/**
* 快速排序算法——partition
*/
public static int partition(int[] array, int start, int end) {
int pivot = (int) (start + Math.random() * (end - start + 1));
int smallIndex = start - 1;
swap(array, pivot, end);
for (int i = start; i <= end; i++)
if (array[i] <= array[end]) {
smallIndex++;
if (i > smallIndex)
swap(array, i, smallIndex);
}
}
/**
* 交换数组内两个元素
*/
public static void swap(int[] array, int i, int j) {
int temp = array[i];
array[i] = array[j];
array[j] = temp;
}
7.堆排序
7.1堆排序算法
堆排序是指利用堆这种数据结构所设计的一种排序算法。堆积是一个近似完全二叉树的结构,并同时满足堆积的性质:即子结点的键值或索引总是小于(或者大于)它的父节点。
7.2堆排序算法Java实现
//声明全局变量,用于记录数组array的长度;
static int len;
/**
* 堆排序算法
*/
public static void HeapSort(int[] array) {
len = array.length;
if (len < 1) return array;
//1.构建一个最大堆
buildMaxHeap(array);
//2.循环将堆首位(最大值)与末位交换,然后在重新调整最大堆
while (len > 0) {
swap(array, 0, len - 1);
len--;
adjustHeap(array, 0);
}
}
/**
* 建立最大堆
*
* @param array
*/
public static void buildMaxHeap(int[] array) {
//从最后一个非叶子节点开始向上构造最大堆
for (int i = (len - 1) / 2; i >= 0; i--) {
adjustHeap(array, i);
}
}
/**
* 调整使之成为最大堆
*
* @param array
* @param i
*/
public static void adjustHeap(int[] array, int i) {
int maxIndex = i;
//如果有左子树,且左子树大于父节点,则将最大指针指向左子树
if (i * 2 < len && array[i * 2] > array[maxIndex])
maxIndex = i * 2;
//如果有右子树,且右子树大于父节点,则将最大指针指向右子树
if (i * 2 + 1 < len && array[i * 2 + 1] > array[maxIndex])
maxIndex = i * 2 + 1;
//如果父节点不是最大值,则将父节点与最大值交换,并且递归调整与父节点交换的位置。
if (maxIndex != i) {
swap(array, maxIndex, i);
adjustHeap(array, maxIndex);
}
}
8.计数排序
8.1计数排序算法
计数排序的核心在于将输入的数据值转化为键存储在额外开辟的数组空间中。 作为一种线性时间复杂度的排序,计数排序要求输入的数据必须是有确定范围的整数。
计数排序是一种稳定的排序算法。计数排序使用一个额外的数组C,其中第i个元素是待排序数组A中值等于i的元素的个数。然后根据数组C来将A中的元素排到正确的位置。它只能对整数进行排序。
8.2计数排序算法动图演示
8.2.计数排序算法java实现
/**
* 计数排序
*/
public static void CountingSort(int[] array) {
if (array.length == 0) return array;
int bias, min = array[0], max = array[0];
for (int i = 1; i < array.length; i++) {
if (array[i] > max)
max = array[i];
if (array[i] < min)
min = array[i];
}
bias = 0 - min;
int[] bucket = new int[max - min + 1];
Arrays.fill(bucket, 0);
for (int i = 0; i < array.length; i++) {
bucket[array[i] + bias]++;
}
int index = 0, i = 0;
while (index < array.length) {
if (bucket[i] != 0) {
array[index] = i - bias;
bucket[i]--;
index++;
} else
i++;
}
}
9.桶排序
9.1.桶排序算法
桶排序是计数排序的升级版。它利用了函数的映射关系,高效与否的关键就在于这个映射函数的确定。
桶排序的工作的原理:假设输入数据服从均匀分布,将数据分到有限数量的桶里,每个桶再分别排序(有可能再使用别的排序算法或是以递归方式继续使用桶排序进行排序)
9.2.桶排序算法Java实现
在这里插入代码片/**
* 桶排序
*/
public static ArrayList BucketSort(ArrayList array, int bucketSize) {
if (array == null || array.size() < 2)
return array;
int max = array.get(0), min = array.get(0);
// 找到最大值最小值
for (int i = 0; i < array.size(); i++) {
if (array.get(i) > max)
max = array.get(i);
if (array.get(i) < min)
min = array.get(i);
}
int bucketCount = (max - min) / bucketSize + 1;
ArrayList> bucketArr = new ArrayList<>(bucketCount);
ArrayList resultArr = new ArrayList<>();
for (int i = 0; i < bucketCount; i++) {
bucketArr.add(new ArrayList());
}
for (int i = 0; i < array.size(); i++) {
bucketArr.get((array.get(i) - min) / bucketSize).add(array.get(i));
}
for (int i = 0; i < bucketCount; i++) {
if (bucketCount == 1)
bucketSize--;
ArrayList temp = BucketSort(bucketArr.get(i), bucketSize);
for (int j = 0; j < temp.size(); j++)
resultArr.add(temp.get(j));
}
return resultArr;
}
10.基数排序
10.1.基数排序算法
基数排序是按照低位先排序,然后收集;再按照高位排序,然后再收集;依次类推,直到最高位。有时候有些属性是有优先级顺序的,先按低优先级排序,再按高优先级排序。最后的次序就是高优先级高的在前,高优先级相同的低优先级高的在前。基数排序基于分别排序,分别收集,所以是稳定的。
10.2.基数排序算法Java实现
/**
* 基数排序
*/
public static int[] RadixSort(int[] array) {
if (array == null || array.length < 2)
return array;
// 1.先算出最大数的位数;
int max = array[0];
for (int i = 1; i < array.length; i++) {
max = Math.max(max, array[i]);
}
int maxDigit = 0;
while (max != 0) {
max /= 10;
maxDigit++;
}
int mod = 10, div = 1;
ArrayList> bucketList = new ArrayList>();
for (int i = 0; i < 10; i++)
bucketList.add(new ArrayList());
for (int i = 0; i < maxDigit; i++, mod *= 10, div *= 10) {
for (int j = 0; j < array.length; j++) {
int num = (array[j] % mod) / div;
bucketList.get(num).add(array[j]);
}
int index = 0;
for (int j = 0; j < bucketList.size(); j++) {
for (int k = 0; k < bucketList.get(j).size(); k++)
array[index++] = bucketList.get(j).get(k);
bucketList.get(j).clear();
}
}
return array;
}
十大排序算法时间空间复杂度
以及横向对比
横向比较一般是在某一时间点上的同层次的比较,纵向比较是单个事物与过去某个时间的状态进行比较。 横向比较法是指对同类的不同对象在统一标准下进行比较的方法。使用该方法按着调研目的确定比较基准和分类属性、要注意事物的可比性、要善于发现和比较本质的不同。