POJ 3905 Perfect Election (2-Sat)
In a country (my memory fails to say which), the candidates {1, 2 …, N} are running in the parliamentary election. An opinion poll asks the question “For any two candidates of your own choice, which election result would make you happy?”. The accepted answers are shown in the table below, where the candidates i and j are not necessarily different, i.e. it may happen that i=j. There are M poll answers, some of which may be similar or identical. The problem is to decide whether there can be an election outcome (It may happen that all candidates fail to be elected, or all are elected, or only a part of them are elected. All these are acceptable election outcomes.) that conforms to all M answers. We say that such an election outcome is perfect. The result of the problem is 1 if a perfect election outcome does exist and 0 otherwise.
Input
Each data set corresponds to an instance of the problem and starts with two integral numbers: 1≤N≤1000 and 1≤M≤1000000. The data set continues with M pairs ±i ±j of signed numbers, 1≤i,j≤N. Each pair encodes a poll answer as follows:
Accepted answers to the poll question Encoding
I would be happy if at least one from i and j is elected. +i +j
I would be happy if at least one from i and j is not elected. -i -j
I would be happy if i is elected or j is not elected or both events happen. +i -j
I would be happy if i is not elected or j is elected or both events happen. -i +j
The input data are separated by white spaces, terminate with an end of file, and are correct.
Output
For each data set the program prints the result of the encoded election problem. The result, 1 or 0, is printed on the standard output from the beginning of a line. There must be no empty lines on output.
Sample Input
3 3 +1 +2 -1 +2 -1 -3
2 3 -1 +2 -1 -2 +1 -2
2 4 -1 +2 -1 -2 +1 -2 +1 +2
2 8 +1 +2 +2 +1 +1 -2 +1 -2 -2 +1 -1 +1 -2 -2 +1 -1
Sample Output
1
1
0
1
Hint
For the first data set the result of the problem is 1; there are several perfect election outcomes, e.g. 1 is not elected, 2 is elected, 3 is not elected. The result for the second data set is justified by the perfect election outcome: 1 is not elected, 2 is not elected. The result for the third data set is 0. According to the answers -1 +2 and -1 -2 the candidate 1 must not be elected, whereas the answers +1 -2 and +1 +2 say that candidate 1 must be elected. There is no perfect election outcome. For the fourth data set notice that there are similar or identical poll answers and that some answers mention a single candidate. The result is 1.
题意:民众投票选举,每个人投两票(可以是某人被选也可以是某人不被选),选举结果使得每个人这两票至少有一票满足,则称选举结果是完美的,先在给出每人的投票情况问是否存在完美的投票结果
思路:
2-SAT问题
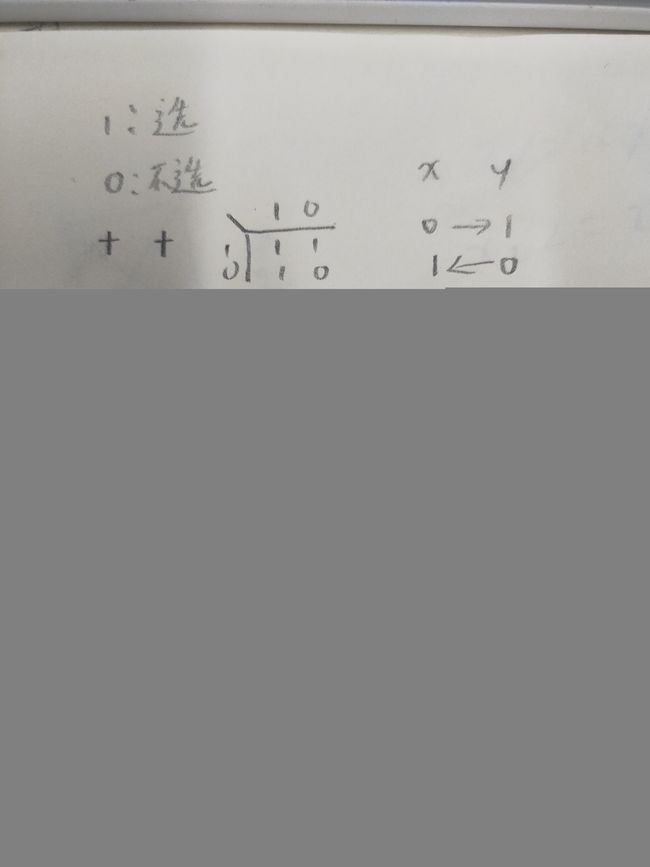 AC代码:
AC代码:
#include
#include
#include
#include
#include
#include
#include
#include
using namespace std;
const int maxn =56000;
const int maxm =8000100;
const int inf = 0x3f3f3f3f;
typedef long long ll;
struct node
{
int v, next;
}edge[maxm];
int head[maxn], dfn[maxn], low[maxn], instack[maxn], Stack[maxn], belong[maxn];
int n,m, tot, index, top, cnt;
void addedge(int u, int v)
{
edge[tot].v = v;
edge[tot].next = head[u];
head[u] = tot++;
return;
}
void tarjan(int u)
{
dfn[u] = low[u] = ++index;
instack[u] = 1;
Stack[top++] = u;
for (int i = head[u]; i != -1; i = edge[i].next)
{
int v = edge[i].v;
if (!dfn[v])
{
tarjan(v);
low[u] = min(low[u], low[v]);
}
else if (instack[v])
{
low[u] = min(low[u], dfn[v]);
}
}
if (low[u] == dfn[u])
{
cnt++;
int v;
do
{
v = Stack[--top];
instack[v] = 0;
belong[v] = cnt;
} while (u != v);
}
}
int main()
{
while (scanf("%d%d", &n,&m) != EOF)
{
memset(head, -1, sizeof(head));
memset(dfn, 0, sizeof(dfn));
tot=index =0;
top = 0;
cnt = 0;
for (int i = 0; i < m; i++)
{
int x,y,a,b;
scanf("%d%d",&a,&b);
x=abs(a);
y=abs(b);
if(a>0&&b>0)
{
addedge(y+n,x);
addedge(x+n,y);
}
if(a<0&&b>0)
{
addedge(y+n,x+n);
addedge(x,y);
}
if(a<0&&b<0)
{
addedge(x,y+n);
addedge(y,x+n);
}
if(a>0&&b<0)
{
addedge(y,x);
addedge(x+n,y+n);
}
}
for (int i =1; i <= n << 1; i++)
{
if (!dfn[i])
{
tarjan(i);
}
}
int flag =0;
for (int i =1;i<=n; i++)
{
if (belong[i] == belong[i+n])
{
flag =1;
}
}
if (flag)
{
printf("0\n");
}
else
{
printf("1\n");
}
}
return 0;
}
注意:这个题卡空间,点集和边集数组大小要分开定义