- 探索约数:试除法,约数之和,最大公约数
Lostgreen
数据结构&算法算法最大公约数
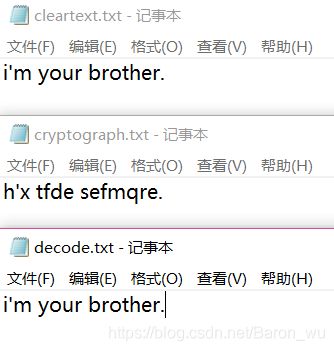
引言约数(Divisor)是数论中的基本概念之一,指能够整除某个数的整数。约数在数学、计算机科学和密码学中有着广泛的应用。本文将详细介绍约数的相关知识,包括试除法求约数、最大公约数算法(如辗转相除法和更相减损术),并阐明这些算法的原理和步骤。1.试除法求约数1.1算法原理试除法是一种简单直观的求约数的方法。对于一个数nnn,如果ddd是nnn的约数,则nnn能被ddd整除。通过遍历1到n\sqrt
- 零基础转行学网络安全怎么样?能找到什么样的工作?
程序员霸哥
网络安全程序员编程web安全安全网络php服务器pythonjava
网络安全对于现代社会来说变得越来越重要,但是很多人对于网络安全的知识却知之甚少。那么,零基础小白可以学网络安全吗?答案是肯定的。零基础转行学习网络安全是完全可行的,但需要明确的是,网络安全是一个既广泛又深入的领域,包含了网络协议、系统安全、应用安全、密码学、渗透测试、漏洞挖掘、安全编程、安全运维等多个方面。。网络安全是一个快速发展的领域,对专业人才的需求不断增长。以下是一些关于零基础转行学习网络安
- openssl源码编译输出库-guidance-傻瓜式教程
代码改变世界ctw
密码学实践强化训练openssl命令行加解密
快速链接:.个人博客笔记导读目录(全部)付费专栏-付费课程【购买须知】:密码学实践强化训练–【目录】目标:下载openssl源码编译输出目标版本,例如使用AndroidNDK编译输出Android使用的32位的库1、下载源码gitclonehttps://github.com/openssl/openssl.git-bopenssl-3.0.9</
- Python下PennyLane构建量子线路:原理、实践与应用
Allen_LVyingbo
量子计算与量子学习python量子计算开发语言
一、引言1.1研究背景与意义量子计算作为当今科技领域的前沿热点,具有突破传统计算限制的巨大潜力,有望在诸多复杂问题的处理上带来革命性的突破。它基于量子力学原理,利用量子比特(qubit)作为信息存储和处理的基本单元,相较于经典比特,能够实现更为强大的信息处理能力。例如,Shor算法在理论上可实现对大整数的快速分解,这对现代密码学产生了深远影响;Grover算法则能在无序数据库中实现快速搜索,大幅提
- Web3时代的开放之门:区块链技术与创新生态
dingzd95
web3区块链物联网
随着区块链技术的蓬勃发展,Web3时代正逐渐到来,带来了对互联网和数字经济的全新思考。在这个时代,区块链不再是简单的加密货币技术,而是一种基础设施,可以支持更广泛的应用场景,重新定义着我们与数字世界的互动方式。本文将探讨Web3时代的开启,以及区块链技术如何促进创新生态的形成。区块链技术的本质区块链技术是一种去中心化的分布式账本技术,通过将数据存储在多个节点上,并使用密码学技术确保数据的安全性和完
- solidity 数学和密码学函数
龑行天下
密码学solidity
数学和密码学函数为开发者提供了一系列强大的工具,用于执行各种数学运算和加密操作addmod(uintx,uinty,uintk)returns(uint)计算(x+y)%k,加法会在任意精度下执行,并且加法的结果即使超过2**256也不会被截取。从0.5.0版本的编译器开始会加入对k!=0的校验(assert)。//SPDX-License-Identifier:MITpragmasolidity
- 了解比特币
lpl还在学习的路上
区块链
比特币(Bitcoin,简称BTC)是世界上第一种去中心化的数字货币,由中本聪(SatoshiNakamoto)在2008年提出,并于2009年正式上线。比特币的核心特点是去中心化、匿名性和稀缺性,它不依赖任何中央机构发行或管理,而是通过区块链技术和密码学确保其安全性和可信度。以下是关于比特币的详细介绍:1.比特币的核心特点去中心化:比特币没有中央发行机构或管理机构,所有交易由全球节点共同维护。匿
- 区块链的数学基础:核心原理与应用解析
silver687
区块链
区块链技术的核心原理和应用离不开其强大的数学基础,以下是对其数学基础、核心原理与应用的详细解析:区块链的数学基础区块链的数学基础主要包括以下几个核心领域:1.密码学:密码学是区块链安全性的基石,主要保障数据的机密性、完整性和不可抵赖性。其中,对称加密算法(如AES)加密和解密使用相同密钥,计算效率高,但不适用于区块链的公开网络环境;非对称加密使用一对密钥(公钥和私钥),用户通过私钥签名交易,其他人
- 【密码学基础】RSA加密算法
Mr.zwX
隐私计算及密码学基础密码学安全
1RSA介绍RSA是一种非对称加密算法,即加密和解密时用到的密钥不同。加密密钥是公钥,可以公开;解密密钥是私钥,必须保密保存。基于一个简单的数论事实:两个大质数相乘很容易,但想要对其乘积进行因式分解却极其困难,因此可以将乘积公开作为加密密钥,即公钥;而两个大质数组合成私钥。2密钥对的生成step1生成N(公钥和私钥的一部分)首先选取两个互为质数的数ppp和qqq(p≠q,gcd(p,q)=1p\n
- CTF密码学常见加密及解密脚本二
mist1star
密码学开发语言
一.摩斯密码摩斯密码的介绍:由美国人萨缪尔·摩尔斯(SamuelMorse)及其助手阿尔弗雷德·维尔(AlfredVail)在1836年发明的。摩斯密码的原理基于两种基本信号:点和划(或称为短音和长音),通过它们的组合来表示字母、数字和符号。摩斯密码的基本原理:1.点和划点(·):最短的信号,表示一个短音划(-):较长的信号,表示一个长音2.间隔字母间间隔:表示两个字母之间的间隔,通常是三个点的长
- 浅谈隐私计算
eso1983
python安全
1.隐私计算概述隐私计算是指在保护数据本身不对外泄露的前提下,实现数据的计算和分析的一系列信息技术。随着数据成为重要的生产要素,数据的流通与融合需求日益增长,但数据隐私安全问题也愈发突出。隐私计算技术旨在平衡数据的价值挖掘与隐私保护,为数据的安全使用提供解决方案。隐私计算涉及到多个主要的关键技术:多方安全计算:基于密码学原理,允许多个参与方在不泄露各自私有数据的情况下,协同计算某个函数。各方将各自
- 《深入浅出HTTPS》读书笔记(7):安全的密码学Hash算法
earthzhang2021
httpshttp网络协议网络1024程序员节
密码学Hash算法除了常规Hash算法的特性,还应该具备下面三个特性。1)强抗碰撞性(CollisionResistance)如果两个不相同的值能够得到同样的摘要值,表示产生了Hash碰撞。密码学中,Hash算法必须具备强抗碰撞性,否则不应该使用。2)弱抗碰撞性(Secondpre-imageResistance)给定一个消息和这个消息对应的摘要值,很难找到一条不同的消息也具有相同的摘要值。如果某
- 《深入浅出HTTPS》读书笔记(5):随机数
earthzhang2021
https网络协议http
密码学中随机数的用途非常大,其他密码学算法内部都会用到随机数。1)效率在软件或者密码学应用中需要大量的随机数,必须在很短的时间内生成随机数。2)随机性生成的随机数只要不存在统计学偏差,那么这个随机数就具备随机性(randomness)。3)不可预测性密码学中的随机数必须具备不可预测性,否则就会存在安全问题,当然非密码学应用使用具备随机性的随机数就足够了。4)不可重现性所谓不可重现性(unrepea
- Python的加密与解密_pyarmor解码
2401_84584583
程序员python网络安全
随着信息化和数字化社会的发展,人们对信息安全和保密的重要性认识不断提高,于是在1997年,美国国家标准局公布实施了“美国数据加密标准(DES)”,民间力量开始全面介入密码学的研究和应用中,采用的加密算法有DES、RSA、SHA等。随着对加密强度需求的不断提高,近期又出现了AES、ECC等。使用密码学可以达到以下目的:保密性:防止用户的标识或数据被读取。数据完整性:防止数据被更改。身份验证:确保数据
- 《深入浅出HTTPS》读书笔记(30):OpenSSL和TLS
earthzhang2021
https1024程序员节开发语言算法网络
《深入浅出HTTPS》读书笔记(30):OpenSSL和TLS通过两个维度了解OpenSSL,首先OpenSSL是一个底层密码库,封装了所有的密码学算法、证书管理、TLS/SSL协议实现。OpenSSL库包含两种类型的库。◎crypto库函数:具体的密码学算法使用库,比如MD5、RSA、DES算法的实现,开发者可以直接使用这些库,可以理解为底层次库。◎EVP接口:高层次库,基于crypto库函数做
- python上一个很好用的密码学库——PyCryptodome
UN_spoken
pythonpycryptodomepython
1.关于安装不要再使用PyCrypto这个库了,因为这个库三年没有维护了,因此使用它的替代库——PyCryptodome,方法都差不多,安装方法也比较简单,使用下面的命令就可以安装了:pip3installpycryptodome如果之前安装了PyCrypto库,那么,请使用下面的命令进行卸载:pipuninstallpycrypto2.关于使用
- 【Numpy核心编程攻略:Python数据处理、分析详解与科学计算】1.24 随机宇宙:生成现实世界数据的艺术
精通代码大仙
numpypythonnumpypython开发语言
1.24随机宇宙:生成现实世界数据的艺术目录随机宇宙:生成现实世界数据的艺术引言复杂联合分布的采样技巧随机游走的蒙特卡洛实现基于物理规律的生成模型随机数在加密中的应用总结参考文献引言复杂联合分布的采样技巧随机游走的蒙特卡洛实现基于物理规律的生成模型随机数在加密中的应用总结参考文献随机数生成分布采样物理模拟密码学应用多元正态分布随机过程布朗运动流体动力学安全随机数随机性检验1.24.1引言在数据科学
- 软件开发中的密码学(国密算法)
自己的九又四分之三站台
#软件架构师的“不归之路“密码学算法
1.软件行业中的加解密在软件行业中,加解密技术广泛应用于数据保护、通信安全、身份验证等多个领域。加密(Encryption)是将明文数据转换为密文的过程,而解密(Decryption)则是将密文恢复为明文的过程。以下是加解密在软件行业中一些常见的应用和技术:1.1.对称加密与非对称加密对称加密:加密和解密使用相同的密钥。常见算法包括AES(高级加密标准)、DES(数据加密标准)、3DES(Trip
- 第30篇:Python开发进阶:网络安全与测试
猿享天开
python从入门到精通pythonweb安全开发语言
第30篇:网络安全与测试目录网络安全概述什么是网络安全常见的安全威胁Python中的网络安全工具常用安全库介绍安全编码实践密码学基础加密与解密哈希函数数字签名安全认证与授权用户认证访问控制OAuth与JWTWeb应用安全常见的Web安全漏洞防护措施安全测试网络安全测试渗透测试自动化测试工具安全漏洞扫描使用Python进行安全测试使用Scapy进行网络嗅探使用Requests进行安全测试使用Beau
- 【13】地址-比特币区块链的地址
AlieNeny
从零到一开发自己的区块链区块链分布式账本哈希算法
1.比特币区块链的地址这就是一个真实的比特币地址:1A1zP1eP5QGefi2DMPTfTL5SLmv7DivfNa。这是史上第一个比特币地址,据说属于中本聪。比特币地址是完全公开的,如果你想要给某个人发送币,只需要知道他的地址就可以了。实际上,所谓的地址,只不过是将公钥表示成人类可读的形式而已。2.密码学相关算法和概念
- 密码学领域三大经典难题:DLP、IFP 与 ECDLP
软件职业规划
经典难题密码学网络服务器
离散对数问题(DLP)基本概念:在有限循环群GGG(通常是整数模ppp乘法群Zp∗Z_p^*Zp∗,其中ppp为素数)中,给定一个生成元ggg和元素h=gxh=g^xh=gx(xxx为整数),离散对数问题是求出整数xxx。例如,在群Z17∗Z_{17}^*Z17∗中,生成元g=3g=3g=3,如果h=12h=12h=12,要求出满足3x≡12(mod17)3^x\equiv12\(mod\17)3
- 全面解析物联网信息安全知识体系
无声远望
本文还有配套的精品资源,点击获取简介:本资料集详细介绍物联网信息安全的多个重要方面,包括基础概念、数学基础、数据安全与隐私保护、集成安全技术、安全分析、防护策略和身份认证。从基本的物联网安全概念到深度探讨密码学基础,再到数据保护技术,再到全面的系统安全设计,安全分析,防御措施以及身份验证技术,这些内容将为研究者、开发者和管理者提供物联网安全的全面视角。1.物联网信息安全基础概念在现代技术不断发展的
- 区块链的数学基础:核心原理与应用解析
一休哥助手
区块链
引言区块链技术作为分布式账本系统,成功地解决了传统中心化系统中的信任问题。其背后隐藏着复杂而精妙的数学原理,包括密码学、哈希函数、数字签名、椭圆曲线、零知识证明等。这些数学工具不仅为区块链提供了安全保障,也为智能合约和去中心化应用(DApps)的开发奠定了基础。本文将深入剖析区块链中的核心数学基础,帮助读者理解其工作原理与实际应用。一、区块链数学基础概述区块链的数学基础可以分为以下几个核心领域:密
- 计算机网络破译密码的题目,密码习题及部分参考答案.doc
耿礼勇
计算机网络破译密码的题目
一、密码学概述部分:1、什么是密码体制的五元组。五元组(M,C,K,E,D)构成密码体制模型,M代表明文空间;C代表密文空间;K代表密钥空间;E代表加密算法;D代表解密算法2、简述口令和密码的区别。密码:按特定法则编成,用以对通信双方的信息进行明、密变换的符号。换而言之,密码是隐蔽了真实内容的符号序列。就是把用公开的、标准的信息编码表示的信息通过一种变换手段,将其变为除通信双方以外其他人所不能读懂
- 计算机密码体制分为哪两类,密码体制的分类.ppt
约会师老马
计算机密码体制分为哪两类
密码体制的分类.ppt密码学基本理论现代密码学起始于20世纪50年代,1949年Shannon的《TheCommunicationTheoryofSecretSystems》奠定了现代密码学的数学理论基础。密码体制分类(1)换位与代替密码体制序列与分组密码体制对称与非对称密钥密码体制数学理论数论信息论复杂度理论数论--数学皇后素数互素模运算,模逆元同余方程组,孙子问题,中国剩余定理因子分解素数梅森
- 《深入浅出HTTPS》读书笔记(29):TLS/SSL协议
earthzhang2021
网络协议算法开发语言httpsssl
《深入浅出HTTPS》读书笔记(29):TLS/SSL协议TLS/SSL协议是一系列算法的组合,相比密码学算法来说,TLS/SSL协议的复杂性就更大了,主要体现在以下方面。◎协议设计的复杂性:一个完整的解决方案考虑的问题非常多,需要考虑扩展性、适用性、性能等方面,一旦方案设计不充分,攻击者不用攻击特定的密码学算法,而会基于协议进行攻击。◎协议实现的严谨性:即使协议设计是完美的,在实现协议的时候,也
- MurmurHash Tips(qbit)
pythonjavahash
简介MurmurHash是一种非加密型哈希函数(Non-cryptographichashfunction),适用于一般的哈希检索操作。与其它流行的哈希函数相比,对于规律性较强的key,MurmurHash的随机分布特征表现更良好。常见的MD5、SHA1是加密型哈希函数(Cryptographichashfunction)Hash算法评价杨保华《区块链·原理、设计与应用》第5章密码学与安全技术中讲
- 高端密码学院笔记285
柚子_b4b4
高端幸福密码学院(高级班)幸福使者:李华第(598)期《幸福》之回归内在深层生命原动力基础篇——揭秘“激励”成长的喜悦心理案例分析主讲:刘莉一,知识扩充:成功=艰苦劳动+正确方法+少说空话。贪图省力的船夫,目标永远下游。智者的梦再美,也不如愚人实干的脚印。幸福早课堂2020.10.16星期五一笔记:1,重视和珍惜的前提是知道它的价值非常重要,当你珍惜了,你就真正定下来,真正的学到身上。2,大家需要
- Tor Browser配置方法
淡水猫.
网络安全服务器
密码学中有两种常见的加密方式:对称加密:加密和解密使用同一个秘钥,如AES、DES等算法。非对称加密:加密和解密使用不相同的密钥,这两个秘钥分别称为公钥(publickey)和私钥(privatekey)——也就是说私钥可以解开公钥加密的数据,反之亦然(很神奇的数学原理)。Tor是一个三重代理(也就是说Tor每发出一个请求会先经过Tor网络的3个节点),其网络中有两种主要服务器角色:中继服务器:负
- python 实现eulers totient欧拉方程算法
luthane
算法python开发语言
eulerstotient欧拉方程算法介绍欧拉函数(Euler’sTotientFunction),通常表示为(),是一个与正整数相关的函数,它表示小于或等于的正整数中与互质的数的数目。欧拉函数在数论和密码学中有广泛的应用。欧拉函数的性质1.**对于质数,有φ(p)=p−1∗∗φ(p)=p−1^{**}φ(p)=p−1∗∗。2.**如果是质数的次幂,即n=pkn=p^kn=pk,则φ(n)=pk−
- Spring的注解积累
yijiesuifeng
spring注解
用注解来向Spring容器注册Bean。
需要在applicationContext.xml中注册:
<context:component-scan base-package=”pagkage1[,pagkage2,…,pagkageN]”/>。
如:在base-package指明一个包
<context:component-sc
- 传感器
百合不是茶
android传感器
android传感器的作用主要就是来获取数据,根据得到的数据来触发某种事件
下面就以重力传感器为例;
1,在onCreate中获得传感器服务
private SensorManager sm;// 获得系统的服务
private Sensor sensor;// 创建传感器实例
@Override
protected void
- [光磁与探测]金吕玉衣的意义
comsci
这是一个古代人的秘密:现在告诉大家
信不信由你们:
穿上金律玉衣的人,如果处于灵魂出窍的状态,可以飞到宇宙中去看星星
这就是为什么古代
- 精简的反序打印某个数
沐刃青蛟
打印
以前看到一些让求反序打印某个数的程序。
比如:输入123,输出321。
记得以前是告诉你是几位数的,当时就抓耳挠腮,完全没有思路。
似乎最后是用到%和/方法解决的。
而今突然想到一个简短的方法,就可以实现任意位数的反序打印(但是如果是首位数或者尾位数为0时就没有打印出来了)
代码如下:
long num, num1=0;
- PHP:6种方法获取文件的扩展名
IT独行者
PHP扩展名
PHP:6种方法获取文件的扩展名
1、字符串查找和截取的方法
1
$extension
=
substr
(
strrchr
(
$file
,
'.'
), 1);
2、字符串查找和截取的方法二
1
$extension
=
substr
- 面试111
文强chu
面试
1事务隔离级别有那些 ,事务特性是什么(问到一次)
2 spring aop 如何管理事务的,如何实现的。动态代理如何实现,jdk怎么实现动态代理的,ioc是怎么实现的,spring是单例还是多例,有那些初始化bean的方式,各有什么区别(经常问)
3 struts默认提供了那些拦截器 (一次)
4 过滤器和拦截器的区别 (频率也挺高)
5 final,finally final
- XML的四种解析方式
小桔子
domjdomdom4jsax
在平时工作中,难免会遇到把 XML 作为数据存储格式。面对目前种类繁多的解决方案,哪个最适合我们呢?在这篇文章中,我对这四种主流方案做一个不完全评测,仅仅针对遍历 XML 这块来测试,因为遍历 XML 是工作中使用最多的(至少我认为)。 预 备 测试环境: AMD 毒龙1.4G OC 1.5G、256M DDR333、Windows2000 Server
- wordpress中常见的操作
aichenglong
中文注册wordpress移除菜单
1 wordpress中使用中文名注册解决办法
1)使用插件
2)修改wp源代码
进入到wp-include/formatting.php文件中找到
function sanitize_user( $username, $strict = false
- 小飞飞学管理-1
alafqq
管理
项目管理的下午题,其实就在提出问题(挑刺),分析问题,解决问题。
今天我随意看下10年上半年的第一题。主要就是项目经理的提拨和培养。
结合我自己经历写下心得
对于公司选拔和培养项目经理的制度有什么毛病呢?
1,公司考察,选拔项目经理,只关注技术能力,而很少或没有关注管理方面的经验,能力。
2,公司对项目经理缺乏必要的项目管理知识和技能方面的培训。
3,公司对项目经理的工作缺乏进行指
- IO输入输出部分探讨
百合不是茶
IO
//文件处理 在处理文件输入输出时要引入java.IO这个包;
/*
1,运用File类对文件目录和属性进行操作
2,理解流,理解输入输出流的概念
3,使用字节/符流对文件进行读/写操作
4,了解标准的I/O
5,了解对象序列化
*/
//1,运用File类对文件目录和属性进行操作
//在工程中线创建一个text.txt
- getElementById的用法
bijian1013
element
getElementById是通过Id来设置/返回HTML标签的属性及调用其事件与方法。用这个方法基本上可以控制页面所有标签,条件很简单,就是给每个标签分配一个ID号。
返回具有指定ID属性值的第一个对象的一个引用。
语法:
&n
- 励志经典语录
bijian1013
励志人生
经典语录1:
哈佛有一个著名的理论:人的差别在于业余时间,而一个人的命运决定于晚上8点到10点之间。每晚抽出2个小时的时间用来阅读、进修、思考或参加有意的演讲、讨论,你会发现,你的人生正在发生改变,坚持数年之后,成功会向你招手。不要每天抱着QQ/MSN/游戏/电影/肥皂剧……奋斗到12点都舍不得休息,看就看一些励志的影视或者文章,不要当作消遣;学会思考人生,学会感悟人生
- [MongoDB学习笔记三]MongoDB分片
bit1129
mongodb
MongoDB的副本集(Replica Set)一方面解决了数据的备份和数据的可靠性问题,另一方面也提升了数据的读写性能。MongoDB分片(Sharding)则解决了数据的扩容问题,MongoDB作为云计算时代的分布式数据库,大容量数据存储,高效并发的数据存取,自动容错等是MongoDB的关键指标。
本篇介绍MongoDB的切片(Sharding)
1.何时需要分片
&nbs
- 【Spark八十三】BlockManager在Spark中的使用场景
bit1129
manager
1. Broadcast变量的存储,在HttpBroadcast类中可以知道
2. RDD通过CacheManager存储RDD中的数据,CacheManager也是通过BlockManager进行存储的
3. ShuffleMapTask得到的结果数据,是通过FileShuffleBlockManager进行管理的,而FileShuffleBlockManager最终也是使用BlockMan
- yum方式部署zabbix
ronin47
yum方式部署zabbix
安装网络yum库#rpm -ivh http://repo.zabbix.com/zabbix/2.4/rhel/6/x86_64/zabbix-release-2.4-1.el6.noarch.rpm 通过yum装mysql和zabbix调用的插件还有agent代理#yum install zabbix-server-mysql zabbix-web-mysql mysql-
- Hibernate4和MySQL5.5自动创建表失败问题解决方法
byalias
J2EEHibernate4
今天初学Hibernate4,了解了使用Hibernate的过程。大体分为4个步骤:
①创建hibernate.cfg.xml文件
②创建持久化对象
③创建*.hbm.xml映射文件
④编写hibernate相应代码
在第四步中,进行了单元测试,测试预期结果是hibernate自动帮助在数据库中创建数据表,结果JUnit单元测试没有问题,在控制台打印了创建数据表的SQL语句,但在数据库中
- Netty源码学习-FrameDecoder
bylijinnan
javanetty
Netty 3.x的user guide里FrameDecoder的例子,有几个疑问:
1.文档说:FrameDecoder calls decode method with an internally maintained cumulative buffer whenever new data is received.
为什么每次有新数据到达时,都会调用decode方法?
2.Dec
- SQL行列转换方法
chicony
行列转换
create table tb(终端名称 varchar(10) , CEI分值 varchar(10) , 终端数量 int)
insert into tb values('三星' , '0-5' , 74)
insert into tb values('三星' , '10-15' , 83)
insert into tb values('苹果' , '0-5' , 93)
- 中文编码测试
ctrain
编码
循环打印转换编码
String[] codes = {
"iso-8859-1",
"utf-8",
"gbk",
"unicode"
};
for (int i = 0; i < codes.length; i++) {
for (int j
- hive 客户端查询报堆内存溢出解决方法
daizj
hive堆内存溢出
hive> select * from t_test where ds=20150323 limit 2;
OK
Exception in thread "main" java.lang.OutOfMemoryError: Java heap space
问题原因: hive堆内存默认为256M
这个问题的解决方法为:
修改/us
- 人有多大懒,才有多大闲 (评论『卓有成效的程序员』)
dcj3sjt126com
程序员
卓有成效的程序员给我的震撼很大,程序员作为特殊的群体,有的人可以这么懒, 懒到事情都交给机器去做 ,而有的人又可以那么勤奋,每天都孜孜不倦得做着重复单调的工作。
在看这本书之前,我属于勤奋的人,而看完这本书以后,我要努力变成懒惰的人。
不要在去庞大的开始菜单里面一项一项搜索自己的应用程序,也不要在自己的桌面上放置眼花缭乱的快捷图标
- Eclipse简单有用的配置
dcj3sjt126com
eclipse
1、显示行号 Window -- Prefences -- General -- Editors -- Text Editors -- show line numbers
2、代码提示字符 Window ->Perferences,并依次展开 Java -> Editor -> Content Assist,最下面一栏 auto-Activation
- 在tomcat上面安装solr4.8.0全过程
eksliang
Solrsolr4.0后的版本安装solr4.8.0安装
转载请出自出处:
http://eksliang.iteye.com/blog/2096478
首先solr是一个基于java的web的应用,所以安装solr之前必须先安装JDK和tomcat,我这里就先省略安装tomcat和jdk了
第一步:当然是下载去官网上下载最新的solr版本,下载地址
- Android APP通用型拒绝服务、漏洞分析报告
gg163
漏洞androidAPP分析
点评:记得曾经有段时间很多SRC平台被刷了大量APP本地拒绝服务漏洞,移动安全团队爱内测(ineice.com)发现了一个安卓客户端的通用型拒绝服务漏洞,来看看他们的详细分析吧。
0xr0ot和Xbalien交流所有可能导致应用拒绝服务的异常类型时,发现了一处通用的本地拒绝服务漏洞。该通用型本地拒绝服务可以造成大面积的app拒绝服务。
针对序列化对象而出现的拒绝服务主要
- HoverTree项目已经实现分层
hvt
编程.netWebC#ASP.ENT
HoverTree项目已经初步实现分层,源代码已经上传到 http://hovertree.codeplex.com请到SOURCE CODE查看。在本地用SQL Server 2008 数据库测试成功。数据库和表请参考:http://keleyi.com/a/bjae/ue6stb42.htmHoverTree是一个ASP.NET 开源项目,希望对你学习ASP.NET或者C#语言有帮助,如果你对
- Google Maps API v3: Remove Markers 移除标记
天梯梦
google maps api
Simply do the following:
I. Declare a global variable:
var markersArray = [];
II. Define a function:
function clearOverlays() {
for (var i = 0; i < markersArray.length; i++ )
- jQuery选择器总结
lq38366
jquery选择器
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40
- 基础数据结构和算法六:Quick sort
sunwinner
AlgorithmQuicksort
Quick sort is probably used more widely than any other. It is popular because it is not difficult to implement, works well for a variety of different kinds of input data, and is substantially faster t
- 如何让Flash不遮挡HTML div元素的技巧_HTML/Xhtml_网页制作
刘星宇
htmlWeb
今天在写一个flash广告代码的时候,因为flash自带的链接,容易被当成弹出广告,所以做了一个div层放到flash上面,这样链接都是a触发的不会被拦截,但发现flash一直处于div层上面,原来flash需要加个参数才可以。
让flash置于DIV层之下的方法,让flash不挡住飘浮层或下拉菜单,让Flash不档住浮动对象或层的关键参数:wmode=opaque。
方法如下:
- Mybatis实用Mapper SQL汇总示例
wdmcygah
sqlmysqlmybatis实用
Mybatis作为一个非常好用的持久层框架,相关资料真的是少得可怜,所幸的是官方文档还算详细。本博文主要列举一些个人感觉比较常用的场景及相应的Mapper SQL写法,希望能够对大家有所帮助。
不少持久层框架对动态SQL的支持不足,在SQL需要动态拼接时非常苦恼,而Mybatis很好地解决了这个问题,算是框架的一大亮点。对于常见的场景,例如:批量插入/更新/删除,模糊查询,多条件查询,联表查询,
![]()
![]()
![]() ,且gcd(a, 26)=1
,且gcd(a, 26)=1![]()
![]()