分析rgb和yuv文件的三个通道的概率分布，并计算各自的熵（编程实现）。

---

一、实验任务

分析rgb和yuv文件的三个通道的概率分布，并计算各自的熵（编程实现）。

注：
1. down.rgb和down.yuv两个文件的分辨率均为256*256。
2. yuv为4:2:0采样空间。
3. 存储格式：rgb文件按每个像素BGR分量依次存放；YUV格式按照全部像素的Y数据块、U数据块和V数据块依次存放。

---

二、实验思路

1. 分别读取R、G、B（或Y、U、V）到数组中。

• RGB文件 按每个像素BGR分量依次存放。
• YUV文件 按照全部像素的Y数据块、U数据块和V数据块依次存放。本实验为4:2:0采样空间。
  4:2:0是指水平和垂直 Y 各取样两个点，UV 各只取样一个点，水平的取样比例是 2:1，重直的取样比例 2:1，也就是色度和亮度差 1/2 * 1/2 = 1/4。

2. 分别统计R、G、B（或Y、U、V）三通道的颜色强度级的频数。
3. 别计算R、G、B（或Y、U、V）三通道的颜色强度级的概率，将概率写入文件。
4. 计算熵并输出。

---

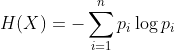
三、代码部分

1.rgb

代码如下：

#include
#include
using namespace std;

#define Res 256*256//分辨率

int main()
{
     
    unsigned char R[Res] = {
      0 }, G[Res] = {
      0 }, B[Res] = {
      0 };    //定义R、G、B分量
    double R1[256] = {
      0 }, G1[256] = {
      0 }, B1[256] = {
      0 };   //定义R、G、B概率分量
    double R2 = 0, G2 = 0, B2 = 0;    //定义R、G、B的熵

    FILE* Picture, * Red, * Green, * Blue;
    fopen_s(&Picture, "/Users/cxr/Desktop/数据压缩/chengxu/Project1/down.rgb", "rb");
    fopen_s(&Red, "/Users/cxr/Desktop/数据压缩/chengxu/Project1/Red.txt", "w");
    fopen_s(&Green, "/Users/cxr/Desktop/数据压缩/chengxu/Project1/Green.txt", "w");
    fopen_s(&Blue, "/Users/cxr/Desktop/数据压缩/chengxu/Project1/Blue.txt", "w");

    if (Picture==0)
        printf( "读取图片失败！");
    else
    {
     
        //分别读取R、G、B到数组中
        unsigned char Array[Res * 3] = {
      0 };
        fread(Array, 1, Res * 3, Picture);
        for (int i = 0, j = 0; i < Res * 3; i = i + 3, j++)
        {
     
            B[j] = *(Array + i);
            G[j] = *(Array + i + 1);
            R[j] = *(Array + i + 2);
        }

        //分别统计R、G、B三通道的256个颜色强度级的频数
        for (int i = 0; i < Res; i++)
        {
     
             R1[R[i]]++; 
             G1[G[i]]++;
             B1[B[i]]++;
        }

        //分别计算R、G、B三通道的256个颜色强度级的概率
        for (int i = 0; i < 256; i++)
        {
     
            R1[i] = R1[i] / (Res);
            B1[i] = B1[i] / (Res);
            G1[i] = G1[i] / (Res);
        }

        //将概率写入文件
        for (int i = 0; i < 256; i++)
        {
     
            fprintf(Red, "%d\t%f\n", i, R1[i]);
            fprintf(Green, "%d\t%f\n", i, G1[i]);
            fprintf(Blue, "%d\t%f\n", i, B1[i]);
        }

        //计算并输出熵
        for (int i = 0; i < 256; i++)
        {
     
            if (R1[i] != 0) {
      R2 += -R1[i] * log(R1[i]) / log(2); }
            if (G1[i] != 0) {
      G2 += -G1[i] * log(G1[i]) / log(2); }
            if (B1[i] != 0) {
      B2 += -B1[i] * log(B1[i]) / log(2); }
        }
        printf("R的熵为%f\n", R2);
        printf("G的熵为%f\n", G2); 
        printf("B的熵为%f\n", B2);
    }
    return 0;
}

运行结果如下：

2.yuv

代码如下：

#include
#include
using namespace std;

#define Res 256*256//分辨率

int main()
{
     
    unsigned char Y[Res] = {
      0 }, U[Res/4] = {
      0 }, V[Res/4] = {
      0 };    //定义Y、U、V分量
    double Y1[256] = {
      0 }, U1[256] = {
      0 }, V1[256] = {
      0 };   //定义Y、U、V概率分量
    double Y2 = 0, U2 = 0, V2 = 0;    //定义Y、U、V的熵

    FILE* Picture, * PartY, * PartU, * PartV;
    fopen_s(&Picture, "/Users/cxr/Desktop/数据压缩/chengxu/Project2/down.yuv", "rb");
    fopen_s(&PartY, "/Users/cxr/Desktop/数据压缩/chengxu/Project2/PartY.txt", "w");
    fopen_s(&PartU, "/Users/cxr/Desktop/数据压缩/chengxu/Project2/PartU.txt", "w");
    fopen_s(&PartV, "/Users/cxr/Desktop/数据压缩/chengxu/Project2/PartV.txt", "w");

    if (Picture == 0)
        printf("读取图片失败！");
    else
    {
     
        //分别读取Y、U、V到数组中
        unsigned char Array[98304];
        fread(Array, 1, Res * 1.5, Picture);
        for (int i = 0; i < Res ; i++)
        {
     
            Y[i] = *(Array + i);
        }

        for (int i = Res; i < Res * 1.25; i++)
        {
     
            U[i-65536] = *(Array + i);
        }

        for (int i = Res * 1.25; i < Res * 1.5; i++)
        {
     
            V[i - 81920] = *(Array + i);
        }

        //分别统计Y、U、V三通道的颜色强度级的频数
        for (int i = 0; i < Res; i++)
        {
     
            Y1[Y[i]]++;
        }

        for (int i = 0; i < (Res/4); i++)
        {
     
            U1[U[i]]++;
            V1[V[i]]++;
        }

        //分别计算Y、U、V三通道的256个颜色强度级的概率
        for (int i = 0; i < 256; i++)
        {
     
            Y1[i] = Y1[i] / (Res);
            U1[i] = U1[i] / (Res/4);
            V1[i] = V1[i] / (Res/4);
        }

        //将概率写入文件
        for (int i = 0; i < 256; i++)
        {
     
            fprintf(PartY, "%d\t%f\n", i, Y1[i]);
            fprintf(PartU, "%d\t%f\n", i, U1[i]);
            fprintf(PartV, "%d\t%f\n", i, V1[i]);
        }

        //计算并输出熵
        for (int i = 0; i < 256; i++)
        {
     
            if (Y1[i] != 0) {
      Y2 += -Y1[i] * log(Y1[i]) / log(2); }
            if (U1[i] != 0) {
      U2 += -U1[i] * log(U1[i]) / log(2); }
            if (V1[i] != 0) {
      V2 += -V1[i] * log(V1[i]) / log(2); }
        }
        printf("Y的熵为%f\n", Y2);
        printf("U的熵为%f\n", U2);
        printf("V的熵为%f\n", V2);
    }
    return 0;
}

运行结果如下：

---

四、结果分析

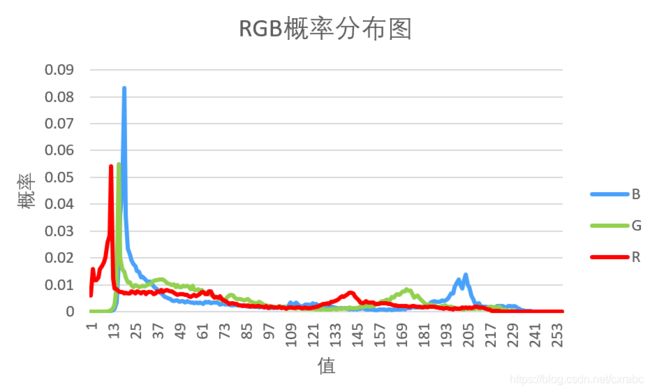
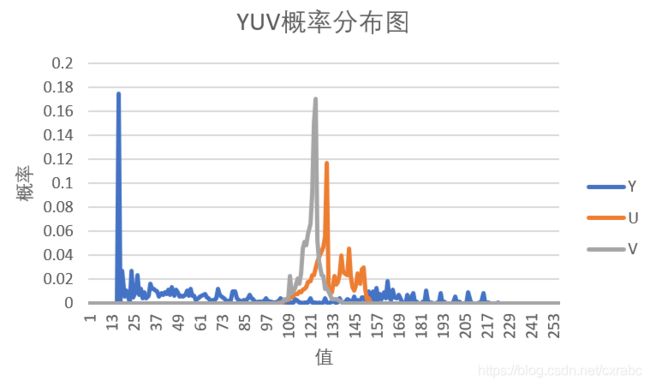
将编程得到的txt文件导入Excel表格中，绘制图像。

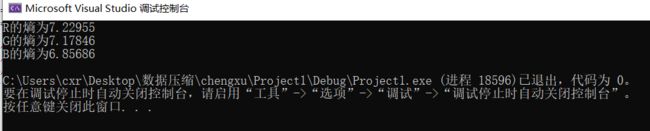
1.rgb的熵及概率分布图

分量	R	G	B
熵	7.22955	7.17846	6.85686

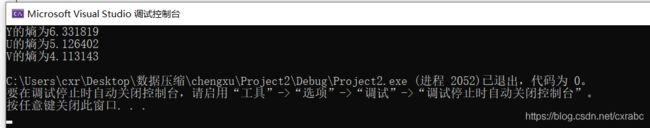
2.yuv的熵及概率分布图

分量	Y	U	V
熵	6.331819	5.126402	4.113143

3.结论

观察RGB与YUV的概率分布图，可以看出YUV分量的值更不均匀，所以它的熵更小。该结论与编程计算的熵的结果相符。