2021 美赛MCM A题记录

---

前言

由于是第一次参加美赛，缺乏相关的经验。好在和两名队友忙忙碌碌了几天，终于赶在结束前一小时把论文提交了。
最近刚好有空，整理了一下美赛的work，把思路分享给小伙伴们，代码已上传至我的github，欢迎follow和star~

https://github.com/TommyGong08/MCM2021A

---

一、中文

1.1我们的工作

我们的目的是研究多种真菌同时存在时的相互影响以及外界环境对真菌生存和分解的影响。为了做出准确合理的预测，我们建立了三个模型：
模型一：不变环境下的真菌相互作用模型
模型二：变化环境下的真菌相互作用模型
模型三：分解速率模型。

1.2 我们的模型

1.2.1 分解模型

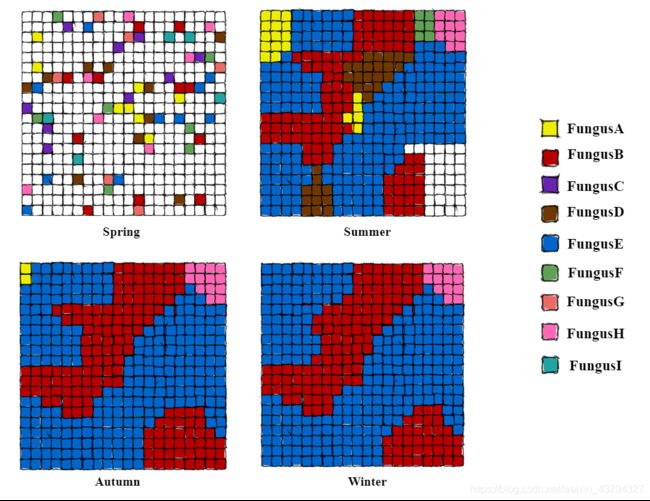
对于模型一，我们基于元胞自动机模型模拟了在环境条件适宜和短期不变的情况下，各真菌种群的变化过程。首先分析了菌株竞争能力的决定因素，建立了各菌株相对竞争能力与占据网格位置的概率映射关系，并制定了网格状态转换规则。我们设计了不同扩展率的真菌参数，并用计算机软件模拟种群变化。我们发现，在不改变和适宜的环境条件下，快速生长的真菌在短期内具有进化优势。

1.2.2 元胞自动机模型

我们的模型验证了环境对物种演替的影响真菌：环境变化可能改变优势菌株的类型，增强耐环境变化能力强的菌株的相对竞争性，削弱耐环境变化能力差的菌株的相对竞争性。此外，我们
测试模型对温度、湿度、真菌延伸率、耐湿性和耐高温性的敏感性。结果表明，该模型对真菌扩展速率、耐湿性和耐高温性的变化更为敏感，更符合实际情况。

1.2.3 模型三

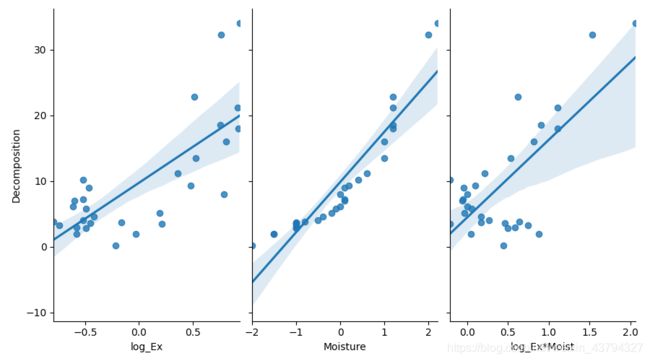
对于模型Ⅲ，为了定量描述各菌株的分解速率，我们采用混合线性回归方程来建立分解速率与延伸率和耐湿性之间的关系。结合模型Ⅱ，计算了某一系统的总分解效率，分析了真菌种类对分解效率的影响。最后，我们根据我们的三个模型预测了生物多样性的重要性和作用。

二、English

2.1 Our Work

Our purpose is to study the mutual influence of multiple fungi when they exist simultaneously and the influence of the external environment on the survival and decomposition of fungi. To make accurate and reasonable predictions, we have established three models: model I: Fungi interaction model under unchanging environment, model II: Fungi interaction model under changing environment and model III: Decomposition rate model.

2.2 Our Model

the same with 1.2

2.2.1 Test on Decomposition Model

For model I, we simulate the changing process of each fungal population based on the cellular automata model when the environmental conditions are suitable and unchanged in the short term. First, we analyze the determinants of the competitive ability of strains, establish the probability mapping relationship between the relative competitive ability of each fungus and occupying the grid position, and formulate the grid state transformation rules. We design fungal parameters with different extension rates and use computer software to simulate population changes. We find that under unchanged and suitable environmental conditions, fast-growing fungi have an advantage in evolution in the short term.

2.2.2 Cellular Automata Model

Our model verifies the influence of the environment on the succession of fungi:environmental changes may change types of dominant strains, enhance the relative competitiveness of strains with strong tolerance to environmental changes, and weaken the relative competition of strains with poor resistance to environmental changes. Additionally, we test the sensitivity of the model to temperature, humidity, fungal extension rate, moisture tolerance and high temperature resistance. It is found that the model is more sensitive to changes in fungal extension rate, humidity resistance and high temperature resistance, while it is more consistent with the real situation.

2.2.3 Model III

For model III, to quantitatively describe the decomposition rate of each strain, we apply a mixed linear regression equation to establish the relationship between the decomposition rate and the extension rate and moisture tolerance. Combining with Model II, we calculate the total decomposition efficiency in a certain system and analyze the effect of fungal species on the decomposition efficiency. Finally, we predict the importance and role of biodiversity based on our three models.