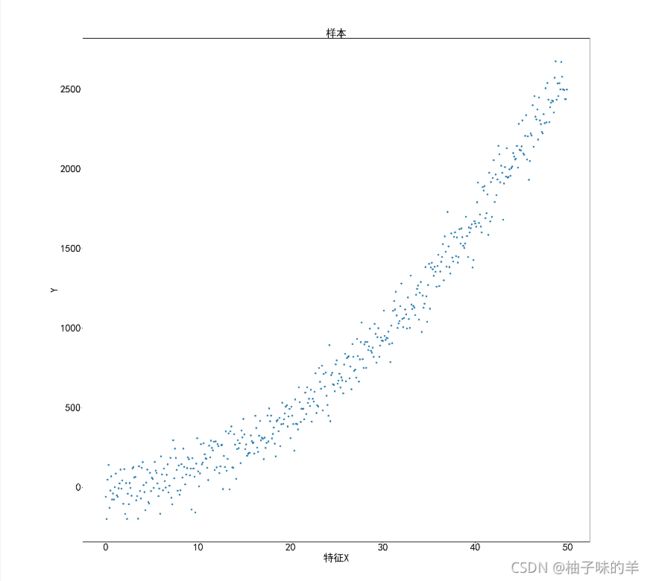
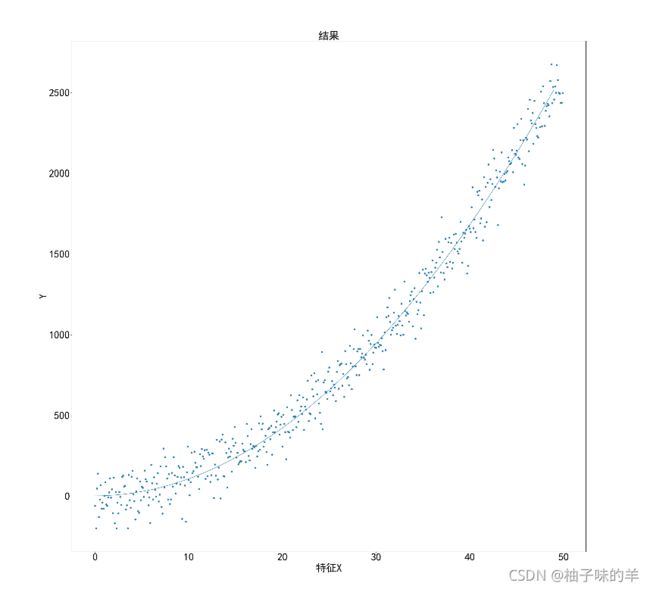
- python汇率_用Python抓取汇率
抓取的是中行的数据:网址代码#-*-coding:utf-8-*-importreimporturllib.requesturl='http://www.boc.cn/sourcedb/whpj/index.html'#网址req=urllib.request.Request(url)response=urllib.request.urlopen(req)the_page=response.rea
- python抓取汇率_09 使用Python爬取中国银行网站选择汇率最坑的一天
爬取2018年8月27日~9月2日的欧元汇率。先说结论:如果是现汇卖出价,可以选择2018-08-3109:19:26,现钞卖出价805.28。我刚问了报销过的人她说任选都行,可以不是中行折算价。最近出差,学校可以以人民币的形式报销路费、住宿费,汇率,可以任选出差期间的任何一天任何时候的中国银行的汇率,中国银行网站上的汇率长这样:如果想要合理利用规则,多回一点本,不妨选择汇率最坑的一天(默默给财务
- 爬虫小结
Crescent_P
python小项目python数据分析
python爬虫小组作业上周布置了python的小组作业,每一组要求爬取老师指定的信息,本组抽到的题目如下:从中国银行网址:http://www.boc.cn/sourcedb/whpj/获取主要外汇(美元、欧元、英镑、加拿大元、澳大利亚元、日元、韩元、新台币、澳门元和港币)的牌价信息,计算出它们的每天平均价。要求把今年5月份每天平均价格保存到Excel文件中,每种外汇的数据保存在一个工作表中,并
- Python 爬虫实战:抓取华尔街日报付费文章摘要的全方位指南
Python爬虫项目
python爬虫开发语言信息可视化数据分析
引言在全球化的信息时代,获取高质量的新闻内容对于研究、投资和决策具有重要意义。《华尔街日报》(TheWallStreetJournal,简称WSJ)作为国际知名的财经媒体,其文章内容备受关注。然而,WSJ的大部分内容属于付费订阅,普通用户无法直接访问。本文将深入探讨如何使用Python爬虫技术,结合最新的工具和方法,抓取WSJ的付费文章摘要。一、了解目标网站结构1.1WSJ网站结构分析WSJ的官方
- Python爬虫实战:使用最新技术爬取头条新闻数据
Python爬虫项目
2025年爬虫实战项目python爬虫开发语言scrapy音视频
一、前言:Python爬虫在现代数据获取中的重要性在当今信息爆炸的时代,数据已经成为最宝贵的资源之一。作为数据获取的重要手段,网络爬虫技术在各个领域发挥着越来越重要的作用。Python凭借其简洁的语法、丰富的库生态系统和强大的社区支持,已经成为网络爬虫开发的首选语言。本文将详细介绍如何使用Python及其最新的爬虫技术来爬取头条新闻数据。我们将从基础概念讲起,逐步深入到高级技巧,最后给出完整的爬虫
- Python爬虫实战:爬取ETF基金持仓变化
Python爬虫项目
python爬虫开发语言信息可视化数据分析
1.项目背景ETF(Exchange-TradedFund,交易型开放式指数基金)作为一种在交易所上市交易的基金,其持仓信息对于投资者具有重要参考价值。了解ETF的持仓变化,可以帮助投资者判断市场趋势和资金流向。本文将通过Python爬虫技术,自动化地获取ETF基金的持仓变化数据,进行存储和分析。2.技术选型与环境准备2.1技术选型编程语言:Python3.8+爬虫框架:Scrapy数据解析:Be
- 【Python】(一)面试题和Py基础题
戏精亿点点菜
python开发语言
1.技术面试题(1)TCP与UDP的区别是什么?答:TCP(TransmissionControlProtocol,传输控制协议)提供的是面向连接,可靠的字节流服务。即客户和服务器交换数据前,必须现在双方之间建立一个TCP连接,之后才能传输数据。并且提供超时重发,丢弃重复数据,检验数据,流量控制等功能,保证数据能从一端传到另一端。UDP(UserDataProtocol,用户数据报协议)是一个简单
- Python 爬虫实战:实时采集外汇汇率数据的全方位指南
Python爬虫项目
python爬虫开发语言信息可视化数据分析
引言在全球化的金融市场中,外汇汇率的实时数据对于投资者、企业和研究人员来说至关重要。通过自动化的方式获取这些数据,不仅可以提高效率,还能为决策提供及时的支持。本文将深入探讨如何使用Python爬虫技术,结合最新的工具和方法,实时采集外汇汇率数据。一、外汇汇率数据的获取途径1.1使用官方API接口许多金融机构和数据提供商提供了官方的API接口,供开发者获取外汇汇率数据。例如:AlphaVantage
- 从零构建智能ai语音助手:ESP32s3+Python+大语言模型实战指南
从零构建智能ai语音助手:ESP32s3+Python+大语言模型实战指南一、项目概述大家好!今天给大家带来一个干货满满的实战项目——基于ESP32S3硬件和Python后端的智能语音助手系统。这个项目将物联网技术与AI技术完美结合,打造一个可以实时对话、意图识别的智能语音交互系统。相比传统的离线语音系统只能识别固定命令词,我们这套系统可以:实现自然语言理解,支持多种表达方式无需预设固定命令词,更
- Python 领域 pytest 的测试用例的可维护性设计
Python领域pytest的测试用例的可维护性设计关键词:pytest、测试用例、可维护性、测试框架、自动化测试、测试设计模式、重构摘要:本文深入探讨了如何在Python测试框架pytest中设计可维护的测试用例。我们将从测试用例可维护性的核心原则出发,分析pytest的特性和最佳实践,介绍多种提高测试代码可维护性的设计模式和技巧。文章包含实际代码示例、项目实战案例以及可维护性评估指标,帮助开发
- Python爬虫小白入门指南,成为大牛必须经历的三个阶段
学习任何一门技术,都应该带着目标去学习,目标就像一座灯塔,指引你前进,很多人学着学着就学放弃了,很大部分原因是没有明确目标,所以,一定要明确学习目的,在你准备学爬虫前,先问问自己为什么要学习爬虫。有些人是为了一份工作,有些人是为了好玩,也有些人是为了实现某个黑科技功能。不过可以肯定的是,学会了爬虫能给你的工作提供很多便利。小白入门必读作为零基础小白,大体上可分为三个阶段去实现。第一阶段是入门,掌握
- python 包管理工具uv
uv--versionuvpythonfinduvpythonlistexportUV_DEFAULT_INDEX="https://mirrors.tuna.tsinghua.edu.cn/pypi/web/simple"#换成私有的repoexportUV_HTTP_TIMEOUT=120uvpythoninstall3.12uvvenvmyenv--python3.12--seeduvhtt
- Python通关秘籍之基础教程(一)
Smile丶Life丶
Python通关指南:从零基础到高手之路python开发语言后端
引言在编程的世界里,Python就像一位温和而强大的导师,它以简洁优雅的语法和强大的功能吸引着无数初学者和专业人士。无论你是想开发网站、分析数据、构建人工智能,还是仅仅想学习编程思维,Python都是你的理想选择。Python的魅力在于它的易读性和广泛的应用场景。它的代码就像英语句子一样自然,即使是完全没有编程经验的人也能快速上手。同时,Python拥有庞大的生态系统,从Web开发(Django、
- Python 包管理工具(uv)
cliffordl
pythonpythonuv开发语言
Python虚拟环境(conda)Python虚拟环境(venv)Python包管理工具(uv)文章目录1.uv的特点2.安装uv2.1.使用官方推荐方式2.2.使用pip安装(Python>=3.8)2.3.使用conda/mamba安装3.基本使用方法3.1.初始化项目并创建虚拟环境3.1.1.CMD运行结果3.1.2.VScode运行结果3.2.安装依赖3.3.生成依赖文件3.4.使用pyp
- Python协程从入门到精通:9个案例解析yield、gevent与asyncio实战
python_chai
Pythonpython开发语言协程并发yield生成器gerrnletgevent
引言痛点分析:传统多线程在高并发场景下的性能瓶颈。协程优势:轻量级、高并发、低资源消耗。本文目标:通过9个代码案例,系统讲解协程的核心技术和应用场景。目录引言1.协程基础:理解yield生成器1.1yield的暂停与恢复机制1.2生产者-消费者模型实战1.3双向通信:send()方法详解2.手动协程控制:greenlet进阶2.1greenlet的显式切换原理2.2多任务协作案例3.自动化协程:g
- Python爬虫在社交平台数据挖掘中的应用:深入探索用户互动
程序员威哥
python爬虫数据挖掘
引言社交媒体已经成为全球用户互动的主要平台,每天都有大量的信息生成,用户之间的互动行为如点赞、评论、分享、转发等构成了宝贵的数据资源。如何利用这些互动数据为商业决策、用户行为分析以及产品优化提供支持,已经成为数据科学与大数据分析领域的一个重要课题。Python作为一款强大的编程语言,凭借其丰富的爬虫库和数据分析工具,已经成为挖掘社交平台数据的重要工具。在本文中,我们将通过Python爬虫技术,深入
- Python 爬虫实战:精准抓取母婴电商平台数据,深入分析用户评价洞察市场趋势
程序员威哥
最新爬虫实战项目python爬虫开发语言
前言随着生活水平的提高,越来越多的年轻父母开始关注母婴产品的质量和品牌。而母婴电商平台成为了他们选择和购买产品的主要渠道之一。母婴产品市场也因此变得异常活跃且充满竞争。在这样的市场环境下,用户评价不仅反映了产品的实际质量,也揭示了消费者的需求和偏好,成为品牌决策的核心依据之一。Python爬虫是获取电商平台用户评价数据、产品详情、价格等关键信息的强大工具。通过抓取和分析这些数据,品牌商可以实时了解
- *Python爬虫应用:从社交媒体数据中提取有价值的用户行为洞察
程序员威哥
python爬虫媒体
引言在现代数字化时代,社交媒体已成为获取用户行为数据的重要来源。每秒钟,数百万条信息在平台上传播,用户的互动行为——点赞、评论、分享、关注等,构成了大量宝贵的行为数据。企业和个人通过分析这些数据,不仅可以理解用户需求、改进产品,还能精准制定营销策略。然而,如何高效地抓取、分析并从中提取有价值的用户行为洞察?这正是Python爬虫和数据分析技术的优势所在。本文将介绍如何利用Python爬虫从社交媒体
- Python异步编程终极指南:用协程与事件循环重构你的高并发系统
title:Python异步编程终极指南:用协程与事件循环重构你的高并发系统date:2025/2/24updated:2025/2/24author:cmdragonexcerpt:深入剖析Python异步编程的核心机制。你将掌握:\n事件循环的底层实现原理与调度算法\nasync/await协程的6种高级用法模式\n异步HTTP请求的性能优化技巧(速度提升15倍+)\n常见异步陷阱的26种解决
- python 异步编程:协程与 asyncio
花_城
Python开发语言后端异步协程
文章目录一、协程(coroutine)1.1协程的概念1.2实现协程的方式二、asyncio异步编程2.1事件循环2.2快速上手2.3运行协程2.4await关键字2.5可等待对象2.5.1协程2.5.2任务(Task)2.5.3asyncio.Future三、concurrent.futures.Future(补充)3.1爬虫案例(asyncio+不支持异步的模块)四、asyncio异步迭代器五
- 突破性能瓶颈,几个高性能Python网络框架,高效实现网络应用
引言随着互联网和大数据时代的到来,高性能网络应用的需求日益增加。Python作为一种流行的编程语言,在高性能网络编程领域也具有广泛的应用。本文将深入探讨基于Python的几种高性能网络框架,分析它们各自的优势和适用场景,帮助开发者选择最适合自己需求的网络框架这里插播一条粉丝福利,如果你正在学习Python或者有计划学习Python,想要突破自我,对未来十分迷茫的,可以点击这里获取最新的Python
- Python面试题:Python中的异步编程:详细讲解asyncio库的使用
超哥同学
Python系列python开发语言面试编程
Python的异步编程是实现高效并发处理的一种方法,它使得程序能够在等待I/O操作时继续执行其他任务。在Python中,asyncio库是实现异步编程的主要工具。asyncio提供了一种机制来编写可以在单线程内并发执行的代码,适用于I/O密集型任务。以下是对asyncio库的详细讲解,包括基本概念、用法、示例以及注意事项。1.基本概念1.1协程(Coroutines)协程是一个特殊的函数,它可以被
- Python 爬虫实战:如何搭建高效的分布式爬虫架构,突破数据抓取极限
程序员威哥
python爬虫分布式
随着互联网数据量的飞速增长,单一爬虫在抓取大量数据时的效率和稳定性往往无法满足需求。在这种情况下,分布式爬虫架构应运而生。分布式爬虫通过多节点并行工作,可以大大提高数据抓取的速度,同时减少单点故障的风险。本文将深入探讨如何使用Python构建一个高效的分布式爬虫架构,从架构设计到技术实现,帮助你突破数据抓取的极限。一、什么是分布式爬虫?分布式爬虫系统将爬虫任务拆分为多个子任务,分布到不同的服务器或
- python程序基本架构_Python 程序基本架构
尤尔小喵喵
python程序基本架构
Python的一般程序基本架构为:输入,处理,输出,这三块。输入:包括两个内容,变量赋值与输入语句处理:包括算术运算,逻辑运算,算法处理这三方面输出:包括打印输出,写入文件,写入数据库这三块下面举两个例子具体了解一下Python的程序基本架构1输入:变量赋值处理:算术运算输出:打印输出x=12#变量赋值x=12y=13#变量赋值y=13z=x+y#算术运算print(z)#打印输出252输入:输入
- 如何让AI真正理解你的意图(自适应Prompt实战指南)
nine是个工程师
大语言模型人工智能prompt
目前的LLM模型,在理解用户意图方面,正在使用自适应Prompt技术,来提升模型的理解能力。目前使用deepseek推理模型能明显看到自适应的一个过程。前言:为什么你的AI总是"答非所问"?相信很多人都遇到过这样的情况:你问:“帮我写一个Python爬虫”AI答:给你一堆理论知识和完整教程(你只想要简单代码)你问:“推荐一部电影”AI答:推荐了《教父》(你想看轻松喜剧)你问:“解释一下机器学习”A
- 如何创建Python工程目录
九月恒心
Pythonpython自动测试
如何创建一个简单但是比较规范的python工程目录,本文是学习了LearnPythontheHardWay相关内容后做的一些笔记。安装python第三方包1.pipfromhttp://pypi.python.org/pypi/pip用于安装python第三方包的工具2.distributefromhttp://pypi.python.org/pypi/distribute已被弃用,是SetupT
- 从单体脚本到模块化设计:Python工程师的架构思维跃迁
引言:从“一团乱麻”到“乐高积木”你是否曾经打开一个Python脚本,里面密密麻麻挤着上千行代码?函数相互缠绕,全局变量随处可见,想改一个小功能却心惊胆战,生怕牵一发而动全身?这就是典型的“单体脚本”(MonolithicScript)困境。作为过来人,我深知这种痛苦。本文将手把手带你跳出这个泥潭,掌握模块化设计的核心思想,并初步建立宝贵的架构设计思维,让你的代码从“勉强运行”跃迁到“优雅可维护”
- python json 反序列化-V1
CATTLECODE
pythonjson开发语言
在编程中,反序列化函数用于将序列化后的数据(如JSON、XML等格式)重新转换为程序可操作的对象或数据结构。以下是不同语言和场景下的实现方式及特点:1.Python中的反序列化(1)标准库json模块json.loads():将JSON字符串反序列化为Python对象(如字典、列表)。importjsonjson_str='{"name":"Alice","age":25}'dat
- AI人工智能与机器学习的大数据融合应用
AI智能探索者
人工智能机器学习大数据ai
AI人工智能与机器学习的大数据融合应用关键词:AI人工智能、机器学习、大数据、融合应用、数据挖掘摘要:本文深入探讨了AI人工智能与机器学习在大数据融合应用方面的相关内容。首先介绍了研究的背景、目的、预期读者和文档结构,对核心术语进行了清晰定义。接着阐述了AI、机器学习和大数据的核心概念及相互联系,给出了形象的文本示意图和Mermaid流程图。详细讲解了核心算法原理,并通过Python源代码进行说明
- day49-ansible初体验
朱包林
linuxpython运维服务器云计算
1.选型工具说明缺点xshell不适应机器过多场景,需要连接后才能用for+ssh/scp+密钥认证密钥认证,免密码登录scp传输文本/脚本ssh远程执行命令或脚本串行saltstack需要安装客户端ansible无客户端(密钥认证)批量部署环境需要新python版本,被红帽收购了Terraform关注基础设施(云环境),一键创建100台云服务器,一键创建负载均衡,数据库产品2.ansible架构
- eclipse maven
IXHONG
eclipse
eclipse中使用maven插件的时候,运行run as maven build的时候报错
-Dmaven.multiModuleProjectDirectory system propery is not set. Check $M2_HOME environment variable and mvn script match.
可以设一个环境变量M2_HOME指
- timer cancel方法的一个小实例
alleni123
多线程timer
package com.lj.timer;
import java.util.Date;
import java.util.Timer;
import java.util.TimerTask;
public class MyTimer extends TimerTask
{
private int a;
private Timer timer;
pub
- MySQL数据库在Linux下的安装
ducklsl
mysql
1.建好一个专门放置MySQL的目录
/mysql/db数据库目录
/mysql/data数据库数据文件目录
2.配置用户,添加专门的MySQL管理用户
>groupadd mysql ----添加用户组
>useradd -g mysql mysql ----在mysql用户组中添加一个mysql用户
3.配置,生成并安装MySQL
>cmake -D
- spring------>>cvc-elt.1: Cannot find the declaration of element
Array_06
springbean
将--------
<?xml version="1.0" encoding="UTF-8"?>
<beans xmlns="http://www.springframework.org/schema/beans"
xmlns:xsi="http://www.w3
- maven发布第三方jar的一些问题
cugfy
maven
maven中发布 第三方jar到nexus仓库使用的是 deploy:deploy-file命令
有许多参数,具体可查看
http://maven.apache.org/plugins/maven-deploy-plugin/deploy-file-mojo.html
以下是一个例子:
mvn deploy:deploy-file -DgroupId=xpp3
- MYSQL下载及安装
357029540
mysql
好久没有去安装过MYSQL,今天自己在安装完MYSQL过后用navicat for mysql去厕测试链接的时候出现了10061的问题,因为的的MYSQL是最新版本为5.6.24,所以下载的文件夹里没有my.ini文件,所以在网上找了很多方法还是没有找到怎么解决问题,最后看到了一篇百度经验里有这个的介绍,按照其步骤也完成了安装,在这里给大家分享下这个链接的地址
- ios TableView cell的布局
张亚雄
tableview
cell.imageView.image = [UIImage imageNamed:[imageArray objectAtIndex:[indexPath row]]];
CGSize itemSize = CGSizeMake(60, 50);
&nbs
- Java编码转义
adminjun
java编码转义
import java.io.UnsupportedEncodingException;
/**
* 转换字符串的编码
*/
public class ChangeCharset {
/** 7位ASCII字符,也叫作ISO646-US、Unicode字符集的基本拉丁块 */
public static final Strin
- Tomcat 配置和spring
aijuans
spring
简介
Tomcat启动时,先找系统变量CATALINA_BASE,如果没有,则找CATALINA_HOME。然后找这个变量所指的目录下的conf文件夹,从中读取配置文件。最重要的配置文件:server.xml 。要配置tomcat,基本上了解server.xml,context.xml和web.xml。
Server.xml -- tomcat主
- Java打印当前目录下的所有子目录和文件
ayaoxinchao
递归File
其实这个没啥技术含量,大湿们不要操笑哦,只是做一个简单的记录,简单用了一下递归算法。
import java.io.File;
/**
* @author Perlin
* @date 2014-6-30
*/
public class PrintDirectory {
public static void printDirectory(File f
- linux安装mysql出现libs报冲突解决
BigBird2012
linux
linux安装mysql出现libs报冲突解决
安装mysql出现
file /usr/share/mysql/ukrainian/errmsg.sys from install of MySQL-server-5.5.33-1.linux2.6.i386 conflicts with file from package mysql-libs-5.1.61-4.el6.i686
- jedis连接池使用实例
bijian1013
redisjedis连接池jedis
实例代码:
package com.bijian.study;
import java.util.ArrayList;
import java.util.List;
import redis.clients.jedis.Jedis;
import redis.clients.jedis.JedisPool;
import redis.clients.jedis.JedisPoo
- 关于朋友
bingyingao
朋友兴趣爱好维持
成为朋友的必要条件:
志相同,道不合,可以成为朋友。譬如马云、周星驰一个是商人,一个是影星,可谓道不同,但都很有梦想,都要在各自领域里做到最好,当他们遇到一起,互相欣赏,可以畅谈两个小时。
志不同,道相合,也可以成为朋友。譬如有时候看到两个一个成绩很好每次考试争做第一,一个成绩很差的同学是好朋友。他们志向不相同,但他
- 【Spark七十九】Spark RDD API一
bit1129
spark
aggregate
package spark.examples.rddapi
import org.apache.spark.{SparkConf, SparkContext}
//测试RDD的aggregate方法
object AggregateTest {
def main(args: Array[String]) {
val conf = new Spar
- ktap 0.1 released
bookjovi
kerneltracing
Dear,
I'm pleased to announce that ktap release v0.1, this is the first official
release of ktap project, it is expected that this release is not fully
functional or very stable and we welcome bu
- 能保存Properties文件注释的Properties工具类
BrokenDreams
properties
今天遇到一个小需求:由于java.util.Properties读取属性文件时会忽略注释,当写回去的时候,注释都没了。恰好一个项目中的配置文件会在部署后被某个Java程序修改一下,但修改了之后注释全没了,可能会给以后的参数调整带来困难。所以要解决这个问题。
&nb
- 读《研磨设计模式》-代码笔记-外观模式-Facade
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
/*
* 百度百科的定义:
* Facade(外观)模式为子系统中的各类(或结构与方法)提供一个简明一致的界面,
* 隐藏子系统的复杂性,使子系统更加容易使用。他是为子系统中的一组接口所提供的一个一致的界面
*
* 可简单地
- After Effects教程收集
cherishLC
After Effects
1、中文入门
http://study.163.com/course/courseMain.htm?courseId=730009
2、videocopilot英文入门教程(中文字幕)
http://www.youku.com/playlist_show/id_17893193.html
英文原址:
http://www.videocopilot.net/basic/
素
- Linux Apache 安装过程
crabdave
apache
Linux Apache 安装过程
下载新版本:
apr-1.4.2.tar.gz(下载网站:http://apr.apache.org/download.cgi)
apr-util-1.3.9.tar.gz(下载网站:http://apr.apache.org/download.cgi)
httpd-2.2.15.tar.gz(下载网站:http://httpd.apac
- Shell学习 之 变量赋值和引用
daizj
shell变量引用赋值
本文转自:http://www.cnblogs.com/papam/articles/1548679.html
Shell编程中,使用变量无需事先声明,同时变量名的命名须遵循如下规则:
首个字符必须为字母(a-z,A-Z)
中间不能有空格,可以使用下划线(_)
不能使用标点符号
不能使用bash里的关键字(可用help命令查看保留关键字)
需要给变量赋值时,可以这么写:
- Java SE 第一讲(Java SE入门、JDK的下载与安装、第一个Java程序、Java程序的编译与执行)
dcj3sjt126com
javajdk
Java SE 第一讲:
Java SE:Java Standard Edition
Java ME: Java Mobile Edition
Java EE:Java Enterprise Edition
Java是由Sun公司推出的(今年初被Oracle公司收购)。
收购价格:74亿美金
J2SE、J2ME、J2EE
JDK:Java Development
- YII给用户登录加上验证码
dcj3sjt126com
yii
1、在SiteController中添加如下代码:
/**
* Declares class-based actions.
*/
public function actions() {
return array(
// captcha action renders the CAPTCHA image displ
- Lucene使用说明
dyy_gusi
Lucenesearch分词器
Lucene使用说明
1、lucene简介
1.1、什么是lucene
Lucene是一个全文搜索框架,而不是应用产品。因此它并不像baidu或者googleDesktop那种拿来就能用,它只是提供了一种工具让你能实现这些产品和功能。
1.2、lucene能做什么
要回答这个问题,先要了解lucene的本质。实际
- 学习编程并不难,做到以下几点即可!
gcq511120594
数据结构编程算法
不论你是想自己设计游戏,还是开发iPhone或安卓手机上的应用,还是仅仅为了娱乐,学习编程语言都是一条必经之路。编程语言种类繁多,用途各 异,然而一旦掌握其中之一,其他的也就迎刃而解。作为初学者,你可能要先从Java或HTML开始学,一旦掌握了一门编程语言,你就发挥无穷的想象,开发 各种神奇的软件啦。
1、确定目标
学习编程语言既充满乐趣,又充满挑战。有些花费多年时间学习一门编程语言的大学生到
- Java面试十问之三:Java与C++内存回收机制的差别
HNUlanwei
javaC++finalize()堆栈内存回收
大家知道, Java 除了那 8 种基本类型以外,其他都是对象类型(又称为引用类型)的数据。 JVM 会把程序创建的对象存放在堆空间中,那什么又是堆空间呢?其实,堆( Heap)是一个运行时的数据存储区,从它可以分配大小各异的空间。一般,运行时的数据存储区有堆( Heap)和堆栈( Stack),所以要先看它们里面可以分配哪些类型的对象实体,然后才知道如何均衡使用这两种存储区。一般来说,栈中存放的
- 第二章 Nginx+Lua开发入门
jinnianshilongnian
nginxlua
Nginx入门
本文目的是学习Nginx+Lua开发,对于Nginx基本知识可以参考如下文章:
nginx启动、关闭、重启
http://www.cnblogs.com/derekchen/archive/2011/02/17/1957209.html
agentzh 的 Nginx 教程
http://openresty.org/download/agentzh-nginx-tutor
- MongoDB windows安装 基本命令
liyonghui160com
windows安装
安装目录:
D:\MongoDB\
新建目录
D:\MongoDB\data\db
4.启动进城:
cd D:\MongoDB\bin
mongod -dbpath D:\MongoDB\data\db
&n
- Linux下通过源码编译安装程序
pda158
linux
一、程序的组成部分 Linux下程序大都是由以下几部分组成: 二进制文件:也就是可以运行的程序文件 库文件:就是通常我们见到的lib目录下的文件 配置文件:这个不必多说,都知道 帮助文档:通常是我们在linux下用man命令查看的命令的文档
二、linux下程序的存放目录 linux程序的存放目录大致有三个地方: /etc, /b
- WEB开发编程的职业生涯4个阶段
shw3588
编程Web工作生活
觉得自己什么都会
2007年从学校毕业,凭借自己原创的ASP毕业设计,以为自己很厉害似的,信心满满去东莞找工作,找面试成功率确实很高,只是工资不高,但依旧无法磨灭那过分的自信,那时候什么考勤系统、什么OA系统、什么ERP,什么都觉得有信心,这样的生涯大概持续了约一年。
根本不是自己想的那样
2008年开始接触很多工作相关的东西,发现太多东西自己根本不会,都需要去学,不管是asp还是js,
- 遭遇jsonp同域下变作post请求的坑
vb2005xu
jsonp同域post
今天迁移一个站点时遇到一个坑爹问题,同一个jsonp接口在跨域时都能调用成功,但是在同域下调用虽然成功,但是数据却有问题. 此处贴出我的后端代码片段
$mi_id = htmlspecialchars(trim($_GET['mi_id ']));
$mi_cv = htmlspecialchars(trim($_GET['mi_cv ']));
贴出我前端代码片段:
$.aj