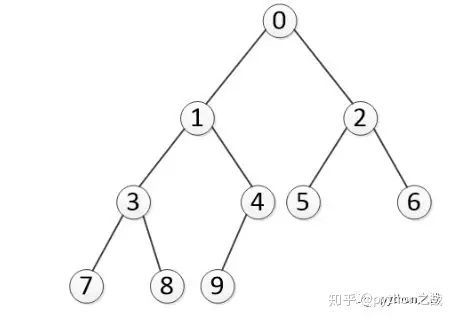
二叉树的遍历比较有意思,首先是遍历的方式比较多,大的来说分为深度遍历和广度遍历,深度遍历又分为先序遍历/中序遍历/后序遍历,其中深度遍历用递归来实现,广度遍历用队列来实现。
深度遍历和广度遍历是相对的概念,深度遍历是沿着树的深度遍历树的节点,尽可能深的搜索树的分支;广度遍历是从树的根层级开始一层一层的遍历,遍历完上一层再遍历下一层;如下:
深度遍历顺序:0-1-3-7-8-4-9-2-5-6(先序遍历)
广度遍历顺序:0-1-2-3-4-5-6-7-8-9
但对于深度遍历而言还有三种方式:先序遍历/中序遍历/后序遍历;先序遍历的顺序为:根节点->左子树->右子树;中序遍历为:左子树->根节点->右子树;当然后序遍历是:左子树->右子树->根节点;其中的序指的是根节点相对于左右节点的遍历位置。
在上二叉树中我们按照深度遍历的三种方式得到的顺序如下:
先序遍历:0-1-3-7-8-4-9-2-5-6
中序遍历:7-3-8-1-9-4-0-5-2-6
后序遍历:7-8-3-9-4-1-5-6-2-0
注意:先序遍历是从上往下看,中序遍历和后续遍历是从下往上看,从哪里开始就决定了什么相对简单二叉树的权重。
深度遍历的实现:
class Node:
"""节点类"""
def __init__(self, elem, lchild=None, rchild=None):
self.elem = elem
self.lchild = lchild
self.rchild = rchild
class Tree:
"""树类"""
def __init__(self, root=None):
self._root = root
def add(self, item):
node = Node(item)
if not self._root:
self._root = node
return
queue = [self._root]
while queue:
cur = queue.pop(0)
if not cur.lchild:
cur.lchild = node
return
elif not cur.rchild:
cur.rchild = node
return
else:
queue.append(cur.rchild)
queue.append(cur.lchild)
def preorder(self, root):
"""
先序遍历-递归实现
:param root:
:return:
"""
if not root:
raise ValueError("ROOT ERROR")
print(root.elem)
self.preorder(root.lchild)
self.preorder(root.rchild)
def inorder(self, root):
"""
中序遍历-递归实现
:param root:
:return:
"""
if not root:
raise ValueError("ROOT ERROR")
self.inorder(root.lchild)
print(root.elem)
self.inorder(root.rchild)
def postorder(self, root):
"""
后序遍历-递归实现
:param root:
:return:
"""
if not root:
raise ValueError("ROOT ERROR")
self.postorder(root.lchild)
self.postorder(root.rchild)
print(root.elem)
广度遍历的实现;
class Node:
"""节点类"""
def __init__(self, elem, lchild=None, rchild=None):
self.elem = elem
self.lchild = lchild
self.rchild = rchild
class Tree:
"""树类"""
def __init__(self, root=None):
self._root = root
def breadth_travel(self, root):
"""
广度优先-队列实现
:param root:
:return:
"""
if not root:
raise ValueError("ROOT ERROR")
queue = [root]
while queue:
node = queue.pop(0)
print(node.elem)
if node.lchild:
queue.append(node.lchild)
elif node.rchild:
queue.append(node.rchild)
递归函数使得二叉树的遍历操作更加的简洁,上面的深度遍历的三种方式除了递归以外,还可以使用堆栈的结构来实现,如果感兴趣可自行实现。