OpenFOAM的圆柱绕流算例记录
圆柱绕流是流体力学里最经典的算例之一,受到众多学者的“喜爱”。初学CFD的人除了空腔、管道流之外,最先接触的莫过于圆柱绕流了。圆柱绕流能反映流体流过障碍物时的各种情况:层流向湍流的转捩、流体的剪切与分离、边界层、卡门涡街现象等等,是深入理解流动机理的重要算例。因为经常要算圆柱绕流,在这里做一些记录,记录一些细节,以防以后忘掉。这次做Re=200时的圆柱绕流算例,画网格用ICEM,CFD软件用开源软件OpenFOAM,后处理用tecplot。算例文件可以看着我下面的内容进行设置,也可以在我的主页找找上传的文件包。
1. 前处理:画网格
用ICEM画网格。圆柱直径D=1m,计算域为圆形直径为30D。因为是层流的网格,第一层网格高度没有严格限制,网格数为160x160。我在b站上传了画网格的视频过程,可以搜chansFoam关注一下。具体过程不在这里详说。
2. OpenFOAM计算
2.1 载入网格及修改边界类型
在OpenFOAM里随便找一个模板算例。首先把刚刚画好的网格msh文件复制到case文件夹中:
在终端输入:
fluentMeshToFoam cylinder2d.msh -2D 1这样就可以把fluent的网格格式转成OpenFOAM能用的。后面-2D的指令是设置z方向厚度的,因为是2维的网格,但是在OpenFOAM里只有三位的算例,所以得z方向拉伸一层。这一层高度我设为1。接下来打开constant/polyMesh/boundary,修改一下边界条件类型,将:
改成:
这么做的原因是,在ICEM中画完网格设置边界条件时,先把所有的边界设置为wall会比较方便,只需要在OpenFOAM里统一修改即可。
2.2 设置初值和边界条件
打开0文件夹,把U和p文件设置成如下所示:
U:
/*--------------------------------*- C++ -*----------------------------------*\
| ========= | |
| \\ / F ield | OpenFOAM: The Open Source CFD Toolbox |
| \\ / O peration | Version: 4.x |
| \\ / A nd | Web: www.OpenFOAM.org |
| \\/ M anipulation | |
\*---------------------------------------------------------------------------*/
FoamFile
{
version 2.0;
format ascii;
class volVectorField;
object U;
}
// * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * //
dimensions [0 1 -1 0 0 0 0];
internalField uniform (17 0 0);
boundaryField
{
IN
{
type fixedValue;
value uniform (17 0 0);
}
OUT
{
type zeroGradient;
}
CYLINDER
{
type fixedValue;
value uniform (0 0 0);
}
frontAndBackPlanes
{
type empty;
}
}
// ************************************************************************* //p:
/*--------------------------------*- C++ -*----------------------------------*\
| ========= | |
| \\ / F ield | OpenFOAM: The Open Source CFD Toolbox |
| \\ / O peration | Version: 4.x |
| \\ / A nd | Web: www.OpenFOAM.org |
| \\/ M anipulation | |
\*---------------------------------------------------------------------------*/
FoamFile
{
version 2.0;
format ascii;
class volScalarField;
object p;
}
// * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * //
dimensions [0 2 -2 0 0 0 0];
internalField uniform 0;
boundaryField
{
IN
{
type zeroGradient;
}
OUT
{
type fixedValue;
value uniform 0;
}
CYLINDER
{
type zeroGradient;
}
frontAndBackPlanes
{
type empty;
}
}
// ************************************************************************* //这都是十分标准的边界条件了。初值的话,压强设置为0,速度为17m/s(Ma=0.05)。
2.3 运动粘度
打开constant/transportProperties,修改nu的数值。Re为200时,nu=17*1/200=0.085。
2.4 数值格式
打开system/fvSchemes,修改如下:
/*--------------------------------*- C++ -*----------------------------------*\
| ========= | |
| \\ / F ield | OpenFOAM: The Open Source CFD Toolbox |
| \\ / O peration | Version: 4.x |
| \\ / A nd | Web: www.OpenFOAM.org |
| \\/ M anipulation | |
\*---------------------------------------------------------------------------*/
FoamFile
{
version 2.0;
format ascii;
class dictionary;
object fvSchemes;
}
// * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * //
ddtSchemes
{
default backward;
}
gradSchemes
{
default Gauss linear;
}
divSchemes
{
default none;
div(phi,U) Gauss linearUpwind grad(U);
}
laplacianSchemes
{
default Gauss linear limited 1;
}
interpolationSchemes
{
default linear corrected;
}
snGradSchemes
{
default corrected;
}
// ************************************************************************* //值得一提的是对流项格式。这里用的是二阶迎风格式。如果用一阶迎风(Gauss upwind),则不会出现卡门涡街,而是收敛到下面的情况,左为x方向速度云图,右为压强云图。阻力系数会趋于稳定,升力系数以10e-6的幅值在0上下浮动(相当于不变)。
2.5 解方程的的设置
在icoFoam算法(SIMPLE)的框架下,设置完数值格式之后,程序就会构造一个非常巨大的稀疏矩阵。接下来的工作就是把这个线性方程组解开:
![]()
其中,x代表流场变量,A为系数矩阵,是一个十分稀疏的矩阵,b代表源场、显式项等。OpenFOAM内置了许多解方程的工具,都在system/fvSolution里进行设置:
/*--------------------------------*- C++ -*----------------------------------*\
| ========= | |
| \\ / F ield | OpenFOAM: The Open Source CFD Toolbox |
| \\ / O peration | Version: 4.x |
| \\ / A nd | Web: www.OpenFOAM.org |
| \\/ M anipulation | |
\*---------------------------------------------------------------------------*/
FoamFile
{
version 2.0;
format ascii;
class dictionary;
object fvSolution;
}
// * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * //
solvers
{
p
{
solver PCG;
preconditioner DIC;
tolerance 1e-07;
relTol 0;
}
pFinal
{
$p;
relTol 0;
}
U
{
solver PBiCG;
preconditioner DILU;
tolerance 1e-08;
relTol 0;
}
}
PISO
{
nCorrectors 2;
nNonOrthogonalCorrectors 2;
pRefCell 0;
pRefValue 0;
}
// ************************************************************************* //习惯上,压强矩阵我用PCG比较多,另外也可以选GAMG。速度矩阵用PBiCG。这些是什么,涉及到算法的问题,可以看一些书,这里不详细说。注意到后面的nCorrectors和nNonOrthogonalCorrectors,分别代表PISO算法校正的次数和非正交修正的次数。刚刚画的圆柱绕流网格严格来说是非正交的,这里设置了两次。
2.6 计算设置
打开system/controlDict进行计算上的一些设置,包括时间步长、开始和结束的时间、多少步输出一次以及输出一些后处理要用的信息。
/*--------------------------------*- C++ -*----------------------------------*\
| ========= | |
| \\ / F ield | OpenFOAM: The Open Source CFD Toolbox |
| \\ / O peration | Version: 4.x |
| \\ / A nd | Web: www.OpenFOAM.org |
| \\/ M anipulation | |
\*---------------------------------------------------------------------------*/
FoamFile
{
version 2.0;
format ascii;
class dictionary;
object controlDict;
}
// * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * //
application icoFoam;
//startFrom startTime;
startFrom latestTime;
startTime 0;
stopAt endTime;
//stopAt writeNow;
endTime 6;
//endTime 500;
//deltaT 0.005;
//deltaT 0.01;
//deltaT 0.02;
deltaT 0.0005;
writeControl runTime;
/*
adjustableRunTime
clockTime
cpuTime
runTime
timeStep
*/
writeInterval 0.5;
/*
secondaryWriteControl cpuTime;
secondaryWriteInterval 1000;
secondaryPurgeWrite 1;
*/
purgeWrite 20;
writeFormat ascii;
writePrecision 8;
writeCompression off;
timeFormat general;
timePrecision 6;
runTimeModifiable true;
// ************************************************************************* //
functions
{
fieldAverage
{
type fieldAverage;
functionObjectLibs ("libfieldFunctionObjects.so");
enabled true;
writeControl outputTime;
//writeControl timeStep;
//writeInterval 100;
//cleanRestart true;
//timeStart 20;
//timeEnd 200;
fields
(
p
{
mean on;
prime2Mean on;
base time;
}
U
{
mean on;
prime2Mean on;
base time;
}
);
}
forceCoeffs
{
type forceCoeffs;
functionObjectLibs ("libforces.so");
patches (CYLINDER);
log true;
rho rhoInf;
rhoInf 1;
CofR (0 0 0);
liftDir (0 1 0);
dragDir (1 0 0);
pitchAxis (0 0 1);
magUInf 17;
lRef 1;
Aref 1;
writeControl timeStep;
writeInterval 1;
}
};这里特别说明计算步长和总时间。计算步长的设置最好使得计算时最大库朗数(Courant,CFL)小于1。虽然说SIMPLE是半隐式的求解方法,隐式格式无条件稳定,我们还是希望流场的计算能稳定一些。这里设置deltaT为0.0005,max CFL在0.5左右。总时长取6s,差不多是20个周期的时间。计算过程如下:
首先考虑圆柱绕流的无量纲频率St数:
一般圆柱绕流的St在0.2左右,f为实际涡脱落的频率,取倒数计算得到一个涡脱落周期的时间:
因此20个周期取6s。做圆柱绕流算例要关注平均场、扰动场和升阻力系数,这些在controlDict后面的functions中进行设置,在此不一一细说。
2.7 开始计算
如果要并行,就要设置decomposeParDict,然后用mpi指令进行计算。这里只用单核进行,在终端直接输入:
icoFoam即可开始计算。计算时间视计算性能来决定。
3. 后处理看结果
算完之后,用tecplot打开postProcessing/forceCoeffs/0,看一下升阻力系数曲线:
可以看到后半段是进入了相对稳定的阶段,流场出现了卡门涡街,升阻力系数呈周期性变化。从升力系数估算到St=0.235,平均阻力系数为1.35。对比一些文献:
这里看出和文献还是有一些出入的,具体原因有网格分辨率、数值格式、计算方法等等,这里面单一个拎出来都是很大的课题,慢慢去掌握吧。后面自己再慢慢修改,这里就不放出来了。后面放一些流场的图:
流向平均速度云图:
平均压强云图:
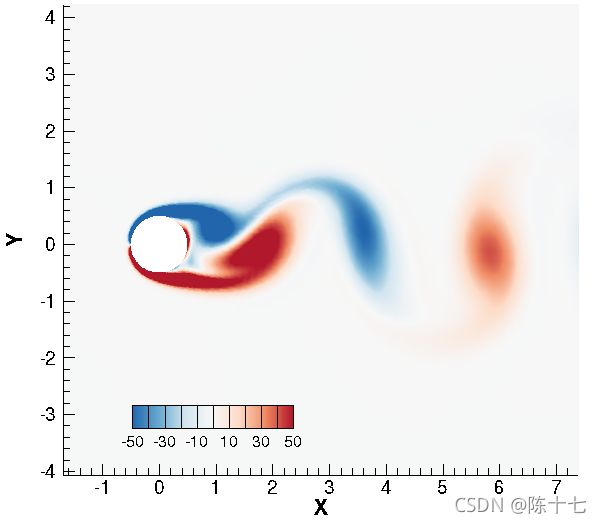
还有涡量图,涡量的计算可以看我以前发的视频。
当然还需要提取某一条线上的流场变量和文献的作对比,现在这篇内容太多了,我放到后面再详细记录。
总结
这次在OpenFOAM上对雷诺数为200的圆柱绕流算例进行了比较完整的模拟仿真,包括画网格、设置算例、计算以及后处理比较结果。CFD计算流体力学是一门涉及面很广同时又需要你每一项都精通的学科,因为如果某一个环节处理不当,是会影响整一个仿真的效果的,包括但不限于网格分辨率、数值格式的选取、计算方法的选择、解方程的设置等等,每一项都需要仔细地进行。好在CFD是活用计算机的一种工具,以程序的形式进行流体力学的模拟,具有知识的复用性,也就可以在设备里储存起来,也方便大家的交流和分享。