Python实现博弈树minmax补全与α-β剪枝算法脚本简介

---

前言

使用Python编程实现博弈树的构建，实现利用MinMax方法补全博弈树缺失值，并结合α-β剪枝算法，实现博弈树的剪枝。实现了整体算法与博弈树的可视化。

---

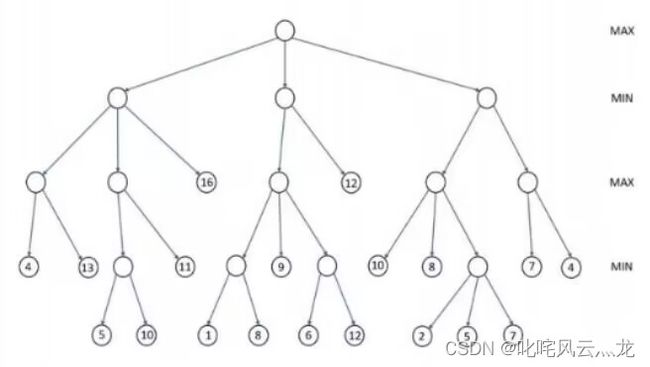
一、题目

博弈树初始结构如下

二、使用步骤

1.递归构建博弈树

代码如下：

class Node(object):

    def __init__(self, val: int=0, max: bool=True) -> None:
        '''
        val: 节点值
        max: 是否为max层
        childern: 子节点列表
        '''
        self.val = val	# 该节点的值
        self.max = max	# 该层是否为max层，默认顶层节点为max层
        self.children : list = []	# 该节点的子节点


class Tree(object):

    def __init__(self) -> None:
        '''
        data_list: 数据列表
        上图所示列表示例：[[[4,13],[[5,10],11],16],[[[1,8],9,[6,12]],12],[[10,8,[2,5,7]],[7,4]]]
        其中节点值为一个int型数值，含有其他子节点的构建为列表
        '''
        self.root = Node()    # Node()，根节点

    def build_tree(self, data_list, root) -> None:
        # 递归构建博弈树
        for i in range(len(data_list)):

            is_max = root.max
            if is_max == True:
                is_max = False  # min层
            else:   
                is_max = True   # max层

            if type(data_list[i]) is tuple:
                # 该节点为叶节点，直接添加节点值
                for val in data_list[i]:
                    root.children.append(Node(val, is_max))
            elif type(data_list[i]) is int:
                # 该节点为叶节点，直接添加节点值
                root.children.append(Node(data_list[i], is_max))
            elif type(data_list[i]) is list:
                # 该节点含有子节点，递归创建
                if type(data_list[i]) is int:
                    # 该节点为叶节点，直接添加节点值
                    root.children.append(Node(data, is_max))
                elif type(data_list[i]) is list:
                    # 该节点含有子节点，递归创建
                    root.children.append(Node(max=is_max))      # 添加子节点
                    self.build_tree(data_list[i], root.children[i])

2.α-β剪枝算法

代码如下：

class AlphaBeta(object):

    def __init__(self, tree, auto=False) -> None:
    	'''
    	tree: 博弈树
    	auto: 补全全部节点值，默认不补全
    	选择不补全时，可视化过程为剪枝过程，节点为0的子节点为剪掉的节点
    	'''
        self.tree = tree	# 博弈树
        self.auto = auto    # 补全全部节点值，可选参数，默认不补全
        self.deep = 0		# 节点深度
        self.alpha = -float('inf')
        self.beta = float('inf')

        if self.auto:
        	# 补全博弈树
            self.complement_value(self.tree.root)
    
    def get_value(self, node) -> int:
        # 获取节点值
        return node.val

    def is_leaf(self, node) -> bool:
        # 判断是否为叶节点
        if len(node.children) == 0:
            return True
        else:
            return False

    def complement_value(self, node):	# 补全博弈树，可选
        # 根据MinMax规则补全博弈树
        if self.is_leaf(node):
            return self.get_value(node)
        if self.get_value(node) != 0:
            return self.get_value(node)

        val_list = []
        for child in node.children:
            temp = self.complement_value(child)
            val_list.append(temp if temp is not None else child.val)
        if node.max:
            node.val = max(val_list)
        else:
            node.val = min(val_list)

    def max_value(self, node, alpha, beta):

        if self.is_leaf(node):
            # 当前节点为叶节点
            return self.get_value(node)

        best = -float('inf')     # 初始化无穷小
        for child in node.children:
            best = max(best, self.min_value(child, alpha, beta))
            if best >= beta:
                return best
            alpha = max(alpha, best)
        node.val = best
        return best

    def min_value(self, node, alpha, beta):

        if self.is_leaf(node):
            # 当前节点为叶节点
            return self.get_value(node)
        
        best = float('inf')     # 初始化无穷大
        for child in node.children:
            best = min(best, self.max_value(child, alpha, beta))
            if best <= alpha:
                return best
            beta = min(beta, best)
        node.val = best
        return best

    def alpha_beta(self):	

        best = self.max_value(self.tree.root, self.alpha, self.beta)
        # return best
        for child in self.tree.root.children:
            if best == child.val:
                return child

3.博弈树可视化

代码如下：

import matplotlib.pyplot as plt


class ShowTree(object):

    def __init__(self, tree) -> None:
		'''
		tree: 博弈树
		'''
        self.tree = tree	# 博弈树

        self.__num_of_leafs = self.get_num_of_leaf(self.tree.root)    # 叶节点数量
        self.__tree_depth = self.get_tree_depth(self.tree.root)       # 树深度

        # 初始化箭头格式
        self.arrow_args = dict(arrowstyle="<-")

    @property
    def num_of_leafs(self):
        return self.__num_of_leafs

    @property
    def tree_depth(self):
        return self.__tree_depth
    
    def get_num_of_leaf(self, node):
        # 获取叶节点数量
        num_of_leafs = 0
        if len(node.children) == 0:
            # 该节点为叶节点，叶节点数量+1
            num_of_leafs += 1
        else:
            for child in node.children:
                num_of_leafs += self.get_num_of_leaf(child)
        return num_of_leafs

    def get_tree_depth(self, node):
        # 获取树的最大深度
        max_tree_depth = 0
        if len(node.children) == 0:
            # 该节点为叶节点，深度为1
            max_tree_depth += 1
        else:
            for child in node.children:
                this_depth = 1 + self.get_tree_depth(child)
            if this_depth > max_tree_depth:
                max_tree_depth = this_depth
        return max_tree_depth

    def box(self, node):
    	# 设置文本框样式
        if node.max:
            boxstyle = "square"
        else:
            boxstyle = "circle"
        return dict(boxstyle=boxstyle,fc="0.8")

    def plot_node(self, node, centerPt, parentPt):
        node_type = self.box(node)
        # node_type = dict(boxstyle="round4",fc="0.8")
        ShowTree.plot.ax1.annotate(node.val, xy=parentPt, \
        xycoords='axes fraction',
        xytext=centerPt, textcoords='axes fraction',\
        va="center",ha="center", bbox=node_type, arrowprops=self.arrow_args)
    
    @staticmethod
    def plot_tree(tree, node, parentPt):
        numLeafs = ShowTree.get_num_of_leaf(tree, node) # 计算树的宽度
        depth = ShowTree.get_tree_depth(tree, node)     # 计算树的高度
        # 输入的第一个节点
        first_node = node
        cntrPt = (ShowTree.plot_tree.xOff + (1.0 + float(numLeafs)) / 2.0 / ShowTree.plot_tree.totalW, ShowTree.plot_tree.yOff)
        # 叶子节点
        ShowTree.plot_node(tree, first_node, cntrPt, parentPt)
        # 减少y的便偏移
        ShowTree.plot_tree.yOff = ShowTree.plot_tree.yOff - 1.0 / ShowTree.plot_tree.totalD 
        for child in node.children:
            if len(child.children) == 0:
                ShowTree.plot_tree.xOff = ShowTree.plot_tree.xOff + 1.0 / ShowTree.plot_tree.totalW
                ShowTree.plot_node(tree, child, (ShowTree.plot_tree.xOff, ShowTree.plot_tree.yOff), cntrPt)
            else:
                ShowTree.plot_tree(tree, child, cntrPt)
        ShowTree.plot_tree.yOff = ShowTree.plot_tree.yOff + 1.0 / ShowTree.plot_tree.totalD

    @staticmethod
    def plot(tree):
        '''
        tree: 需要绘制的树，类型为ShowTree()
        '''
        fig = plt.figure(1, facecolor='white')
        # Clear figure清除所有轴，但是窗口打开，这样它可以被重复使用
        fig.clf()
        axprops = dict(xticks=[], yticks=[])
        ShowTree.plot.ax1 = plt.subplot(111, frameon=False, **axprops)
        # ShowTree.plot_node(show_tree, show_tree.tree.root, (0.5,0.1),(0.1,0.5))
        ShowTree.plot_tree.totalW = float(ShowTree.get_num_of_leaf(tree, tree.tree.root))
        ShowTree.plot_tree.totalD = float(ShowTree.get_tree_depth(tree, tree.tree.root))
        ShowTree.plot_tree.xOff = -0.5 / ShowTree.plot_tree.totalW
        ShowTree.plot_tree.yOff = 1.0
        ShowTree.plot_tree(tree, tree.tree.root,(0.5, 1.0))
        plt.show()

4.测试实例

代码如下：

if __name__ == '__main__':

    data = [[[4,13],[[5,10],11],16],[[[1,8],9,[6,12]],12],[[10,8,[2,5,7]],[7,4]]]	# 初始博弈树值列表
    tree = Tree()	# 实例化空树
    tree.build_tree(data, tree.root)	# 递归构建博弈树
    alpha_beta = AlphaBeta(tree, auto=True)	# α-β剪枝
    best = alpha_beta.alpha_beta()		# 获取结果
    print(best.val)

    from plot_tree import ShowTree
    show_tree = ShowTree(tree)	# 实例化博弈树可视化算法

    show_tree.plot(show_tree)	# 可视化博弈树

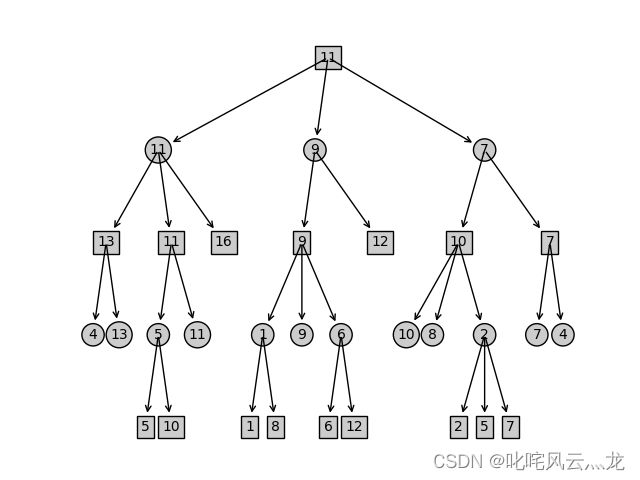
5.结果展示

6.全部代码

全部代码如下alpha_beta.zip

---

总结

利用Python编程实现了α-β剪枝算法，并利用matplotlib实现了博弈树的可视化。