用Python绘制傅里叶级数和泰勒级数逼近已知函数的动态过程
文章目录
-
- Taylor级数
- Fourier级数
本文代码: Fourier级数和Taylor级数对原函数的逼近动画
Taylor级数
级数是对已知函数的一种逼近,比较容易理解的是Taylor级数,通过多项式来逼近有限区间内的函数,其一般形式为
f ( x ) = ∑ n = 0 N a n x n f(x)=\sum_{n=0}^N a_nx^n f(x)=n=0∑Nanxn
其中最著名的应该是自然指数,根据其导数不变的特点,我们可以很容易得到其表达式
e x = ∑ n = 0 N x n n ! e^x=\sum_{n=0}^N \frac{x^n}{n!} ex=n=0∑Nn!xn
随着N的不断增加,其逼近过程如图所示
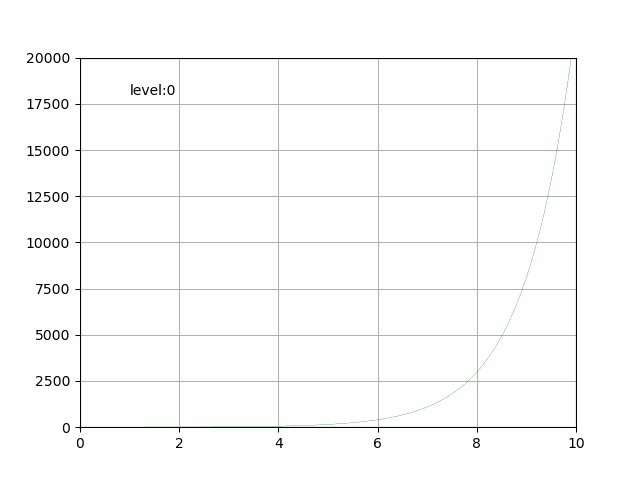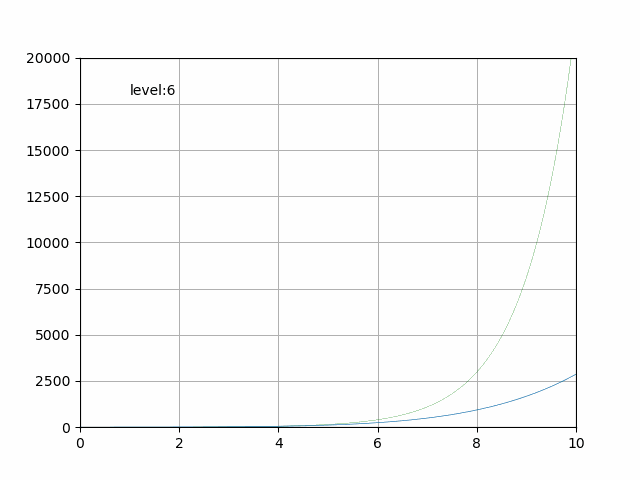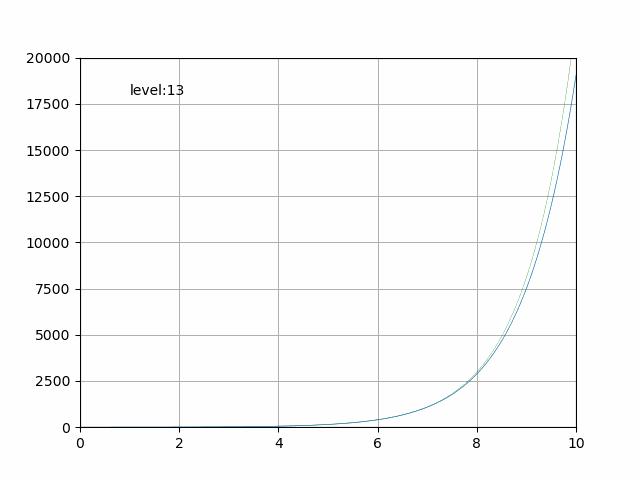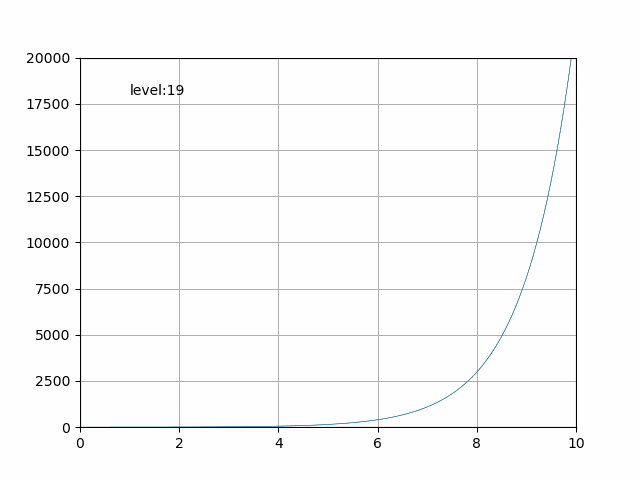
其中,Taylor级数的实现方法如下,除了exp函数之外,还包括sin和cos函数的Taylor级数。
def Taylor(x,funcType='exp',n=0):
func = {
'exp' : lambda x,n : x**n/fac(n),
'sin' : lambda x,n : (-1)**n*x**(2*n+1)/fac(2*n+1),
'cos' : lambda x,n : (-1)**n*x**(2*n)/fac(2*n)
}
return func[funcType](x,n)
绘图代码如下
def approxGif(num=30, funcType='exp'):
funcType = func if type(func)==str else 'auto'
if type(func)==str:
func = funcDict[func]
x = np.linspace(0,10,1000)
Y =func if type(func)==type(x) else func(x)
if method in ['Taylor','taylor']:
y = Taylor(x,funcType,0)
elif method in ['Fourier','fourier']:
y = Fourier(x,funcType,0)
num = range(num)
#画图初始化
fig = plt.figure()
ax = fig.add_subplot(111,autoscale_on=False,
xlim=(0,10),ylim=(min(Y)-0.5,max(Y)+0.5))
ax.plot(x,Y,color='g',lw=0.2)
ax.grid()
line, = ax.plot([],[],lw=0.5)
time_text = ax.text(0.1,0.9,'',transform=ax.transAxes)
# 动画初始化
def init():
line.set_data([],[])
time_text.set_text('level:'+str(0))
return line, time_text
# 动画迭代
def animate(n):
nonlocal y
y = Taylor(x,funcType,n) if n==0 else y+Taylor(x,funcType,n)
line.set_data(x,y)
time_text.set_text('level:'+str(n))
print(n)
return line, time_text
ani = animation.FuncAnimation(fig,animate,
num,interval=200,blit=False,init_func=init)
#ani.save(funcType+'.gif',writer='pillow')
plt.show()
Fourier级数
Fourier级数也是本着相同的思维,只不过采用了不同频率的三角函数作为其空间中的基底。
对于以 2 π 2\pi 2π为周期的方波信号
f ( x ) = { 1 x ∈ [ 0 , π ) − 1 x ∈ [ − π , 0 ) f(x)=\left\{\begin{aligned} 1\quad &x \in[0,\pi)\\ -1\quad &x\in[-\pi,0) \end{aligned}\right. f(x)={1−1x∈[0,π)x∈[−π,0)
其Fourier级数为
f ( x ) = 4 π ∑ n = 0 N sin x 2 n + 1 f(x)=\frac{4}{\pi}\sum_{n=0}^N\frac{\sin x}{2n+1} f(x)=π4n=0∑N2n+1sinx
实现为
def Fourier(x,funcType='square',n=0):
func = {
'square' : lambda x,n : 4/np.pi*np.sin((2*n+1)*x)/(2*n+1),
'tri' : lambda x,n: np.pi/2 if n == 0 \
else -4/np.pi*np.cos((2*n-1)*x)/(2*n-1)**2,
'oblique': lambda x,n : 2*np.sin((n+1)*x)/(n+1)*(-1)**n
}
return func[funcType](x,n)
绘图代码为
def square(x):
x = np.mod(x,np.pi*2)
x[x>np.pi] = -1
x[x!=-1] = 1
return x
def tri(x):
return np.pi-np.abs(np.mod(x,2*np.pi)-np.pi)
funcDict = {
'exp':np.exp,
'sin':np.sin,
'cos':np.cos,
'square':square,
'tri':tri,
}
# func支持三种输入模式,即字符串,函数以及numpy数组
def approxGif(func='square',method='fourier',num=30):
funcType = func if type(func)==str else 'auto'
if type(func)==str:
func = funcDict[func]
x = np.linspace(0,10,1000)
Y =func if type(func)==type(x) else func(x)
if method in ['Taylor','taylor']:
y = Taylor(x,funcType,0)
elif method in ['Fourier','fourier']:
y = Fourier(x,funcType,0)
num = range(num)
#画图初始化
fig = plt.figure()
ax = fig.add_subplot(111,autoscale_on=False,
xlim=(0,10),ylim=(min(Y)-0.5,max(Y)+0.5))
ax.plot(x,Y,color='g',lw=0.2)
ax.grid()
line, = ax.plot([],[],lw=0.5)
time_text = ax.text(0.1,0.9,'',transform=ax.transAxes)
# 动画初始化
def init():
line.set_data([],[])
time_text.set_text('level:'+str(0))
return line, time_text
# 动画迭代
def animate(n):
nonlocal y
if method in ['taylor','Taylor']:
y = Taylor(x,funcType,n) if n==0 \
else y+Taylor(x,funcType,n)
elif method in ['fourier','Fourier']:
y = Fourier(x,funcType,n) if n==0 \
else y+Fourier(x,funcType,n)
pass
line.set_data(x,y)
time_text.set_text('level:'+str(n))
print(n)
return line, time_text
ani = animation.FuncAnimation(fig,animate,
num,interval=200,blit=False,init_func=init)
#ani.save(funcType+'.gif',writer='pillow')
plt.show()
如图所示
相应地三角波为
 |
上述只是给出了几个直观的例子,用以表明Taylor级数和Fourier级数的使用方法,从而让我们具备这种通过多项式或者三角函数来逼近已知函数的意识。而对于已知表达形式的函数 y = f ( x ) y=f(x) y=f(x),Taylor级数和Fourier级数都有导数或者积分的表示形式。