暴力算法 --- 莫队
文章目录
-
-
- 莫队
-
- 基础莫队
- 带修改莫队
- 树上莫队
- 回滚莫队
- 例题:
-
- ABC293_G
-
莫队
什么是莫队?
答:优雅的暴力!!!
基础莫队
重复的数
题目描述:给出一个长度为 N N N的序列,有若干查询,每次查询区间 [ l i , r i ] [l_i, r_i] [li,ri]出现 k i k_i ki次的数有多少个?
解题思路:最直观的做法就是拿到区间 [ l , r ] [l, r] [l,r]后,暴力计算,用 c n t [ x ] cnt[x] cnt[x]保存 x x x出现了几个, p o s [ t ] pos[t] pos[t]出现 t t t次的数有几个。此时,时间复杂度最坏为: O ( n 2 ) \mathcal O(n^2) O(n2)
可以尝试来优化一下这个操作:使用两个指针 L L L和 R R R(初始化: L = 1 , R = 0 L = 1, R = 0 L=1,R=0),对于每次查询,依次的把这两个指针移动到目标区间 [ l , r ] [l, r] [l,r]的同时,更新 c n t cnt cnt和 p o s pos pos数组。假设现在目标区间为: [ 1 , 5 ] [1, 5] [1,5], L = 2 , R = 5 L = 2, R = 5 L=2,R=5;移动 L L L指针左移L--,并更新 c n t cnt cnt和 p o s pos pos数组:
L --;
pos[cnt[a[L]]] --;
cnt[a[L]] ++;
pos[cnt[a[L]]] ++;
移完以后,同理移动 R R R指针:
R ++;
pos[cnt[a[R]]] --;
cnt[a[R]] ++;
pos[cnt[a[R]]] ++;
可以发现上述都是把不在指针区间 [ L , R ] [L, R] [L,R]的数加进来了,因此可以封装成一个函数:
void add(int x) { // 把一个数x加入当指针区间内
pos[cnt[x]] --;
cnt[x] ++;
pos[cnt[x]] ++;
}
有增加就有删除,删除也是一样的:
void del(int x) { // 把一个数从指针区间删除
pos[cnt[x]] --;
cnt[x] --;
pos[cnt[x]] ++;
}
为了减少 [ L , R ] [L, R] [L,R]这两个指针的移动次数,可以先将序列分成 n \sqrt n n块,并从 1 ∼ n 1 \sim \sqrt n 1∼n编号,对于所有的查询按照块编号,从小到大排序,对于同一块,按照区间右端点升序排序。排完序后,我们再按照上述的操作去移动 L , R L, R L,R指针,这样的时间复杂度为: O ( n n ) \mathcal O(n\sqrt n) O(nn)
简单证明一下:
- 区间排序,
sort一遍, O ( n l o g n ) \mathcal O(n log n) O(nlogn) - L L L指针的移动:对于每块最坏跨越整个块 n \sqrt n n,最坏有 n n n块需要移动,总的: O ( n n ) \mathcal O(n\sqrt n) O(nn)
- R R R指针的移动,因为对于每块,区间是按照右端点升序排序,所以在块内最多也移动 n \sqrt n n,最坏有 n n n块需要移动,总的 O ( n n ) \mathcal O(n \sqrt n) O(nn)
综上述:莫队的总时间复杂度为: O ( n l o g n ) + O ( n n ) + O ( n n ) = O ( n n ) \mathcal O(nlogn) + \mathcal O (n \sqrt n) + \mathcal O(n \sqrt n) = \mathcal O(n \sqrt n) O(nlogn)+O(nn)+O(nn)=O(nn)
AC_Code:
#include 带修改莫队
P1903 [国家集训队] 数颜色 / 维护队列
题目描述:有长度为 N N N的序列,现在有两个操作:
- Q L R Q \ L \ R Q L R 区间 [ L , R ] [L, R] [L,R]有多少个不同的数
- R P C R \ P \ C R P C 把 P P P位置的数改成 C C C
解题思路:查询操作可以用莫队轻松解决,可是这个修改要怎么办呢?我们可以加上一维时间,使之变为待修改莫队!具体操作如下:
增加一维时间t,在把区间移动到目标区间后,检查当前时间是不是目标时间,不是的话,跟修改 L , R L, R L,R指针一样去修改时间t。
这里要注意的是,比如你现在把某个位置的数 x x x改成了 c c c,此时的时间为 t 1 t_1 t1,在把 x x x修改为 c c c后,这个时候你要把原来的数 x x x记下来,如果以后询问小于 t 1 t_1 t1的时候,这个位置的数是 x x x而不是 c c c,这里有个简化操作,可以直接把 x x x和 c c c互换即可,下次操作直接更改就行(具体细节看代码
AC_Code:
#include 树上莫队
COT2 - Count on a tree II
题目描述:给定 N N N个节点的树,每个节点有一个颜色。现在有 m m m次询问,每次询问给出两个节点 u , v u,v u,v,输出 u , v u,v u,v路径上的节点颜色个数。颜色为 ≤ 2 × 1 0 9 \le 2 × 10^9 ≤2×109的非负整数
解题思路:
先得到树的欧拉序。
欧拉序特点:
每个节点出现两次,且他们中间的节点为这个节点子树上的点
欧拉序的求法:
在dfs的时候,得到一个点加入序列,退出的时候也加入序列,这样就得到了欧拉序
得到欧拉序有什么用呢,可以尝试将两个节点映射成欧拉序上的一段区间,这样问题就可以转换为莫队求区间不同数了。
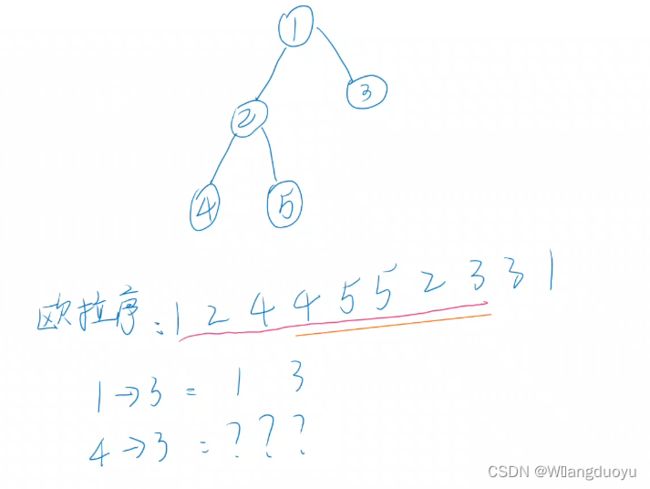
看上图,1->3很容易得到(就是图中红线)而且之间出现两次的数刚好不会算在其中。但是4->3怎么算?用第一个4指定不行,只能用第二个4,但是我们发现少了1,但是1是什么呢,是4和3的lca!所以,可以得到下面两个结论!(保证 f i r s t [ x ] < f i r s t [ y ] first[x] < first[y] first[x]<first[y],不满足就交换一下)
- 如果 L C A ( x , y ) = = x LCA(x, y) == x LCA(x,y)==x,区间为 [ f i r s t [ x ] , f i r s t [ y ] [first[x], first[y] [first[x],first[y] 对应上图1->3情况
- 如果 L C A ( x , y ) ! = x LCA(x, y) \ != x LCA(x,y) !=x,区间为 [ s e c o n d [ x ] , f i r s t [ y ] ] [second[x], first[y]] [second[x],first[y]],同时标记lca为 L C A ( x , y ) LCA(x, y) LCA(x,y),在计算的时候加上lca
AC_Code:
#include 回滚莫队
// TODO 待补
例题:
ABC293_G
题目链接:点我
题意:有一个长度为 N N N的序列,现在有 m m m次询问,每次询问一段区间内 ( i < j < k ) (i \lt j\lt k) (i<j<k)并且 a i = a j = a k a_i = a_j = a_k ai=aj=ak的对数
sol:利用莫队,往区间加数 X X X的时候,对答案增加 c n t [ x ] ∗ ( c n t [ x ] − 1 ) / 2 cnt[x] * (cnt[x] - 1) / 2 cnt[x]∗(cnt[x]−1)/2,当前这一个和已有的匹配,删除同理
Code:
#include