PTA甲级-1010 Radix c++
文章目录
-
-
- Input Specification:
- Output Specification:
- Sample Input 1:
- Sample Output 1:
- Sample Input 2:
- Sample Output 2:
-
- 一、题干大意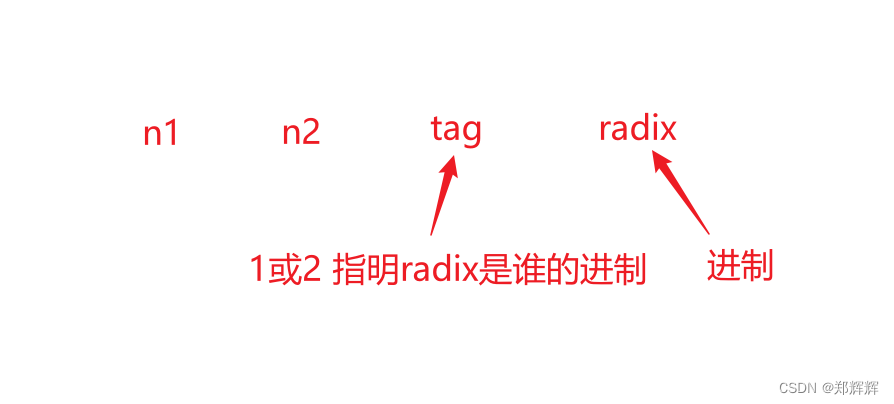
- 二、题解要点
- 三、具体实现
- 总结
Given a pair of positive integers, for example, 6 and 110, can this equation 6 = 110 be true? The answer is yes, if 6 is a decimal number and 110 is a binary number.
Now for any pair of positive integers N1 and N2, your task is to find the radix of one number while that of the other is given.
Input Specification:
Each input file contains one test case. Each case occupies a line which contains 4 positive integers:
N1 N2 tag radix
Here N1 and N2 each has no more than 10 digits. A digit is less than its radix and is chosen from the set { 0-9, a-z } where 0-9 represent the decimal numbers 0-9, and a-z represent the decimal numbers 10-35. The last number radix is the radix of N1 if tag is 1, or of N2 if tag is 2.
Output Specification:
For each test case, print in one line the radix of the other number so that the equation N1 = N2 is true. If the equation is impossible, print Impossible. If the solution is not unique, output the smallest possible radix.
Sample Input 1:
6 110 1 10
Sample Output 1:
2
Sample Input 2:
1 ab 1 2
Sample Output 2:
Impossible
一、题干大意
给出两个字符串和其中一个字符串的进制,求出另一个字符串的进制,使两者的值相等。最后输出求出的进制,如果不存在这种进制就输出 “Impossible”
二、题解要点
- 两个函数,一个用来进行进制转化,也就是把任意进制的字符串转化为十进制的数。另一个用二分法求出进制。
- 一定要注意:在二分法求进制的过程中,求出的进制是有可能上溢的,如果不解决就只能拿15分。
三、具体实现
/**
*@Author:hhzheng
*@Date:2023/2/6 10:58
*@Filename:PAT甲级1010 Radix
*/
#include总结
全网的写法思路基本差不多,决定成败的就是在如何这个进制的上溢。