Definition of regularity in PDE theory

Regularity is one of the vague yet very useful terms to talk about a vast variety of results in a uniform way. Other examples of such words include “dynamics” in dynamical systems (I have never seen a real definition of this term but everyone uses it, and it vaguely means the way a system changes over time) or “canonical” (roughly meaning that with just the information given, a canonical choice is the most obvious choice) in more algebraic contexts.

In general, more regularity means more desirable properties.

Typically this means one or several of the following:

Higher integrability, i.e. a function lies in a more restrictive Lp space, i.e. higher powers of the function are integrable

Higher differentiability, i.e. higher (weak) derivatives of the function exist.

正则性是一个模糊但非常有用的术语，可以用统一的方式谈论各种各样的结果。这类词的其他例子包括动力系统中的“动力学”（我从未见过这个词的真正定义，但每个人都使用它，它模糊地意味着系统随时间变化的方式）或更多代数上下文中的“规范”（大致意思是，只要给出信息，规范选择是最明显的选择）。

一般来说，更多的正则性意味着更理想的特性。

通常，这意味着以下一项或多项：

更高的可积性，即函数位于更严格的Lp空间中，即函数的更高幂是可积的

更高的可微性，即存在函数的更高（弱）导数。（百度翻译）

Furthermore, the issue of regularity is often isolated in proofs, so e.g. if you want to prove existence and uniqueness of a classical solution (i.e. “strongly” differentiable function that actually satisfies a PDE in exactly the way that it is posed) to a PDE, you first show existence and uniqueness of a weak solution which is often much easier (and allows to use tools from, for example, Hilbert space theory which are not available in most cases when looking for strong solutions).

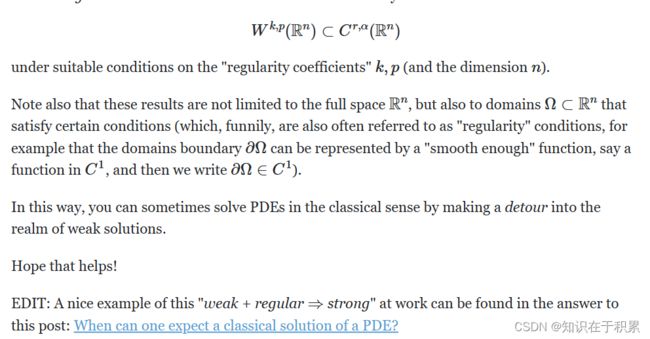
And only then you proceed to show that this solution is actually much more regular, for example, it actually possesses not only weak but even strong derivatives. A typical way to achieve this is using the Sobolev embedding theorems which tell you that if a function has sufficiently many weak derivatives, or rather, sufficiently regular weak derivatives (i.e. lies in a Sobolev space Wkp with large enough k and large enough p), then it can be identified with a function that is actually strongly differentiable. This statement is called the “second part of the Sobolev embedding theorem” in the article linked above and is nicely summarised in the statement

此外，正则性问题通常在证明中是孤立的，因此，例如，如果你想证明一个经典解（即“强”可微函数，它实际上以其提出的方式满足PDE）对PDE的存在性和唯一性，你首先展示了弱解的存在性和唯一性，这通常要容易得多（并允许使用希尔伯特空间理论中的工具，例如，在寻找强解时，这些工具在大多数情况下都不可用）。（百度翻译）

继续或进一步的去证明这个解是更加正则的，例如，它实际上不仅具有弱导数，甚至具有强导数。实现这一点的一个典型方法是使用Sobolev嵌入定理，该定理告诉你，如果一个函数有足够多的弱导数，或者更确切地说，有足够正则的弱导数（即位于具有足够大的k和足够大的p的Sobolev空间Wkp中），那么它可以被识别为一个实际上是强可微的函数。这个陈述在上面链接的文章中被称为“索博列夫嵌入定理的第二部分”，并在陈述中得到了很好的总结（百度翻译）

https://math.stackexchange.com/questions/2372091/definition-of-regularity-in-pde-theory

https://en.wikipedia.org/wiki/Sobolev_inequality#Sobolev_embedding_theorem

https://math.stackexchange.com/questions/3230253/what-is-regularity-of-solutions