基于matlab的lorenz混沌系统仿真与分析

目录

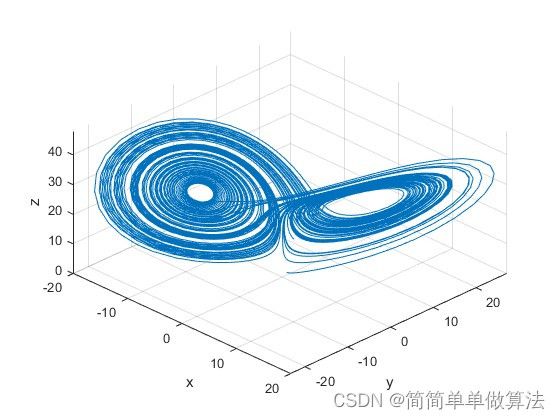
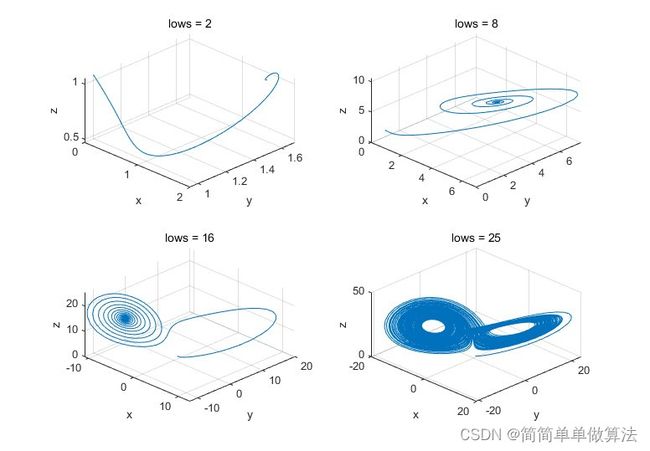
1.算法运行效果图预览

2.算法运行软件版本

3.部分核心程序

4.算法理论概述

5.算法完整程序工程

---

1.算法运行效果图预览

 

2.算法运行软件版本

matlab2022a

3.部分核心程序

.....................................................................................
lows     = 28;
[t1,XX]  = ode45(@func_lorenz2,[0 100],[0 1 1.05]);%use the function of ode45 to solve the function
RR1      = XX; 

lows     = 28.05;
[t1,XX]  = ode45(@func_lorenz2,[0 100],[0 1 1.05]);%use the function of ode45 to solve the function
RR2      = XX; 

lows     = 27.95;
[t1,XX]  = ode45(@func_lorenz2,[0 100],[0 1 1.05]);%use the function of ode45 to solve the function
RR3      = XX; 



L = min([length(RR1),length(RR2),length(RR3)]);
%先针对p值变大的情况来计算rate
D1 = [RR1(1:L,1) - RR2(1:L,1)  ,  RR1(1:L,2) - RR2(1:L,2)  ,  RR1(1:L,3) - RR2(1:L,3)];

%计算rate
D1d= [diff(RR1(1:L,1) - RR2(1:L,1)) , diff(RR1(1:L,2) - RR2(1:L,2)) , diff(RR1(1:L,3) - RR2(1:L,3))];



%再针对p值变小的情况来计算rate
D2 = [RR1(1:L,1) - RR3(1:L,1)  ,  RR1(1:L,2) - RR3(1:L,2)  ,  RR1(1:L,3) - RR3(1:L,3)];
%计算rate
D2d= [diff(RR1(1:L,1) - RR3(1:L,1)) , diff(RR1(1:L,2) - RR3(1:L,2)) , diff(RR1(1:L,3) - RR3(1:L,3))];

figure;
subplot(211);
plot(D1d(:,1),'m-');hold on
plot(D1d(:,2),'r-');hold on
plot(D1d(:,3),'b-');hold off
title('28.1,[0,1,1.05]');
legend('X','Y','Z');
grid on;

subplot(212);
plot(D2d(:,1),'m-');hold on
plot(D2d(:,2),'r-');hold on
plot(D2d(:,3),'b-');hold off
title('27.9,[0,1,1.05]');
legend('X','Y','Z');
grid on;



%上面是相近的P值来计算最后的rate，下面考虑改变初始值，来计算rate
%上面是相近的P值来计算最后的rate，下面考虑改变初始值，来计算rate
global XX;
global lows;

lows     = 28;
[t1,XX]  = ode45(@func_lorenz2,[0 100],[0 1 1.05]);%use the function of ode45 to solve the function
RR1      = XX; 

[t1,XX]  = ode45(@func_lorenz2,[0 100],[0 1 1.06]);%use the function of ode45 to solve the function
RR2      = XX; 

[t1,XX]  = ode45(@func_lorenz2,[0 100],[0 1 1.04]);%use the function of ode45 to solve the function
RR3      = XX; 



L = min([length(RR1),length(RR2),length(RR3)]);
%先针对p值变大的情况来计算rate
D1 = [RR1(1:L,1) - RR2(1:L,1)  ,  RR1(1:L,2) - RR2(1:L,2)  ,  RR1(1:L,3) - RR2(1:L,3)];
%计算rate
D1d= [diff(RR1(1:L,1) - RR2(1:L,1)) , diff(RR1(1:L,2) - RR2(1:L,2)) , diff(RR1(1:L,3) - RR2(1:L,3))];



%再针对p值变小的情况来计算rate
D2 = [RR1(1:L,1) - RR3(1:L,1)  ,  RR1(1:L,2) - RR3(1:L,2)  ,  RR1(1:L,3) - RR3(1:L,3)];
%计算rate
D2d= [diff(RR1(1:L,1) - RR3(1:L,1)) , diff(RR1(1:L,2) - RR3(1:L,2)) , diff(RR1(1:L,3) - RR3(1:L,3))];

figure;
subplot(211);
plot(D1d(:,1),'m-');hold on
plot(D1d(:,2),'r-');hold on
plot(D1d(:,3),'b-');hold off
title('28,[0,1,1.06]');
legend('X','Y','Z');
grid on;

subplot(212);
plot(D2d(:,1),'m-');hold on
plot(D2d(:,2),'r-');hold on
plot(D2d(:,3),'b-');hold off
title('28,[0,1,1.04]');
legend('X','Y','Z');
grid on;
07_002m

4.算法理论概述

       洛伦兹混沌系统是一种非线性动力系统，最初由爱德华·洛伦兹（Edward Lorenz）于1963年引入，它的简单方程组引发了混沌理论的开创性研究。该系统是混沌现象的典型范例，展示了复杂、不可预测的行为，即使在简单的数学方程下也可以观察到这种行为。

原理：
       洛伦兹混沌系统由三个耦合的一阶常微分方程组组成，这三个方程描述了三个状态变量（或者说是维度）之间的关系。这些状态变量表示系统在空间中的位置，从而形成一个三维相空间，该相空间中的轨迹表现出异常的复杂性和混沌特性。

洛伦兹方程组描述如下：

         其中，x、y 和 z 是状态变量，t是时间，而 \sigmaσ、\rhoρ 和 \betaβ 则是系统的参数。这些参数的取值会影响洛伦兹系统的动态行为。 

系统特点：

1. 敏感依赖于初始条件： 洛伦兹系统展现出敏感依赖于初始条件的特点，即微小的初始条件变化可能导致长时间内的轨迹发生巨大的分离。
2. 奇异吸引子： 洛伦兹系统的相空间中，轨迹围绕着一个被称为“奇异吸引子”的复杂结构。这个吸引子是一个分形结构，展现了无规则且不可重复的形态。
3. 周期性和混沌性共存： 洛伦兹系统在参数空间内可以存在周期性行为和混沌行为，这种现象被称为“周期倍增路线到混沌”。

       混沌的产生原理： 洛伦兹混沌系统的混沌行为来源于非线性项的存在。当参数取值在一定范围内，非线性项的影响会导致相空间中的轨迹错综复杂地交织在一起，这使得系统的演化变得高度不可预测。

        洛伦兹混沌系统是混沌现象的经典范例，它揭示了非线性系统的复杂行为和对初始条件的敏感性。通过简单的数学方程，洛伦兹系统展现出了无法预测的、高度不稳定的轨迹，这一发现在混沌理论的发展中具有重要地位，深刻影响了许多领域，包括天气预测、物理学、生物学等。

5.算法完整程序工程

OOOOO

OOO

O