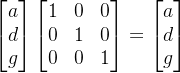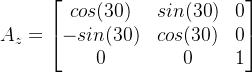- 火爆全网的条形竞赛图,Python轻松实现
统计学家
image这个动图叫条形竞赛图,非常适合制作随时间变动的数据。我已经用streamlit+bar_chart_race实现了,然后白嫖了heroku的服务器,大家通过下面的网址上传csv格式的表格就可以轻松制作条形竞赛图,生成的视频可以保存本地。https://bar-chart-race-app.herokuapp.com/本文我将实现过程介绍一下,白嫖服务器+部署留在下期再讲。纯matplot
- 【无标题】Python---day9 模块化编程概念(模块、包、导入)及常见系统模块总结和第三方模块管理
AnAn__kang
pythonjava服务器
系列文章目录前言跟着博主学Python,今天我们来到了第九天的学习,模块化编程的概念。Python作为一门编程语言,本身就是用于对模块以及各种包的使用来达到我们自己想到创作的目的。所以今天博主就给大家盘点一下有关于各种常见的包以及如何进行导入的。一.模块Module,模块1.1基本概念定义:模块是一个Python文件,每个.py.py.py文件就是一个模块。作用:用于组织代码,避免代码重复,提高复
- Python --- day 10 Opencv模块的使用
AnAn__kang
pythonopencv开发语言
系列文章目录前言今天博主带大家进入Opencv的学习,这是一个专门针对处理图像和视频的一个模块,大家以理解为主,增强自己的编程思维,再后续我们训练模型时会大批量的处理图片时会经常用到这个模块。1OpenCV介绍OpenCV(开放源代码计算机视觉库)是一个开源的计算机视觉和机器学习软件库。由一系列C++类和函数构成,用于图像处理、计算机视觉领域的算法实现。1.1OpenCV优势**开源免费:**完全
- 【无标题】Python --- Day5 函数的位置传参、关键词传参及其可变性和解包操作
AnAn__kang
python前端人工智能
系列文章目录前言今天小伙伴们跟我进入第五天的Python课程学习,主要是关于函数的位置传参,关键传参和可变性和解包传参这其中的具体定义以及它们的使用场景`一、调用传参函数调用时传递参数的方式有多种,包括位置传参、关键词传参、多个参数解包、参数默认值等。1.1位置传参最常见的传参方式,参数按定义的顺序依次传入函数。示例:defgreet(name,age):print(f"Hello,{name}.
- 时序数据库在数据库领域的行业应用
数据库管理艺术
数据库时序数据库ai
时序数据库在数据库领域的行业应用关键词:时序数据库、数据库领域、行业应用、时间序列数据、实时分析摘要:本文深入探讨了时序数据库在数据库领域的行业应用。首先介绍了时序数据库的背景知识,包括其目的、适用读者、文档结构和相关术语。接着阐述了时序数据库的核心概念、架构和工作原理,通过Python代码详细讲解了核心算法。还介绍了相关的数学模型和公式,并举例说明。在项目实战部分,给出了开发环境搭建、源代码实现
- Python --- Day3 推导式及 常见语句和内置函数的学习!!!
系列文章目录前言相信各位伙伴们在前俩次的文章和Python的基础学习中大有收获,这次我们将进入推导式,常见语句和内置函数的学习!跟着博主一起成为一名Ai的算法工程师!一、推导式用更简洁的方式创建列表、字典和集合。是Python特有的一种表达式形式。1.1列表推导式a=[1,2,3,4]result=[x*2forxina]#创建一个新列表,元素是原列表每个元素的两倍1.2字典推导式a=['a','
- cuda编程python接口_使用Python写CUDA程序的方法
weixin_39822184
cuda编程python接口
使用Python写CUDA程序有两种方式:*Numba*PyCUDAnumbapro现在已经不推荐使用了,功能被拆分并分别被集成到accelerate和Numba了。例子numbaNumba通过及时编译机制(JIT)优化Python代码,Numba可以针对本机的硬件环境进行优化,同时支持CPU和GPU的优化,并且可以和Numpy集成,使Python代码可以在GPU上运行,只需在函数上方加上相关的指
- 基于 Python 的网站信息探测工具设计与实现
计算机毕业设计指导
python网络服务器
基于Python的网站信息探测工具设计与实现摘要在渗透测试与网络安全评估中,信息探测是最基础且关键的一步。通过对目标网站的操作系统、服务器、CMS、端口、目录结构等信息进行自动化探测,可为后续攻击路径识别提供基础数据支撑。传统工具如WhatWeb、FOFA等虽功能强大,但在定制化与扩展性方面受限。本文设计并实现了一款基于Python的轻量级网站信息探测工具,支持URL/IP扫描、开放端口探测、CM
- 使用CrewAI创建一个研究团队
AI量化投资
php开发语言多智能体智能体人工智能
本指导文档将带你一步步完成使用CrewAI框架创建你的第一个AI代理团队的过程。通过这个简单的示例,你将学习如何构建一个研究团队,用于研究和分析指定主题,并生成一份综合报告。本教程基于CrewAI官方文档,适合初学者快速上手。前提条件在开始之前,请确保你已完成以下准备工作:安装Python:确保你的系统安装了Python版本在3.10到3.13之间。你可以通过以下命令检查Python版本:pyth
- Python成第四个支持CUDA的编程语言
Python成第四个支持CUDA的编程语言3月19日NVIDIA的GTC2013图形技术大会将开幕,在此之前会有很多宣传造势内容,其中最重大也是最主要的就是NVIDIA老总黄仁勋的开幕词了,其他合作伙伴也会发布各自的演讲。ContinuumAnalytics联合NVIDIA宣布将会引入新的PythonCUDA编译器——NumbaPro,Python也成为继C、C++以及Fortan之后的第四个支持
- Python FastMCP:让你的AI工具链飞起来
PythonFastMCP:让你的AI工具链飞起来FastMCPFastMCP是什么?1.工具(Tools):赋予LLM执行能力2.Resources(资源):安全数据通道3.Prompts(提示模板):标准化LLM交互4.组件协同:构建项目AI工具链5.部署架构与性能优化博主热门文章推荐:官方文档:FastMCP官方文档:https://gofastmcp.com/MCP协议规范:https:/
- Python 解析 PDF 文件的基础方法
电脑维修员xy
pythonpdf前端
```htmlPython解析PDF文件的基础方法Python解析PDF文件的基础方法在现代数据处理和信息提取任务中,PDF文件是一种常见的文档格式。然而,PDF文件的结构复杂且难以直接解析,尤其是当需要从中提取文本或数据时。幸运的是,Python提供了多种强大的库来帮助我们轻松地解析PDF文件。1.PyPDF2库PyPDF2是一个功能强大的Python库,用于处理PDF文件。它可以读取、分割、合
- socket网络通信TCP与UDP原理及代码实现(c++、python)
目录Socket原理通信协议原理TCPUDP代码实现TCPC++pythonUDPC++pythonSocket原理Socket(套接字)是计算机网络中用于实现进程间通信的一种机制,特别是在不同主机之间通过网络进行数据传输时。它是网络编程的核心概念之一,为应用程序提供了统一的接口,使得开发者可以通过网络发送和接收数据。可以将Socket类比为电话系统中的“电话机”。两台设备通过Socket建立连接
- 【Qt Designer使用快捷键】
QtDesigner简介QtDesigner是Qt框架提供的可视化界面设计工具,用于快速创建GUI(图形用户界面)。用户可通过拖拽控件(如按钮、文本框等)设计界面,无需手动编写布局代码。生成的界面文件(.ui)可通过pyuic或uic工具转换为代码(如Python或C++),与业务逻辑集成。常用快捷键及用途通用操作Ctrl+N:新建界面文件。Ctrl+O:打开现有.ui文件。Ctrl+S:保存当前
- “重复”定义函数的睿智(Python/与ai助手“智普清言”深度交流)
梦幻精灵_cq
笔记学习
镜像双胞谬重复,定制便捷巧活工。 笔记模板由python脚本于2025-07-1612:16:30创建,本篇笔记适合至少通晓一门语言,熟悉基本编程范式的coder翻阅。学习的细节是欢悦的历程 博客的核心价值:在于输出思考与经验,而不仅仅是知识的简单复述。Python官网: 这里,才python前沿。英文原版,原汁原味,才是寻根溯源的正统。地址:https://www.python.org/F
- 偶拾《退让》,一阙仿七律带出的文化思考(中文诗创作)
梦幻精灵_cq
笔记学习
礼貌温言沐春风,谦让理解通彼此。 笔记模板由python脚本于2025-07-0111:29:03创建,本篇笔记适合喜欢中文仿古七言诗的coder翻阅。学习的细节是欢悦的历程 博客的核心价值:在于输出思考与经验,而不仅仅是知识的简单复述。Python官网: 这里,才python前沿。英文原版,原汁原味,才是寻根溯源的正统。地址:https://www.python.org/Free: 大咖
- 008、Python+fastapi,第一个后台管理项目走向第8步:ubutun 20.04下配置远程桌面、安装vscode+python环境配置
浪淘沙jkp
学习fastapi
一、说明白飘了3个月无影云电脑,开始选了个windowsserver非常不好用,后台改为ubuntu想升级到22,没成功,那就20.04吧。今天先安装下开发环境,后续2个月就想把他当做开发服务器,不知道行不行,公网ip是否可以外部链接。本来想装个宝塔面板直接管理,不过那玩意用了一次,决定说方便也不方便,还是放弃,要用也搞个掏钱的,你懂的,免费的不放心啊那我们就一个一个安装好了,大概要安装mysql
- python爬大学生就业信息报告_Python语言爬虫——Python 岗位分析报告
weixin_39578457
本文主要向大家介绍了Python语言爬虫——Python岗位分析报告,通过具体的内容向大家展示,希望对大家学习Python语言有所帮助。前两篇我们分别爬取了糗事百科和妹子图网站,学习了Requests,BeautifulSoup的基本使用。不过前两篇都是从静态HTML页面中来筛选出我们需要的信息。这一篇我们来学习下如何来获取Ajax请求返回的结果。本篇以拉勾网为例来说明一下如何获取Ajax请求内容
- 快速入门Robocorp:用Python构建和操作工作流
jaioyfpo
python开发语言
快速入门Robocorp:用Python构建和操作工作流引言在现代开发环境中,自动化是提高效率和降低成本的关键。Robocorp作为一个强大的平台,它帮助您使用Python构建和操作工作流,无论在何地运行都可以保持无缝连接和高扩展性。本文将带领您快速入门Robocorp的基本安装和设置,并展示如何使用ActionServer进行项目的创建和管理。主要内容1.安装和设置要开始使用Robocorp,首
- Python关于pandas的基础知识
WeiJingYu.
pythonpandas开发语言
一.扫盲(一)、pandas是什么pandas是Python的一个第三方数据处理库,它提供了高效、灵活的数据结构(如Series和DataFrame),能方便地对结构化数据进行清洗、转换、分析和处理。(二)、pandas与NumPy的关系NumPy是Python中用于科学计算的基础库,主要用于存储和处理数值型数组。但它有一个局限,就是不能直接存储和处理字符串等非数值类型的数据。而pandas是在N
- Python 爬虫——Pyppeteer
Python爬虫——PyppeteerPythonSpider——Pyppeteer一、爬虫的两种方式二、Pyppeteer三、爬虫实现PythonSpider——Pyppeteer爬虫具有时效性,该文产生于2023年末一、爬虫的两种方式爬虫大致可以分为两类方式:直接请求直接请求的方式一般是使用python的HTTP请求库发起HTTP请求,然后接收返回的数据再进行解析,这种方式存在很大的局限性。当
- Python关于numpy的基础知识数组的升维
WeiJingYu.
pythonnumpy开发语言
在Python数据处理中,numpy是常用的科学计算库,数组操作是其核心内容之一。下面通过代码示例,展示如何从Python自带列表构建numpy一维数组,再进一步升维构建二维数组。\importnumpyasnp#一维数组构建:从Python列表到numpy一维数组list1=[1,2,3,4,5]#Python自带的列表数据类型print("Python列表list1:",list1)v=np.
- Selenium Python 代码之打开网页自动填充内容并搜索
iCloudEnd
SeleniumPython代码之打开网页自动填充内容并搜索流程通过id找到文本框inputElement.send_keys(Keys.BACK_SPACE)发送删除键,清除一下之前文字inputElement.send_keys(Keys.BACK_SPACE)发送需要查询对内容并送个回车inputElement=driver1.find_element_by_id("TextBox1")in
- python双引号打不出来_在python 3中使用单引号和双引号时出错 - python
weixin_39897749
python双引号打不出来
使用os.system()函数时,我在python中遇到了EOL错误。以下是代码行生成错误:os.system("catsubdomains.txt|cut-d'"'-f1")基本上,我试图使用分号[“]修改输出字符串(双引号)参考方案如果需要在带"的字符串中编写",则可以将其写为\""catsubdomains.txt|cut-d'\"'-f1"在PythonCloudFunction中使用错误
- python办自动化--读取邮箱中特定的邮件,并下载特定的附件
宝山哥哥
python办公自动化python自动化信息可视化
系列文章目录python办公自动化–数据可视化(pandas+matplotlib)–生成条形图和饼状图python办公自动化–数据可视化(pandas+matplotlib)–生成折线图python办公自动化–数据可视化(pandas读取excel文件,matplotlib生成可视化图表)python办公自动化-openpyxl学习-工资表生成工资条python办公自动化–使用将csv大文件分割
- Here-Document的`<<` 与 `<<-` 与 `<<<` 多解说笔记250722
kfepiza
#Linux#控制台命令行Shellbashcmd等笔记linuxbash
Here-Document的poem.txt静夜思床前明月光疑是地上霜FORMATTED#2.空格敏感的配置catconfig.ymlindentation:level:4#必须4空格SPACE何时用tabs.txt重要制表符:→这里Tab会被保留但行首Tab会被移除TABS#2.空格缩进的环境#(如Python脚本)技术原理图解HereDocumentquery.sqlSELECT*FROM${
- 第二阶段-第二章—8天Python从入门到精通【itheima】-133节(SQL——DQL——基础查询)
Patrick_kafka
sqlpython数据库开发语言学习android程序人生
目录133节——DQL:基础查询1.学习目标2.基础数据查询:select3.进行过滤的基础数据查询:where4.代码演练5.小节总结6.关于MySQL和SQL的DDL、DML、DCL、DQL的最底层逻辑MySQL与SQL的底层逻辑:从磁盘到内存的数据流解析一、DDL(数据定义语言):构建数据大厦的蓝图二、DML(数据操作语言):数据流动的三重关卡三、DCL(数据控制语言):权限的多维管控四、D
- Python爬虫教程:抓取地方政府网站的公开文件与政策信息
Python爬虫项目
python爬虫开发语言数据分析mysql
1.引言在信息化时代,政府网站已成为信息公开的重要渠道。各级地方政府网站上发布的政策、公告和公开文件,通常包含了政府决策、法律法规等关键信息。爬取这些公开数据,可以为研究人员、政策分析师、企业决策者等提供有价值的数据支持。本文将通过Python爬虫技术,展示如何抓取地方政府网站上的公开文件、政策等信息。我们将使用最新的爬虫技术,如requests、BeautifulSoup、Selenium等工具
- Python 库手册:xml.etree.ElementTree 处理 XML 数据模块
xml.etree.ElementTree(简称ElementTree)是Python标准库中用于解析、创建和操作XML数据的模块。它提供了一种轻量、易用的方式来读取、修改和写入XML文件,适用于配置文件处理、数据交换、网络通信等应用场景。常见应用场景:(1)读取XML配置文件并提取参数。(2)修改XML数据结构(如节点属性、内容)。(3)创建新的XML文档并保存。(4)从WebAPI获取的XML
- [学习] 笛卡尔坐标系的任意移动与旋转详解
极客不孤独
学习算法信号处理
笛卡尔坐标系的任意移动与旋转详解文章目录笛卡尔坐标系的任意移动与旋转详解**1.笛卡尔坐标系基础****2.坐标变换原理****2.1平移变换****2.2旋转变换****3.组合变换**Python仿真与动态展示**动画说明**:**关键数学原理**:1.笛卡尔坐标系基础笛卡尔坐标系用(x,y)(x,y)(x,y)表示平面内任意点的位置,原点为(0,0)(0,0)(0,0)。几何图形可视为点的集
- 微信开发者验证接口开发
362217990
微信 开发者 token 验证
微信开发者接口验证。
Token,自己随便定义,与微信填写一致就可以了。
根据微信接入指南描述 http://mp.weixin.qq.com/wiki/17/2d4265491f12608cd170a95559800f2d.html
第一步:填写服务器配置
第二步:验证服务器地址的有效性
第三步:依据接口文档实现业务逻辑
这里主要讲第二步验证服务器有效性。
建一个
- 一个小编程题-类似约瑟夫环问题
BrokenDreams
编程
今天群友出了一题:
一个数列,把第一个元素删除,然后把第二个元素放到数列的最后,依次操作下去,直到把数列中所有的数都删除,要求依次打印出这个过程中删除的数。
&
- linux复习笔记之bash shell (5) 关于减号-的作用
eksliang
linux关于减号“-”的含义linux关于减号“-”的用途linux关于“-”的含义linux关于减号的含义
转载请出自出处:
http://eksliang.iteye.com/blog/2105677
管道命令在bash的连续处理程序中是相当重要的,尤其在使用到前一个命令的studout(标准输出)作为这次的stdin(标准输入)时,就显得太重要了,某些命令需要用到文件名,例如上篇文档的的切割命令(split)、还有
- Unix(3)
18289753290
unix ksh
1)若该变量需要在其他子进程执行,则可用"$变量名称"或${变量}累加内容
什么是子进程?在我目前这个shell情况下,去打开一个新的shell,新的那个shell就是子进程。一般状态下,父进程的自定义变量是无法在子进程内使用的,但通过export将变量变成环境变量后就能够在子进程里面应用了。
2)条件判断: &&代表and ||代表or&nbs
- 关于ListView中性能优化中图片加载问题
酷的飞上天空
ListView
ListView的性能优化网上很多信息,但是涉及到异步加载图片问题就会出现问题。
具体参看上篇文章http://314858770.iteye.com/admin/blogs/1217594
如果每次都重新inflate一个新的View出来肯定会造成性能损失严重,可能会出现listview滚动是很卡的情况,还会出现内存溢出。
现在想出一个方法就是每次都添加一个标识,然后设置图
- 德国总理默多克:给国人的一堂“震撼教育”课
永夜-极光
教育
http://bbs.voc.com.cn/topic-2443617-1-1.html德国总理默多克:给国人的一堂“震撼教育”课
安吉拉—默克尔,一位经历过社会主义的东德人,她利用自己的博客,发表一番来华前的谈话,该说的话,都在上面说了,全世界想看想传播——去看看默克尔总理的博客吧!
德国总理默克尔以她的低调、朴素、谦和、平易近人等品格给国人留下了深刻印象。她以实际行动为中国人上了一堂
- 关于Java继承的一个小问题。。。
随便小屋
java
今天看Java 编程思想的时候遇见一个问题,运行的结果和自己想想的完全不一样。先把代码贴出来!
//CanFight接口
interface Canfight {
void fight();
}
//ActionCharacter类
class ActionCharacter {
public void fight() {
System.out.pr
- 23种基本的设计模式
aijuans
设计模式
Abstract Factory:提供一个创建一系列相关或相互依赖对象的接口,而无需指定它们具体的类。 Adapter:将一个类的接口转换成客户希望的另外一个接口。A d a p t e r模式使得原本由于接口不兼容而不能一起工作的那些类可以一起工作。 Bridge:将抽象部分与它的实现部分分离,使它们都可以独立地变化。 Builder:将一个复杂对象的构建与它的表示分离,使得同
- 《周鸿祎自述:我的互联网方法论》读书笔记
aoyouzi
读书笔记
从用户的角度来看,能解决问题的产品才是好产品,能方便/快速地解决问题的产品,就是一流产品.
商业模式不是赚钱模式
一款产品免费获得海量用户后,它的边际成本趋于0,然后再通过广告或者增值服务的方式赚钱,实际上就是创造了新的价值链.
商业模式的基础是用户,木有用户,任何商业模式都是浮云.商业模式的核心是产品,本质是通过产品为用户创造价值.
商业模式还包括寻找需求
- JavaScript动态改变样式访问技术
百合不是茶
JavaScriptstyle属性ClassName属性
一:style属性
格式:
HTML元素.style.样式属性="值";
创建菜单:在html标签中创建 或者 在head标签中用数组创建
<html>
<head>
<title>style改变样式</title>
</head>
&l
- jQuery的deferred对象详解
bijian1013
jquerydeferred对象
jQuery的开发速度很快,几乎每半年一个大版本,每两个月一个小版本。
每个版本都会引入一些新功能,从jQuery 1.5.0版本开始引入的一个新功能----deferred对象。
&nb
- 淘宝开放平台TOP
Bill_chen
C++c物流C#
淘宝网开放平台首页:http://open.taobao.com/
淘宝开放平台是淘宝TOP团队的产品,TOP即TaoBao Open Platform,
是淘宝合作伙伴开发、发布、交易其服务的平台。
支撑TOP的三条主线为:
1.开放数据和业务流程
* 以API数据形式开放商品、交易、物流等业务;
&
- 【大型网站架构一】大型网站架构概述
bit1129
网站架构
大型互联网特点
面对海量用户、海量数据
大型互联网架构的关键指标
高并发
高性能
高可用
高可扩展性
线性伸缩性
安全性
大型互联网技术要点
前端优化
CDN缓存
反向代理
KV缓存
消息系统
分布式存储
NoSQL数据库
搜索
监控
安全
想到的问题:
1.对于订单系统这种事务型系统,如
- eclipse插件hibernate tools安装
白糖_
Hibernate
eclipse helios(3.6)版
1.启动eclipse 2.选择 Help > Install New Software...> 3.添加如下地址:
http://download.jboss.org/jbosstools/updates/stable/helios/ 4.选择性安装:hibernate tools在All Jboss tool
- Jquery easyui Form表单提交注意事项
bozch
jquery easyui
jquery easyui对表单的提交进行了封装,提交的方式采用的是ajax的方式,在开发的时候应该注意的事项如下:
1、在定义form标签的时候,要将method属性设置成post或者get,特别是进行大字段的文本信息提交的时候,要将method设置成post方式提交,否则页面会抛出跨域访问等异常。所以这个要
- Trie tree(字典树)的Java实现及其应用-统计以某字符串为前缀的单词的数量
bylijinnan
java实现
import java.util.LinkedList;
public class CaseInsensitiveTrie {
/**
字典树的Java实现。实现了插入、查询以及深度优先遍历。
Trie tree's java implementation.(Insert,Search,DFS)
Problem Description
Igna
- html css 鼠标形状样式汇总
chenbowen00
htmlcss
css鼠标手型cursor中hand与pointer
Example:CSS鼠标手型效果 <a href="#" style="cursor:hand">CSS鼠标手型效果</a><br/>
Example:CSS鼠标手型效果 <a href="#" style=&qu
- [IT与投资]IT投资的几个原则
comsci
it
无论是想在电商,软件,硬件还是互联网领域投资,都需要大量资金,虽然各个国家政府在媒体上都给予大家承诺,既要让市场的流动性宽松,又要保持经济的高速增长....但是,事实上,整个市场和社会对于真正的资金投入是非常渴望的,也就是说,表面上看起来,市场很活跃,但是投入的资金并不是很充足的......
- oracle with语句详解
daizj
oraclewithwith as
oracle with语句详解 转
在oracle中,select 查询语句,可以使用with,就是一个子查询,oracle 会把子查询的结果放到临时表中,可以反复使用
例子:注意,这是sql语句,不是pl/sql语句, 可以直接放到jdbc执行的
----------------------------------------------------------------
- hbase的简单操作
deng520159
数据库hbase
近期公司用hbase来存储日志,然后再来分析 ,把hbase开发经常要用的命令找了出来.
用ssh登陆安装hbase那台linux后
用hbase shell进行hbase命令控制台!
表的管理
1)查看有哪些表
hbase(main)> list
2)创建表
# 语法:create <table>, {NAME => <family&g
- C语言scanf继续学习、算术运算符学习和逻辑运算符
dcj3sjt126com
c
/*
2013年3月11日20:37:32
地点:北京潘家园
功能:完成用户格式化输入多个值
目的:学习scanf函数的使用
*/
# include <stdio.h>
int main(void)
{
int i, j, k;
printf("please input three number:\n"); //提示用
- 2015越来越好
dcj3sjt126com
歌曲
越来越好
房子大了电话小了 感觉越来越好
假期多了收入高了 工作越来越好
商品精了价格活了 心情越来越好
天更蓝了水更清了 环境越来越好
活得有奔头人会步步高
想做到你要努力去做到
幸福的笑容天天挂眉梢 越来越好
婆媳和了家庭暖了 生活越来越好
孩子高了懂事多了 学习越来越好
朋友多了心相通了 大家越来越好
道路宽了心气顺了 日子越来越好
活的有精神人就不显
- java.sql.SQLException: Value '0000-00-00' can not be represented as java.sql.Tim
feiteyizu
mysql
数据表中有记录的time字段(属性为timestamp)其值为:“0000-00-00 00:00:00”
程序使用select 语句从中取数据时出现以下异常:
java.sql.SQLException:Value '0000-00-00' can not be represented as java.sql.Date
java.sql.SQLException: Valu
- Ehcache(07)——Ehcache对并发的支持
234390216
并发ehcache锁ReadLockWriteLock
Ehcache对并发的支持
在高并发的情况下,使用Ehcache缓存时,由于并发的读与写,我们读的数据有可能是错误的,我们写的数据也有可能意外的被覆盖。所幸的是Ehcache为我们提供了针对于缓存元素Key的Read(读)、Write(写)锁。当一个线程获取了某一Key的Read锁之后,其它线程获取针对于同
- mysql中blob,text字段的合成索引
jackyrong
mysql
在mysql中,原来有一个叫合成索引的,可以提高blob,text字段的效率性能,
但只能用在精确查询,核心是增加一个列,然后可以用md5进行散列,用散列值查找
则速度快
比如:
create table abc(id varchar(10),context blog,hash_value varchar(40));
insert into abc(1,rep
- 逻辑运算与移位运算
latty
位运算逻辑运算
源码:正数的补码与原码相同例+7 源码:00000111 补码 :00000111 (用8位二进制表示一个数)
负数的补码:
符号位为1,其余位为该数绝对值的原码按位取反;然后整个数加1。 -7 源码: 10000111 ,其绝对值为00000111 取反加一:11111001 为-7补码
已知一个数的补码,求原码的操作分两种情况:
- 利用XSD 验证XML文件
newerdragon
javaxmlxsd
XSD文件 (XML Schema 语言也称作 XML Schema 定义(XML Schema Definition,XSD)。 具体使用方法和定义请参看:
http://www.w3school.com.cn/schema/index.asp
java自jdk1.5以上新增了SchemaFactory类 可以实现对XSD验证的支持,使用起来也很方便。
以下代码可用在J
- 搭建 CentOS 6 服务器(12) - Samba
rensanning
centos
(1)安装
# yum -y install samba
Installed:
samba.i686 0:3.6.9-169.el6_5
# pdbedit -a rensn
new password:123456
retype new password:123456
……
(2)Home文件夹
# mkdir /etc
- Learn Nodejs 01
toknowme
nodejs
(1)下载nodejs
https://nodejs.org/download/ 选择相应的版本进行下载 (2)安装nodejs 安装的方式比较多,请baidu下
我这边下载的是“node-v0.12.7-linux-x64.tar.gz”这个版本 (1)上传服务器 (2)解压 tar -zxvf node-v0.12.
- jquery控制自动刷新的代码举例
xp9802
jquery
1、html内容部分 复制代码代码示例: <div id='log_reload'>
<select name="id_s" size="1">
<option value='2'>-2s-</option>
<option value='3'>-3s-</option

![]()
![]()
![]()
![]() 轴,
轴,![]()
![]() 轴,
轴,![]() 代表
代表![]() 轴。
轴。![]() 矩阵
矩阵![]() 就很简单了(
就很简单了(![]() 代表旋转)
代表旋转)![]() 旋转
旋转![]() 旋转
旋转![]() 旋转
旋转![]()
![]()
![]()
![]()
![]()
![]()
![]() 轴旋转了
轴旋转了![]() °的坐标系。按照上面绕
°的坐标系。按照上面绕![]() 轴旋转的矩阵公式可得到
轴旋转的矩阵公式可得到![]()
![]()
![]()
![]()
![]()
![]()