Shamir门限方案的秘钥分享（包括逆元求解）

Shamir门限方案的秘钥分享（不要求支持大数）

题目描述

【实验目的】

1. 通过基于Shamir门限方案的密钥分割及恢复的演示，理解密钥分割的重要性，理解密钥分割的基本原理和作用，掌握基于Shamir门限方案的密钥分割软件的使用

【实验原理】

秘密共享体制为将秘密分给多人掌管提供了可能。例如重要场所的通行、遗嘱的生效等都必须由两人或多人同时参与才能生效，这时都需要将秘密分给多人掌管并同时参与才能恢复。在实际应用中，秘密共享的要求多种多样、形形色色，每一种解决问题的方案都可成为一种体制。1979年，Shamir在提出秘密分享思想的同时，利用拉格朗日插值多项式理论设计出了一个具体的（t,n）秘密分享方案。1979年以后，人们通过对秘密共享问题的分析研究，构建出了许多类型的秘密共享体制。具有代表性和一般性的除了有Shamir提出的(t,n)门限体制，还有Blakley提出的矢量体制，Asmuth和Bloom提出的同余类体制，Karnin提出的矩阵法体制等。

一般地，一个由秘密分发者D和参与者P1，P2，…，Pn构成的（t,n）秘密分享体制包含下面两个协议：

（1） 秘密分发协议：在这个协议中，秘密分发者D在n个参与者中分享秘密s，每个参与者Pi获得一个碎片si，i=1,2，…，n。

（2） 秘密重构协议：在这个协议中，任意不少于t个参与者一起合作，以自己的碎片为输入，重构原秘密s。

一个安全的（t,n）秘密分享体制必须同时提供两个性质：一方面，任意t个参与者通过提供自己的碎片能够协作地恢复出原秘密s；另一方面，任意少于t个参与者即便拥有自己的碎片也无法计算关于原秘密s的任何信息。一般称这里的t为门限值。

Shamir提出的基于LaGrange插值公式的密钥分存思想是,利用有限域GF§上的t-1次多项式

h(x)= at- 1xt- 1+ … + a1x+ a0 mod p (1)

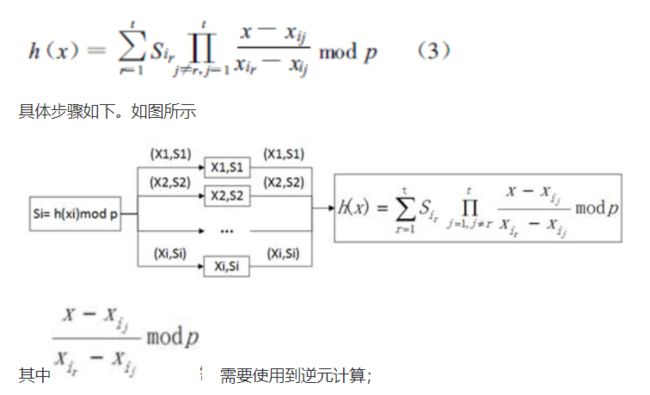
构造秘密共享的（t,n）门限体制。其中，所选的随机素数p要大于最大可能的秘密数S和参与者总数n，并且公开；S=h(0)=a0，而at-1，at-2，… ，a1为选用的随机系数，这些都需要保密，在生成n个秘密份额之后即可销毁。通过计算多项式h(x)对n个不同xi的取值就给出每个人的秘密份额:

Si= h(xi)mod p，i= 1,2,3,… ,n (2)

每一(xi,Si)对就是曲线h上的一个点，可看作是用户的标识符。由于任意t个点都可唯一地确定相应的t- 1次多项式，所以，秘密S可以从t个秘密份额重构。给定任意t个秘密份额Si1，Si2，… ，Sit，由LaGrange插值公式重构的多项式为

关于逆元，可以参看https://blog.csdn.net/wcxyky/article/details/103366577其中有些例子不够完善，请谨慎选取，小心验证。

具体例子：

n=5, t=3, p=17; 随机选取K=13,a1=10,a2=2;

于是有

输入

大素数p

子秘钥总数n

门限值t

输出

设置的密钥与恢复的密钥的差值

输入样例1

1006000813
10
3

输出样例1

0

AC代码

import java.util.*;

/**
 * @author xzx
 * @date 2022/10/29
 */
public class Main {

    static class Point {
        public long x;
        public long y;

        public Point(long x, long y) {
            this.x = x;
            this.y = y;
        }
    }

    private static long k;
    private static long a1;
    private static long a2;

    public static void main(String[] args) {
        //处理输入
        Scanner scanner = new Scanner(System.in);
        long p = scanner.nextInt();
        long n = scanner.nextInt();
        long t = scanner.nextInt();
        long key = calculate(p, n, t);
        //System.out.println("恢复的密钥："+key);
        long dis = key - k;
        System.out.println(dis);
    }

    /**
     * 推演
     *
     * @param prime     大素数p
     * @param total     子秘钥总数n
     * @param threshold 门限值t
     * @return
     */
    private static long calculate(long prime, long total, long threshold) {
        randomGenerateParams();
        List<Long> points = getTotalPoint(total, prime);
        List<Point> list = getThresholdPoint(prime, total, threshold, points);
        return getKey(list, prime);
    }

    /**
     * 恢复密钥
     *
     * @param list  选取的点
     * @param prime mod
     * @return
     */
    private static long getKey(List<Point> list, long prime) {
        long res = 0;
        for (int i = 0; i < list.size(); i++) {
            Point point = list.get(i);
            long val = point.y;
            for (int j = 0; j < list.size(); j++) {
                if (i == j) {
                    continue;
                }
                val *= list.get(j).x;
                val %= prime;
                long inv = getInv(point.x - list.get(j).x, prime);
                //System.out.println(point.x - list.get(j).x + "关于" + prime + "的逆元是：" + inv);
                val *= inv;
                val %= prime;
            }
            res += val;
            res %= prime;
        }
        return res;
    }

    /**
     * 求解逆元
     *
     * @param a
     * @param mod
     * @return
     */
    static long getInv(long a, long mod) {
        return qkpow(a, mod - 2, mod);
    }

    /**
     * 费马小定理/欧拉定理
     *
     * @param a
     * @param p
     * @param mod
     * @return
     */
    static long qkpow(long a, long p, long mod) {
        long t = 1, tt = a % mod;
        while (p != 0) {
            if ((p & 1) != 0) t = t * tt % mod;
            tt = tt * tt % mod;
            p >>= 1;
        }
        return t < 0 ? t + mod : t;
    }

    /**
     * 解密的时候随机选取t个点（不重复）
     *
     * @param prime
     * @param total
     * @param threshold
     * @param points
     * @return
     */
    private static List<Point> getThresholdPoint(long prime, long total, long threshold, List<Long> points) {
        boolean[] used = new boolean[(int) total + 1];
        Point[] list = new Point[(int) threshold];
        Random random = new Random();
        for (int i = 0; i < threshold; i++) {
            while (true) {
                int x = random.nextInt((int) total) + 1;
                if (!used[x]) {
                    used[x] = true;
                    list[i] = new Point(x, points.get(x));
                    break;
                }
            }
        }
        return Arrays.asList(list);
    }


    /**
     * 获取total个点
     *
     * @param total
     * @param prime
     * @return
     */
    private static List<Long> getTotalPoint(long total, long prime) {
        Long[] points = new Long[(int) total + 1];
        for (int i = 1; i <= total; i++) {
            long y = (k + a1 * i + a2 * i * i) % prime;
            //System.out.println("(x" + i + ", y" + i + ") = " + "(" + i + ", " + y + ")");
            points[i] = y;
        }
        return Arrays.asList(points);
    }

    /**
     * 随机选取K,a1,a2
     */
    private static void randomGenerateParams() {
        Random random = new Random();
        // 1-20
        k = random.nextInt(20) + 1;
        //System.out.println("设置的密钥: " + k);
        // 1-10
        a1 = random.nextInt(10) + 1;
        a2 = random.nextInt(2) + 1;
    }
}