代码随想录算法训练营第十六天 | 104.二叉树的最大深度 559.n叉树的最大深度,111.二叉树的最小深度,222.完全二叉树的节点个数
代码随想录算法训练营第十六天 | 104.二叉树的最大深度 559.n叉树的最大深度,111.二叉树的最小深度,222.完全二叉树的节点个数
- 104.二叉树的最大深度 559.n叉树的最大深度
-
- 递归法
- 迭代法
- :eyes:题目总结:eyes:
- 111.二叉树的最小深度
-
- :computer:递归法
- :computer:迭代法
- :eyes:题目总结:eyes:
- 222.完全二叉树的节点个数
-
- :computer:递归法
- :computer:迭代法
- :computer:根据完全二叉树定义写
- :balloon:心得收获
104.二叉树的最大深度 559.n叉树的最大深度
题目链接104.二叉树的最大深度
视频讲解
给定一个二叉树,找出其最大深度,二叉树的深度为根节点到最远叶子节点的最长路径上的节点数
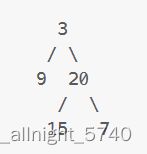
返回它的最大深度 3
本题可以使用前序(中左右),也可以使用后序遍历(左右中),使用前序求的就是深度,使用后序求的是高度
二叉树节点的深度:指从根节点到该节点的最长简单路径边的条数或者节点数(取决于深度从0开始还是从1开始)
二叉树节点的高度:指从该节点到叶子节点的最长简单路径边的条数或者节点数(取决于高度从0开始还是从1开始)
而根节点的高度就是二叉树的最大深度,所以本题中我们通过后序求的根节点高度来求的二叉树最大深度
递归法
后序
class solution {
public:
int getdepth(TreeNode* node) {
if (node == NULL) return 0;
int leftdepth = getdepth(node->left); // 左
int rightdepth = getdepth(node->right); // 右
int depth = 1 + max(leftdepth, rightdepth); // 中
return depth;
}
int maxDepth(TreeNode* root) {
return getdepth(root);
}
};
前序
class solution {
public:
int result;
void getdepth(TreeNode* node, int depth) {
result = depth > result ? depth : result; // 中
if (node->left == NULL && node->right == NULL) return ;
if (node->left) { // 左
depth++; // 深度+1
getdepth(node->left, depth);
depth--; // 回溯,深度-1
}
if (node->right) { // 右
depth++; // 深度+1
getdepth(node->right, depth);
depth--; // 回溯,深度-1
}
return ;
}
int maxDepth(TreeNode* root) {
result = 0;
if (root == NULL) return result;
getdepth(root, 1);
return result;
}
};
迭代法
使用迭代法的话,使用层序遍历是最为合适的,因为最大的深度就是二叉树的层数,和层序遍历的方式极其吻合
class solution {
public:
int maxDepth(TreeNode* root) {
if (root == NULL) return 0;
int depth = 0;
queue<TreeNode*> que;
que.push(root);
while(!que.empty()) {
int size = que.size();
depth++; // 记录深度
for (int i = 0; i < size; i++) {
TreeNode* node = que.front();
que.pop();
if (node->left) que.push(node->left);
if (node->right) que.push(node->right);
}
}
return depth;
}
};
题目链接559.n叉树的最大深度
给定一个 n 叉树,找到其最大深度,最大深度是指从根节点到最远叶子节点的最长路径上的节点总数

递归法
class solution {
public:
int maxDepth(Node* root) {
if (root == 0) return 0;
int depth = 0;
for (int i = 0; i < root->children.size(); i++) {
depth = max (depth, maxDepth(root->children[i]));
}
return depth + 1;
}
};
迭代法
class solution {
public:
int maxDepth(Node* root) {
queue<Node*> que;
if (root != NULL) que.push(root);
int depth = 0;
while (!que.empty()) {
int size = que.size();
depth++; // 记录深度
for (int i = 0; i < size; i++) {
Node* node = que.front();
que.pop();
for (int j = 0; j < node->children.size(); j++) {
if (node->children[j]) que.push(node->children[j]);
}
}
}
return depth;
}
};
题目总结
根节点的高度就是二叉树的最大深度,所以本题中我们通过后序求的根节点高度来求的二叉树最大深度
111.二叉树的最小深度
题目链接
视频链接
给定一个二叉树,找出其最小深度,最小深度是从根节点到最近叶子节点的最短路径上的节点数量

递归法
class Solution {
public:
int getDepth(TreeNode* node) {
if (node == NULL) return 0;
int leftDepth = getDepth(node->left); // 左
int rightDepth = getDepth(node->right); // 右
// 中
// 当一个左子树为空,右不为空,这时并不是最低点
if (node->left == NULL && node->right != NULL) {
return 1 + rightDepth;
}
// 当一个右子树为空,左不为空,这时并不是最低点
if (node->left != NULL && node->right == NULL) {
return 1 + leftDepth;
}
int result = 1 + min(leftDepth, rightDepth);
return result;
}
int minDepth(TreeNode* root) {
return getDepth(root);
}
};
迭代法
class Solution {
public:
int minDepth(TreeNode* root) {
if (root == NULL) return 0;
int depth = 0;
queue<TreeNode*> que;
que.push(root);
while(!que.empty()) {
int size = que.size();
depth++; // 记录最小深度
for (int i = 0; i < size; i++) {
TreeNode* node = que.front();
que.pop();
if (node->left) que.push(node->left);
if (node->right) que.push(node->right);
if (!node->left && !node->right) { // 当左右孩子都为空的时候,说明是最低点的一层了,退出
return depth;
}
}
}
return depth;
}
};
题目总结
一定要看清题目描述
222.完全二叉树的节点个数
题目链接
视频讲解
给你一棵 完全二叉树 的根节点 root ,求出该树的节点个数
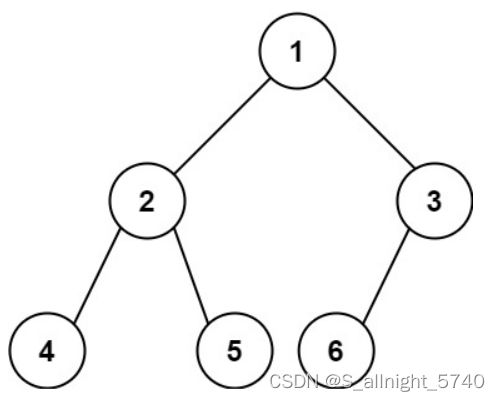
输入:root = [1,2,3,4,5,6]
输出:6
递归法
class Solution {
private:
int getNodesNum(TreeNode* cur) {
if (cur == NULL) return 0;
int leftNum = getNodesNum(cur->left); // 左
int rightNum = getNodesNum(cur->right); // 右
int treeNum = leftNum + rightNum + 1; // 中
return treeNum;
}
public:
int countNodes(TreeNode* root) {
return getNodesNum(root);
}
};
迭代法
class Solution {
public:
int countNodes(TreeNode* root) {
queue<TreeNode*> que;
if (root != NULL) que.push(root);
int result = 0;
while (!que.empty()) {
int size = que.size();
for (int i = 0; i < size; i++) {
TreeNode* node = que.front();
que.pop();
result++; // 记录节点数量
if (node->left) que.push(node->left);
if (node->right) que.push(node->right);
}
}
return result;
}
};
根据完全二叉树定义写
class Solution {
public:
int countNodes(TreeNode* root) {
if (root == nullptr) return 0;
TreeNode* left = root->left;
TreeNode* right = root->right;
int leftDepth = 0, rightDepth = 0; // 这里初始为0是有目的的,为了下面求指数方便
while (left) { // 求左子树深度
left = left->left;
leftDepth++;
}
while (right) { // 求右子树深度
right = right->right;
rightDepth++;
}
if (leftDepth == rightDepth) {
return (2 << leftDepth) - 1; // 注意(2<<1) 相当于2^2,所以leftDepth初始为0
}
return countNodes(root->left) + countNodes(root->right) + 1;
}
};
心得收获
理解二叉树高度和深度的定义,理解前序和后序遍历的区别,以及完全二叉树的定义