怒刷LeetCode的第11天(Java版)
目录
第一题
题目来源
题目内容
解决方法
方法一:迭代
方法二:递归
方法三:指针转向
第二题
题目来源
题目内容
解决方法
方法一:快慢指针
方法二:Arrays类的sort方法
方法三:计数器
方法四:额外的数组
第三题
题目来源
题目内容
解决方法
方法一:双指针
方法二:List
方法三:交换元素
方法四:递归
第一题
题目来源
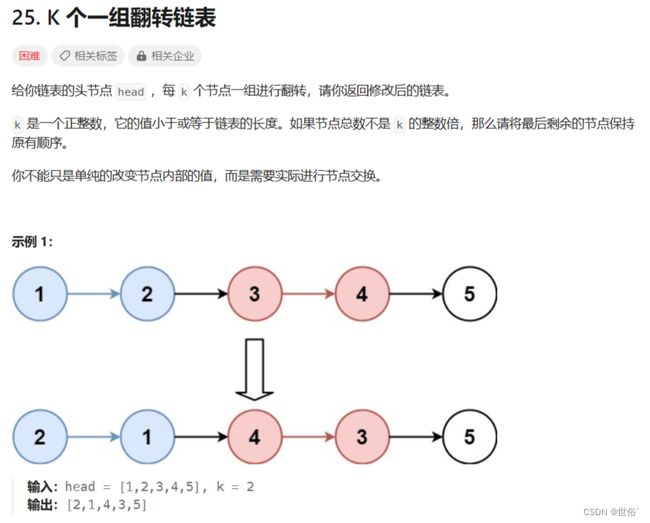
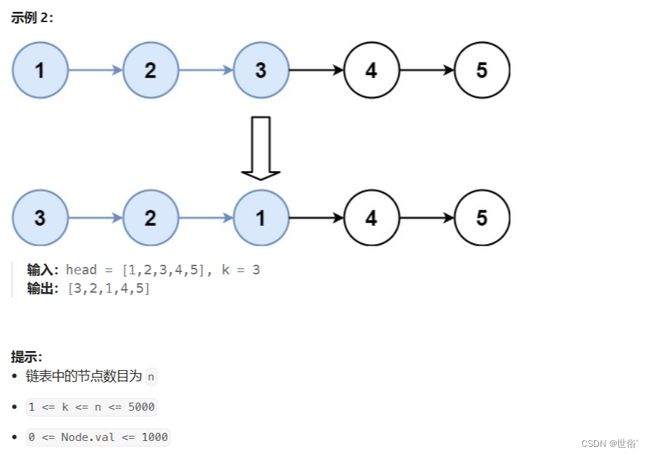
25. K 个一组翻转链表 - 力扣(LeetCode)
题目内容
解决方法
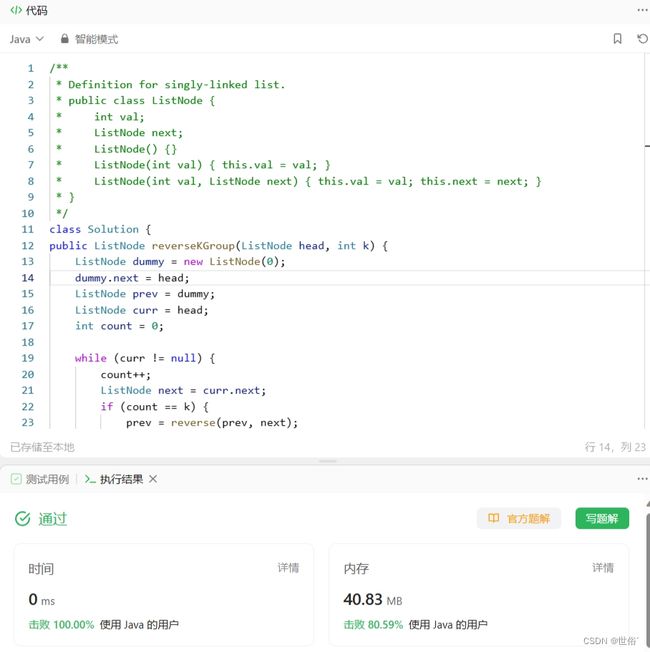
方法一:迭代
题目描述中要求将链表每 k 个节点作为一组进行翻转。这是一个比较经典的链表问题,可以使用迭代的方法来解决。具体思路如下:
- 首先定义两个指针 prev 和 curr,分别指向当前组的前一个节点和当前节点。
- 使用一个计数器 count 来记录当前组中已经遍历的节点数目。
- 遍历链表,对于每个节点 curr:
- 将 curr 的下一个节点保存到 next。
- 如果 count 等于 k,则说明当前组内的节点已经遍历完毕,需要进行翻转。具体操作如下:
- 调用 reverse 函数对 prev 和 curr 之间的节点进行翻转,返回翻转后的头节点,并将它赋值给新的 prev。
- 将翻转后的最后一个节点的 next 指向 next,即连接下一组的头节点。
- 更新 curr 和 prev,将它们都指向 next。
- 将 count 重置为 0,表示下一组的遍历开始。
- 如果 count 不等于 k,则说明当前组内的节点还未满 k 个,继续遍历下一个节点。
- 将 curr 更新为 next,继续遍历下一个节点。
- 将 count 增加 1。
- 返回翻转后的链表头节点。
/**
* Definition for singly-linked list.
* public class ListNode {
* int val;
* ListNode next;
* ListNode() {}
* ListNode(int val) { this.val = val; }
* ListNode(int val, ListNode next) { this.val = val; this.next = next; }
* }
*/
class Solution {
public ListNode reverseKGroup(ListNode head, int k) {
ListNode dummy = new ListNode(0);
dummy.next = head;
ListNode prev = dummy;
ListNode curr = head;
int count = 0;
while (curr != null) {
count++;
ListNode next = curr.next;
if (count == k) {
prev = reverse(prev, next);
count = 0;
}
curr = next;
}
return dummy.next;
}
private ListNode reverse(ListNode start, ListNode end) {
ListNode prev = start;
ListNode curr = start.next;
ListNode first = curr;
while (curr != end) {
ListNode next = curr.next;
curr.next = prev;
prev = curr;
curr = next;
}
start.next = prev;
first.next = curr;
return first;
}
}复杂度分析:
时间复杂度分析:
- 遍历链表需要 O(n) 的时间,其中 n 是链表的长度。
- 在每个节点组内进行翻转操作时,需要遍历 k 个节点,即 O(k) 的时间复杂度。
- 总共有 n/k 个节点组,所以翻转操作的总时间复杂度是 O((n/k) * k) = O(n)。
因此,总的时间复杂度是 O(n)。
空间复杂度分析:
- 我们只使用了常数级别的额外空间,例如指针变量和辅助节点。
- 因此,空间复杂度是 O(1)。
综上所述,该解法的时间复杂度为 O(n),空间复杂度为 O(1)。
LeetCode运行结果:
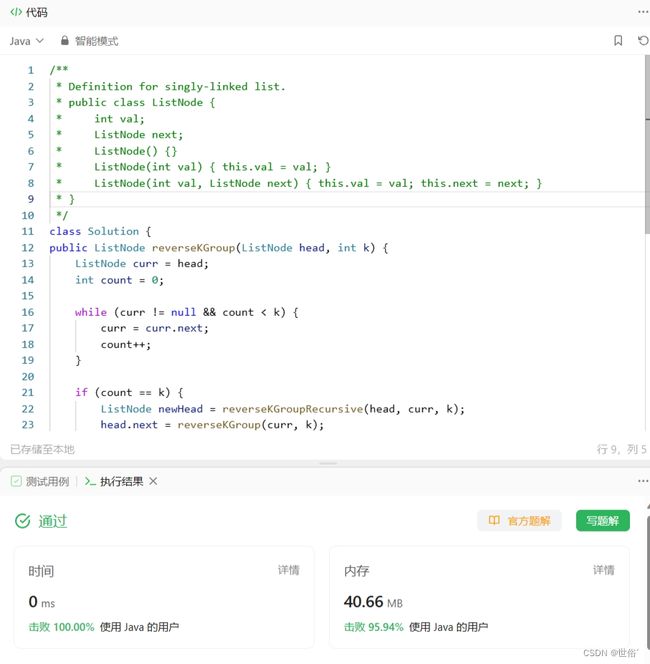
方法二:递归
除了迭代的解法,还可以使用递归的思路来实现链表的翻转。
具体的递归思路如下:
- 定义一个函数
reverseKGroupRecursive,其功能是将以head为头节点的链表每 k 个节点进行翻转,并返回翻转后的链表的头节点。 - 首先遍历链表,找到第 k+1 个节点
next。- 如果
next!=null,说明当前组内有至少 k 个节点,可以进行翻转操作。- 调用
reverse函数对以head为头节点、以next为尾节点的子链表进行翻转,并将翻转后的尾节点返回,将其赋值给newHead。 - 将
head的 next 指针指向下一组的头节点,即调用reverseKGroupRecursive(next, k)。 - 返回
newHead,作为翻转后的链表的头节点。
- 调用
- 如果
next==null,说明剩余的节点数少于 k 个,无需翻转,直接返回head。
- 如果
/**
* Definition for singly-linked list.
* public class ListNode {
* int val;
* ListNode next;
* ListNode() {}
* ListNode(int val) { this.val = val; }
* ListNode(int val, ListNode next) { this.val = val; this.next = next; }
* }
*/
class Solution {
public ListNode reverseKGroup(ListNode head, int k) {
ListNode curr = head;
int count = 0;
while (curr != null && count < k) {
curr = curr.next;
count++;
}
if (count == k) {
ListNode newHead = reverseKGroupRecursive(head, curr, k);
head.next = reverseKGroup(curr, k);
return newHead;
}
return head;
}
private ListNode reverseKGroupRecursive(ListNode head, ListNode tail, int k) {
ListNode prev = tail;
ListNode curr = head;
while (curr != tail) {
ListNode next = curr.next;
curr.next = prev;
prev = curr;
curr = next;
}
return prev;
}
}复杂度分析:
时间复杂度分析:
- 遍历链表需要 O(n) 的时间,其中 n 是链表的长度。
- 递归调用了 n/k 次,每次都需要翻转 k 个节点,因此每次递归操作的时间复杂度是 O(k)。
- 整个算法的时间复杂度是 O((n/k) * k) = O(n)。
因此,总的时间复杂度是 O(n)。
空间复杂度分析:
- 递归的深度是 n/k,因此递归调用栈的最大深度是 O(n/k)。
- 每次递归操作需要常数级别的额外空间,例如指针变量和辅助节点。
- 因此,空间复杂度是 O(n/k),在最坏情况下为 O(n)。需要注意的是,在实际使用中链表长度较大时,递归方法可能会导致递归调用栈溢出。
综上所述,递归解法的时间复杂度为 O(n),空间复杂度为 O(n/k)(在最坏情况下为 O(n))。
需要注意的是,递归方法对于链表长度较大时可能会导致递归调用栈溢出,因此在实际使用中需要注意链表长度是否适合使用递归解法。
LeetCode运行结果:
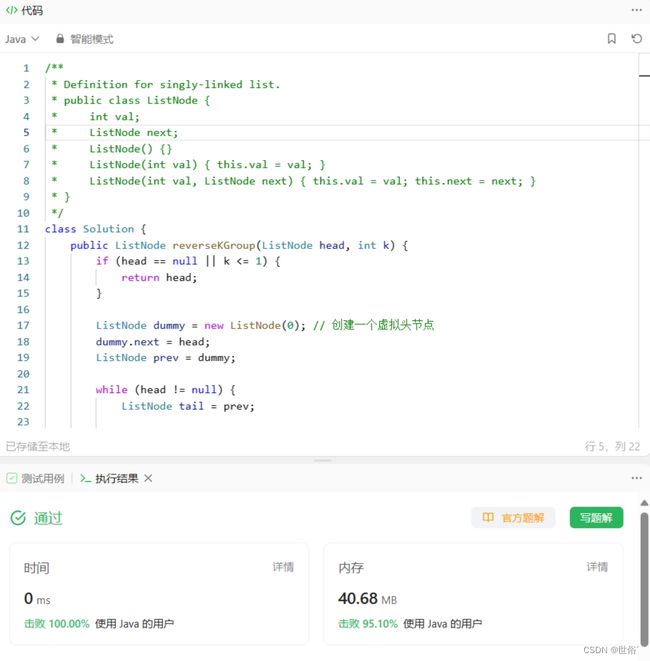
方法三:指针转向
除了迭代、递归的方法,还可以使用指针转向的思路来实现链表的翻转。
/**
* Definition for singly-linked list.
* public class ListNode {
* int val;
* ListNode next;
* ListNode() {}
* ListNode(int val) { this.val = val; }
* ListNode(int val, ListNode next) { this.val = val; this.next = next; }
* }
*/
class Solution {
public ListNode reverseKGroup(ListNode head, int k) {
if (head == null || k <= 1) {
return head;
}
ListNode dummy = new ListNode(0); // 创建一个虚拟头节点
dummy.next = head;
ListNode prev = dummy;
while (head != null) {
ListNode tail = prev;
// 判断剩余节点数是否大于等于 k
for (int i = 0; i < k; i++) {
tail = tail.next;
if (tail == null) {
// 剩余的节点数不满 k 个,直接返回结果
return dummy.next;
}
}
ListNode nextGroupHead = tail.next; // 下一组节点的头节点
// 翻转当前组内的节点
ListNode[] reversed = reverse(head, tail);
head = reversed[0];
tail = reversed[1];
// 将翻转后的组连接到结果链表中
prev.next = head;
tail.next = nextGroupHead;
// 更新 prev 和 head,准备处理下一组
prev = tail;
head = nextGroupHead;
}
return dummy.next;
}
private ListNode[] reverse(ListNode head, ListNode tail) {
ListNode prev = tail.next;
ListNode curr = head;
while (prev != tail) {
ListNode next = curr.next;
curr.next = prev;
prev = curr;
curr = next;
}
return new ListNode[] {tail, head};
}
}
该方法通过指针转向的方式,每次翻转一组的节点。其中,reverse 方法用于翻转当前组内的节点。
复杂度分析:
时间复杂度分析:
- 翻转一组的时间复杂度为 O(k),其中 k 是每组节点的数量。
- 对于包含 n 个节点的链表,一共有 n/k 组需要翻转。
- 因此,总的时间复杂度为 O((n/k) * k) = O(n)。
空间复杂度分析:
- 空间复杂度取决于额外使用的变量和递归调用栈的空间。
- 额外使用的变量有 dummy、prev、tail 和 nextGroupHead,它们占用的空间是常数级别的,因此不会随着输入规模 n 的增加而增加。
- 在递归实现中,递归调用栈的深度最多为 n/k,因为总共有 n/k 组需要翻转。
- 因此,递归调用栈的空间复杂度为 O(n/k)。
- 综上所述,总的空间复杂度为 O(1)。
综合来说,该方法的时间复杂度为 O(n),空间复杂度为 O(1)。需要注意的是,在处理不满 k 个节点的情况时,需要额外判断并返回结果。
LeetCode运行结果:
第二题
题目来源
26. 删除有序数组中的重复项 - 力扣(LeetCode)
题目内容
解决方法
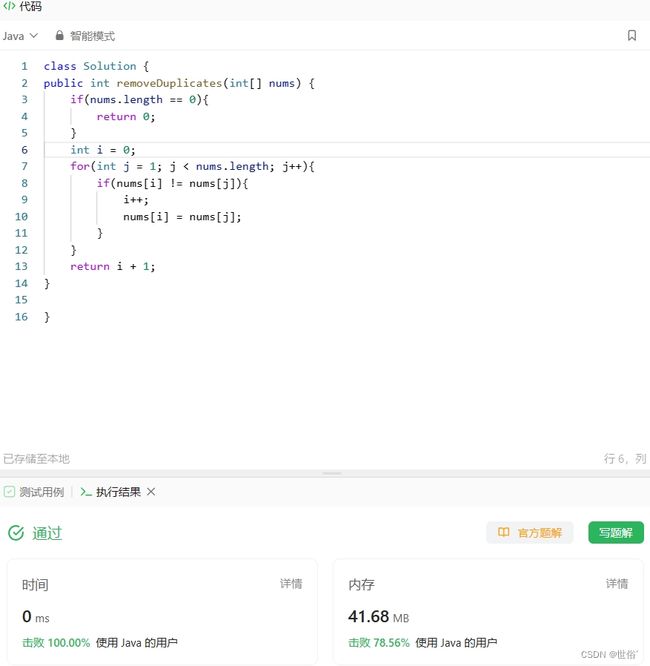
方法一:快慢指针
由于数组已经按非严格递增排列,可以利用快慢指针来遍历数组。慢指针指向当前不重复的元素,快指针用于遍历整个数组。如果快指针指向的元素与慢指针指向的元素不同,说明找到了一个新的不重复元素,将其存放在慢指针的下一个位置,并将慢指针后移一位。最后返回慢指针加1即可。
class Solution {
public int removeDuplicates(int[] nums) {
if(nums.length == 0){
return 0;
}
int i = 0;
for(int j = 1; j < nums.length; j++){
if(nums[i] != nums[j]){
i++;
nums[i] = nums[j];
}
}
return i + 1;
}
}复杂度分析:
-
时间复杂度:O(n),其中n为数组的长度。该算法通过使用快慢指针,只需一次遍历数组即可完成操作。
-
空间复杂度:O(1)。该算法只使用了常数级的额外空间,不随输入规模的增加而增加。
LeetCode运行结果:
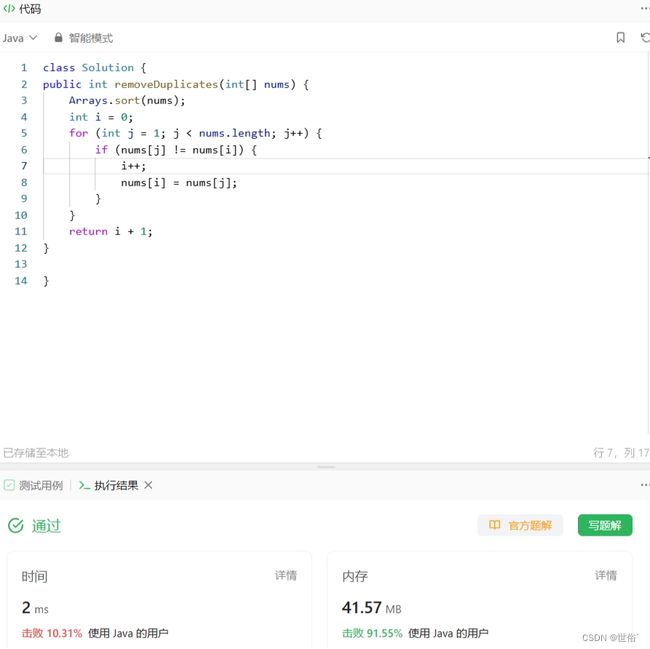
方法二:Arrays类的sort方法
除了使用快慢指针的解法外,在Java中还可以使用Arrays类的sort方法来解决该问题。
该方法首先对数组进行排序,使得重复元素相邻。然后使用快慢指针的方法遍历数组,将不重复的元素放到慢指针所指向的位置,并移动慢指针。最后返回慢指针加1作为新数组的长度。
class Solution {
public int removeDuplicates(int[] nums) {
Arrays.sort(nums);
int i = 0;
for (int j = 1; j < nums.length; j++) {
if (nums[j] != nums[i]) {
i++;
nums[i] = nums[j];
}
}
return i + 1;
}
}复杂度分析:
- 时间复杂度:O(n log n),其中n为数组的长度。排序需要O(n log n)的时间复杂度,遍历数组需要O(n)的时间复杂度。
- 空间复杂度:O(1),原地修改数组,不需要额外的空间。
LeetCode运行结果:
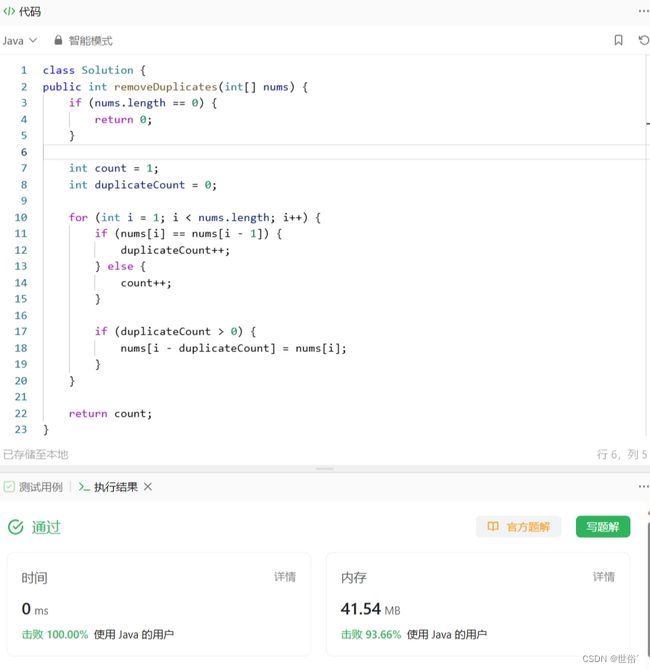
方法三:计数器
除了前面提到的思路和方法,还可以使用一个计数器来记录重复元素的个数,并根据计数器的值进行相应的操作。
- 该方法使用count变量来记录数组中不重复元素的个数,duplicateCount变量来记录重复元素的个数。
- 遍历数组时,如果当前元素与前一个元素相同,则将duplicateCount加1;否则将count加1。
- 同时,如果duplicateCount大于0,则将当前元素向前移动duplicateCount个位置,相当于删除了重复元素。
- 最后返回count作为新数组的长度。
class Solution {
public int removeDuplicates(int[] nums) {
if (nums.length == 0) {
return 0;
}
int count = 1;
int duplicateCount = 0;
for (int i = 1; i < nums.length; i++) {
if (nums[i] == nums[i - 1]) {
duplicateCount++;
} else {
count++;
}
if (duplicateCount > 0) {
nums[i - duplicateCount] = nums[i];
}
}
return count;
}
}复杂度分析:
- 时间复杂度:O(n),其中n为数组的长度。需要遍历整个数组一次。
- 空间复杂度:O(1),原地修改数组,不需要额外的空间。
在遍历数组时,只有在出现重复元素时才会对数组进行修改。对数组的修改是通过将重复元素向前移动来实现的,而不是删除重复元素。因此,不需要额外的空间来存储删除后的新数组。这种方法的空间复杂度是常数级别的。
综上所述,使用计数器的解法是一种高效的解决方案,具有线性的时间复杂度和常数级别的空间复杂度。
LeetCode运行结果:
方法四:额外的数组
除了之前提到的思路和方法,还可以使用额外的数组来存储不重复的元素。
该方法创建一个新的数组uniqueNums,用于存储不重复的元素。遍历原始数组时,如果当前元素与前一个元素不相同,则将当前元素添加到uniqueNums数组中,并将计数器count加1。最后,将uniqueNums数组中的元素赋值回原数组nums,并返回count作为新数组的长度。
class Solution {
public int removeDuplicates(int[] nums) {
if (nums.length == 0) {
return 0;
}
int[] uniqueNums = new int[nums.length];
uniqueNums[0] = nums[0];
int count = 1;
for (int i = 1; i < nums.length; i++) {
if (nums[i] != nums[i - 1]) {
uniqueNums[count] = nums[i];
count++;
}
}
// 将uniqueNums数组中的元素赋值回原数组
for (int i = 0; i < count; i++) {
nums[i] = uniqueNums[i];
}
return count;
}
}复杂度分析:
- 时间复杂度:O(n),其中n为数组的长度。需要遍历整个数组一次,并进行一次数组元素的复制操作。
- 空间复杂度:O(n),因为需要使用额外的数组来存储不重复的元素。
在遍历原始数组时,只有在出现与前一个元素不相同的元素时才将其添加到新数组中。因此,新数组的长度最多为原数组的长度,即使用了额外的O(n)空间。
综上所述,使用额外数组的解法是一种线性时间复杂度的解决方案,但需要额外的空间来存储新的数组。这种方法适用于不要求原地修改数组的情况。
LeetCode运行结果:
第三题
题目来源
27. 移除元素 - 力扣(LeetCode)
题目内容
解决方法
方法一:双指针
这道题可以使用双指针的方法来解决。定义两个指针:慢指针slow和快指针fast。
算法的思路如下:
- 初始化慢指针slow为0。
- 遍历数组,如果当前元素nums[fast]等于给定值val,则快指针fast向前移动一步,跳过该元素。
- 如果当前元素nums[fast]不等于给定值val,则将nums[fast]赋值给nums[slow],同时慢指针slow向前移动一步。
- 重复步骤2和步骤3,直到快指针fast遍历完整个数组。
- 返回慢指针slow的值。
class Solution {
public int removeElement(int[] nums, int val) {
int slow = 0;
for (int fast = 0; fast < nums.length; fast++) {
if (nums[fast] != val) {
nums[slow] = nums[fast];
slow++;
}
}
return slow;
}
}复杂度分析:
- 时间复杂度: 遍历数组所需的时间为 O(n),其中 n 是数组的长度。在每次遍历时,只有快指针需要移动,而慢指针最多移动了 n 次。因此,整个算法的时间复杂度为 O(n)。
- 空间复杂度: 该算法使用了常数个额外变量,不随输入规模 n 变化,因此空间复杂度为 O(1)。无论输入数组的长度如何,算法都只需要固定的额外空间。
综上所述,该算法的时间复杂度为 O(n),空间复杂度为 O(1)。
LeetCode运行结果:
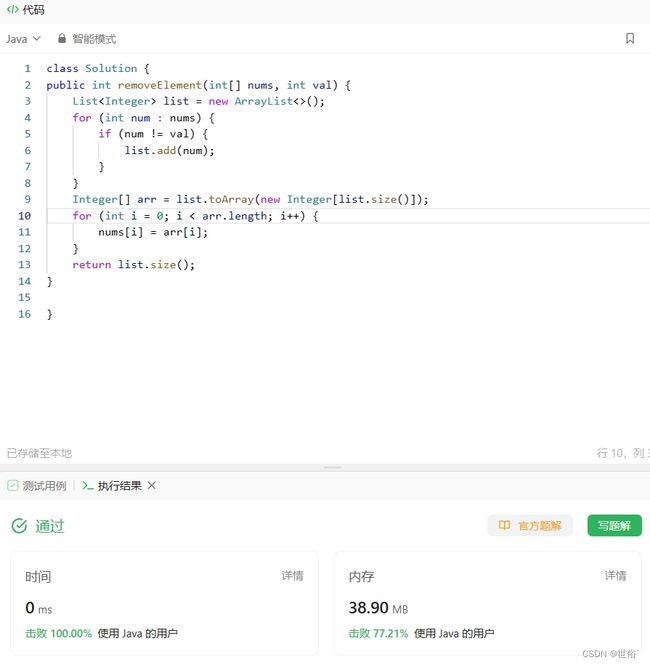
方法二:List
除了双指针的方法,还可以使用 Java 中的 List 来解决这个问题。具体的做法如下:
- 创建一个 List 集合(例如 ArrayList)来存储不等于给定值val的元素。
- 遍历数组,如果当前元素nums[i]不等于给定值val,则将其添加到 List 中。
- 将 List 转换为数组并返回。
class Solution {
public int removeElement(int[] nums, int val) {
List list = new ArrayList<>();
for (int num : nums) {
if (num != val) {
list.add(num);
}
}
Integer[] arr = list.toArray(new Integer[list.size()]);
for (int i = 0; i < arr.length; i++) {
nums[i] = arr[i];
}
return list.size();
}
} 这种方法的本质是通过使用 List 集合来存储不等于给定值val的元素,然后将 List 转换为数组并重新赋值给原数组。最后返回 List 的大小即为移除元素后的新长度。
复杂度分析:
时间复杂度:
- 遍历数组并将不等于给定值val的元素添加到 List,时间复杂度为 O(n),其中 n 是数组的长度。
- 将 List 转换为数组,需要遍历 List 中的元素,时间复杂度同样为 O(n)。
综上所述,整个算法的时间复杂度为 O(n)。
空间复杂度:
- 创建了一个大小为 n 的 List 来存储不等于给定值val的元素,占用了额外的 O(n) 空间。
- 将 List 转换为数组时,需要创建一个新的数组,大小为 List 的大小,同样占用了额外的 O(n) 空间。
综上所述,整个算法的空间复杂度为 O(n)。
相比之下,双指针和的方法在空间复杂度上是优于使用 List 的方法的,因为它只需要常数个额外变量,即空间复杂度为 O(1)。因此,建议使用双指针的方法来解决这个问题,以获得更好的空间效率。
LeetCode运行结果:
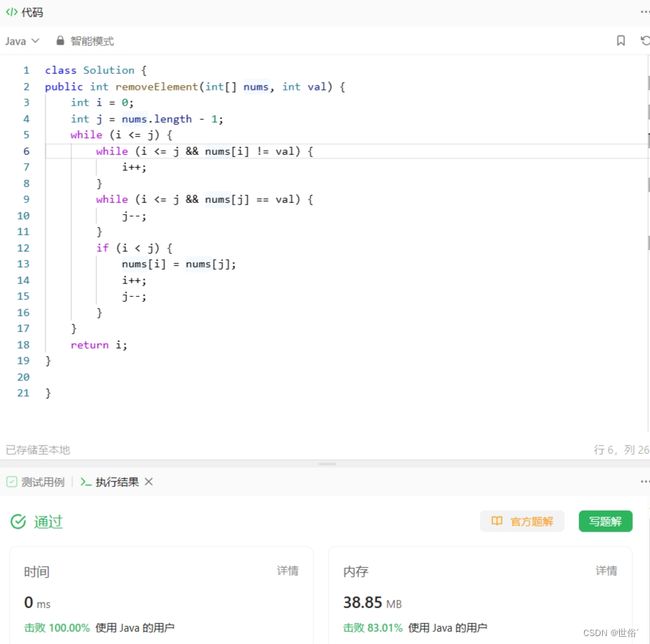
方法三:交换元素
除了双指针、List,还可以使用交换元素的思路来解决这个问题。具体的做法如下:
- 初始化两个指针i和j,初始值分别为0和数组长度-1。
- 循环移动指针i,直到找到第一个等于给定值val的元素。
- 循环移动指针j,直到找到第一个不等于给定值val的元素。
- 如果i小于j,则将元素nums[j]赋值给nums[i],并将指针i和j分别加1和减1。
- 重复步骤2到步骤4,直到i大于等于j。
- 返回指针i,即为移除元素后的新长度。
class Solution {
public int removeElement(int[] nums, int val) {
int i = 0;
int j = nums.length - 1;
while (i <= j) {
while (i <= j && nums[i] != val) {
i++;
}
while (i <= j && nums[j] == val) {
j--;
}
if (i < j) {
nums[i] = nums[j];
i++;
j--;
}
}
return i;
}
}这种交换元素的方法通过使用两个指针分别从头尾向中间移动,并通过交换元素的方式来实现移除元素的目的。
复杂度分析:
- 时间复杂度:使用两个指针分别从头尾向中间移动,最坏情况下需要遍历整个数组,时间复杂度为 O(n),其中 n 是数组的长度。
- 空间复杂度:这种方法只使用常数个额外变量,即空间复杂度为 O(1)。
综上所述,使用交换元素的方法的时间复杂度为 O(n),空间复杂度为 O(1)。与双指针的方法相比,交换元素的方法具有相同的时间复杂度,但空间复杂度更低,因为不需要额外的数据结构(如 List)来存储元素。从空间效率的角度来看,交换元素的方法是较优的选择。
LeetCode运行结果:
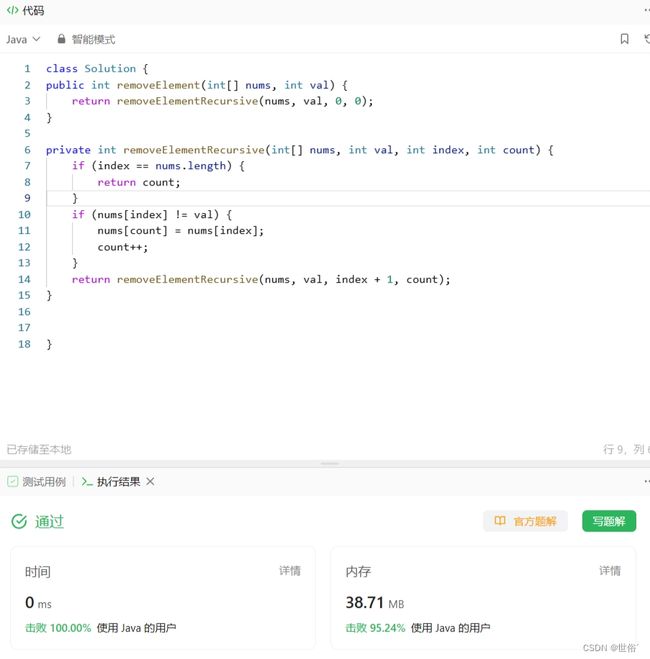
方法四:递归
除了双指针、交换元素和使用 List 的方法,还可以利用递归来解决这个问题。具体的做法如下:
- 编写一个递归函数,传入当前遍历到的索引位置和计数器 count。
- 在递归函数中,判断当前遍历到的元素是否等于给定值 val:
- 如果相等,则将计数器 count 加1,并调用递归函数继续处理下一个元素。
- 如果不相等,则将当前元素覆盖掉索引位置 count,并调用递归函数处理下一个元素。
- 递归函数的终止条件是遍历完整个数组,返回计数器 count 的值。
- 在主函数中,调用递归函数,传入初始索引0和计数器初始值0。
class Solution {
public int removeElement(int[] nums, int val) {
return removeElementRecursive(nums, val, 0, 0);
}
private int removeElementRecursive(int[] nums, int val, int index, int count) {
if (index == nums.length) {
return count;
}
if (nums[index] != val) {
nums[count] = nums[index];
count++;
}
return removeElementRecursive(nums, val, index + 1, count);
}
}这种递归的方法通过逐个处理数组元素,将不等于给定值 val 的元素覆盖到数组的前部分,从而实现移除元素的目的。
复杂度分析:
- 时间复杂度:递归函数需要遍历整个数组,因此时间复杂度取决于数组的长度,为 O(n),其中 n 是数组的大小。
- 空间复杂度:递归调用会占用一定的栈空间,最坏情况下递归深度为 n,因此空间复杂度为 O(n)。
综上所述,使用递归的方法的时间复杂度为 O(n),空间复杂度为 O(n)。
需要注意的是,递归方法的空间复杂度相对较高,因为每次递归调用都需要在栈上保存一些信息。在处理大规模数组时,可能会导致栈溢出。因此,如果要处理大规模数组,建议使用其他方法,如双指针或交换元素的方法,以保证较低的空间开销。
LeetCode运行结果: