【COMP305 LEC6 LEC 7】
LEC 6
Topic 2. The McCulloch-Pitts Neuron (1943)
1. What kind of propositions can be represented by a single MP neuron (without time)?
OR:
中间那条线就是 a1 + a2 = 1
分成了两半:1. on the line 和 above the line
2. below the line
换句话说:所以促进神经元输出的在线的一边;而抑制神经元输出的在另一边
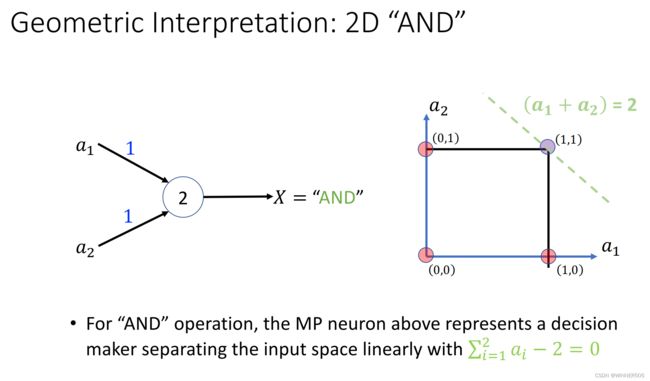
AND:
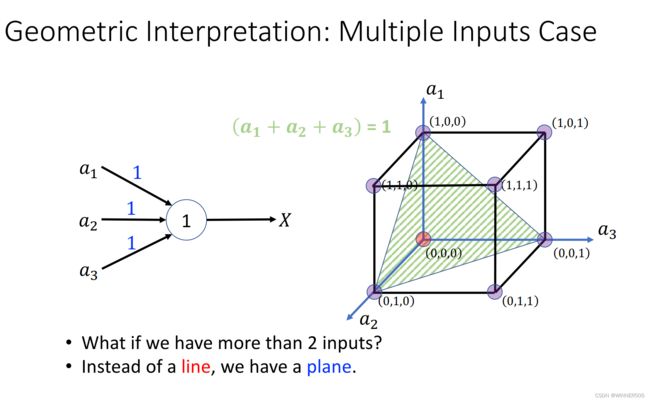
Multiple inputs case:
A single MP neuron can be used to represent some Boolean functions which are linearly separable.
Linear separability (for Boolean functions): There exists a line (plane) such that all inputs which produce a 1 for the function lie on one side of the line (plane) and all inputs which produce a 0 lie on other side of the line (plane).
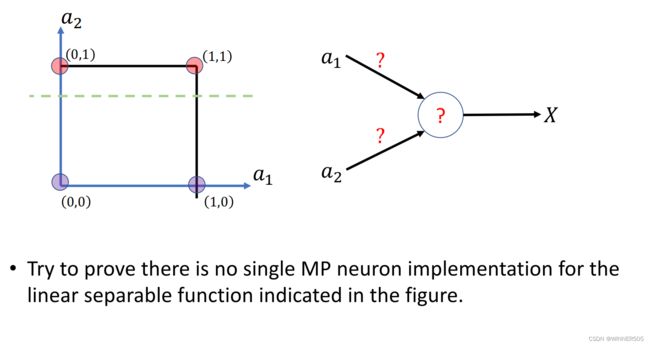
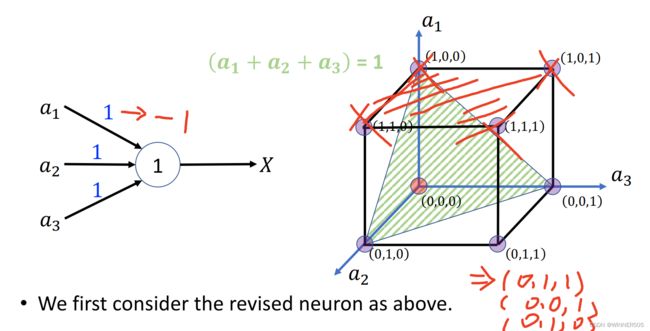
不是每一个线性可分的函数都可以用一个MP神经元来表示
例子:
Summary:
LEC 7
Although the McCulloch-Pitts neuron model was very simplistic, it can perform
the basic logic operations AND, OR and NOT
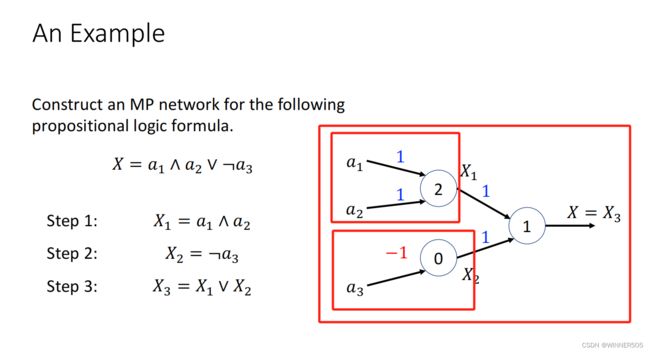
An MP neural network can implement any multivariable propositional logic
function , with the thresholds and weights being appropriately selected.
MP神经网络可以实现任何多变量命题逻辑函数,阈值和权值被适当地选择。
Furthermore, the discrete time, or unity delay property of the model makes it
even possible to build a sequential digital circuitry
此外,该模型的离散时间或统一延迟特性甚至使建立一个顺序的数字电路成为可能
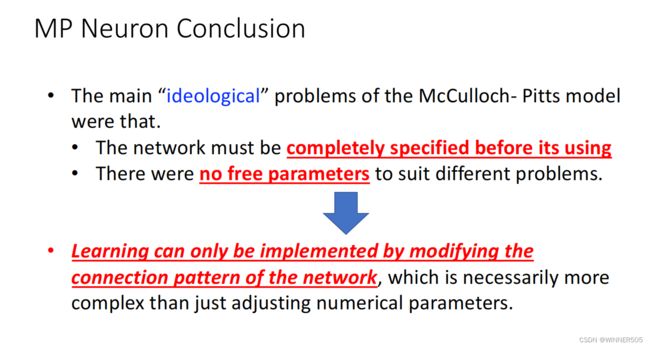
Though the threshold elements are, from the combinatorial point of view, more
versatile than conventional logic gates, there was a problem with assumed
unlimited fan-in (number of inputs of a logic gate):
虽然从组合的角度来看,阈值元素比传统的逻辑门更通用,但在假设的无限扇入(逻辑门的输入数量)方面存在一个问题:
但:
the implementation of the compact electronic model was not feasible in the
days of bulky vacuum tubes
Now-days a possible way of overcoming the hardware difficulties could be the use of
optical computing elements 光学计算原件
capable of providing unlimited fan-in by changing the angle of lens.
• But still not quite clear yet…