【数据结构】堆的实现
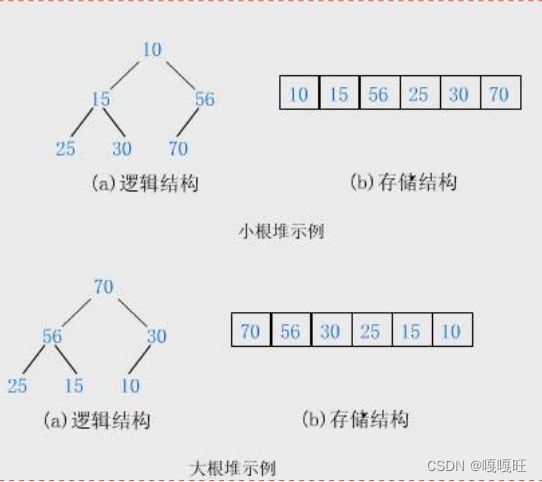
大小堆的概念
将根节点最大的堆叫做最大堆或大根堆,根节点最小的堆叫做最小堆或小根堆。
堆的接口函数
void HeapInit(Heap*st);//堆的初始化
void swap(int* str1, int* str2);//交换两个数据
void Adjustup(int* a, int child);//向上调整
void HeapPush(Heap* st, int x);//插入元素
void AdjustDown(int* a, int n, int parent);//向下调整
bool HeapEmpty(Heap* st);//堆是否为空
int HeapSize(Heap* st);//堆数据个数
void HeapPop(Heap* hp);//堆元素的删除
void HeapDestroy(Heap* st);//堆的销毁
int HeapTop(Heap* st);//堆顶元素
定义堆结构体
typedef struct Heap
{
int* a;
int size;
int capacity;
}Heap;
初始化堆
void HeapInit(Heap*st)
{
st->a = NULL;
st->capacity = 0;
st->size = 0;
}
交换两个数据
void swap(int* str1, int* str2)
{
int tmp = *str1;
*str1 = *str2;
*str2 = tmp;
}
判断堆是否为空
bool HeapEmpty(Heap* st)
{
assert(st);
if (st->size == 0)
{
return true;
}
else
{
return false;
}
}
堆元素的个数
int HeapSize(Heap* st)
{
assert(st);
return st->size;
}
堆的销毁
void HeapDestroy(Heap* st)
{
assert(st);
free(st->a);
st->a = NULL;
st->size = 0;
st->capacity = 0;
}
堆顶元素
int HeapTop(Heap* st)
{
assert(st);
assert(!HeapEmpty(st));
return st->a[0];
}
向上调整
void Adjustup(int* a, int child)
{
int parent = (child - 1) / 2;
while (child > 0)
{
if (a[child] < a[parent])
{
swap(&a[child], &a[parent]);
child = parent;
parent = (child - 1) / 2;
}
else
{
break;
}
}
}
插入数据一般就是插到最后一个,但是插入的数据不能保证该堆还是大堆或者小堆,所以要使用向上调整,举例说明
这是一个小堆
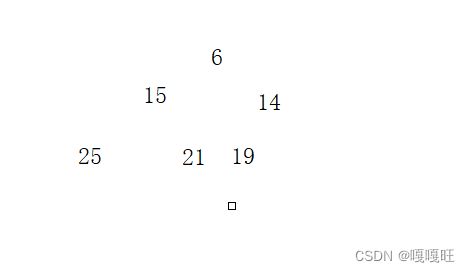
插入一个数据4后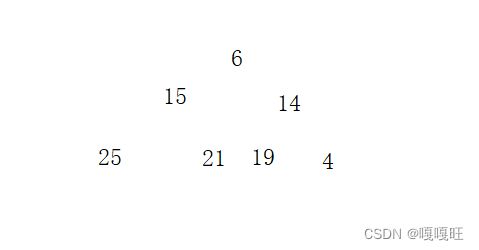
然后就不是堆了,要变成堆,必须将4向上调整,影响的只有他的祖先6和14

4作为向上调整的孩子,他的下标为6,他的父亲14,下标为2,
下标对应关系为 parent = (child - 1) / 2
因为是小堆,如果孩子小于父亲的话,交换孩子与父亲的数据
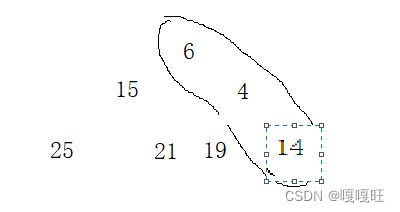
原来父亲与孩子的关系如下
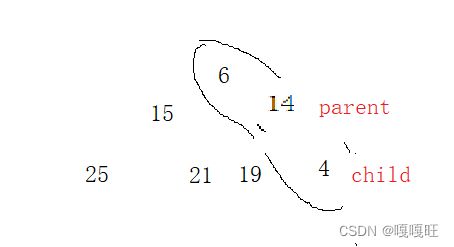
交换父亲和孩子的数据后,改变孩子和父亲的下标如下
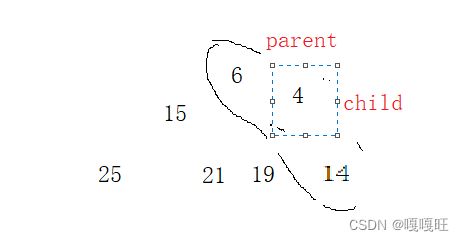
对应操作是 child = parent; parent = (child - 1) / 2;
继续比较孩子和父亲的值,如果孩子小于父亲,就交换.

然后改变父亲与孩子的下标改变

对应操作为 child = parent; parent = (child - 1) / 2;
==注意:当父亲大于孩子时,一直循环,当孩子为堆顶元素时,调整结束,child下标为0,所以循环结束条件为child>0,为什么不用parent<0作为循环结束的条件呢,是因为当child=0时,parent= (child - 1) / 2=0,所以不能用父亲判断.可能在循环过程中遇到父亲小于孩子,这样的话已经成为小堆了.直接break跳出.
插入数据
void HeapPush(Heap* st, int x)
{
if (st->capacity == st->size)
{
int newcapcity = st->capacity == 0 ? 4 : st->capacity * 2;
int* tmp = (int*)realloc(st->a, newcapcity*sizeof(int));
if (tmp == NULL)
{
perror("realloc fail");
}
st->a = tmp;
st->capacity = newcapcity;
}
st->a[st->size] = x;
st->size++;
Adjustup(st->a, st->size - 1);
}
每插入一个数据向上调整,随时保证他是小堆
向下调整
void AdjustDown(int* a, int n, int parent)
{
int child = parent * 2 + 1;
while (child < n)
{
if (child + 1 < n && a[child + 1] < a[child])
{
child++;
}
if (a[child] < a[parent])
{
swap(&a[child], &a[parent]);
parent= child;
child = parent * 2 + 1;
}
else
{
break;
}
}
}
删除数据
void HeapPop(Heap* hp)
{
assert(hp);
assert(!HeapEmpty(hp));
swap(&hp->a[0], &hp->a[hp->size - 1]);
hp->size--;
AdjustDown(hp->a,hp->size, 0);
}
删除数据我们是删除的堆顶数据,如果直接删掉堆顶数据的话,父子关系全部乱套,就不是堆了.
所以我们采取的是将堆顶元素和堆的最后一个元素交换,然后删除最后一个元素,接着描述堆数据个数的size–;接下来就是要调整堆顶数据,让其保证还是小堆.
比如还是之前的例子
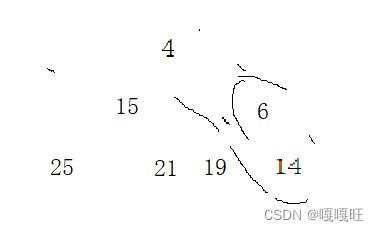
要删除堆顶元素时,先交换堆顶元素和堆尾元素
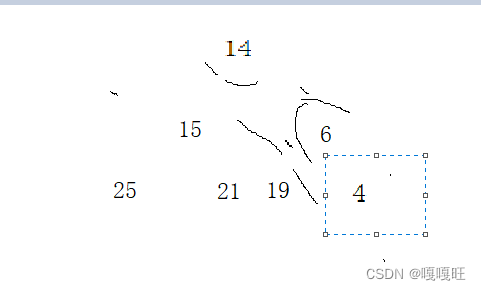
然后删除堆尾元素

然后选取堆顶元素孩子小的.
if (child + 1 < n && a[child + 1] < a[child])
{
child++;
}
这样保证child下标对应的一定是小孩子,child + 1 < n 这个处理没有右孩子的情况
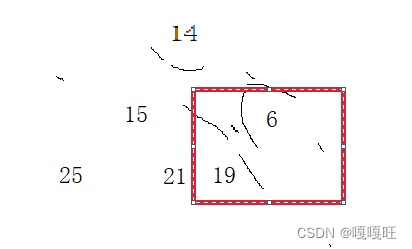
比如说这里,没有右孩子,child下标范围0-n-1(n为堆数据个数).选出左右孩子中小的,和父亲交换,交换完了,将孩子下标给父亲,孩子的下标更新为孩子的孩子的下标

如果只有一个孩子并且父亲大于孩子
则执行这个

如果孩子大于父亲的话,就已经是小堆了,直接break;
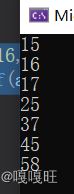
这里循环的条件是child主函数测试
int main()
{Heap hp;
HeapInit(&hp);
int arr[] = {17,25,15,37,45,16,58};
int sz = sizeof(arr) / sizeof(arr[0]);
int i = 0;
for (i = 0; i < sz; i++)
{
HeapPush(&hp, arr[i]);//将数组中的元素压入堆中
}
while (!HeapEmpty(&hp))//如果堆不为空
{
int top = HeapTop(&hp);//取堆顶元素
printf("%d\n", top);//打印堆顶元素
HeapPop(&hp);//删除堆顶元素
}
return 0;
}
编译运行