C++矩阵运算QT应用之Eigen库

前言

本文主要描述在c++中应用Eigen进行矩阵（向量）的表示运算，以及Eigen库的下载和配置。

一. Eigen库介绍、下载及配置

Eigen是C++中可以用来调用并进行矩阵计算的一个库，里面封装了一些类，需要的头文件和功能如下：

有关Eigen的详细介绍可以查看其官网主页：http://eigen.tuxfamily.org/dox/index.html

• 下载
  Eigen的下载地址如下：http://eigen.tuxfamily.org/index.php?title=Main_Page#Download
  在网页右上方选择选择不同版本及格式的压缩包，我这里选择的最新（2023.4.24）发布版本3.4.0的zip文件，点击直接进行下载。
• 配置
  （1）将下载的压缩包进行解压；
  ----------------在VS中配置------------------
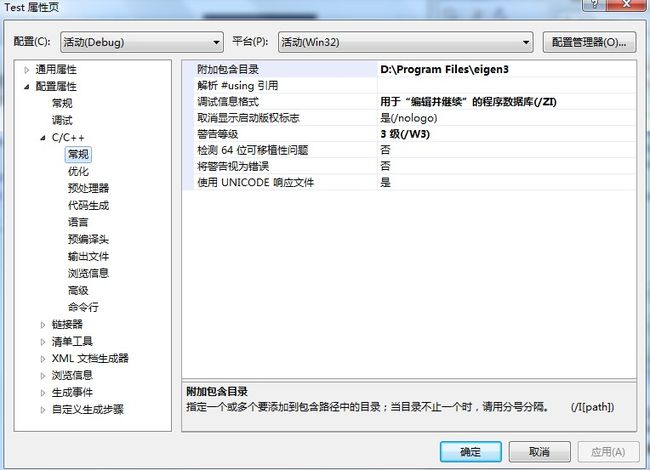
  右键 项目属性—》配置属性—》C/C++—》常规—》附加包含目录（对话框右边），在对应条款右侧的编辑框中添加解压的Eigen文件夹完整地址目录即可。（如引用下图）
  
  ----------------在QT中配置------------------
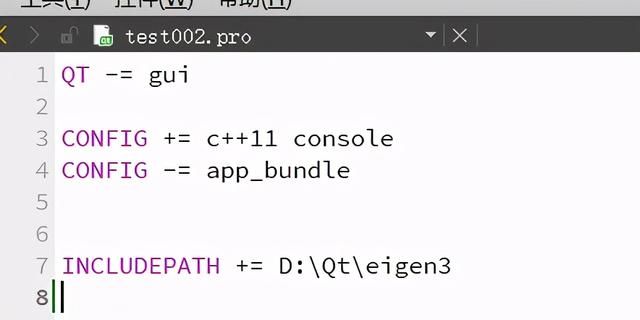
  在QT creator中新建控制台应用项目，在define bulid system中选择qmake，下一步选择MinGW构建套件。完成项目创建后，在项目下的pro文件中添加：INCLUDEPATH += D:\Program Files\eigen3，如下图：
  
  PS：要在命令窗口中显示结果，需要设置：左侧菜单栏项目–》运行–》勾选在终端运行 即可。
  

二. 使用

(1）Eigen库的引用–一个栗子

#include 
#include 
#include 
//typedef Eigen::MatrixXd Matrix;
//typedef  Eigen::VectorXd Vector;
using namespace Eigen;

using namespace std;
int main(int argc, char *argv[])
{
    QCoreApplication a(argc, argv);
    //    Matrix m(3,3);
    MatrixXd m(3,3);
    m<<1,2,3,
        4,5,6,
        7,8,9;
    //    Vector v(3);
    VectorXd v(3);
    v<<1,2,3;
    Vector3d w(11,22,33);
//    cout<<"m*v=\n"<
//    cout<
//    cout<(0,0)<
//    cout<
//    m.row(2)<<11,12,13;   //第2行赋值
//    cout<
//    MatrixXd mm;
//    mm=m;
//    cout<
    Eigen::Matrix<int, 3, 4> mat1;
    Eigen::Matrix<double, 3, Dynamic> mat2;
    Eigen::Matrix2cd mat3;

    return a.exec();
}

（2）矩阵的定义
矩阵模板函数中一共包含六个模板参数，前三个是比较常用的，分别表示矩阵元素的类型、行数、列数。在矩阵定义的时候可以使用 Dynamic 来表示行或者列数未知。

template<typename _Scalar, int _Rows, int _Cols, int _Options, int _MaxRows, int _MaxCols>
class Eigen::Matrix< _Scalar, _Rows, _Cols, _Options, _MaxRows, _MaxCols >

Eigen::Matrix<int, 3, 4> mat1;              //  3x4 的 int 类型的矩阵 mat1
Eigen::Matrix<double, 3, Dynamic> mat2;     //  3x? 的 double 类型的矩阵 mat2
Eigen::Matrix<float, Dynamic, 4> mat3;      //  ?x4 的 float 类型的矩阵 mat3
Eigen::Matrix<long, Dynamic, Dynamic> mat4; //  ?x? 的 long 类型的矩阵 mat4

在 Eigen 中 typedef 了很多矩阵的类型，通常命名为 Matrix 前缀加一个长度为 1∼4的字符串 S 的命名——MatrixS。其中 S 可以用来判断该矩阵类型，数字 n 表示 n ∗ n，n 的范围是2∼4，字母 d、f、i、c 表示 double、float、int、complex,另外 X 表示行或者列数未知的矩阵。

typedef Matrix<std::complex<double>, 2, 2> Eigen::Matrix2cd;            //  2x2 的 cd 类型的矩阵
typedef Matrix<double, 2, 2> Eigen::Matrix2d;                           //  2x2 的 d 类型的矩阵
typedef Matrix<std::complex<double>, 2, Dynamic> Eigen::Matrix2Xcd;     //  2x? 的 cd 类型的矩阵
typedef Matrix<std::complex<float>, Dynamic, 2> Eigen::MatrixX2cf;      //  ?x2 的 cf 类型的矩阵
typedef Matrix<std::complex<double>, Dynamic, Dynamic> Eigen::MatrixXcd;//  ?x? 的 cd 类型的矩阵
typedef Matrix<int, Dynamic, Dynamic> Eigen::MatrixXi;                  //  ?x? 的 i 类型的矩阵

• 说明1 ：行/列优先。Eigen库中的矩阵默认存储是列优先的，和matlab很相似，而在C++中的存储方式是行优先，如果为了不搞混，可以使用矩阵模板中的第四个参数（默认是 ColMajor），关于行/列优先可参考https://blog.csdn.net/shenck1992/article/details/50041777

Matrix<int, 5, 6, RowMajor> matRow; //  行优先的 5x6 的 int 类型矩阵

• 说明2：静/动态矩阵。动态矩阵是Eigen 中可以用 Dynamic 表示行或者列数未知，如果定义一个矩阵时并不能确定矩阵的大小，只有在运行时才可以确定大小进行动态分配，而静态矩阵是在定义时便明确给定了行数以及列数，在编译时就可以分配好内存。

MatrixXd m = MatrixXd::Random(3,3);
Matrix3d m = Matrix3d::Random();

MatrixXd表示任意大小的元素类型为double的矩阵变量，其大小在运行时被赋值后才能知道；MatrixXd::Random(3,3)表示产生一个元素类型为double的33的临时矩阵对象。
Matrix3d表示元素类型为double大小为33的矩阵变量，其大小在编译时就知道。
（3）矩阵访问/赋值

======================矩阵元素、块访问，矩阵赋值===================
x = mat(a, b);  //  获取到矩阵 mat 的 a 行 b 列的元素并赋值给 x
mat(b, a) = x;  //  将 x 赋值给矩阵 mat 的 b 行 a 列
mat1 = mat2;    //  将矩阵 mat2 赋值（拷贝）给矩阵 mat1

mat = mat1.block(i, j, p, q);   //  从矩阵 mat1 的 i 行 j 列开始获取一个 p 行 q 列的子矩阵
mat = mat1.block<p, q>(i, j);   //  从矩阵 mat1 的 i 行 j 列开始获取一个 p 行 q 列的子矩阵（动态矩阵）

mat = mat1.row(i);  //  获取 mat1 的第 i 行
mat = mat1.col(j);  //  获取 mat1 的第 j 列  

Matrix3f m;
m << 1, 2, 3,
4, 5, 6,
7, 8, 9;
std::cout << m;

mat.fill(n);    //  将 mat 的所有元素均赋值为 n

看如下例子：

	MatrixXd m(2, 2); //MatrixXd表示是任意尺寸的矩阵ixj, m(2,2)代表一个2x2的方块矩阵
	m(0, 0) = 3; //代表矩阵元素a11
	m(1, 0) = 2.5; //a21
	m(0, 1) = -1; //a12
	m(1, 1) = m(1, 0) + m(0, 1);//a22=a21+a12
	cout << "m="<<endl<<m << endl;//输出矩阵m
	cout << "m(2)="<<m(2) << endl; //这里注意，这里的二维数组是列优先，m(2)取得是第二列第一行的数

输出结果：

（4）矩阵计算

//=====================================加减法=============================================
	Matrix2d a;//这里直接定义的a是个2*2的方阵
	a << 1, 2,
		3, 4;
	MatrixXd b(2, 2);
	b << 2, 3,
		1, 4;
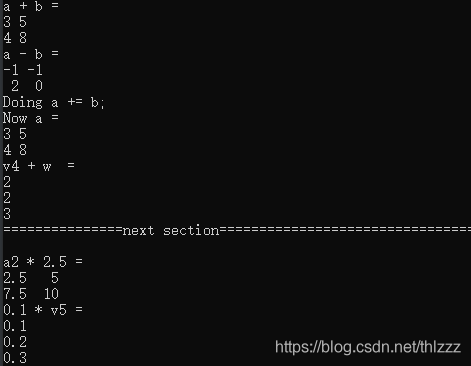
	cout << "a + b =\n" << a + b << endl;//矩阵加法
	cout << "a - b =\n" << a - b << endl;//矩阵减法
	cout << "Doing a += b;" << endl;
	a += b;//a = a + b，同时重新赋值a
	cout << "Now a =\n" << a << endl;
	Vector3d v4(1, 2, 3);
	Vector3d w(1, 0, 0);
	cout << "v4 + w  =\n" <<  w + v4 << endl;//向量加减法
	cout << "===============next section===============================================" << endl << endl;

	//=================================矩阵数乘===============================================
	Matrix2d a2;
	a2 << 1, 2,
		3, 4;
	Vector3d v5(1, 2, 3);
	cout << "a2 * 2.5 =\n" << a2 * 2.5 << endl;//矩阵数乘
	cout << "0.1 * v5 =\n" << 0.1 * v5 << endl;//向量数乘

//====================================矩阵/向量乘法========================================
	Matrix2d m4;
	m4 << 1, 2,
		3, 4;
	Vector2d u6(-1, 1), v6(2, 0);
	cout << "Here is m4*m4:\n" << m4 * m4 << endl;//矩阵-矩阵
	cout << "Here is m4*u6:\n" << m4 * u6 << endl;//矩阵-向量
	cout << "Here is u6^T*m4:\n" << u6.transpose()*m4 << endl;//向量-矩阵
	cout << "Here is u6^T*v6:\n" << u6.transpose()*v6 << endl;//向量-向量
	cout << "Here is u6*v6^T:\n" << u6 * v6.transpose() << endl;//向量-向量
	cout << "===============next section===============================================" << endl << endl;

//==============================转置，共轭,伴随以及逆矩阵=======================================

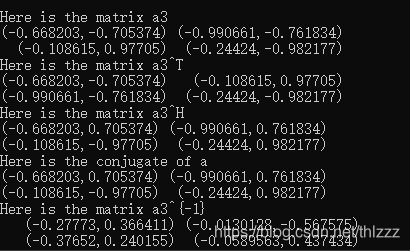
	MatrixXcf a3 = MatrixXcf::Random(2, 2);//定义2-by-2随机矩阵
	cout << "Here is the matrix a3\n" << a3 << endl;//矩阵a3
	cout << "Here is the matrix a3^T\n" << a3.transpose() << endl;//a3的转置
	cout << "Here is the matrix a3^H\n" << a3.conjugate() << endl;//a3的共轭
	cout << "Here is the adjoint of a3\n" <<a3.adjoint( << endl;//a3的伴随矩阵
	cout << "Here is the matrix a3^{-1}\n" << a3.inverse() << endl;//a3的逆
	cout << "===============next section===============================================" << endl << endl;

ps：伴随矩阵图片结果有问题，请自动忽略。

//=====================================点乘/叉乘============================================
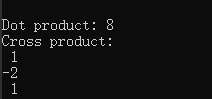
	Vector3d v7(1, 2, 3);
	Vector3d w7(0, 1, 2);
	cout << "Dot product: " << v7.dot(w7) << endl;//向量点乘
	cout << "Cross product:\n" << v7.cross(w7) << endl;//向量叉乘
	cout << "===============next section===============================================" << endl << endl;

//==================================== 其他功能==============================================

	Matrix3d m5;
	m5 << 1, 2, 3,
		1, 2, 1,
		0, 2, 4;
	cout << "Here is m5.determinant():   " << m5.determinant() << endl;// 行列式
	cout << "Here is m5.sum():           " << m5.sum() << endl;//所有元素之和
	cout << "Here is m5.prod():          " << m5.prod() << endl;//所有元素之积
	cout << "Here is m5.mean():          " << m5.mean() << endl;//元素的平均数
	cout << "Here is m5.minCoeff():      " << m5.minCoeff() << endl;//最小元素
	cout << "Here is m5.maxCoeff():      " << m5.maxCoeff() << endl;//最大元素
	cout << "Here is m5.trace():         " << m5.trace() << endl;//迹（对角元素之和)
	cout << "===============next section===============================================" << endl << endl;

当前矩阵的行数、列数、大小可以通过rows()，cols()和size()来获取，上面用“=”改变矩阵大小的方式除此之外，对于动态矩阵可以通过resize()函数来动态修改矩阵的大小.
需注意：
(1) 固定大小的矩阵是不能使用resize（）来修改矩阵的大小；
(2) resize（）函数会析构掉原来的数据，因此调用resize（）函数之后将不能保证元素的值不改变。

//==================================== 矩阵改变大小============================================
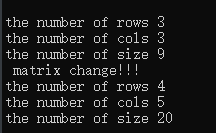
MatrixXd m6(3,3);
	cout << " the number of rows " << m6.rows() << endl;
	cout << " the number of cols " << m6.cols() << endl;
	cout << " the number of size " << m6.size() << endl;
	m6.resize(4, 5);
	cout << "matrix change!!!" << endl;
	cout << " the number of rows " << m6.rows() << endl;
	cout << " the number of cols " << m6.cols() << endl;
	cout << " the number of size " << m6.size() << endl;

参考资料

• http://www.360doc.com/content/20/1230/15/277688_954383797.shtml
• https://blog.csdn.net/thlzzz/article/details/110451022
• https://blog.csdn.net/txwtech/article/details/126090883